27.9: Ion-Cyclotron Resonance
- Page ID
- 22391
A gaseous ion in a magnetic field moves in a circular orbit with an angular frequency \(\omega_c\) such that \(\omega_c = \left( e/m \right) \left( H_0/c \right)\), in which \(e/m\) is the ratio of charge to mass, \(H_0\) is the applied magnetic field, and \(c\) is the velocity of light. The frequency \(\omega_c\) is called the "cyclotron frequency" and is the basis of the cyclotron particle accelerator used in nuclear physics. Now suppose a radio-frequency field is imposed on the ions from a variable oscillator, as shown in Figure 27-15. When the frequency of the oscillator \(\omega\) equals \(\omega_c\), the ions absorb energy and move faster through larger orbits, but at the same frequency \(\omega_c\).
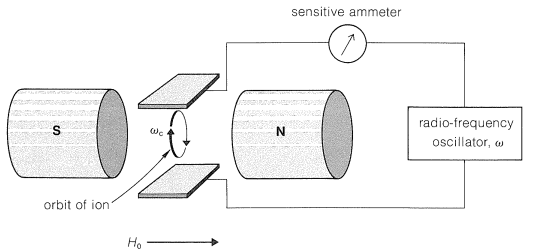
Ion-cyclotron resonance combines features of mass spectroscopy in that the ratio \(e/m\) is involved, and of NMR spectroscopy in that detection depends on absorption of energy from a radio-frequency oscillator. The chemical applications depend on reactions between the ions during the time they remain in the cyclotron, which may be many seconds. Suppose then that we generate \(\ce{OH}^\ominus\) by electron bombardment of a gaseous mixture of water and 2-methyl-2-propanol (tert-butyl alcohol). The \(\ce{OH}^\ominus\) ion can be detected by its characteristic frequency \(\omega = \left( e/m \right) \left( H_0/c \right)\), in which \(e/m = 1/17\). Now, because the reaction \(\ce{(CH_3)_3COH} + \ce{OH}^\ominus \rightarrow \ce{(CH_3)_3CO}^\ominus + \ce{H_2O}\) occurs, a new ion of \(e/m = 1/73\) appears. The reverse reaction, \(\ce{(CH_3)_3CO}^\ominus + \ce{H_2O} \rightarrow \ce{(CH_3)_3COH} + \ce{OH}^\ominus\), does not occur to a measurable extent. From this we can infer that \(\ce{(CH_3)_3COH}\) is a stronger acid than \(\ce{H_2O}\) in the gas phase. These experiments clearly are related to chemical-ionization mass spectroscopy (Section 27-7), and provide the basis for determining the gas-phase acidities of alkynes and water, discussed in Section 11-8. A detailed gas-phase acidity scale has been established by this means.
Many unusual reactions occur between ions and neutral molecules in the gas phase, which can be detected by ion-cyclotron resonance; a few examples are
\[\ce{CH_3F^+} \: \text{(from electron impact)} + \ce{CH_3F} \rightarrow \ce{CH_3} \overset{+}{\ce{F}} \ce{H} + \cdot \ce{CH_2F} \: \text{(} \ce{H} \cdot \: \text{atom transfer)}\]
\[\ce{CH_3FH^+} + \ce{N_2} \rightarrow \ce{CH_3N_2^+} + \ce{HF} \: \: \: \: \: \text{(nucleophilic displacement)}\]
\[\ce{CH_3FH^+} + \ce{Xe} \rightarrow \ce{CH_3Xe^+} + \ce{HF} \: \: \: \: \: \text{(nucleophilic displacement)}\]
Clearly, in gas-phase reactions \(\ce{HF}\) is an extremely good leaving group in being rapidly displaced both by \(\ce{Xe}\) and \(\ce{N_2}\). From our discussions of leaving groups in Section 8-7C, we can infer that \(\ce{H_2F}^\oplus\) must be a very strong acid in the gas phase and the available evidence indicates that this is so.
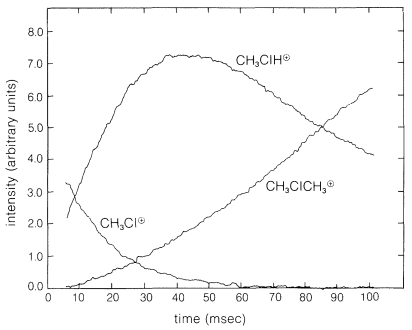
It is possible to measure the concentrations of the ions as a function of time and thus determine the rates of reaction of ions with neutral molecules in the gas phase. Figure 27-16 shows the results of a typical experiment wherein a sequence of reactions occurs that involves chloromethane as the neutral molecule and begins with the ion \(\ce{CH_3Cl}^\oplus\) formed by a short burst \(\left( 10 \: \text{msec} \right)\) of \(16 \: \text{KeV}\) electrons. The originally formed \(\ce{CH_3Cl}^\oplus\) ions react with \(\ce{CH_3Cl}\) to yield \(\ce{CH_3ClH}^\oplus + \cdot \ce{CH_2Cl}\). The buildup of \(\ce{CH_3ClH}^\oplus\) and the disappearance of \(\ce{CH_3Cl}^\oplus\) clearly are coupled. A slower reaction, \(\ce{CH_3ClH}^\oplus + \ce{CH_3Cl} \rightarrow \ce{(CH_3)_2Cl}^\oplus + \ce{HCl}\), then takes over the action.
Contributors and Attributions
John D. Robert and Marjorie C. Caserio (1977) Basic Principles of Organic Chemistry, second edition. W. A. Benjamin, Inc. , Menlo Park, CA. ISBN 0-8053-8329-8. This content is copyrighted under the following conditions, "You are granted permission for individual, educational, research and non-commercial reproduction, distribution, display and performance of this work in any format."


