8.1: Oxidation and Reduction - Four Views
- Page ID
- 152182
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)- Define oxidation and reduction.
- Assign oxidation numbers to atoms in simple compounds.
- Recognize a reaction as an oxidation-reduction reaction.
Oxygen is an element that has been known for centuries. In its pure elemental form, oxygen is highly reactive, and it readily makes compounds with most other elements. It is also the most abundant element by mass in the Earth's crust. The class of reactions called oxidation and reduction were originally defined with respect to the element oxygen.
Many elements simply combine with oxygen to form the oxide of that element. Heating magnesium in air allows it to combine with oxygen to form magnesium oxide.
\[2 \ce{Mg} \left( s \right) + \ce{O_2} \left( g \right) \rightarrow 2 \ce{MgO} \left( s \right) \nonumber \]
Many compounds react with oxygen as well, often in very exothermic processes that are generally referred to as combustion reactions. For example, when methane burns, carbon dioxide and water are produced.
\[\ce{CH_4} \left( g \right) + 2 \ce{O_2} \left( g \right) \rightarrow \ce{CO_2} \left( g \right) + 2 \ce{H_2O} \left( g \right) \nonumber \]
Carbon dioxide is an oxide of carbon, while water is an oxide of hydrogen. Early scientists viewed oxidation as a process in which a substance was reacted with oxygen to produce one or more oxides. In the previous examples, magnesium and methane are being oxidized.
The definitions of oxidation and reduction were eventually broadened to include similar types of reactions that do not necessarily involve oxygen. Oxygen is more electronegative than any element except for fluorine. Therefore, when oxygen is bonded to any element other than fluorine, electrons from the other atom are shifted away from that atom and toward the oxygen atom. An oxidation-reduction reaction (sometimes abbreviated as a redox reaction) is a reaction that involves the full or partial transfer of electrons from one reactant to another. Oxidation involves a full or partial loss of electrons, while reduction involves a full or partial gain of electrons.
Redox reactions require that we keep track of the electrons assigned to each atom in a chemical reaction. How do we do that? We use oxidation numbers to keep track of electrons in atoms. Oxidation numbers are assigned to atoms based on four rules. Oxidation numbers are not necessarily equal to the charge on the atom (although sometimes they can be); we must keep the concepts of charge and oxidation numbers separate.
Assigning Oxidation Numbers
The oxidation number is a positive or negative number that is assigned to an atom to indicate its degree of oxidation or reduction. In oxidation-reduction processes, the driving force for chemical change is in the exchange of electrons between chemical species. A series of rules have been developed to help us.
The rules for assigning oxidation numbers to atoms are as follows:
- Atoms in their elemental state are assigned an oxidation number of 0.
Examples: \(\ce{H_2}\), \(\ce{Br_2}\), \(\ce{Na}\), \(\ce{Be}\), \(\ce{K}\), \(\ce{O_2}\), \(\ce{P_4}\), all have oxidation number of 0. - Atoms in monatomic (i.e., one-atom) ions are assigned an oxidation number equal to their charge.
Example: In MgCl2, magnesium has an oxidation number of +2, while each chlorine atom has an oxidation number of −1 - In compounds, fluorine is assigned a −1 oxidation number; oxygen is usually assigned a −2 oxidation number [except in peroxide compounds (where it is −1) and in binary compounds with fluorine (where it is positive)]; and hydrogen is usually assigned a +1 oxidation number [except when it exists as the hydride ion (H−), in which case rule 2 prevails].
Example: In H2O, the H atoms each have an oxidation number of +1, while the O atom has an oxidation number of −2, even though hydrogen and oxygen do not exist as ions in this compound. - In compounds, all other atoms are assigned an oxidation number so that the sum of the oxidation numbers on all the atoms in the species equals the charge on the species (which is zero if the species is neutral).
Example: In SO2. Each O atom has an oxidation number of −2; for the sum of the oxidation numbers to equal the charge on the species (which is zero), the S atom is assigned an oxidation number of +4. Does this mean that the sulfur atom has a 4+ charge on it? No, it means only that the S atom is assigned a +4 oxidation number by our rules of apportioning electrons among the atoms in a compound.
Oxidation numbers are usually written with the sign first, then the magnitude, to differentiate them from charges.
Assign oxidation numbers to the atoms in each substance.
- Cl2
- GeO2
- CaCl2
Solution
- Cl2 is the elemental form of chlorine. Rule 1 states each atom has an oxidation number of 0.
- By rule 3, oxygen is normally assigned an oxidation number of −2. For the sum of the oxidation numbers to equal the charge on the species (zero), the Ge atom is assigned an oxidation number of +4.
- The Ca2+ ion has an oxidation number of +2 by rule 2. Also according to rule 2, each chlorine atom is assigned an oxidation number of −1.
Assign oxidation numbers to the atoms in H3PO4.
- Answer
-
H: +1; O: −2; P: +5
Oxidation and Reduction Reactions
The processes of oxidation and reduction are commonly viewed in terms of the gain or loss of electrons or the increase or decrease in oxidation numbers. However, many reactions in organic chemistry that involve the gain or loss of oxygen or hydrogen are also considered as redox reactions.
The table below summarizes the processes of oxidation and reduction.
| Oxidation | Reduction |
|---|---|
| Complete loss of electrons (ionic reaction) | Complete gain of electrons (ionic reaction) |
| Increase in oxidation number | Decrease in oxidation number |
| Gain of oxygen | Loss of oxygen |
| Loss of hydrogen in a molecular compound | Gain of hydrogen in a molecular compound |
1. Oxidation is complete loss of electrons. Reduction is complete gain of electrons
To understand electron-transfer reactions like the one between zinc metal and hydrogen ions, chemists separate them into two parts: one part focuses on the loss of electrons, and one part focuses on the gain of electrons. The loss of electrons is called oxidation. The gain of electrons is called reduction.
Electrons that are lost are written as products; electrons that are gained are written as reactants. For example, the reaction below represents when acid is added to zinc metal.
\[\ce{Zn (s) + 2H^{+} (aq) → Zn^{2+}(aq) + H2(g)} \nonumber \]
zinc atoms are oxidized to Zn2+. The half reaction for the oxidation reaction, omitting phase labels, is as follows:
\[\ce{Zn → Zn^{2+} + 2e^{−}} \nonumber \]
This half reaction is balanced in terms of the number of zinc atoms, and it also shows the two electrons that are needed as products to account for the zinc atom losing two negative charges to become a 2+ ion. With half reactions, there is one more item to balance: the overall charge on each side of the reaction. If you check each side of this reaction, you will note that both sides have a zero net charge.
Hydrogen is reduced in the reaction. The balanced reduction half reaction is as follows:
\[\ce{2H^{+} + 2e^{−} → H2} \nonumber \]
There are two hydrogen atoms on each side, and the two electrons written as reactants serve to neutralize the 2+ charge on the reactant hydrogen ions. Again, the overall charge on both sides is zero.
LEO stands for Losing Electrons is Oxidation, while GER stands for Gaining Electrons is Reduction.
This guy knows his redox reactions.
2. Oxidation is an increase in oxidation number. Reduction is a decrease in oxidation number.
Consider the reaction below between elemental iron and copper sulfate:
\[\ce{Fe} + \ce{CuSO_4} \rightarrow \ce{FeSO_4} + \ce{Cu} \nonumber \]
In the course of the reaction, the oxidation number of \(\ce{Fe}\) increases from zero to \(+2\). The oxidation number of copper decreases from \(+2\) to \(0\). This result is in accordance with the activity series. Iron is above copper in the series, so will be more likely to form \(\ce{Fe^{2+}}\) while converting the \(\ce{Cu^{2+}}\) to metallic copper \(\left( \ce{Cu^0} \right)\).
3. Oxidation is gain of oxygen. Reduction is loss of oxygen.
Oxidation and reduction can also be defined in terms of changes in composition. The original meaning of oxidation was “adding oxygen,” so when oxygen is added to a molecule, the molecule is being oxidized. The reverse is true for reduction: if a molecule loses oxygen atoms, the molecule is being reduced. For example, the acetaldehyde (\(CH3CHO\)) molecule takes on an oxygen atom to become acetic acid (\(\ce{CH3COOH}\)).
\[\ce{2CH3CHO + O2 → 2CH_3COOH} \nonumber \]
Thus, acetaldehyde is being oxidized.
4. Oxidation is loss of hydrogen in a molecular compound. Reduction is gain of hydrogen in a molecular compound.
Oxidation and reduction can be defined in terms of the gain or loss of hydrogen atoms. If a molecule adds hydrogen atoms, it is being reduced. If a molecule loses hydrogen atoms, the molecule is being oxidized. For example, in the conversion of acetaldehyde into ethanol CH3CHO, hydrogen atoms are added to acetaldehyde, so the acetaldehyde is being reduced:
\[\ce{CH3CHO + H2 → CH3CH2OH} \nonumber \]
In each conversion, indicate whether oxidation or reduction is occurring.
- N2 → NH3
- CH3CH2OHCH3 → CH3COCH3
- HCHO → HCOOH
Solution
- Hydrogen is being added to the original reactant molecule, so reduction is occurring.
- Hydrogen is being removed from the original reactant molecule, so oxidation is occurring.
- Oxygen is being added to the original reactant molecule, so oxidation is occurring.
In each conversion, indicate whether oxidation or reduction is occurring.
- CH4 → CO2 + H2O
- NO2 → N2
- CH2=CH2 → CH3CH3
Answers
- oxidation
- reduction
- reduction
Identify what is being oxidized and reduced in this redox reaction.
\[\ce{2Na + Br2 → 2NaBr} \nonumber \]Solution
Both reactants are the elemental forms of their atoms, so the Na and Br atoms have oxidation numbers of 0. In the ionic product, the Na+ ions have an oxidation number of +1, while the Br− ions have an oxidation number of −1.
\[2\underset{0}{Na}+\underset{0}{Br_{2}}\rightarrow 2\underset{+1 -1}{NaBr} \nonumber \]
Sodium is increasing its oxidation number from 0 to +1, so it is being oxidized; bromine is decreasing its oxidation number from 0 to −1, so it is being reduced:
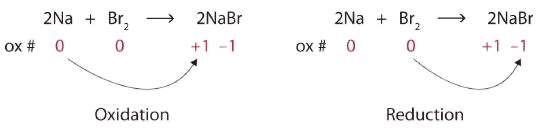
Because oxidation numbers are changing, this is a redox reaction. The total number of electrons being lost by sodium (two, one lost from each Na atom) is gained by bromine (two, one gained for each Br atom).
Identify what is being oxidized and reduced in this redox reaction.
\[\ce{C + O2 → CO2}\nonumber \]
- Answer
-
C is being oxidized from 0 to +4; O is being reduced from 0 to −2.
For each half reaction, identify if there was a loss or gain of electrons.
- 2N3- → N2
- Cr6+ → Cr3+
Solutions
a. When we compare the overall charges on each side of the equation, we find a charge of 2(-3)=-6 on the left but a charge of 0 on the right. This equation is not properly balanced. To balance it, six electrons must be added on the right side as follows:
\[\ce{2N^{3-} → N2 + 6e^{-}}\nonumber \]
So there was a loss of six electrons. Now the equation is balanced, not only in terms of elements but also in terms of charge.
b. When we compare the overall charges on each side of the equation, we find a charge of +6 on the left and a charge of +3 on the right. This equation is not properly balanced. To end up with an oxidation number of +3 on the right side 3 electrons must be added on the left side as follows:
\[\ce{Cr^{6+} + 3e^{-} → Cr^{3+}\nonumber \]
So there was a gain of three electrons. Now the equation is balanced in terms of charge.
For each half reaction, identify if there was a loss or gain of electrons.
- O2 → 2O2-
- Cu+ → Cu2+
Answers
a. gain of four electrons
b. loss of one electron
Write and balance the redox reaction that has silver ions and aluminum metal as reactants and silver metal and aluminum ions as products.
Solution
We start by using symbols of the elements and ions to represent the reaction:
\[\ce{Ag^{+} + Al → Ag + Al^{3+}} \nonumber \]
The equation looks balanced as it is written. However, when we compare the overall charges on each side of the equation, we find a charge of +1 on the left but a charge of +3 on the right. This equation is not properly balanced. To balance it, let us write the two half reactions. Silver ions are reduced, and it takes one electron to change Ag+ to Ag:
\[\ce{Ag^{+} + e^{−} → Ag} \nonumber \]
Aluminum is oxidized, losing three electrons to change from Al to Al3+:
\[\ce{Al → Al^{3+} + 3e^{−}} \nonumber \]
To combine these two half reactions and cancel out all the electrons, we need to multiply the silver reduction reaction by 3:
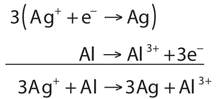
Now the equation is balanced, not only in terms of elements but also in terms of charge.
Write and balance the redox reaction that has calcium ions and potassium metal as reactants and calcium metal and potassium ions as products.
Summary
- Rules for determining oxidation numbers are listed.
- Examples of oxidation number determinations are provided.
- Chemical reactions in which electrons are transferred are called oxidation-reduction, or redox, reactions.
- Oxidation is defined in terms of the loss of electrons, gain of oxygen, loss of hydrogen, or an increase in oxidation number.
- Reduction is defined in terms of gain of electrons, loss of oxygen, gain of hydrogen, or a decrease in oxidation number.
- Oxidation and reduction always occur together, even though they can be written as separate chemical equations.
Contributors and Attributions
- Libretext: Beginning Chemistry (Ball et al.)
- Libretext: The Basics of GOB Chemistry (Ball et al.)
- Libretext: Chemistry for Allied Health (Soult)
Ed Vitz (Kutztown University), John W. Moore (UW-Madison), Justin Shorb (Hope College), Xavier Prat-Resina (University of Minnesota Rochester), Tim Wendorff, and Adam Hahn.

