6.13E: Madelung Constants
- Page ID
- 33354
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)There are many factors to be considered such as covalent character and electron-electron interactions in ionic solids. But for simplicity, let us consider the ionic solids as a collection of positive and negative ions. In this simple view, appropriate number of cations and anions come together to form a solid. The positive ions experience both attraction and repulsion from ions of opposite charge and ions of the same charge. The Madelung constant is a property of the crystal structure and depends on the lattice parameters, anion-cation distances, and molecular volume of the crystal.
1D Crystal
Before considering a three-dimensional crystal lattice, we shall discuss the calculation of the energetics of a linear chain of ions of alternate signs (Figure \(\PageIndex{1}\)).
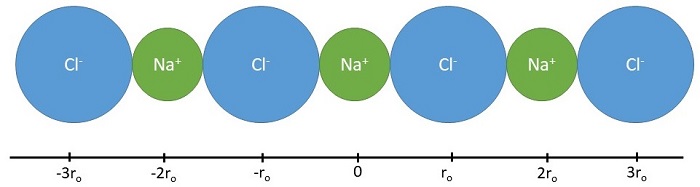
Let us select the positive sodium ion in the middle (at \(x=0\)) as a reference and let \(r_0\) be the shortest distance between adjacent ions (the sum of ionic radii). The Coulomb energy of the other ions in this 1D lattice on this sodium atom can be decomposed by proximity (or "shells").
- Nearest Neighbors (first shell): This reference sodium ion has two negative chloride ions as its neighbors on either side at \(\pm r_0\) so the Coulombic energy of these interactions is \[ \underbrace{ \dfrac{-e^2}{4 \pi \epsilon_o r_o}}_{\text{left chloride ion}} + \underbrace{ \dfrac{-e^2}{4 \pi \epsilon_o r_o}}_{\text{right chloride ion}} = - \dfrac{2e^2}{4 \pi \epsilon_o r_o} \label{eq1}\]
- Next Nearest Neighbors (second shell): Similarly the repulsive energy due to the next two positive sodium ions at a distance of \(2r_0\) is \[ \underbrace{ \dfrac{+e^2}{4 \pi \epsilon_o (2r_o)}}_{\text{left sodium ion}} + \underbrace{ \dfrac{+e^2}{4 \pi \epsilon_o (2r_o)}}_{\text{right sodium ion}} = + \dfrac{2e^2}{4 \pi \epsilon_o (2r_o)} \label{eq2}\]
- Next Next Nearest Neighbors (third shell): The attractive Coulomb energy due to the next two chloride ions neighbors at a distance \(3r_0\) is \[ \underbrace{ \dfrac{-e^2}{4 \pi \epsilon_o (3r_o)}}_{\text{left chloride ion}} + \underbrace{ \dfrac{-e^2}{4 \pi \epsilon_o (3r_o)}}_{\text{right chloride ion}} = - \dfrac{2e^2}{4 \pi \epsilon_o (3r_o)} \label{eq3}\]
and so on. Thus the total energy due to all the ions in the linear array is
\[ E = - \dfrac{2e^2}{4 \pi \epsilon_o r_o} + \dfrac{2e^2}{4 \pi \epsilon_o (2r_o)} - \dfrac{2e^2}{4 \pi \epsilon_o (3r_o)} - \ldots\]
or
\[ E= \dfrac{e^2}{4 \pi \epsilon_o r_o} \left[ 2 \left (1 -\dfrac{1}{2} + \dfrac{1}{3} - \dfrac{1}{4} + \ldots \right) \right] \label{eq6}\]
We can use the following Maclaurin expansion
\[ \ln(1+x)=x-\frac{x^2}{2}+\frac{x^3}{3}- \frac{x^3}{4} + \cdots\]
to simplify the sum in the parenthesis of Equation \ref{eq6} as \(\ln (1+ 1)\) to obtain
\[ \begin{align} E &= \dfrac{e^2}{4 \pi \epsilon_o r_o} \left[ 2 \ln 2 \right] \label{eq7} \\[4pt] &= \dfrac{e^2}{4 \pi \epsilon_o } M \end{align} \]
The first factor of Equation \ref{eq7} is the Coulomb energy for a single pair of sodium and chloride ions, while the \(2 \ln 2\) factor is the Madelung constant (\(M \approx 1.38 \)) per molecule. The Madelung constant is named after Erwin Medelung and is a geometrical factor that depends on the arrangement of ions in the solid. If the lattice were different (when considering 2D or 3D crystals), then this constant would naturally differ.
3D Crystal
In three dimensions the series does present greater difficulty and it is not possible to sum the series conveniently as in the case of one-dimensional lattice. As an example, let us consider the the \(\ce{NaCl}\) crystal. In the following discussion, assume \(r\) be the distance between \(\ce{Na^{+}}\) and \(\ce{Cl^-}\) ions. The nearest neighbors of \(\ce{Na^{+}}\) are six \(\ce{Cl^-}\) ions at a distance 1r, 12 \(\ce{Na^{+}}\) ions at a distance 2r, eight \(\ce{Cl^-}\) ions at 3r, six \(\ce{Na^{+}}\) ions at 4r, 24 \(\ce{Na^{+}}\) ions at 5r, and so on. Thus, the electrostatic potential of a single ion in a crystal by approximating the ions by point charges of the surrounding ions:
\[ E_{ion-lattice} = \dfrac{Z^2e^2}{4\pi\epsilon_or} M \label{12.5.4}\]
For NaCl is a poorly converging series of interaction energies:
\[ M= \dfrac{6}{1} - \dfrac{12}{2} + \dfrac{8}{3} - \dfrac{6}{4} + \dfrac{24}{5} ... \label{21.5.5}\]
with
- \(Z\) is the number of charges of the ions, (e.g., 1 for NaCl),
- \(e\) is the charge of an electron (\(1.6022 \times 10^{-19}\; C\)),
- \(4\pi \epsilon_o\) is 1.11265x10-10 C2/(J m).
The Madelung constant depends on the structure type and Equation \(\ref{21.5.5}\) is applicable only for the sodium chloride (ei.g, rock salt) lattice geometry. Other values for other structural types are given in Table \(\PageIndex{2}\). \(A\) is the number of anions coordinated to cation and \(C\) is the numbers of cations coordinated to anion.
|
|
|
|
A : C | Type |
|---|---|---|---|---|
| NaCl | NaCl | 1.74756 | 6 : 6 | Rock salt |
| CsCl | CsCl | 1.76267 | 6 : 6 | CsCl type |
| CaF2 | Cubic | 2.51939 | 8 : 4 | Fluorite |
| CdCl2 | Hexagonal | 2.244 | ||
| MgF2 | Tetragonal | 2.381 | ||
| ZnS (wurtzite) | Hexagonal | 1.64132 | ||
| TiO2 (rutile) | Tetragonal | 2.408 | 6 : 3 | Rutile |
| bSiO2 | Hexagonal | 2.2197 | ||
| Al2O3 | Rhombohedral | 4.1719 | 6 : 4 | Corundum |
|
A is the number of anions coordinated to cation and C is the numbers of cations coordinated to anion. |
||||
There are other factors to consider for the evaluation of lattice energy and the treatment by Max Born and Alfred Lande led to the formula for the evaluation of lattice energy for a mole of crystalline solid. The Born–Landé equation (Equation \(\ref{21.5.6}\)) is a means of calculating the lattice energy of a crystalline ionic compound and derived from the electrostatic potential of the ionic lattice and a repulsive potential energy term
\[ U= \dfrac{N_A M Z^2e^2}{4\pi \epsilon_o r} \left( 1 - \dfrac{1}{n} \right) \label{21.5.6}\]
where
- \(N_A\) is Avogadro constant;
- \(M\) is the Madelung constant for the lattice
- \(z^+\) is the charge number of cation
- \(z^−\) is the charge number of anion
- \(e\) is elementary charge, 1.6022×10−19 C
- \(ε_0\) is the permittivity of free space
- \(r_0\) is the distance to closest ion
- \(n\) is the Born exponent that is typically between 5 and 12 and is determined experimentally. \(n\) is a number related to the electronic configurations of the ions involved (Table \(\PageIndex{3}\)).
| Atom/Molecule | n |
|---|---|
| He | 5 |
| Ne | 7 |
| Ar | 9 |
| Kr | 10 |
| Xe | 12 |
| LiF | 5.9 |
| LiCl | 8.0 |
| LiBr | 8.7 |
| NaCl | 9.1 |
| NaBr | 9.5 |
Estimate the lattice energy for \(\ce{NaCl}\).
Solution
Using the values giving in the discussion above, the estimation is given by
\[\begin{align*} U_{NaCl} &= \dfrac{(6.022 \times 10^{23} /mol) (1.74756 ) (1.6022 \times 10 ^{-19})^2 (1.747558)}{ 4\pi \, (8.854 \times 10^{-12} C^2/m ) (282 \times 10^{-12}\; m)} \left( 1 - \dfrac{1}{9.1} \right) \nonumber \\[4pt] &= - 756 \,kJ/mol\nonumber \end{align*} \nonumber\]
Much more should be considered in order to evaluate the lattice energy accurately, but the above calculation leads you to a good start. When methods to evaluate the energy of crystallization or lattice energy lead to reliable values, these values can be used in the Born-Hable cycle to evaluate other chemical properties, for example the electron affinity, which is really difficult to determine directly by experiment.

