12.2.3: Mechanistic Possibilities
- Page ID
- 385532
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)A mechanism is the sequence of elementary steps by which a reaction proceeds. There are two aspects that describe the mechanism of a substitution reaction. One is called the stoichiometric mechanism, and the other one is called the intimate mechanism. The stoichiometric part of the mechanism is defined by the identity of the intermediate, or lack thereof. The intimate part of the mechanism is defined by the rate limiting step, and how the rate is dependent on the identity of the incoming ligand.
Stoichiometric Mechanisms
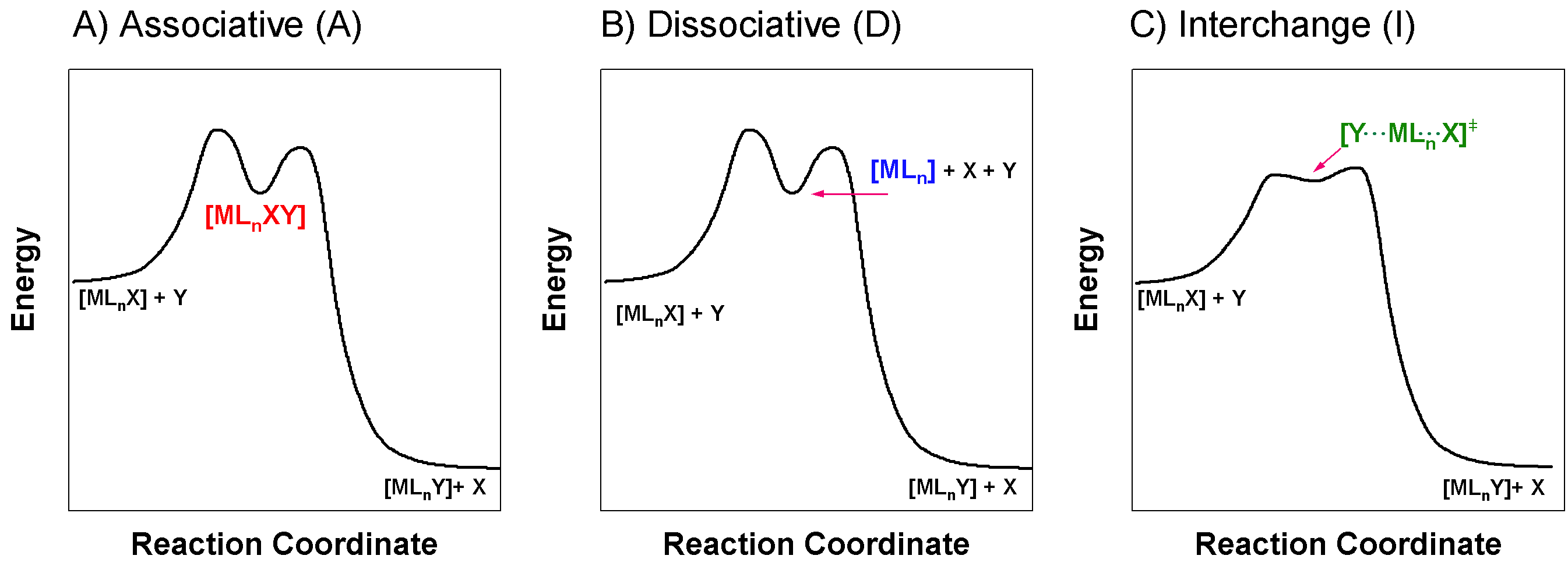
The stoichiometric part of the mechanism is defined by the identity of the intermediate, or lack thereof. There are three classes of stoichiometric mechanisms based on the type of intermediates that can be characterized. If the intermediate can be isolated it is either an Associative (higher-coordination number intermediate) or Dissociative (lower-coordination number intermediate) reaction. On the other hand, if the intermediate cannot be isolated because it is short-lived (or does not exist) the reaction is classified as Interchange.
Associative (A) Mechanism
Associative (A) mechanisms involve a first step where the incoming ligand bonds to the metal ion, creating an intermediate with a higher coordination number. The leaving group leaves in a second step to form the product. The generic reaction below illustrates the steps of an associative mechanism. Note that the intermediate, \(\color{red}{\ce{ML_{n}XY}}\), has a higher coordination number than that of the reactant, \(\ce{ML_{n}X}\).
\[\begin{array}{rc}
\text{Overall Reaction:} & \ce{ML_{n}X + Y <=> ML_{n}Y + X} \\
\hline \text{Step 1 (Association):} & \ce{ML_{n}X + Y <=>} \color{red}{\ce{ML_{n}XY}}\\
\text{Step 2 (Dissociation):} & \textcolor{red}{\ce{ML_{n}XY}} \ce{<=> ML_{n}Y + X}
\end{array} \nonumber \]
Associative mechanisms are typical of square planar, \(d^8\) complexes. The important features that distinguish this mechanism from others is that the intermediate is long-lived enough that it is detectable, and that the intermediate has a higher coordination number than the reactant complex.
Dissociative (D) Mechanism
Dissociative (D) mechanisms involve a first step where a bond between the metal ion and the leaving group breaks, creating an intermediate with a lower coordination number. The entering group enters in a second step to form the product. The generic reaction below illustrates the steps of a dissociative mechanism. Note that the intermediate, \(\color{blue}{\ce{ML_{n}}}\), has a lower coordination number than that of the reactant, \(\ce{ML_{n}X}\).
\[\begin{array}{rc}
\text{Overall Reaction:} & \ce{ML_{n}X + Y <=> ML_{n}Y + X} \\
\hline \text{Step 1 (Dissociation):} & \ce{ML_{n}X <=>}\textcolor{blue}{\ce{ML_{n}}}\ce{ + X}\\
\text{Step 2 (Association):} & \textcolor{blue}{\ce{ML_{n}}}\ce{ + Y <=> ML_{n}Y}
\end{array} \nonumber \]
The important features that distinguish this mechanism from others is that the intermediate is long-lived enough that it is detectable, and that the intermediate has a lower coordination number than the reactant complex.
Interchange (I) Mechanism
Interchange (I) Mechanisms take place in one concerted step where the entering group enters as the leaving group leaves. Bond formation and bond breaking occur simultaneously. In the case of an interchange mechanism, no intermediate is detectable. This means that either there is no intermediate, or that the intermediate is too high-energy and short-lived to be detected. The generic reaction below illustrates the single step of an interchange mechanism. Note that the species, \(\color{green}{\ce{[Y\bond{...}ML_{n}\bond{...}X]^{\ddagger}}}\), can be defined as either a transition state or a very short-lived intermediate. The distinction between these two possibilities is difficult; when the intermediate is undetectable it is not considered a true intermediate.
\[\begin{array}{rc}
\text{Overall Reaction:} & \ce{ML_{n}X + Y <=> ML_{n}Y + X} \\
\hline \text{Step 1 (Concerted):} & \ce{ML_{n}X + Y <=>}\textcolor{green}{ \ce{[Y\bond{...}ML_{n}\bond{...}X]^{\ddagger}}} \ce{+ <=> ML_{n}Y + X}
\end{array} \nonumber \]
The interchange mechanism is common for many six-coordinate (octahedral) metal complexes. The hallmark feature that distinguishes the interchange mechanism from other possible mechanisms is the absence of a detectable intermediate. If an intermediate is detected, the mechanism is considered associative (A). Another piece of evidence that can indicate an interchange mechanism is stereochemical changes from reactant to product. If specific sterochemistry (ie cis or trans relationships) is changed, it may be taken as evidence that an intermediate exists long enough to allow rearrangement to occur.
Distinguishing A, D, and I Mechanisms
Figure \(\PageIndex{1}\) shows examples of possible reaction profiles for each of the stoichiometric mechanisms described above. The profile of A and D mechanisms can be similar; they each require an intermediate, and the primary difference between A and D is the sequence of steps. An I mechanism lacks a true intermediate. These three cases can be difficult to distinguish experimentally, and especially if characterization of an intermediate is difficult. Notice that the reaction coordinate diagram for A and D pathways, shown in Figure \(\PageIndex{1}\), are similar. The only necessary difference between them is in the identity of the intermediate species.
Intimate Mechanisms
The intimate mechanism is defined by characteristics of the rate-limiting step. The reaction is classified as associatively-activated (using a subscript, \(a\)) if the rate changes by more than 10-fold as the entering group is varied. The reaction is classified as dissociatively-activated (using a subscript, \(d\)) if the rate changes by less than 10-fold as the entering group is varied.
Associatively-Activated Mechanisms
The rate-limiting step of an associatively activated pathway is formation of a bond between the entering group and the central metal ion. These reactions also require formation of an encounter complex in a pre-equilibrium step prior to bond forming or bond breaking steps discussed here (see Eigen-Wilkins Mechanism).
Associatively-activated A and I (\(\ce{A_{a}}\) and \(\ce{I_{a}}\))
In the case of an associatively-activated associative pathway (\(\bf\ce{A_{a}}\)) or an associatively-activated interchange pathway (\(\bf\ce{I_{a}}\)), the rate-limiting step is the association of the entering group (Y) with the reactant, \(\ce{ML_{n}X}\). In the case of the \(\ce{A_{a}}\) pathway, it is the first step (bolded) below.
\[\begin{array}{rcc}
& \ce{A_{a}} \text{ Mechanism} & \\
\hline \textbf{Step 1 (Association):} & \bf \ce{ML_{n}X + Y <=>[k_1][k_{-1}] ML_{n}XY} & \Longleftarrow \textbf{RATE LIMITING ASSOCIATION}\\
\text{Step 2 (Dissociation):} & \ce{ML_{n}XY <=>[k_2][k_{-2}] ML_{n}Y + X}
\end{array} \nonumber \]
In the case of \(\ce{A_{a}}\), the first step shown above is slower than the second step (\( k_1 < k_2\)). In this case, an intermediate may not be detected because its steady state concentration is close to zero (ie it reacts as soon as it forms). Thus, distinguishing between \(\ce{A_{a}}\) and \(\ce{I_{a}}\) mechanisms is often impossible.
Associatively-activated D (\(\ce{D_{a}}\))
In the case of an associatively-activated dissociative reaction (\(\bf\ce{D_{a}}\)), the rate limiting step is the association of the entering group (Y) with the intermediate \(\ce{ML_{n}}\); the second step (bolded) in the mechanism shown below:
\[\begin{array}{rcc}
& \ce{D_{a}} \text{ Mechanism} & \\
\hline \text{Step 1 (Dissociation):} & \ce{ML_{n}X <=>[k_1][k_{-1}] ML_{n} + X} &\\
\textbf{Step 2 (Association):} & \bf \ce{ML_{n}X + Y <=>[k_2][k_{-2}] ML_{n}Y} & \Longleftarrow \textbf{RATE LIMITING ASSOCIATION}
\end{array} \nonumber \]
In this case, the intermediate concentration should be measurable since the second step is slower than the first (\( k_1 > k_2\)).
Draw the reaction coordinate diagrams (aka reaction profiles) of reactions that proceed through \(\ce{A_{a}}\), \(\ce{I_{a}}\), and \(\ce{D_{a}}\) pathways. Use these diagrams to explain why it is impossible to distinguish between \(\ce{A_{a}}\) and \(\ce{I_{a}}\).
- Hint
-
Notice that the reaction coordinate diagrams for A and D pathways, shown in Figure \(\PageIndex{1}\), are similar. The only necessary difference between them is the identity of the intermediate. These diagrams can be modified to represent associatively-activated pathways by altering the relative energy of the transition state for the associative step; the energy barrier for the associative step must be greater than that of the dissociative step. In other words, the difference between \(\ce{A_a}\), \(\ce{D_a}\) is in the relative energies of the first and second transition states. In the case of \(\ce{I_a}\), the diagram could be similar to the I profile shown in Figure \(\PageIndex{1}\), with the exception that the energy barrier for making the M-Y bond is greater than the energy barrier for breaking the M-X bond.
- Answer
-
The three reaction coordinate diagrams shown in the figure below represent \(\ce{A_{a}}\), \(\ce{I_{a}}\), and \(\ce{D_{a}}\) mechanisms respectively. If your diagram does not match this perfectly, that may be OK. The important features are:
- \(\ce{A_{a}}\): This reaction profile should show an intermediate with higher coordination number than the reactant. The intermediate should be relatively stable. The energy barrier for formation of this intermediate is the associative step, and therefore it should also be the rate-limiting step for an \(\ce{A_{a}}\) mechanism; in other words, the first step should have the highest energy barrier.
- \(I_a\): This reaction profile should lack a stable intermediate. The energy barrier for creating of the M-Y bond must be rater-limiting. The diagram shown below is an acceptable solution, but a diagram like shown in Panel C of Figure \(\PageIndex{1}\) is also correct. It is not clear from the diagram alone whether bond breaking or bond making are rate-limiting; this nuance would lie primarily in the identity of the transition state, and whether the M-Y bond is stronger or weaker than the M-X bond in the transition state.
- \(\ce{D_{a}}\): This reaction profile should show an intermediate with lower coordination number than the reactant. The intermediate should be relatively stable. The energy barrier for association of the entering group with the intermediate (the second step) must be rate-limiting in a \(\ce{D_{a}}\) mechanism. In other words, the second step should have the highest energy barrier.
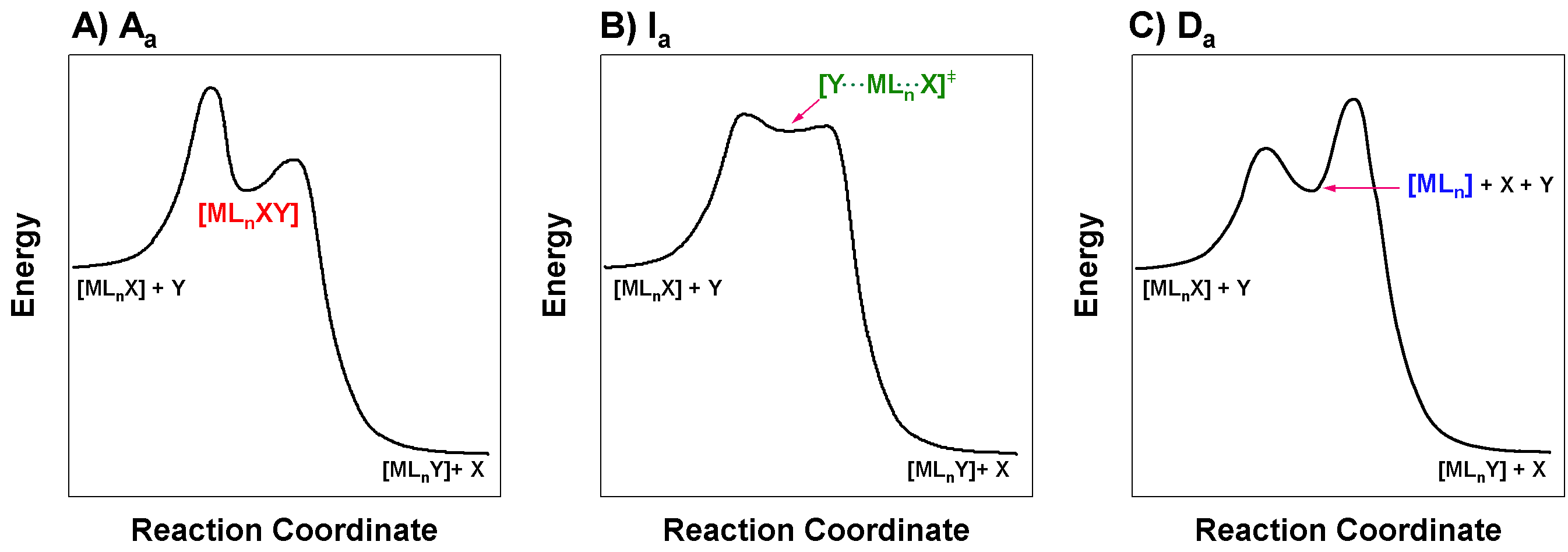
Figure for Excercise \(\PageIndex{1}\): Reaction coordinate diagrams for associatively-activated mechanisms. (A) Associatively-activated associative, \(\ce{A_a}\). (B) Associatively-activated interchange, \(\ce{I_a}\). (C) Associatively-activated dissociative, \(\ce{D_a}\). (CC-BY-SA; Kathryn Haas) The \(\ce{A_{a}}\) and \(I_a\) mechanisms have different reaction profiles while still the rate-limiting step is the initial association of the incoming ligand to the metal center. These two types of mechanisms are difficult to distinguish because in the case of \(\ce{A_{a}}\), there is a relatively small energy barrier for reaction of the intermediate to form product; thus this intermediate is not long-lived and would be difficult to detect. Experimental distinction between \(\ce{A_{a}}\) and \(I_a\) lies in the detection and characterization of this intermediate, and since it may be elusive in \(\ce{A_{a}}\), it is difficult to unambiguously determine whether an associatively-activated mechanism is \(\ce{A_{a}}\) or \(I_a\).
Dissociatively-Activated Mechanisms
When the rate-determining step is the breaking of a bond between the central metal ion and the leaving group, it is considered a dissociatively-activated (subscript \(d\)) mechanism. The rates of these reactions are largely independent of the identity of the incoming ligand.
Dissociatively-activated A (\(\ce{A_{d}}\))
In the case of a dissociatively-activated associative pathway (\(\bf\ce{A_{d}}\)), the rate-limiting step is the dissociation of the leaving group (X) from the intermediate, \(\ce{ML_{n}XY}\); the second step (bolded) below.
\[\begin{array}{rcc}
& \ce{A_{d}} \text{ Mechanism} & \\
\hline \text{Step 1 (Association):} & \ce{ML_{n}X + Y <=>[k_1][k_{-1}] ML_{n}XY} & \\
\textbf{Step 2 (Dissociation):} & \bf \ce{ML_{n}XY <=>[k_2][k_{-2}] ML_{n}Y + X} & \Longleftarrow \textbf{RATE LIMITING DISSOCIATION}
\end{array} \nonumber \]
In this case, the intermediate concentration should be measurable since the second step is slower than the first (\( k_1 > k_2\).
Dissociatively-activated D and I (\(\ce{D_{a}}\) and \(\ce{I_{a}}\))
In the case of a dissociatively-activated dissociative reaction (\(\bf\ce{D_{d}}\)), or a dissociatively-activated interchange pathway (\(\bf\ce{I_{d}}\)), the rate limiting step is the dissociation of the leaving group (X) from the reactant, \(\ce{ML_{n}X}\). For \(\ce{D_{d}}\) the rate limiting step is the first step (bolded) in the mechanism shown below:
\[\begin{array}{rcc}
& \ce{D_{d}} \text{ Mechanism} & \\
\hline \textbf{Step 1 (Dissociation):} & \bf \ce{ML_{n}X <=>[k_1][k_{-1}] ML_{n} + X} & \Longleftarrow \textbf{RATE LIMITING DISSOCIATION} \\
\text{Step 2 (Association):} & \ce{ML_{n}X + Y <=>[k_2][k_{-2}] ML_{n}Y} &
\end{array} \nonumber \]
In the case of \(\ce{D_{d}}\), the first step shown above is slower than the second step (\( k_1 < k_2\) and an intermediate may not be detected because its steady state concentration is close to zero (ie it reacts as soon as it forms). Thus, distinguishing between \(\ce{D_{d}}\) and \(\ce{I_{d}}\) mechanisms is often impossible.
You should complete Excercise \(\PageIndex{1}\) before attempting this excercise.
Draw the reaction coordinate diagrams (aka reaction profiles) of reactions that proceed through \(\ce{A_{d}}\), \(\ce{I_{d}}\), and \(\ce{D_{d}}\) pathways.
- Answer
-
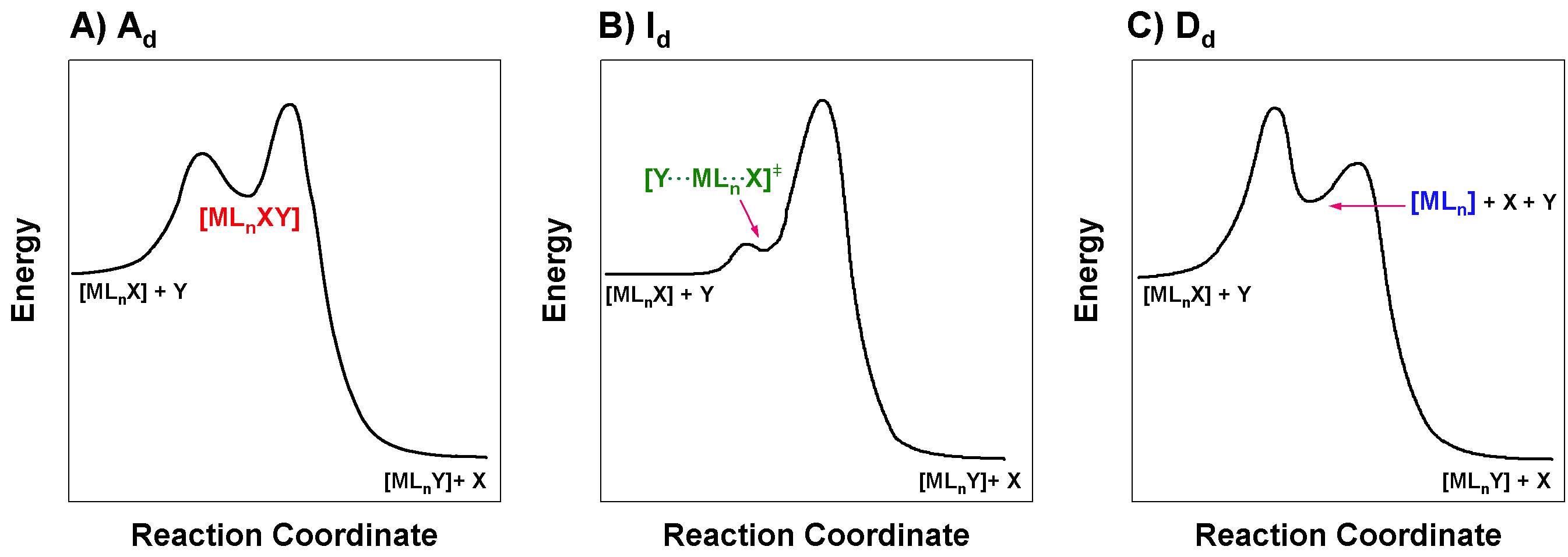
Figure for Excercise \(\PageIndex{2}\): Reaction coordinate diagrams for dissociatively-activated mechanisms. (A) Dissociatively-activated associative, \(\ce{A_d}\). (B) Dissociatively-activated interchange, \(\ce{I_d}\). (C) Dissociatively-activated dissociative, \(\ce{D_d}\). (CC-BY-SA; Kathryn Haas)
Curated or created by Kathryn Haas


