8.6.1: Properties of the Group 13 Elements and Boron Chemistry

As elements, Boron acts like an electron deficient nonmetal and the other group 13 elements like metals that exhibit varying degrees of covalency.

Selected properties of the Group 13 elements are given in Table \(\sf{\PageIndex{1}}\).

Table \(\sf{\PageIndex{1}}\). Selected Properties of Group 13 Elements other than Nihonium. For more information about how this data was sourced see note 1.

Element	Elemental Form	Melting Point (\(\sf{^{\circ}C}\))	Pauling Electronegativity	Ionization Energy (kJ/mol)	Electron Affinity (kJ/mol)	Atomic Radius (Å)	Covalent Radius (Å)
Boron, B	Network Covalent Solid characterized by the sharing of electrons in boron clusters, primarily linked icosahedra, like those depicted schematically below.	2180	2.04	801	27	1.92	0.84
Aluminum or Aluminium,^2Al	FCC Metal	660	1.61	577	42	1.84	1.24
Gallium, Ga	Metal with a nonstandard crystal structure that may be described as packed Ga2 units, which are shown linked by the thicker pink lines in the structure below.	30	1.81	579	41	1.87	1.23
Indium, In	Distorted close packed metal	157	1.78	558	36	1.93	1.42
Thallium, Tl	HCP Metal	304	1.8	589	28.9	1.96	1.44

From the data given in Table \(\sf{\PageIndex{1}}\) several things are apparent:

1. There is a discontinuity between the properties of boron and the group 13 metals in rows 3 and higher.

2. Boron forms covalent compounds characterized by multicenter bonding and cluster formation; the other elements are metals that exhibit varying degrees of covalency.

3. Trends in the properties of the row 3+ group 13 metals are far from simple, likely in part because

• The atomic properties of Ga and In are influenced by the d-block contraction, giving both a relatively high electronegativity, which when combined with their small radii promote stronger covalent interactions.
• The atomic properties of Tl are also influenced by the lanthanide contraction, resulting in an increase in electronegativity and ionization energy relative to In.

4. The interplay between changing covalent and metallic bonding interactions on going from Al to Tl results in a decrease in melting point from Al to Ga followed by an increase in melting point on going from Ga to Tl.

The group 13 elements are prepared by reduction of halides or oxides or, in the case of boron, thermal decomposition of the hydride.

Boron can be prepared by reduction of its halides or high temperature thermal decomposition of its hydrides.

\[\sf{2~BCl_3~~+~~3~H_2~~\longrightarrow~~2~B~~+~~6~HCl} \nonumber \]

\[\sf{B_2H_6~~\overset{\Delta}{\longrightarrow}~~2~B~~+~~3~H_2} \nonumber \]

The group 13 metals are made by electrolytic reduction of the cations in thermally accessible molten salts. The best known example is the production of aluminum in the Hall-Héroult process.

\[\sf{2~Al_2O_3~~+~~3~C(s,~graphite)~~\overset{electrolysis, 940-980^{\circ}C, KF, Na_2AlF_6}{\longrightarrow}~~4~Al(s)~~+~~3~CO_2(g)} \nonumber \]

Boron forms covalent bonds and engages in cluster and/or bridge bonding when there are too few electrons to form enough pairwise bonds to satisfy an octet.

Boron possesses only three valence electrons and can be stablilized by sharing five more. It does this in three main ways.

1. Boron forms ordinary covalent bonds and/or gains electrons to give stable molecules or ions. For example, while the boron of monomeric BH₃ may not have access to enough valence electrons to achieve a stable octet, it can satisfy its octet by forming an adduct with a Lewis base. A well known example is the borohydride anion, BH₄^-.

\[\sf{BH_3~~+~~H^-~~\longrightarrow~~BH_4^-} \nonumber \]

A large number of boron compounds are of this sort. These include

a. The borates and boric acid derivatives which comprise a few ppm of the Earth's crust. These contain borate anions similar to the representative set shown in Scheme \(\sf{\PageIndex{II}}\). Of these, sodium borate or borox is commonly used in household detergents. More precisely, there is a mixture of boraxes, all of which are of formula [Na(H₂O)_x]₂H₂B₄O₉ (often written as Na₂B₄O₇·xH₂O) and differ in the number of waters of hydration. All contain the tetraborate anion, B₄O₅(OH)₄^2-, which has the structure shown at right in Scheme \(\sf{\PageIndex{II}}\)..

Scheme \(\sf{\PageIndex{II}}\). The tetraborate anion.

As illustrated by the structures depicted in Scheme \(\sf{\PageIndex{II}}\), the boron in borates is found in BO₄ tetrahedra and trigonal planar BO₃ units linked together in various ways.

The borates illlustrate the acid-base properties of these conventional boron compounds. Borate anions may be considered as derived from hydrolysis of boric oxide to boric acid followed by acid dissociation.

\[\sf{\underset{boric~oxide}{B_2O_3}~~+~~3~H_2O~~\longrightarrow~~\underset{boric~acid}{2~B(OH)_3}} \nonumber \]

\[\sf{B(OH)_3~~\rightleftharpoons~~H^+~~+~~B(OH)_2O^-} \nonumber \]

\[\sf{B(OH)_3~~\rightleftharpoons~~3~H^+~~+~~BO_3^{3-}} \nonumber \]

In this respect boron oxide, like all metal oxides, is capable of acting as a Brønsted acid, albeit a very weak one. Consequently the borate anions, as the conjugate bases of weak acids, are Brønsted bases.

The trigonal planar boron sites in species like boric acid illustrate the ability of trigonal planar boron to act as a Lewis acid, specifically by forming a Lewis base adduct.

\[\sf{B(OH)_3~~+~~OH^-~~\rightleftharpoons~~B(OH)_4^-} \nonumber \]

Notice that the three coordinate boron centers in Scheme \(\sf{\PageIndex{II}}\) are not drawn as possessing an octet, even though it would be possible to draw resonance forms which satisfy boron's octet by drawing resonance structures in which there are B=O bonds. This is because it is unclear that such resonance structures contribute significantly to the bonding in trigonal planar boron centers. To see why, it is helpful to consider the boron trihalides.

b. The boron trihalides. Structurally, boron trihalides like BCl₃ possess a trigonal planar structure like that in the BO₃ units of boric acid and metal borates. What is notable about the structure is that the B-X bonds are shorter than those in analogous tetrahedral structures, as illustrated in Scheme \(\sf{\PageIndex{III}}\).

Scheme \(\sf{\PageIndex{III}}\). Increase in the B-Cl bond length as the boron center goes from trigonal pyramidal to tetrahedral coordination on formation of an adduct with ammonia. Reproduced from a drawing by Ben Mills based on reference 6.

Figure \(\sf{\PageIndex{2C}}\).

Figure \(\sf{\PageIndex{2}}\). (A) Resonance explanation for short B-F bonds involving contributions from structures in which the partial charges run counter to the expected B\(\sf{^{\delta +}}\)-X\(\sf{^{\delta -}}\) polarity based on electronegativity and calculations, the latter of which support (B) an ionic explanation in terms of a need to reduce the strength of the greater number of repulsions (represented by magenta arrows) between F atoms in tetrahedral geometries compared to trigonal planar ones. (C) The shorter B-X bonds in trigonal planar vs. tetrahedral geometry may also be explained as following from the ligand close packing model. All distances are taken from reference 7.

c. Borazine and the polymorphs of boron nitride. One class of compounds in which multiple bonds involving boron are believed to play some role are borazine and the hexagonal polymorph of boron nitride, BN.

Borazine, B_3,N₃H₆, is isoelectronic and isostructural to benzene, C₆H₆, and has very similar properties. Both have a planar hexagonal molecular structure, are nonpolar, and exist as relatively low boiling liquids at room temperature. The existence of delocalized \(\pi\) bonding in the borazine ring may be inferred in that the B-N bond distances of 1.44 Å in borazine is less than the ~1.6 Å B-N distances typical for B-N single bonds in tetrahedral systems⁸ and close to the 1.42 Å C-C bond distance in benzene. While in benzene the electron delocalization is spread evenly around the carbon ring system, in borazine the greater electronegativity of N ensures that the electron density above and below the borazine is more heavily concentrated near the more electronegative N atoms. This is represented by the resonance and orbital descriptions of \(\pi\) bonding depicted in Scheme \(\sf{\PageIndex{IV}}\). As a result of the greater electron density on N and the resulting electron deficiency on B, the B and N atoms of borazine retain considerable Lewis acid and base character, respectively.

Scheme \(\sf{\PageIndex{IV}}\). (A) Resonance representation of the bonding in borazine and (B) representation of the lowest energy \(\pi\) bonding MO in borazine showing the greater electron density on the more electronegative N atoms.

Figure \(\sf{\PageIndex{3A}}\). The B and N in each layer are trigonal planar and arranged in planar layers of interlocking hexagonal rings. As with borazine, the \(\pi\) system is delocalized, although again the electron density is somewhat localized on the N atoms and unlike graphite, hexagonal BN is not a good conductor. In keeping with the polarity of the B-N bonds and the respective electron densities on B and N, the layers are organized so that the B atoms in one layer are arranged directly above and below the N atoms in the next and vice versa, although the intersheet spacing of 3.3 Å suggests that there is no interlayer bonding. Further, as with graphite the ability of the sheets to move about relatively freely makes hexagonal BN a good lubricant.

Although the layered hexagonal structure of boron nitride is the most stable, BN also forms two polymorphs in which the B and N atoms are tetrahedrally coordinated; these are analogous to the cubic diamond and Lonsdaleite forms of carbon and are given in Figures \(\sf{\PageIndex{3B}}\) and \(\sf{\PageIndex{3C}}\). They may be thought of as consisting of layers of linked interlocking B₃N₃ chairs. The two forms differ in terms of whether the layers are arranged so as to be linked in the form of chair or boat conformation B₃N₃ rings.

Figure \(\sf{\PageIndex{3}}\). (A) Layered structure of BN along with two resonance structures illustrating the bonding in a boron nitride layer, (B) cubic BN and a schematic depiction emphasizing the linking of layers of chair conformation B₃N₃ rings by chair conformation B₃N₃ rings and (C) Wurtzite-like hexagonal BN and a schematic depiction emphasizing the linking of layers by boat conformation B₃N₃ rings. The arrangement of B and N atoms in cubic and Wurtzitle-like hexagonal BN are analogous to those of C in cubic diamond and Lonsdaleite, respectively. The ball and stick images of layered, cubic, and Wurtzite-like hexagonal structures of BN are by Benjah-bmm27 - Own work, Public Domain, https://commons.wikimedia.org/w/inde...?curid=2129062, commons.wikimedia.org/w/inde...?curid=2129084, and commons.wikimedia.org/w/inde...?curid=2129086.

2. Boron can achieve stable bonding configurations by sharing electrons via bridge bonds. These are common in boron hydrides. For instance, another way the B of BH₃ can achieve an octet is to form two-center three-electron bridge bonds, as in diborane.

\[ \nonumber \]

Valence bond and molecular orbital descriptions of the "two-electron three-center" bridge bonds in these molecules have already been given in 8.2.1. Hydrogen's Chemical Properties.

Those who wish to review the MO description of bonding are invited to consider the derivation of the MOs of diborane given in Example \(\PageIndex{1}\).

Exercise \(\PageIndex{1}\). The molecular orbitals of Diborane

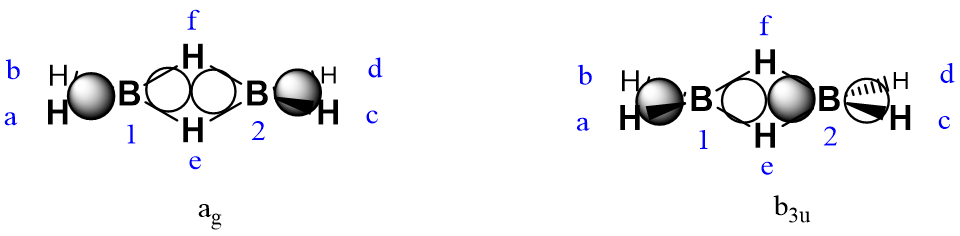
Use the projection operator method to derive MOs for diborane. The structure of diborane is shown below, along with the atom labels and coordinate system which will be used in the answer key.

Answer

Diborane has D_2h symmetry, under which the following sets of orbitals will transform into one another and should be considered as a set:

H_1s,a, H_1s,b, H_1s,c, H_1s,d

H_1s,f, H_1s,e

B_1s,1, B_1s,2

B_px,1, B_px,2

B_py,1, B_py,2

B_pz,1, B_pz,2

Using the projection operator method with the following generator orbitals gives:

Generator orbital	E	C2 (z)	C2 (y)	C2 (x)	i	σ (xy)	σ (xz)	σ (yz)
Set 1: H1s,a	H1s,a	H1s,c	H1s,d	H1s,b	H1s,d	H1s,b	H1s,a	H1s,c
Set 2: H1s,e	H1s,e	H1s,f	H1s,e	H1s,f	H1s,f	H1s,e	H1s,f	H1s,e
Set 3: B1s,1	B1s,1	B1s,2	B1s,2	B1s,1	B1s,2	B1s,1	B1s,1	B1s,2
Set 4: Bpx,1	Bpx,1	-Bpx,2	-Bpx,2	Bpx,1	-Bpx,2	Bpx,1	Bpx,1	-Bpx,2
Set 5: Bpy,1	Bpy,1	-Bpy,2	Bpy,2	-Bpy,1	-Bpy,2	Bpy,1	-Bpy,1	Bpy,2
Set 6: Bpz,1	Bpz,1	Bpz,2	-Bpz,2	-Bpz,1	-Bpz,2	-Bpz,1	Bpz,1	Bpz,2

The use of these projections to generate orbitals is straightforward but a lot of work.

For Set 1, the terminal H 1s orbitals, taking the 1s orbital on H_a as the generator orbital:

Ψ(A_g)? = [1(H_1sa) + (1)( H_1sc) + (1)(H_1sd) + (1)( H_1sb) + (1)( H_1sd) + (1)( H_1sb) +(1)(H_1sa) + (1)( H_1sc)]

Ψ (A_g) = 2H_1sa + 2H_1sb + 2H_1sc + 2H_1sd         So, there will be one a_g orbital (w/ all 1s in phase)

Ψ(B_1g)? = [1(H_1sa) + (1)( H_1sc) + (-1)(H_1sd) + (-1)( H_1sb) + (1)( H_1sd) + (1)( H_1sb) +(-1)(H_1sa) + (-1)( H_1sc)]

Ψ (B_1g) = 0H_1sa + 0H_1sb + 0H_1sc + 0H_1sd         No b_1g orbital

Ψ(B_2g)? = [1(H_1sa) + (-1)( H_1sc) + (1)(H_1sd) + (-1)( H_1sb) + (1)( H_1sd) + (-1)( H_1sb) +(1)(H_1sa) + (-1)( H_1sc)]

Ψ (B_2g) = 2H_1sa - 2H_1sb - 2H_1sc + 2H_1sd         So, there will be one b_2g orbital

Ψ(B_3g)? = [1(H_1sa) + (-1)( H_1sc) + (-1)(H_1sd) + (1)( H_1sb) + (1)( H_1sd) + (-1)( H_1sb) +(-1)(H_1sa) + (-1)( H_1sc)]

Ψ (B_3g) = = 0H_1sa + 0H_1sb + 0H_1sc + 0H_1sd         No b_3g orbital

Ψ(A_u)? = [1(H_1sa) + (1)( H_1sc) + (1)(H_1sd) + (1)( H_1sb) + (-1)( H_1sd) + (-1)( H_1sb) +(-1)(H_1sa) + (-1)( H_1sc)]

Ψ (A_u) = 0H_1sa + 0H_1sb + 0H_1sc + 0H_1sd         So, there will be no a_u orbital

Ψ(B_1u)? = [1(H_1sa) + (1)( H_1sc) + (-1)(H_1sd) + (-1)( H_1sb) + (-1)( H_1sd) + (-1)( H_1sb) +(1)(H_1sa) + (1)( H_1sc)]

Ψ (B_1u) = 2H_1sa - 2H_1sb + 2H_1sc - 2H_1sd         So, there will be one b_1u orbital

Ψ(B_2u)? = [1(H_1sa) + (-1)( H_1sc) + (1)(H_1sd) + (-1)( H_1sb) + (-1)( H_1sd) + (1)( H_1sb) +(-1)(H_1sa) + (1)( H_1sc)]

Ψ (B_2u) = 0H_1sa + 0H_1sb + 0H_1sc + 0H_1sd         So, there will be no b_2u orbital

Ψ(B_3u)? = [1(H_1sa) + (-1)( H_1sc) + (-1)(H_1sd) + (1)( H_1sb) + (-1)( H_1sd) + (1)( H_1sb) +(1)(H_1sa) + (-1)( H_1sc)]

Ψ (B_3u) = 2H_1sa + 2H_1sb - 2H_1sc - 2H_1sd         So, there will be one b_3u orbital

So the first set gives the following terminal hydrogen 1s group orbitals:

For Set 2, The H 1s orbitals on the bridging H, taking the 1s orbital on H_e as the generator orbital.

Ψ(A_g)? = [1(H_1se) + (1)( H_1sf) + (1)(H_1se) + (1)( H_1sf) + (1)( H_1sf) + (1)( H_1se) +(1)(H_1sf) + (1)( H_1se)]

Ψ (A_g) = 4H_1se + 4H_1sf         So, there will be one a_g orbital (w/ both 1s in phase)

Ψ(B_1g)? = [1(H_1se) + (1)( H_1sf) + (-1)(H_1se) + (-1)( H_1sf) + (1)( H_1sf) + (1)( H_1se) +(-1)(H_1sf) + (-1)( H_1se)

Ψ (B_1g) = 0H_1se + 0H_1sf         No b_1g orbital

Ψ(B_2g)? = [1(H_1se) + (-1)( H_1sf) + (1)(H_1se) + (-1)( H_1sf) + (1)( H_1sf) + (-1)( H_1se) +(1)(H_1sf) + (-1)( H_1se)]

Ψ (B_2g) = 0H_1se + 0H_1sf         So, there will be no b_2g orbital

Ψ(B_2g)? = [1(H_1se) + (-1)( H_1sf) + (-1)(H_1se) + (1)( H_1sf) + (1)( H_1sf) + (-1)( H_1se) +(-1)(H_1sf) + (1)( H_1se)]

Ψ (B_3g) = = 0H_1sa + 0H_1sb + 0H_1sc + 0H_1sd         No b_3g orbital

Ψ(A_u)? = [1(H_1se) + (1)( H_1sf) + (1)(H_1se) + (1)( H_1sf) + (-1)( H_1sf) + (-1)( H_1se) +(-1)(H_1sf) + (-1)( H_1se)]

Ψ (A_u) = 0H_1se + 0H_1sf         So, there will be no a_u orbital

Ψ(B_1u)? = [1(H_1se) + (1)( H_1sf) + (-1)(H_1se) + (-1)( H_1sf) + (-1)( H_1sf) + (-1)( H_1se) +(1)(H_1sf) + (1)( H_1se)]

Ψ (B_1u) = 0H_1se + 0H_1sf         So, there will be no b_1u orbital

Ψ(B_2u)? = [1(H_1se) + (-1)( H_1sf) + (1)(H_1se) + (-1)( H_1sf) + (-1)( H_1sf) + (1)( H_1se) +(-1)(H_1sf) + (1)( H_1se)]

Ψ (B_2u) = 4H_1se - 4H_1sf         So, there will be a b_2u orbital

Ψ(B_3u)? = [1(H_1se) + (-1)( H_1sf) + (-1)(H_1se) + (1)( H_1sf) + (-1)( H_1sf) + (1)( H_1se) +(1)(H_1sf) + (-1)( H_1se)]

Ψ (B_3u) = 0H_1se + 0H_1sf         So, there will be no b_3u orbital

So the second set gives the following bridging hydrogen 1s group orbitals:

For Set 3, The B 2s orbitals, taking the 2s orbital on B1 as the generator orbital:

Ψ(A_g)? = [1(B_s1) + (1)( B_s2) + (1)( B_s2) + (1)( B_s1) + (1)( B_s2) + (1)( B_s1) +(1)( B_s1) + (1)( B_s2)]

Ψ (A_g) = 4B_s,1 + 4B_s,2         So, there will be one a_g orbital (w/ the B 1s in phase)

Ψ(B_1g)? = [1(B_s1) + (1)( B_s2) + (-1)( B_s2) + (-1)( B_s1) + (1)( B_s2) + (1)( B_s1) +(-1)( B_s1) + (-1)( B_s2)]

Ψ (B_1g) = 0B_s,1 + 0B_s,2         No b_1g orbital

Ψ(B_2g)? = [1(B_s1) + (-1)( B_s2) + (1)( B_s2) + (-1)( B_s1) + (1)( B_s2) + (-1)( B_s1) +(1)( B_s1) + (-1)( B_s2)]

Ψ (B_2g) = 0B_s,1 + 0B_s,2         No b_2g orbital

Ψ(B_2g)? = [1(B_s1) + (-1)( B_s2) + (-1)( B_s2) + (1)( B_s1) + (1)( B_s2) + (-1)( B_s1) +(-1)( B_s1) + (1)( B_s2)]

Ψ (B_3g) = 0B_s,1 + 0B_s,2         No b_3g orbital

Ψ(A_u)? = [1(B_s1) + (1)( B_s2) + (1)( B_s2) + (1)( B_s1) + (-1)( B_s2) + (-1)( B_s1) +(-1)( B_s1) + (-1)( B_s2)]

Ψ (A_u) = 0B_s,1 + 0B_s,2         So, there will be no a_u orbital

Ψ(B_1u)? = [1(B_s1) + (1)( B_s2) + (-1)( B_s2) + (-1)( B_s1) + (-1)( B_s2) + (-1)( B_s1) +(1)( B_s1) + (1)( B_s2)]

Ψ (B_1u) = 0B_s1 + 0B_s2         So, there will be no b_1u orbital

Ψ(B_2u)? = [1(B_s1) + (-1)( B_s2) + (1)( B_s2) + (-1)( B_s1) + (-1)( B_s2) + (1)( B_s1) +(-1)( B_s1) + (1)( B_s2)]

Ψ (B_2u) = 0B_s1 + 0B_s2         So, there will be no b_2u orbital

Ψ(B_3u)? = [1(B_s1) + (-1)( B_s2) + (-1)( B_s2) + (1)( B_s1) + (-1)( B_s2) + (1)( B_s1) +(1)( B_s1) + (-1)( B_s2)]

Ψ (B_3u) = 4B_s1 - 4B_s2         So, there will be one b_3u orbital

The boron 2s group orbitals are:

For Set 4: The B 2p_x orbitals, using the 2p_x orbital on B1 as the generator orbital:

Ψ(A_g)? = [1(B_px,1) + (1)(-B_px2) + (1)(-B_px2) + (1)( B_px1) + (1)( -B_px2) + (1)(B_px1) +(1)(B_px1) + (1)(-B_px2)]

Ψ (A_g) = 4B_1px1 - 4B_2px1         So, there will be one a_g orbital (w/ the B 2p_x antiphase)

Ψ(B_1g)? = [1(B_px,1) + (1)(-B_px2) + (-1)(-B_px2) + (-1)( B_px1) + (1)( -B_px2) + (1)(B_px1) +(-1)(B_px1) + (-1)(-B_px2)]

Ψ (B_1g) = 0B_1px1 + 0B_1px2         No b_1g orbital

Ψ(B_2g)? = [1(B_px,1) + (-1)(-B_px2) + (1)(-B_px2) + (-1)( B_px1) + (1)( -B_px2) + (-1)(B_px1) +(1)(B_px1) + (-1)(-B_px2)]

Ψ (B_2g) = 0B_1px1 + 0B_1px2         No b_2g orbital

Ψ(B_3g)? = [1(B_px,1) + (-1)(-B_px2) + (-1)(-B_px2) + (1)( B_px1) + (1)( -B_px2) + (-1)(B_px1) +(-1)(B_px1) + (1)(-B_px2)]

Ψ (B_3g) = 0B_1px1 + 0B_1px2         No b_3g orbital

Ψ(A_u)? = [1(B_px,1) + (1)(-B_px2) + (1)(-B_px2) + (1)( B_px1) + (-1)( -B_px2) + (-1)(B_px1) +(-1)(B_px1) + (-1)(-B_px2)]

Ψ (A_u) = 0B_1px1 + 0B_1px2         So, there will be no a_u orbital

Ψ(B_1u)? = [1(B_1s1) + (1)( B_1s2) + (-1)( B_1s2) + (-1)( B_1s1) + (-1)( B_1s2) + (-1)( B_1s1) +(1)( B_1s1) + (1)( B_1s2)]

Ψ (B_1u) = 0B_1px1 + 0B_1px2         So, there will be no b_1u orbital

Ψ(B_2u)? = [1(B_px,1) + (-1)(-B_px2) + (1)(-B_px2) + (-1)( B_px1) + (-1)( -B_px2) + (1)(B_px1) +(-1)(B_px1) + (1)(-B_px2)]

Ψ (B_2u) = 0B_1px1 + 0B_1px2         So, there will be no b_2u orbital

Ψ(B_3u)? = [1(B_px,1) + (-1)(-B_px2) + (-1)(-B_px2) + (1)( B_px1) + (-1)( -B_px2) + (1)(B_px1) +(1)(B_px1) + (-1)(-B_px2)]

Ψ (B_3u) = 4B_px1 + 4B_px2         So, there will be one b_3u orbital

So the B 2p_x group orbitals are

For Set 5: The B 2p_y orbitals, taking 2p_y on B1 as the generator orbital:

Ψ(A_g)? = [1(B_py1) + (1)(-B_py2) + (1)(B_py2) + (1)(-B_py1) + (1)(-B_py2) + (1)(B_py1) +(1)(-B_py1) + (1)(B_py2)]

Ψ (A_g) = 0 B_py1 + 0B_py2         So, there will be no a_g orbital

Ψ(B_1g)? = [1(B_py1) + (1)(-B_py2) + (-1)(B_py2) + (-1)(-B_py1) + (1)(-B_py2) + (1)(B_py1) +(-1)(-B_py1) + (-1)(B_py2)]

Ψ (B_1g) = 2B_py1 - 2B_py2         One b_1g orbital

Ψ(B_2g)? = [1(B_py1) + (-1)(-B_py2) + (1)(B_py2) + (-1)(-B_py1) + (1)(-B_py2) + (-1)(B_py1) +(1)(-B_py1) + (-1)(B_py2)]

Ψ (B_2g) = 0B_py1 + 0B_py2 ;         No b_2g orbital

Ψ(B_2g)? = [1(B_py1) + (-1)(-B_py2) + (-1)(B_py2) + (1)(-B_py1) + (1)(-B_py2) + (-1)(B_py1) +(-1)(-B_py1) + (1)(B_py2)]

Ψ (B_2g) = 0B_py1 + 0B_py2 ;         No b_3g orbital

Ψ(A_u)? = [1(B_py1) + (1)(-B_py2) + (1)(B_py2) + (1)(-B_py1) + (-1)(-B_py2) + (-1)(B_py1) +(-1)(-B_py1) + (-1)(B_py2)]

Ψ (A_u) = 0B_py1 + 0B_py2 ;         So, there will be no a_u orbital

Ψ(B_1u)? = [1(B_py1) + (1)(-B_py2) + (-1)(B_py2) + (-1)(-B_py1) + (-1)(-B_py2) + (-1)(B_py1) +(1)(-B_py1) + (1)(B_py2)]

Ψ (B_1u) = 0B_py1 + 0B_py2 ;         So, there will be no b_1u orbital

Ψ(B_2u)? =[1(B_py1) + (-1)(-B_py2) + (1)(B_py2) + (-1)(-B_py1) + (-1)(-B_py2) + (1)(B_py1) +(-1)(-B_py1) + (1)(B_py2)]

Ψ (B_2u) = 4B_py1 + 4B_py2;         So, there will be a b_2u orbital

Ψ(B_3u)? = [1(B_py1) + (-1)(-B_py2) + (-1)(B_py2) + (1)(-B_py1) + (-1)(-B_py2) + (1)(B_py1) +(1)(-B_py1) + (-1)(B_py2)]

Ψ (B_3u) = 0B_py1 + 0B_py2 ;         So, there will be no b_3u orbital

So the B 2p_y group orbitals are

For Set 6: The B 2p_z orbitals, taking the 2p_z orbital of B1 as the generator orbital.

Ψ(A_g)? = [1(B_pz1) + (1)(B_pz2) + (1)(-B_pz2) + (1)(-B_pz1) + (1)(-B_pz2) + (1)(-B_pz1) +(1)(B_pz1) + (1)(B_pz2)]

Ψ (A_g) = 0 B_pz1 + 0 B_pz2         So, there will be an a_g orbital

Ψ(B_1g)? = [1(B_pz1) + (1)(B_pz2) + (-1)(-B_pz2) + (-1)(-B_pz1) + (1)(-B_pz2) + (1)(-B_pz1) +(-1)(B_pz1) + (-1)(B_pz2)]

Ψ (B_1g) = 0 B_pz1 + 0B_pz2         So, there will be no b_1g orbital

Ψ(B_2g)? = [1(B_pz1) + (-1)(B_pz2) + (1)(-B_pz2) + (-1)(-B_pz1) + (1)(-B_pz2) + (-1)(-B_pz1) +(1)(B_pz1) + (-1)(B_pz2)]

Ψ (B_2g) = 4 B_pz1 - 4B_pz2         So, there will be a b_2g orbital

Ψ(B_3g)? = [1(B_pz1) + (-1)(B_pz2) + (-1)(-B_pz2) + (1)(-B_pz1) + (1)(-B_pz2) + (-1)(-B_pz1) +(-1)(B_pz1) + (1)(B_pz2)]

Ψ (B_3g) = 0 B_pz1 + 0B_pz2         So, there will be no b_3g orbital

Ψ(A_u)? = [1(B_pz1) + (1)(B_pz2) + (1)(-B_pz2) + (1)(-B_pz1) + (-1)(-B_pz2) + (-1)(-B_pz1) +(-1)(B_pz1) + (-1)(B_pz2)]

Ψ (A_u) = 0 B_pz1 + 0B_pz2         So, there will be no a_u orbital

Ψ(B_1u)? = [1(B_pz1) + (1)(B_pz2) + (-1)(-B_pz2) + (-1)(-B_pz1) + (-1)(-B_pz2) + (-1)(-B_pz1) +(1)(B_pz1) + (1)(B_pz2)]

Ψ (B_1u) = 4 B_pz1 + 4B_pz2         So, there will be a b_1u orbital

Ψ(B_2u)? = [1(B_pz1) + (-1)(B_pz2) + (1)(-B_pz2) + (-1)(-B_pz1) + (-1)(-B_pz2) + (1)(-B_pz1) +(-1)(B_pz1) + (1)(B_pz2)]Ψ (B_2u) = 0 B_pz1 + 0B_pz2         So, there will be no b_2u orbital

Ψ(B_3u)? = [1(B_pz1) + (-1)(B_pz2) + (-1)(-B_pz2) + (1)(-B_pz1) + (-1)(-B_pz2) + (1)(-B_pz1) +(1)(B_pz1) + (-1)(B_pz2)]

Ψ (B_3u) = 0 B_pz1 + 0B_pz2         So, there will be no b_3u orbital

So the B 2p_z group orbitals are

Finally, to construct MOs for the diborane group, orbitals of the same symmetry should be allowed to mix. The exact way in which this will occur should ideally be predicted by performing a quantum chemical calculation. However, an approximate diagram can be qualitatively estimated from the atomic orbital energies (which are conveniently available in a periodic table of atomic orbital energies). The result is given in 8.2.1. Hydrogen's Chemical Properties, reproduced for convenience below.

3. Share electrons among multiple boron and other atoms in a cluster. Such clusters are very common among the boron hydrides (boranes) and their derivatives. Examples are given in Scheme \(\sf{\PageIndex{V}}\).

Scheme \(\sf{\PageIndex{V}}\). Examples of electron-deficient boron clusters. These consist of a cluster H-B: groups in which the B atoms are held together by sharing electrons among the atoms of the cluster. The B-B and B---B bonds in these images are drawn to help define the cluster shape, in which the B atoms tend to occupy the vertices of a deltahedron.

Many aspects of the bonding in clusters will be explained in more detail in Section 15.4 Cluster Compounds, which also presents Wade's rules and the Polyhedral Skeletal Electron Pair Theory which may be used to rationalize their stability. This section instead focuses on outlining some features of borane chemistry.

Electron-deficient clusters are distinguished based on whether their shape is that of a complete deltahedron - a type of polyhedron in which all the faces are equilateral triangles. In closo-clusters the vertex atoms comprise a complete deltahedron while in nido-, arachno-, and hypho- clusters, one, two, and three of the deltahedron's vertices are unoccupied, respectively. For instance, in Scheme \(\sf{\PageIndex{V}}\), tetrahedral B₄H₄^2- and octahedral B₆H₆^2- are closo- while square pyramidal B₅H₉ is nido- since it corresponds to an octahedron with a missing vertex.

Notice also that there are bridging B-H-B bonds along the edges of the open vertices in the nido- and arachno- clusters of Scheme \(\sf{\PageIndex{IV}}\). This is a common feature of such clusters, although these bonds are not always present since the hydrogens can often be deprotonated to give stable anionic clusters.

\[\sf{B_5H_9~~+~~MH~~\overset{low~T}{\longrightarrow}~~M^+~~+~~B_5H_8^-~~H_2~~~~~~~~~~(M~=~alkali~metal)} \nonumber \]

Many boranes are anionic. The most iconic is icosahedral closo-dodecaborate, B₁₂H₁₂^2-, the structure of which is shown in Figure \(\sf{\PageIndex{3}}\).

Figure \(\sf{\PageIndex{3}}\). Structure of closo-dodecaborate, B₁₂H₁₂^2-, showing boron atoms in cream and H atoms in white. The diameter of the cluster is similar to that of a benzene ring. Rendered from COD ID: 4306523 as reported in reference 9.

Typically, the higher boranes are formed by pyrolytic removal of H₂, H^- , and/or H⁺ units from BH₃ or BH₄^-. This process may be conceptualized as giving rise to BH, BH^-, and BH₂ units which coalesce into clusters. The process may be seen in part by considering the preparation of closo-dodecaborate. First the diborane is pyrolyzed to give arachno-decaborane(14), B₁₀H₁₄, in a process in which the loss of B-H and B-H-B bonds is compensated for by the formation of cluster bonds in which multiple B atoms share the bonding electrons. The resulting arachno-decaborane(14), B₁₀H₁₄, has the icosahedral shape of dodecaborate.

\[ \nonumber \]

The two missing vertices in the arachno- cluster are added by allowing the cluster to react with additional BH₃ units, this time in the form of the Et₃N-BH₃ adduct.

\[ \nonumber \]

Finally, it should be noted that elements other than boron can form clusters and that, consequently, boron forms mixed clusters with a variety of elements. Some examples include the carboranes depicted in Scheme \(\sf{\PageIndex{VI}}\).

Scheme \(\sf{\PageIndex{VI}}\). Some carborane clusters, including the ortho-, meta-, and para-"carborane", C₂B₁₀H_12^.

Note \(\sf\PageIndex{1}\): Conjuncto Boranes

In addition to ordinary closo-, nido-, arachno-, and hypho- boranes, there are conjuncto-boranes, which consist of two clusters joined via a B-B linkage, at a vertex, edge, or face. An example of such a cluster is shown in Scheme \(\sf{\PageIndex{VI}}\).

Scheme \(\sf{\PageIndex{VII}}\). A face-sharing conjuncto- borane.

Note \(\sf\PageIndex{2}\): Boron in Medicinal Chemistry: Neutron Capture Therapy

Boranes and their derivatives are the subject of ongoing research into effective neutron capture therapies for the treatment of tumors of the head and neck. The basis of these therapies is the nuclear chemistry of its ¹⁰B isotope, which comprises 19.6% of naturally occurring boron (the remaining 80.4% is ¹¹B). Boron-10 can absorb neutrons to give excited ¹¹B, which undergoes radiative decay via alpha emission:

\[\sf{Neutron ~capture:~~~~\ce{^{10}_5B~~+~~^1_1n~~\longrightarrow~~[^{11}_5B]$^{*}$}} \nonumber \]

\[\sf{alpha~emission:~~~~\ce{[^{11}_5B]$^{*}$~~\longrightarrow~~^7_3Li~~+~~^4_2He}} \nonumber \]

This sequence of reactions in medical applications to treat tumors is called neutron capture therapy. The basic principles of neutron capture therapy are summarized in Figure \(\sf{\PageIndex{4}}\).

Figure \(\sf{\PageIndex{4}}\). Use of neutron capture therapy to destroy cancerous cells. The image is by Pat Kenny (Illustrator) / Public domain.

As can be seen from Figure \(\sf{\PageIndex{4}}\), the process involves irradiating the boron-infused tumor with neutrons. These neutrons are poorly absorbed by tissues comprised primarily of the big six elements of biochemistry (C, H, N, O, S, and P) and so minimally damage normal tissue. However, in boron-infused tumor cells, \(\ce{$[$^{11}_5B$]$^{$*$}}\) produced by neutron capture degrades to give high energy \(\sf{^7_3Li}\) and \(\sf{^4_2He}\) that damage the surrounding cancerous tissue.

Effective neutron capture therapy requires the use of a boron reagent that is otherwise nontoxic and can be selectively delivered to cancer cells in therapeutically-effective concentrations. This has been a considerable research challenge and the development of such reagents is a subject of ongoing research. Many of the compounds tried so far are borane or carborane amino or nucleic acid analogues or conjugates of borane and various biomolecules, although borane-infused liposomes (liposomes are preferentially taken up and retained by tumors) have recently been shown effective against certain types of tumors in mice.

Notes and References

1. Nihonium is not included, since as a metastable synthetic element with isotope-dependent half-lives of less than 10 seconds it is poorly characterized. The boron structure is taken from https://chem.libretexts.org/Bookshelves/Inorganic_Chemistry/Book%3A_Inorganic_Chemistry_(Saito)/04%3A_Chemistry_of_Nonmetallic_Elements/4.02%3A_Main_group_elements_of_2nd_and_3rd_periods_and_their_compounds. The FCC and HCP structures are original PNGs by Daniel Mayer and DrBob, traced in Inkscape by User:Stannered - Cubic, face-centered.png: Lattice face centered cubic.svg:, CC BY-SA 3.0, commons.wikimedia.org/w/inde...?curid=1735631; By !Original: DornelfVector: DePiep - Own work based on: Hexagonal close packed.png, CC BY-SA 3.0, commons.wikimedia.org/w/inde...curid=20183889; those of Ga and In are rendered using Mercury from data deposited in the Materials Project Database as reported in references 4 and 5, respectively. Electronegativity, ionization energy, electron affinity, and radii values are taken from the Royal Society of Chemistry periodic table database at https://www.rsc.org/periodic-table/.

2. Called Aluminium in the UK and other countries where British English is used.

3. A. Jain*, S.P. Ong*, G. Hautier, W. Chen, W.D. Richards, S. Dacek, S. Cholia, D. Gunter, D. Skinner, G. Ceder, K.A. Persson (*=equal contributions)
The Materials Project: A materials genome approach to accelerating materials innovation APL Materials, 2013, 1(1), 011002.

4. Sharma, B.D. and Donohue, J. Zeitschrift fuer Kristallographie, Kristallgeometrie, Kristallphysik, Kristallchemie 1962, 117, 293.

5. Hull, A.W.; Davey, W.P.", Physical Review 1921, 17, 266-267.

6. Robinson, E. A.; Johnson, S. A.; Tang, T.-H.; Gillespie, R. J. Inorganic Chemistry 1997, 36 (14), 3022-3030.

7. Robinson, E.A.; Heard, G.L.; Gillespie, R.J. Journal of Molecular Structure 485–486 (1999) 305–319.

8. Orpen, A. G.; Brammer, L.; Allen, F. H.; Kennard, O.; Watson, D. G.; Taylor, R., Appendix A: Typical Interatomic Distances in Organic Compounds and Organometallic Compounds and Coordination Complexes of the d- and f-block metals. In Structure Correlation, Wiley-VCH Verlag GmbH: 2008; pp 752-858.

9. Jae-Hyuk Her; Muhammed Yousufuddin; Wei Zhou; Satish S. Jalisatgi; James G. Kulleck; Jason A. Zan; Son-Jong Hwang; Robert C. Bowman; Terrence J. Udovic Inorganic Chemistry 2008, 47, 9757-9759.

10. Kueffer, P. J.; Maitz, C. A.; Khan, A. A.; Schuster, S. A.; Shlyakhtina, N. I.; Jalisatgi, S. S.; Brockman, J. D.; Nigg, D. W.; Hawthorne, M. F., Boron neutron capture therapy demonstrated in mice bearing EMT6 tumors following selective delivery of boron by rationally designed liposomes. Proceedings of the National Academy of Sciences 2013, 110 (16), 6512-6517.

Contributors and Attributions

Stephen Contakes, Westmont College