6.6.2: Hard-Hard and Soft-Soft preferences may be explained and quantified in terms of electrostatic and covalent and electronic stabilization on the stability of Lewis acid-base adducts
- Page ID
- 162917
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)The Hard Soft Acid Base Principle is a Conceptual Tool for Thinking About Patterns of Lewis Acid-Base Reactivity
The explanation of the trends in metal distribution, halide salt solubility, and preferred metal coordination patterns is rooted in Arland, Chatt, and Davies' observation that Lewis acids and bases could be classified into two groups based on their propensity to form stable compounds with one another (e.g. acids in a class tend to form more stable adducts with bases in the same class than they did with bases in the other).1 Arland, Chatt, and Davies somewhat boringly termed these groups class a and class b but today they are known by Ralph Pearson's name for them. Pearson called the class a acids and bases hard and class b acids and bases soft. These terms reflect how "soft" these substance's electron clouds are towards distortion or, in other words, their polarizability (Figure \(\PageIndex{1}\)). Pearson terms acids and bases which are relatively polarizable soft and those which are difficult to polarize hard.
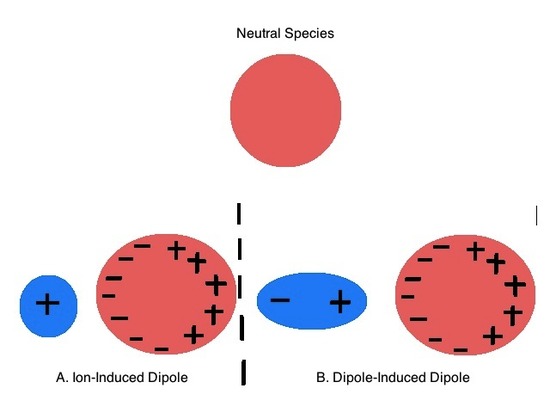
Recognizing Hard and Soft Acids and Bases
Hard acids and bases come in two varieties:
- hard acid and base sites that possess few valence electrons and for which polarization therefore involves distorting core electrons, which are difficult to distort because they are close to the nucleus and experience a high nuclear charge. The most common examples of such substances are Lewis acid hard acids towards the left of the periodic table.
- hard acid and base sites with a high charge density (highly charged relative to size) and/or which are electron deficient. In these cases polarization involves distorting electrons that already experience strong unshielded electrostatic interactions.
Soft acids and bases also come in two varieties
- soft acids and bases which posses many valence electrons and so are more readily polarized. In consequence, all other things being equal, soft acids and bases are more likely to be found towards the middle or right of the periodic table.
- soft acids and bases with little charge density and/or which are relatively electron rich.
Note that the hard-soft classification should not be thought of as if all hard acids and bases are equally hard and all soft acids and bases equally soft. There is a graduation in hardness and softness and a number of intermediate acids and bases which do not fit neatly in either category. With this caveat in mind, representative hard, soft, and borderline acids are given below. Notice how they illustrate the trends just outlined.
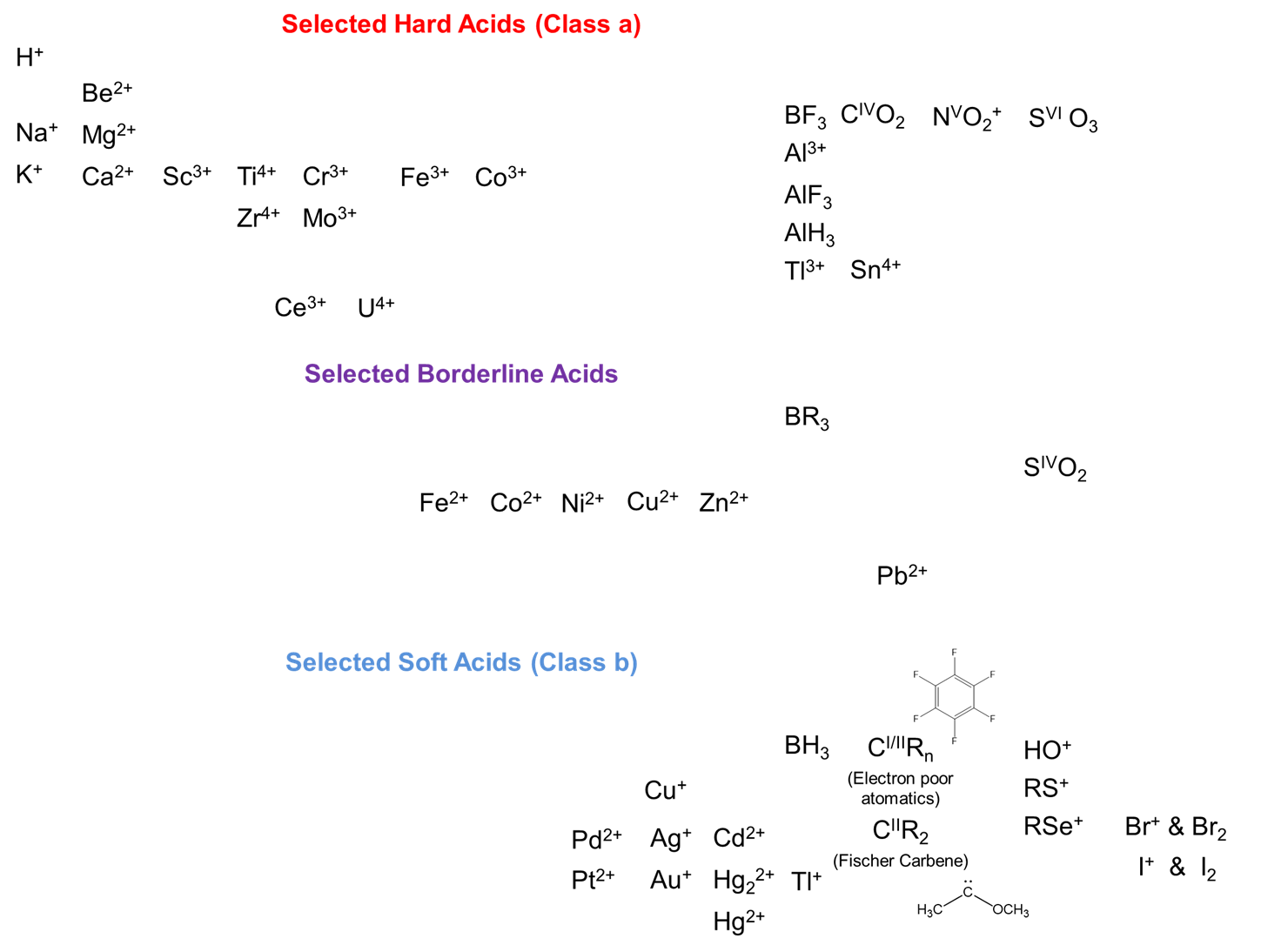
As expected, hard acids tend to be found towards the left side of the periodic table and involve higher oxidation states and/or electron donating substituents while soft acids are more common to the right of the periodic table and involve lower oxidation states and/or electron donating substituents.
Illustrative hard, soft, and borderline bases are given below. Again, notice how these substances illustrate the general trends.
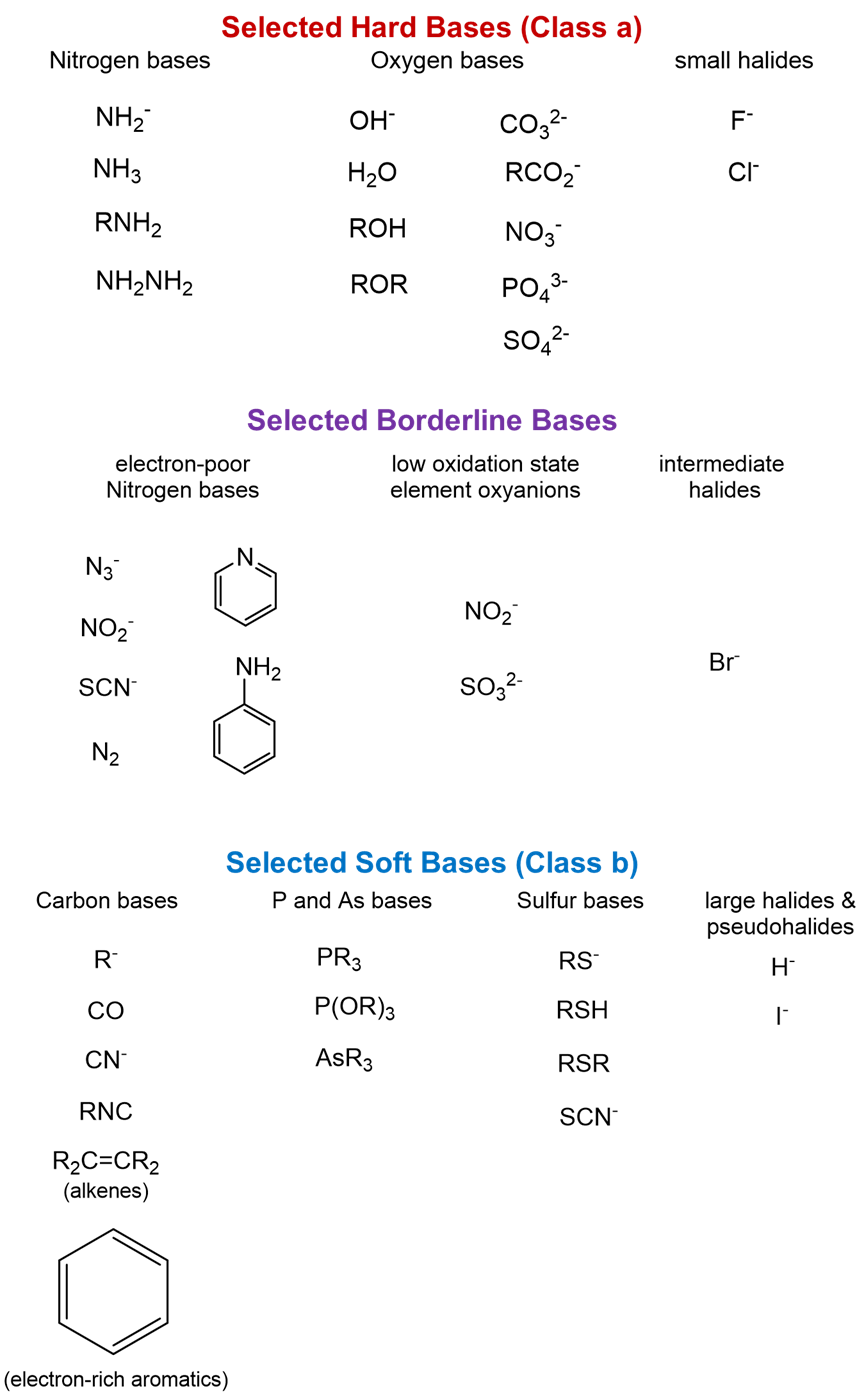
Qualitative Estimation of the Relative Hardness and Softness of Lewis Acids and Bases
As can be seen from the examples above, hard acids are relatively electron-poor and hard bases electron-rich since they have comparatively
- small frontier orbitals, reflective of their relatively small atom/ion/fragment sizes
- high (for acids) or low (for bases) oxidation states on the base atom, reflected in a large positive formal charge (for acids) or negative formal charge (for bases)
- low polarizability, due to loss or gain of substantial numbers of electrons, or the localization of
- positive charge on an electropositive element or an atom bearing electron-withdrawing substituents
- negative charge on an electronegative element or an atom bearing electron-donating substituents
In contrast to hard acids and bases, soft acids are relatively electron-rich and soft bases larger and more electron poor since they have comparatively
- large frontier orbitals, reflective of their relatively large atom/ion/fragment sizes
- low oxidation states, often resulting in small or nonexistent atomic charges
- high polarizability, as might be expected of species in which electron-electron repulsions are lower and electrons are spread over a large volume. Sometimes this is indicated by
- positive charge on an electronegative element or an atom bearing electron-donating substituents
- negative charge on an electropositive element or an atom bearing electron-withdrawing substituents
Rank the acids or bases in each set in increasing order of expected hardness.
- Cr2+ and Cr3+
- H+, Cs+, and Tl+
- SCN- (acting as a base at N) and SCN- (acting as a base at S)
- AlF3, AlH3, AlMe3
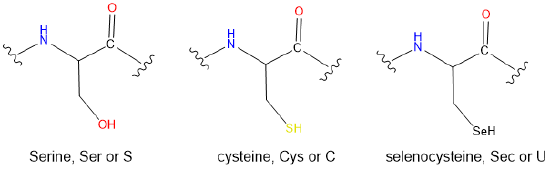
- The side chains of the following proteinogenic amino acids
- Answer
-
(a) Cr2+ < Cr3+ All other things being equal, hardness increases with oxidation state.
(b) Tl+ < Cs+ < H+ The order reflects Cs+ and Tl+'s larger size relative to H+ (which doesn't possess any electrons that can be polarized anyway) and that Tl+ still possesses two valence electrons while Cs+ possesses none.
(c) SCN- (acting as a base at S) < SCN- (acting as a base at N). The order reflects the greater electronegativity of N than S and N's possession of a more negative formal charge of -1.
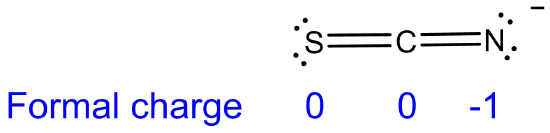
(d) AlH3 < AlMe3 < AlF3. The hardness increases as the substituents on the Lewis acid Al center become less electron donating and more electron withdrawing (and, incidentally, harder bases) as their electronegativity increases in the order H- < CH3- < F-. Note that the order of electron donating ability for H- and CH3- is the opposite observed for carbocations, for which hyperconjugation plays a larger role.
(e) Sec < Cys < Ser. The hardness increases as the electronegativity of the Lewis base chalcogen increases on going from a selenol to a thiol to an alcohol.
The Hard-Soft Acid-Base Principle (HSAB Principle)
The Hard-Soft acid-base principle (HSAB Principle) explains patterns in Lewis acid-base reactivity in terms of a like reacts with like preference. Both thermodynamically and kinetically, hard acids prefer hard bases and soft acids soft bases. Specifically,
- Thermodynamically, hard acids form stronger acid-base complexes with hard bases while soft acids form stronger complexes with soft bases.
- Kinetically, hard acids/electrophiles react more quickly with hard bases/nucleophiles while soft acids/electrophiles react more quickly with soft bases/neucleophiles.
Applications of the HSAB principle include
1. Predicting the equilibrium or speed of Lewis acid-base metathesis and displacement reactions. In a Lewis acid-base metathesis reaction the acids and bases swap partners \[\ce{A1:B1 + A2:B2 <=>[k_1, K_{eq}] A1:B2 + A2:B1} \nonumber \]
For example, the equilibrium position of the metathesis reaction between \(\ce{TlF}\) and \(\ce{K2S}\) favors the products:
\[\ce{2TlF + H2S <=>> Tl2S + 2KF} \nonumber \]
consistent with the HSAB's hard-hard and soft-soft preference.
 \[ \nonumber \]
\[ \nonumber \]
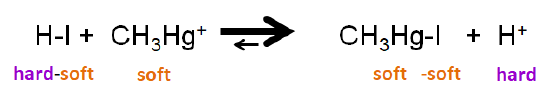
The HSAB principle also allows for prediction of the position of displacement reactions, in which a Lewis acid or base forms an adduct using a base or acid from an existing Lewis acid-base complex. In these reactions, the displacement of acid or base from the reactant complex may be thought of as a sort of metathesis reaction, one in which in the unbound acid or base switches places with one in the complex. For example, the reaction between \(\ce{HI}\) and methylmercury cation
\[\ce{HI + HgSCH3^{+} <=> CH3SHgI + H^{+}} \nonumber \]
involves displacement of an iodide from \(\ce{HI}\) to give \(\ce{CH3HgI}\). The position of the equilibrium favors \(\ce{CH3HgI}\) since both \(\ce{CH3Hg^{+}}\) and \(\ce{I^{-}}\) are soft, while \(\ce{H^{+}}\) is a hard acid.
 \[ \nonumber \]
\[ \nonumber \]
Predict the position of equilibrium for the following reaction.
\[\ce{Fe2O3 + 3Ag2S <=> Fe2S3 + 3Ag2O} \nonumber \]
- Answer
-
The equilibrium will favor the reactants (K<1) since the hard-hard and soft-soft interactions in the reactants are more stable than the hard-soft interactions in the products.

Predict whether \(K\) for the following equilibria will be <<1, ~1, or >>1.
- \(\ce{2HF + (CH_3Hg)_2S ⇌ 2CH_3HgF + H_2S}\)
- \(\ce{Ag(NH_3)_2^+ + 2PH_3 ⇌ Ag(PH_3)_2^+ + 2NH_3}\)
- \(\ce{Ag(PH_3)_2^+ + 2H_3B-SH_2 ⇌ 2H_3B-PH_3 + Ag(SH_2)_2^{+}}\)
- \(\ce{H_3B-NH_3 + F_3B-SH_2 ⇌ H_3B-SH_2 + F_3B-NH_3}\)
- Answer
-
a. K< < 1 since the reactant adducts are hard-hard and soft-soft while the products involve hard-soft interactions.
b. K>>1 since the reactant complex, diamine silver(I), is a complex of a hard base, NH3, with the soft acid, Ag+, while the product is a complex of the same soft acid with the soft base phosphine.
c. K~1 since all the adducts amongst the reactants and products involve soft acids and bases.
d. K>>1 since BH3 is a softer acid than BF3, so it will form a stronger complex with the softer base H2S while the harder BF3 forms a stronger complex with the harder base NH3.
2. Predicting the relative strengths of a given set of Lewis acids or bases towards a particular substrate. Consider, for example, the relative strengths of a BH3, BMe3, and BF3 towards group 15 hydrides like NH3, PH3, and AsH3. Of the boranes listed, the hardest acid BF3 is the strongest acid towards the hard base NH3 while BH3 is the strongest towards AsH3.†
Which acid will form the most stable complex with \(\ce{CO}\): \(\ce{BH3}\), \(\ce{BF3}\), or \(\ce{BMe3}\)?
- Answer
-
\(\ce{BH3}\). Since \(\ce{CO}\) forms complexes primarily through its carbon lone pair, it is a soft base and so will form the strongest complex with the softest Lewis acid.
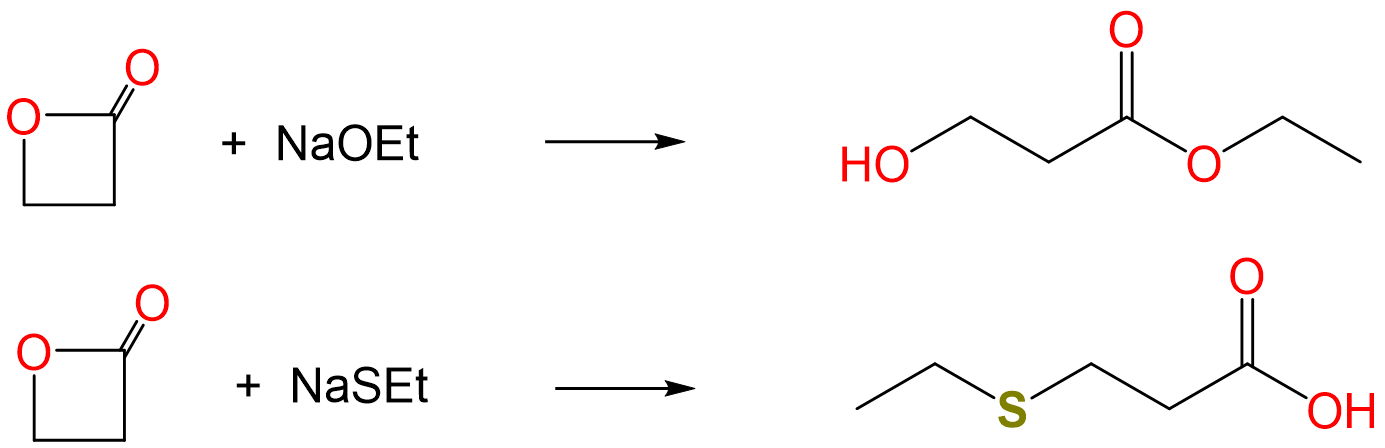
When lactones react with nucleophiles, they can undergo ring opening reactions to give either an alcohol or a carboxylic acid, as shown for propiolactone below:
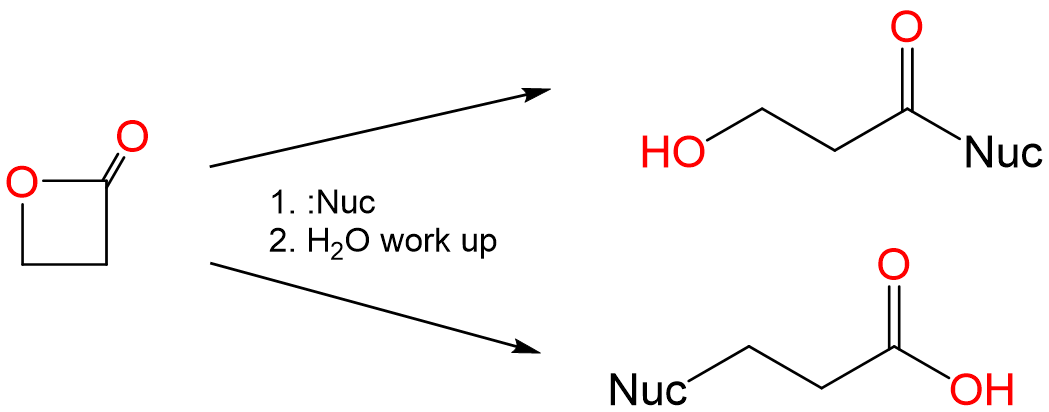
In the reaction above, sterically unhindered alkoxides give one product and sterically unhindered thioalkoxides the other. Explain why this is the case and predict the products of the reaction between propiolactone and the sodium salts of ethoxide and thioethoxide.
- Answer
-
The two reaction products correspond to nucleophilic attack at the lactones' two electrophilic carbon centers. Specifically, the acid is produced by attack at the softer CI center of the CH2 directly attached to the ester oxygen and the alcohol by nucleophilic attack at the harder CIII center of the ester carbonyl.
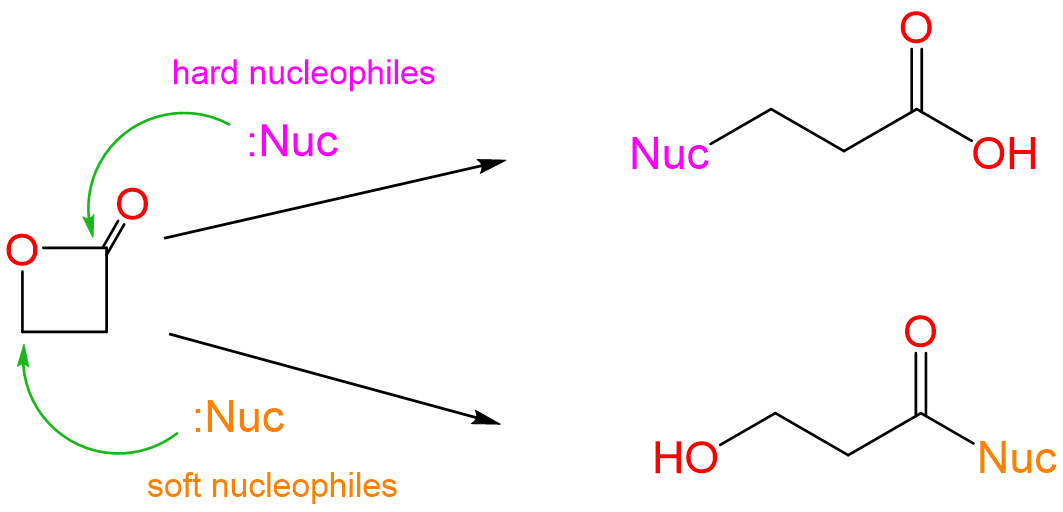
Consequently, it is reasonable to expect that the harder base ethoxide will nucleophilically attack the harder carbonyl carbon while the softer thioethoxide will attack the softer methylene carbon.
The theoretical interpretation of the hard-soft acid-base principle is that hard-hard preferences reflect superior electrostatic stabilization while soft-soft preferences reflect superior covalent stabilization.
The hard-hard and soft-soft preferences in Lewis acid-base interactions reflect that
- The lone pair of a hard base is strongly stabilized electrostatically by a hard acid.
- The lone pair of a soft base is strongly stabilized by forming a covalent bond with a soft acid.
- The lone pair of a hard or soft base is comparatively weakly stabilized by an acid opposite to it in hardness or softness since the overall electrostatic and covalent stabilization of the adduct is comparatively weak.
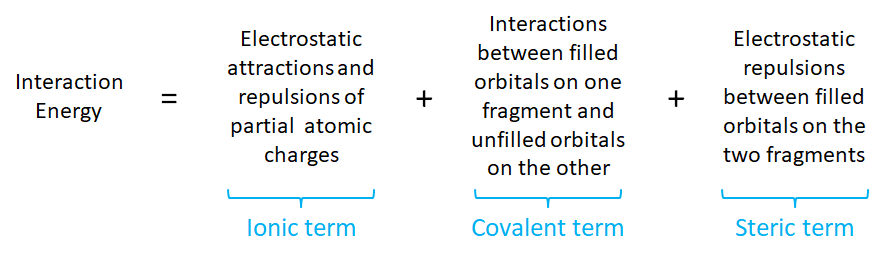
To see why this is the case it is helpful to divide the contributions to the interaction energy between an acid and a base as follows:
 \[ \nonumber \]
\[ \nonumber \]
Of the three contributions to the interaction energy, only the ionic and covalent terms directly relate to the hardness of the interacting acid and base. One approach to thinking about how hardness influences the ionic and covalent contributions is to consider the frontier orbitals involved in the acid-base interaction. This is sometimes done through the use of the Salem-Klopman equation,1,* although in the treatment which follows a more qualitative approach will be employed.
Both hard acids and bases will have comparatively low energy HOMO levels and high energy LUMO levels, with a correspondingly high HOMO-LUMO gap. In contrast, soft acids and bases will have comparatively high-energy HOMO levels and low-energy LUMO levels, giving a comparatively smaller HOMO-LUMO gap.
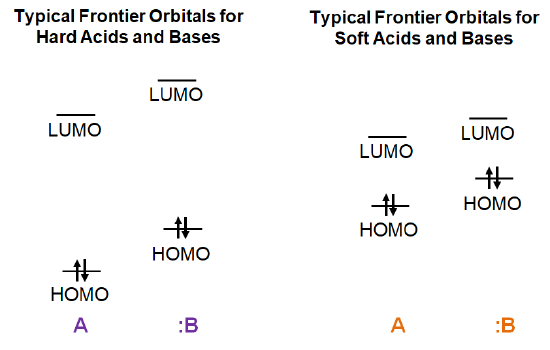
Given this, consider the frontier orbital interactions involved in the formation of an acid-base complex for the possible cases, as illustrated schematically below.
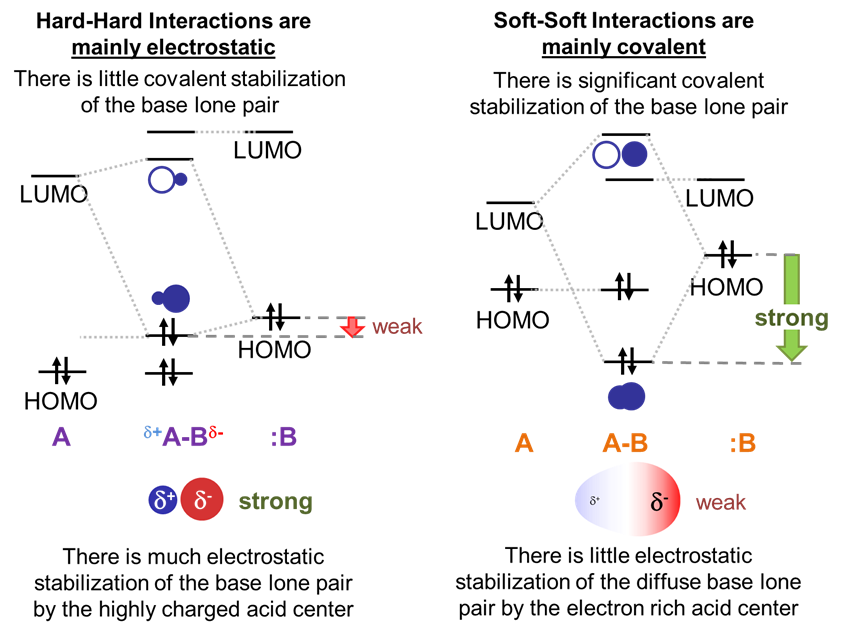
The large gap in energy between hard bases’ highly stabilized HOMO lone pairs and the high energy LUMO of hard acids ensures that in hard acid-hard base adducts the dominant stabilizing interaction will involve electrostatic attraction between the base lone pair and the electropositive Lewis acid center. Fortunately, since the electron clouds in hard bases are relatively dense and electron rich while hard Lewis acids are highly charged and small, these electrostatic interactions are strong.
In contrast, in soft acid-soft base adducts, the dominant stabilizing interaction will be covalent. This is because the small gap in energy between a soft base HOMO and soft acid LUMO enables the formation of a well-stabilized bonding orbital with significant electron density between the acid and base.
The orbitals' interactions between hard acids and soft bases and soft acids and hard bases are intermediate between the hard acid-hard base and soft acid-soft base cases.
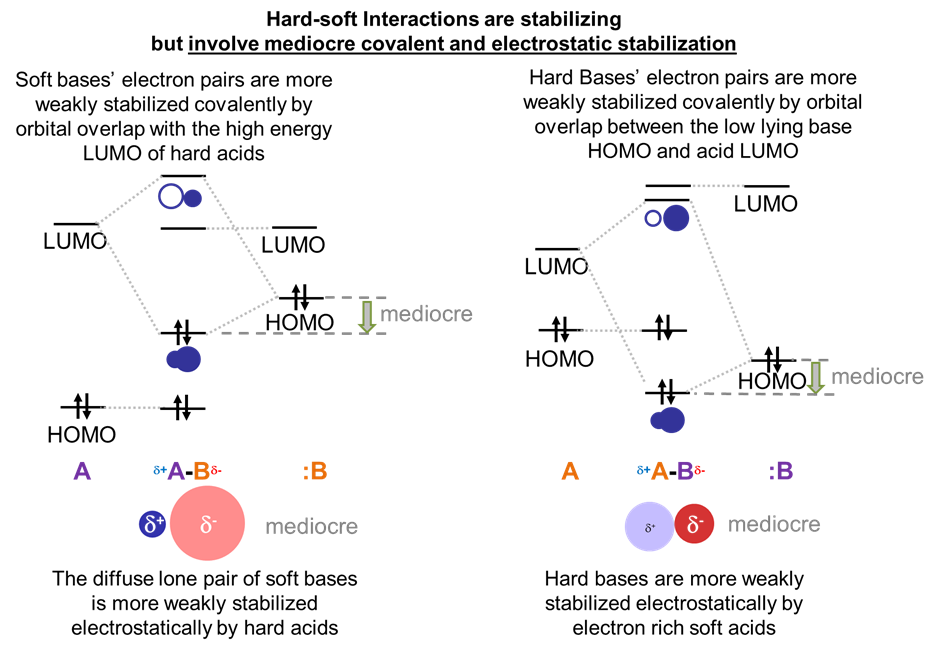
This means that the adducts are stable relative to free acid and base – just not as well stabilized as in the hard acid and hard base case. In the case of hard acids and soft bases the hard acids are less able to stabilize the soft bases’ relatively diffuse electron pair electrostatically and there isn’t as much covalent stabilization as in adducts of soft acids and bases due to the hard acid’s high energy.
References
1. Ahrland, S.; Chatt, J.; Davies, N. R., The relative affinities of ligand atoms for acceptor molecules and ions. Quarterly Reviews, Chemical Society 1958, 12 (3), 265-276.
2. Pearson, R. G., Hard and Soft Acids and Bases. Journal of the American Chemical Society 1963, 85 (22), 3533-3539.
3. Fleming, I., Molecular orbitals and organic chemical reactions. Reference ed.; Wiley: Hoboken, N.J., 2010.
Notes
* Despite the fruitfulness of this observation, in general it is important to reduce the potential for observer bias by checking observations like these against compounds reported in the chemical literature and databases like the Inorganic Crystal Structure and Cambridge Crystallographic Databases.
** These are very soluble in water, to the point where some solutions are perhaps better described as solutions of water in the halide.
† This can be predicted based on the relative hardness of BF3, BR3, and BH3 in the list of hard and soft acids. However, for those of you who may be confused as to why H is considered a better electron donor for the purposes of softening a Lewis acid center while alkyl groups are better electron donors for the purposes of stabilizing carbocations in organic chemistry, the dominant effect is the lower electronegativity of H relative to carbon (in CH3). The effect of electron donation due to hyperconjugation isn't as great for thermodynamically stable bases like BX3/BR3.
†† For more on the Salem-Klopman equation see Fleming, I., Molecular orbitals and organic chemical reactions. Reference ed.; Wiley: Hoboken, N.J., 2010; pp. 138-143.

