6.5.2: Hydrogen bonds may be considered as a special type of Lewis acid-base interaction in which a Lewis acid hydrogen ion is shared between Lewis bases
- Page ID
- 162914
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)A hydrogen bond is a bonding interaction in which an E-H hydrogen bond donor unit acts as a Lewis acid in donating a hydrogen ion to a hydrogen bond acceptor, A.
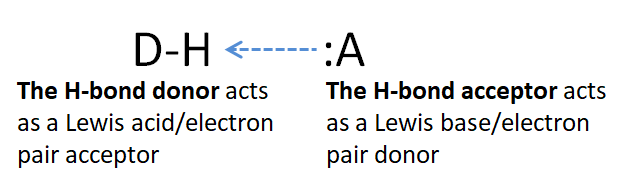
Classically, hydrogen bonds (H-bonds) have been defined as a special type of intermolecular force between D-H and :A, where D & A = N, O, F, and occasionally S. The D-H is said to be the hydrogen bond donor while :A is the hydrogen bond acceptor.

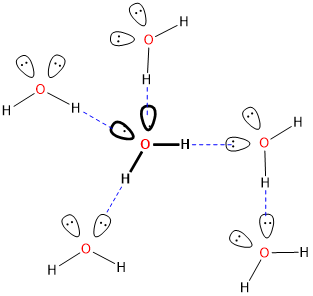
Consider, for instance, a hydrogen bond between two water molecules:
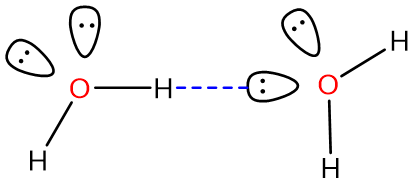
An O-H bond in the water on the left serves as the hydrogen bond donor while one of the lone pairs on the oxygen of the water on the right acts as the hydrogen bond acceptor.* The strength of this classical hydrogen bonding interaction is ~12-20 kJ/mol, much greater than typical dipole-dipole interaction energies (<4 kJ/mol), and even though the hydrogen bonds in water are somewhat flexible, the O-H---O hydrogen bond axis prefers to be linear in many cases [1]. These factors suggest that hydrogen bonds are more than just a special type of dipole-dipole interaction involving hydrogen. Instead, hydrogen bonds involve a combination of factors, including:
- dipole-dipole interactions
- covalent bonding
- dispersion forces
- X-H \(\pi\)-interactions**
The relative importance of these interactions can differ significantly from one system to another.
In considering how to better understand hydrogen bonding, both Brønsted and Lewis acid-base concepts have been employed. In Brønsted terms, hydrogen bonding has been described as involving a partial transfer of a hydrogen ion from D to A. The Lewis definition is often more useful for understanding the role of covalency, however, owing to the ease with which Lewis theory accommodates orbital interactions through the Frontier orbital approach to chemical bonding and reactivity. Moreover, from the classical definition of hydrogen bonding as involving donation of H by a D-H unit and its acceptance by a lone pair on A, it is apparent that in some sense D-H is acting as a Lewis acid in donating an electron-deficient H to A while A is acting as a Lewis base in using its lone pair to accept it. In this respect, the hydrogen bond is perhaps better thought of as involving Lewis acid-base adduct formation between a D-H bond and A.
This more expansive definition of a hydrogen bond as involving a Lewis acid-base interaction accommodates the much wider variety of hydrogen bonding interactions that have been recognized since the 1990s. While the classical definition of hydrogen bonding accounts for much of the hydrogen bonding that occurs in water, organic, and biomolecular systems, the large number of multicenter H-bonds† and the ability of C-H, P-H, and Au-H to function as hydrogen bond donors and for \(\pi\) systems to function as hydrogen bond acceptors is excluded by the classical definition of a hydrogen bond. In contrast, the Lewis-based definition allows for the understanding of covalent contributions to hydrogen bonding in such systems in terms of the overlap of frontier orbitals on D, H, and A. Consider, for example, the frontier orbital interactions involved in a typical hydrogen bond between D-H and A:††
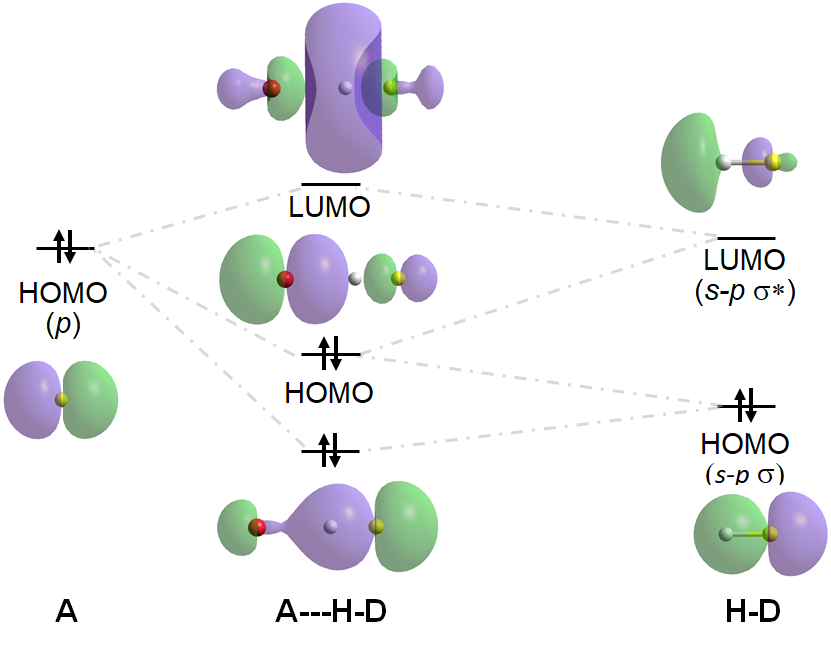
Hydrogen bonds like the one above are called asymmetric hydrogen bonds since the D-H bond is shorter and more stabilized than the A-H bond. When D and A are of equal or similar energy, however, the D-H and A-H interactions are of similar energy, and the H will be centered in between D and A, making it difficult to identify which atom is the donor and which is the acceptor. Such H-bonds are called symmetric H-bonds. An example is the H-bond in [F---H----F]-, for which the stabilizing and destabilizing frontier orbital interactions are
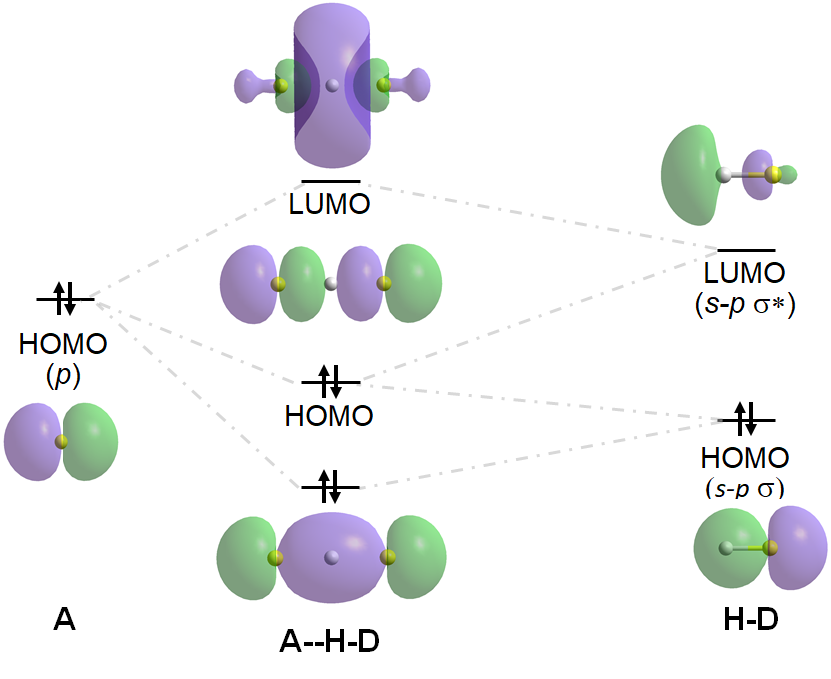
In general, symmetric hydrogen bonds are stronger and more stable than asymmetric ones. In fact, one approach to predicting the strength of classical hydrogen bonds called Gili's pKa Slide Rule‡ considers the pKa of the protonated form of both the neutral donor (D-H) and the neutral acceptor (A, which is then protonated to H-A+). In this approach the H-bond is considered to involve transfer of a hydronium ion between the donor and acceptor, and the pKa values of the donor and acceptor serve as proxies for D- and A's ability to draw the hydrogen ion in the H-bond to itself. When the
- donor is more basic (i.e., its pKa is higher) relatively little transfer occurs, and the hydrogen bond is better represented as D-H----A
- acceptor is more basic (i.e., its pKa is higher) the hydrogen ion is transferred from D to A, giving a hydrogen bond better represented as D----H-A
- donor and acceptor are about equally basic (have similar pKa values) the hydrogen ion is roughly shared equally between D and A in a symmetric H-bond, D--H--A
Specifically, Gili proposed the following criteria for predicting the strength of these ordinary hydrogen bonds:
| Hydrogen Bond type | \( \Delta pK_a\) |
|---|---|
| Strong (11.5-15.8 kcal/mol) | 0-3 |
| Medium Strong (6.8-11.5 kcal/mol) | 3-11 |
| Medium (4.1-6.8) kcal/mol | 11-21 |
| Medium Weak (2.5-4.1 kcal/mol) | 21-31 |
| Weak (1.1-2.5 kcal/mol) | >31 |
Experimentally, the existence of hydrogen bonds and their approximate strength is inferred from crystallographic bond distances and angles, changes in the D-H vibrational frequency, and shifts in 1H NMR peak positions, although all such measures should be interpreted with caution.
If hydrogen bonds involve full or partial coordinate covalent bond formation between a D-H bond and an acceptor site, then the resulting adduct should exhibit evidence of bond formation. In practice such evidence is sought from three main sources:
1. Crystallographic bond distances and angles. In the structure of crystalline solids, hydrogen bonds can be distinguished from Van der Waals contacts in that
- the D----A distance is closer than that expected from the Van der Waals surfaces of D and A.
- when the H can be located, the D-H distance is longer than non-hydrogen-bonded D-H bonds in similar systems.
- for two-centered hydrogen bonds unconstrained by external factors, the D-H---A angle will approach linearity, as VSEPR theory would predict for a D:H:A unit.
2. D-H vibrational frequencies. Since hydrogen bonding involves weakening of the D-H bond and the formation of an H-A bond, in theory both the D-H and A-H vibrational modes will be found in the IR spectrum of a hydrogen-bonded system, albeit redshifted to lower wavenumbers than the unweakened D-H and A-H bonds. In practice, the shift in the D-H vibrational band to lower wavenumbers (along with a broadening and intensifying of the band) is taken as evidence for hydrogen bonding (Figure 6.5.1.1).
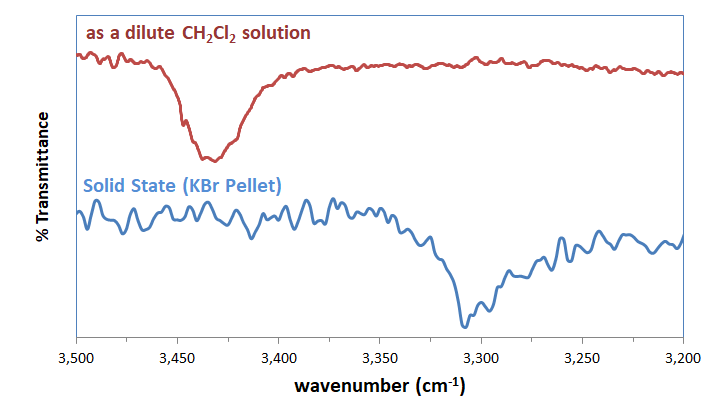
3. Shifts in the 1H NMR peak position of the donor hydrogen. As the hydrogen is partially transferred from D to A it becomes deshielded and so is shifted downfield, sometimes by as much as several ppm.
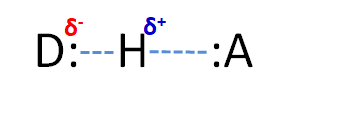
Care should be taken in applying these criteria since:
- The criteria work best for classical hydrogen bonded systems. Other aspects of the bonding in a given system can influence apparent bond strengths and NMR chemical shifts, particularly for intramolecular H-bonds and in nonclassical hydrogen bonding systems.
- Stronger hydrogen bonds are more likely to adhere to these criteria than weaker ones. Moreover, stronger hydrogen bonds generally exhibit greater linearity, shorter distances relative to those expected from Van der Waals radii, larger stretching frequency lowering for D-H, and greater downfield shifts in their NMR spectra.
References and Further Reading
- Steiner, T., The Hydrogen Bond in the Solid State. Angewandte Chemie International Edition 2002, 41 (1), 48-76.
- Grabowski, S.J. Hydrogen Bonding—New Insights; Springer: Dordrecht, The Netherlands, 2006.
- Gilli G., Gilli P. The Nature of the Hydrogen Bond, Oxford University Press; Oxford, UK: 2009.
- Scheiner, S., Special Issue: Intramolecular Hydrogen Bonding 2017. Molecules 2017, 22 (9), 1521.
- Schmidbaur, H.; Raubenheimer, H. G.; Dobrzańska, L., The gold–hydrogen bond, Au–H, and the hydrogen bond to gold, Au⋯H–X. Chemical Society Reviews 2014, 43 (1), 345-380.
- Gilli, P.; Pretto, L.; Bertolasi, V.; Gilli, G., Predicting Hydrogen-Bond Strengths from Acid−Base Molecular Properties. The pKa Slide Rule: Toward the Solution of a Long-Lasting Problem. Accounts of Chemical Research 2009, 42 (1), 33-44.
- Laurence, C.; Gal, J.-F. o., Lewis basicity and Affinity scales: Data and Measurement. John Wiley: Chichester, West Sussex, U.K., 2010.
- Scheiner, S., Assessment of the Presence and Strength of H-Bonds by Means of Corrected NMR. Molecules 2016, 21 (11), 1426.
- Nícha, J.; Foroutan-Nejad, C.; Straka, M., 1H NMR is not a proof of hydrogen bonds in transition metal complexes. Nature Communications 2019, 10 (1), 1643.
Notes
* Of course in solutions where there are many hydrogen bonding interactions many of the water molecules might simultaneously act as a hydrogen bond donor and acceptor.
** X-H \(\pi\) interactions are interactions between the partial positive charge on the H end of a polar X-H bond and a negatively-charged \(\pi\)-bond. These are particularly for understanding hydrogen bonded systems in which as \(\pi\)-system serves as the hydrogen bond acceptor,. Examples of X-H interactions in which benzene is the acceptor are illustrated below.

† In a multicenter H-bond the D-H is donated to multiple acceptors simultaneously.
†† The MOs shown in the diagrams were calculated by performing ab initio calculations at the 6-31G** level. Those for the symmetric H-bond correspond to FHF- and the assymetric H bond to OHF-.
‡ So called because it involves a sliding bar chart with common classes of H-bond donors and acceptors that may be slid along the rule in a manner similar to the operation of a pre-late 20th Century device for performing mathematical computations called a slide rule.
‡‡ for example, intramolecular hydrogen bonds are sometimes constrained to by the overall geometry of the interacting groups.

