7.2: Heat
- Page ID
- 24202
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\dsum}{\displaystyle\sum\limits} \)
\( \newcommand{\dint}{\displaystyle\int\limits} \)
\( \newcommand{\dlim}{\displaystyle\lim\limits} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)- Distinguish the related properties of heat, thermal energy, and temperature
- Define and distinguish specific heat and heat capacity, and describe the physical implications of both
- Perform calculations involving heat, specific heat, and temperature change
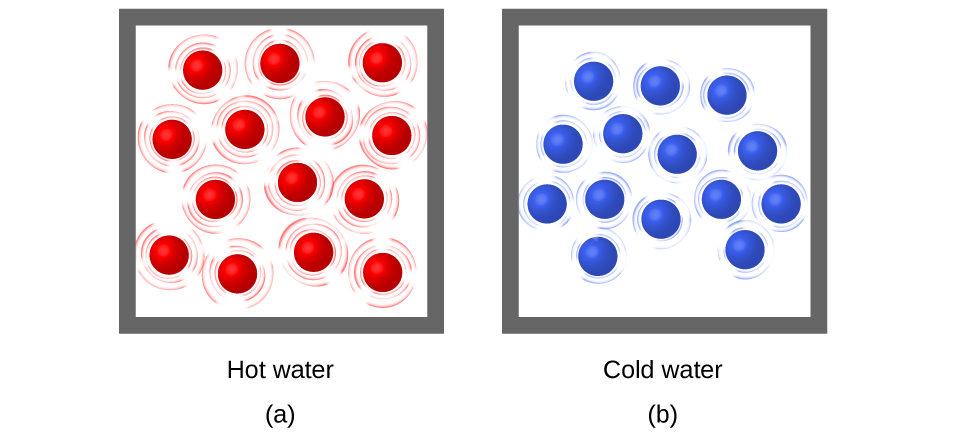
Thermal energy is kinetic energy associated with the random motion of atoms and molecules. Temperature is a quantitative measure of “hot” or “cold.” When the atoms and molecules in an object are moving or vibrating quickly, they have a higher average kinetic energy (KE), and we say that the object is “hot.” When the atoms and molecules are moving slowly, they have lower KE, and we say that the object is “cold” (Figure \(\PageIndex{1}\)). Assuming that no chemical reaction or phase change (such as melting or vaporizing) occurs, increasing the amount of thermal energy in a sample of matter will cause its temperature to increase. And, assuming that no chemical reaction or phase change (such as condensation or freezing) occurs, decreasing the amount of thermal energy in a sample of matter will cause its temperature to decrease.
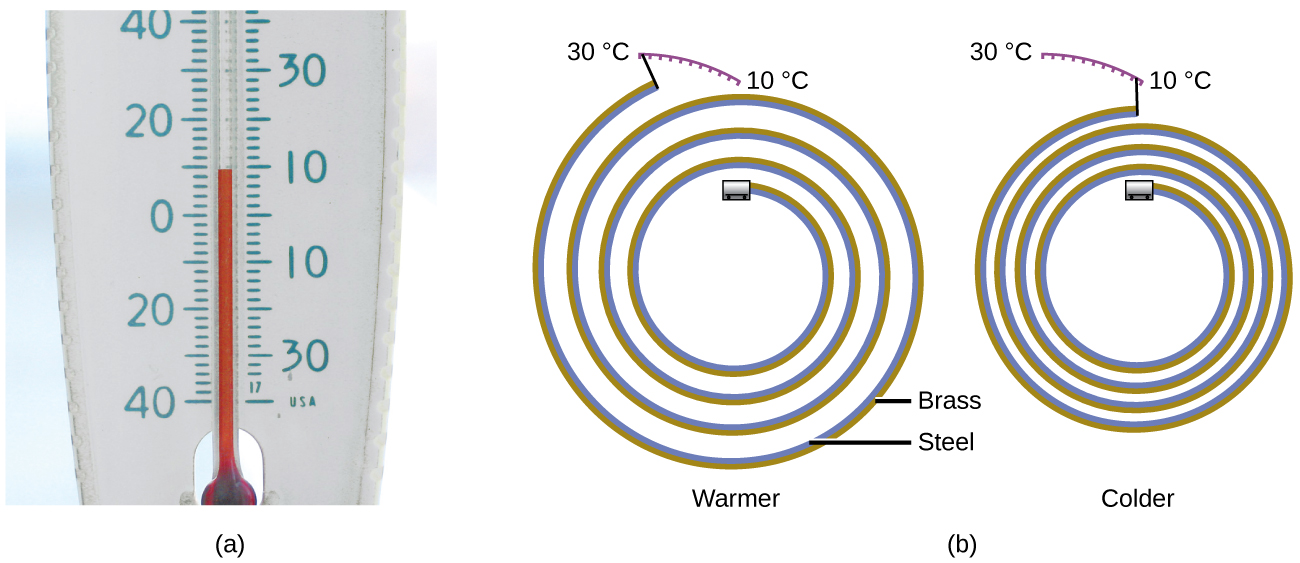
Most substances expand as their temperature increases and contract as their temperature decreases. This property can be used to measure temperature changes, as shown in Figure \(\PageIndex{2}\). The operation of many thermometers depends on the expansion and contraction of substances in response to temperature changes.

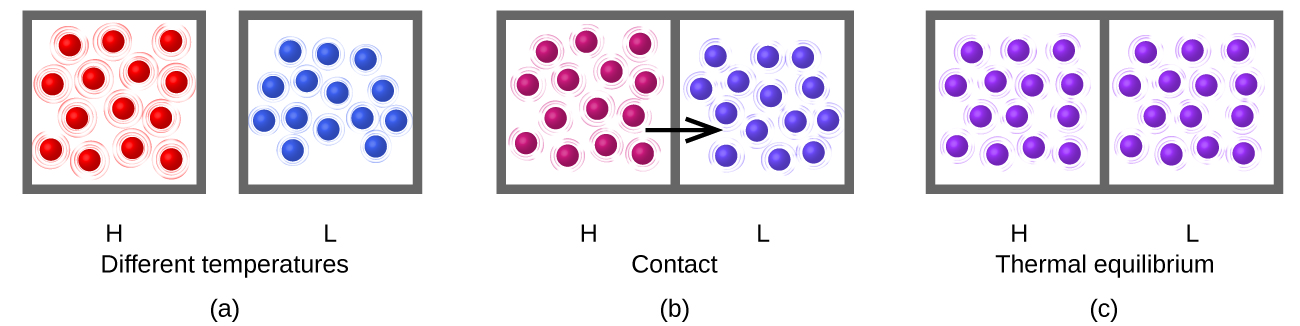
Heat (q) is the transfer of thermal energy between two bodies at different temperatures. Heat flow (a redundant term, but one commonly used) increases the thermal energy of one body and decreases the thermal energy of the other. Suppose we initially have a high temperature (and high thermal energy) substance (H) and a low temperature (and low thermal energy) substance (L). The atoms and molecules in H have a higher average KE than those in L. If we place substance H in contact with substance L, the thermal energy will flow spontaneously from substance H to substance L. The temperature of substance H will decrease, as will the average KE of its molecules; the temperature of substance L will increase, along with the average KE of its molecules. Heat flow will continue until the two substances are at the same temperature (Figure \(\PageIndex{3}\)).
Matter undergoing chemical reactions and physical changes can release or absorb heat. A change that releases heat is called an exothermic process. For example, the combustion reaction that occurs when using an oxyacetylene torch is an exothermic process—this process also releases energy in the form of light as evidenced by the torch’s flame (Figure \(\PageIndex{4}\)a). A reaction or change that absorbs heat is an endothermic process. A cold pack used to treat muscle strains provides an example of an endothermic process. When the substances in the cold pack (water and a salt like ammonium nitrate) are brought together, the resulting process absorbs heat, leading to the sensation of cold.

Historically, energy was measured in units of calories (cal). A calorie is the amount of energy required to raise one gram of water by 1 degree C (1 kelvin). However, this quantity depends on the atmospheric pressure and the starting temperature of the water. The ease of measurement of energy changes in calories has meant that the calorie is still frequently used. The Calorie (with a capital C), or large calorie, commonly used in quantifying food energy content, is a kilocalorie. The SI unit of heat, work, and energy is the joule. A joule (J) is defined as the amount of energy used when a force of 1 newton moves an object 1 meter. It is named in honor of the English physicist James Prescott Joule. One joule is equivalent to 1 kg m2/s2, which is also called 1 newton–meter. A kilojoule (kJ) is 1000 joules. To standardize its definition, 1 calorie has been set to equal 4.184 joules.
Heat Capacity
We now introduce two concepts useful in describing heat flow and temperature change. The heat capacity (C) of a body of matter is the quantity of heat (q) it absorbs or releases when it experiences a temperature change (ΔT) of 1 degree Celsius (or equivalently, 1 kelvin)
\[C=\dfrac{q}{ΔT} \label{7.2.1}\]
Heat capacity is determined by both the type and amount of substance that absorbs or releases heat. It is therefore an extensive property—its value is proportional to the amount of the substance.
For example, consider the heat capacities of two cast iron frying pans. The heat capacity of the large pan is five times greater than that of the small pan because, although both are made of the same material, the mass of the large pan is five times greater than the mass of the small pan. More mass means more atoms are present in the larger pan, so it takes more energy to make all of those atoms vibrate faster. The heat capacity of the small cast iron frying pan is found by observing that it takes 18,150 J of energy to raise the temperature of the pan by 50.0 °C
\[C_{\text{small pan}}=\dfrac{18,140 J}{50.0\; °C} =363\; J/°C \label{7.2.2}\]
The larger cast iron frying pan, while made of the same substance, requires 90,700 J of energy to raise its temperature by 50.0 °C. The larger pan has a (proportionally) larger heat capacity because the larger amount of material requires a (proportionally) larger amount of energy to yield the same temperature change:
\[C_{\text{large pan}}=\dfrac{90,700\; J}{50.0\;°C}=1814\; J/°C \label{7.2.3}\]
The specific heat capacity (c) of a substance, commonly called its “specific heat,” is the quantity of heat required to raise the temperature of 1 gram of a substance by 1 degree Celsius (or 1 kelvin):
\[c = \dfrac{q}{m\Delta T} \label{7.2.4}\]
Specific heat capacity depends only on the kind of substance absorbing or releasing heat. It is an intensive property—the type, but not the amount, of the substance is all that matters. For example, the small cast iron frying pan has a mass of 808 g. The specific heat of iron (the material used to make the pan) is therefore:
\[c_{iron}=\dfrac{18,140\; J}{(808\; g)(50.0\;°C)} = 0.449\; J/g\; °C \label{7.2.5}\]
The large frying pan has a mass of 4040 g. Using the data for this pan, we can also calculate the specific heat of iron:
\[c_{iron}=\dfrac{90,700 J}{(4,040\; g)(50.0\;°C)}=0.449\; J/g\; °C \label{7.2.6}\]
Although the large pan is more massive than the small pan, since both are made of the same material, they both yield the same value for specific heat (for the material of construction, iron). Note that specific heat is measured in units of energy per temperature per mass and is an intensive property, being derived from a ratio of two extensive properties (heat and mass). The molar heat capacity, also an intensive property, is the heat capacity per mole of a particular substance and has units of J/mol °C (Figure \(\PageIndex{5}\)).

The heat capacity of an object depends on both its mass and its composition. For example, doubling the mass of an object doubles its heat capacity. Consequently, the amount of substance must be indicated when the heat capacity of the substance is reported. The molar heat capacity (Cp) is the amount of energy needed to increase the temperature of 1 mol of a substance by 1°C; the units of Cp are thus J/(mol•°C).The subscript p indicates that the value was measured at constant pressure. The specific heat (Cs) is the amount of energy needed to increase the temperature of 1 g of a substance by 1°C; its units are thus J/(g•°C).
We can relate the quantity of a substance, the amount of heat transferred, its heat capacity, and the temperature change either via moles (Equation 7.3.2) or mass (Equation 7.3.3):
\[q = nC_pΔT \label{7.3.2}\]
where
- \(n\) is the number of moles of substance and
- \(C_p\) is the molar heat capacity (i.e., heat capacity per mole of substance)
\[q = mC_sΔT \label{7.3.3}\]
where
The specific heats of some common substances are given in Table \(\PageIndex{1}\). Note that the specific heat values of most solids are less than 1 J/(g•°C), whereas those of most liquids are about 2 J/(g•°C). Water in its solid and liquid states is an exception. The heat capacity of ice is twice as high as that of most solids; the heat capacity of liquid water, 4.184 J/(g•°C), is one of the highest known.
Liquid water has a relatively high specific heat (about 4.2 J/g °C); most metals have much lower specific heats (usually less than 1 J/g °C). The specific heat of a substance varies somewhat with temperature. However, this variation is usually small enough that we will treat specific heat as constant over the range of temperatures that will be considered in this chapter. Specific heats of some common substances are listed in Table \(\PageIndex{1}\).
| Substance | Symbol (state) | Specific Heat (J/g °C) |
|---|---|---|
| helium | He(g) | 5.193 |
| water | H2O(l) | 4.184 |
| ethanol | C2H6O(l) | 2.376 |
| ice | H2O(s) | 2.093 (at −10 °C) |
| water vapor | H2O(g) | 1.864 |
| nitrogen | N2(g) | 1.040 |
| air | mixture | 1.007 |
| oxygen | O2(g) | 0.918 |
| aluminum | Al(s) | 0.897 |
| carbon dioxide | CO2(g) | 0.853 |
| argon | Ar(g) | 0.522 |
| iron | Fe(s) | 0.449 |
| copper | Cu(s) | 0.385 |
| lead | Pb(s) | 0.130 |
| gold | Au(s) | 0.129 |
| silicon | Si(s) | 0.712 |
The value of C is intrinsically a positive number, but ΔT and q can be either positive or negative, and they both must have the same sign. If ΔT and q are positive, then heat flows from the surroundings into an object. If ΔT and q are negative, then heat flows from an object into its surroundings.
If we know the mass of a substance and its specific heat, we can determine the amount of heat, q, entering or leaving the substance by measuring the temperature change before and after the heat is gained or lost:
\[\begin{align}q&=\ce{(specific\: heat)×(mass\: of\: substance)×(temperature\: change)}\label{7.2.7}\\q&=c×m×ΔT=c×m×(T_\ce{final}−T_\ce{initial})\end{align}\]
In this equation, \(c\) is the specific heat of the substance, m is its mass, and ΔT (which is read “delta T”) is the temperature change, Tfinal − Tinitial. If a substance gains thermal energy, its temperature increases, its final temperature is higher than its initial temperature, Tfinal − Tinitial has a positive value, and the value of q is positive. If a substance loses thermal energy, its temperature decreases, the final temperature is lower than the initial temperature, Tfinal − Tinitial has a negative value, and the value of q is negative.
The Movement of Heat in a Substance: https://youtu.be/gaJQYke-lVY
A flask containing \(8.0 \times 10^2\; g\) of water is heated, and the temperature of the water increases from 21 °C to 85 °C. How much heat did the water absorb?
Solution
To answer this question, consider these factors:
- the specific heat of the substance being heated (in this case, water)
- the amount of substance being heated (in this case, 800 g)
- the magnitude of the temperature change (in this case, from 21 °C to 85 °C).
The specific heat of water is 4.184 J/g °C, so to heat 1 g of water by 1 °C requires 4.184 J. We note that since 4.184 J is required to heat 1 g of water by 1 °C, we will need 800 times as much to heat 800 g of water by 1 °C. Finally, we observe that since 4.184 J are required to heat 1 g of water by 1 °C, we will need 64 times as much to heat it by 64 °C (that is, from 21 °C to 85 °C).
This can be summarized using the equation:
\[ \begin{align} q &=c×m×ΔT=c\times m \times (T_\ce{final}−T_\ce{initial}) \\ &=\mathrm{(4.184\:J/\cancel{g}°C)×(800\:\cancel{g})×(85−20)°C}\\
&=\mathrm{(4.184\:J/\cancel{g}°\cancel{C})×(800\:\cancel{g})×(65)°\cancel{C}}\\
&=\mathrm{210,000\: J(=210\: kJ)}
\end{align}\]
Because the temperature increased, the water absorbed heat and \(q\) is positive.
How much heat, in joules, must be added to a \(5.00 \times 10^2 \;g\) iron skillet to increase its temperature from 25 °C to 250 °C? The specific heat of iron is 0.451 J/g °C.
Answer:\(5.05 \times 10^4\; J\)
Note that the relationship between heat, specific heat, mass, and temperature change can be used to determine any of these quantities (not just heat) if the other three are known or can be deduced.
A piece of unknown metal weighs 348 g. When the metal piece absorbs 6.64 kJ of heat, its temperature increases from 22.4 °C to 43.6 °C. Determine the specific heat of this metal (which might provide a clue to its identity).
Solution
Since mass, heat, and temperature change are known for this metal, we can determine its specific heat using the relationship:
\[q=c \times m \times \Delta T=c \times m \times (T_{final}−T_{initial})\]
Substituting the known values:
\[6,640\; J=c \times (348\; g) \times (43.6 − 22.4)\; °C\]
Solving:
\[c=\dfrac{6,640\; J}{(348\; g) \times (21.2°C)} =0.900\; J/g\; °C\]
Comparing this value with the values in Table \(\PageIndex{1}\), this value matches the specific heat of aluminum, which suggests that the unknown metal may be aluminum.
A piece of unknown metal weighs 217 g. When the metal piece absorbs 1.43 kJ of heat, its temperature increases from 24.5 °C to 39.1 °C. Determine the specific heat of this metal, and predict its identity.
Answer\(c = 0.45 \;J/g \;°C\); the metal is likely to be iron from checking Tabel 7.2.1
A home solar energy storage unit uses 400 L of water for storing thermal energy. On a sunny day, the initial temperature of the water is 22.0°C. During the course of the day, the temperature of the water rises to 38.0°C as it circulates through the water wall. How much energy has been stored in the water? (The density of water at 22.0°C is 0.998 g/mL.)
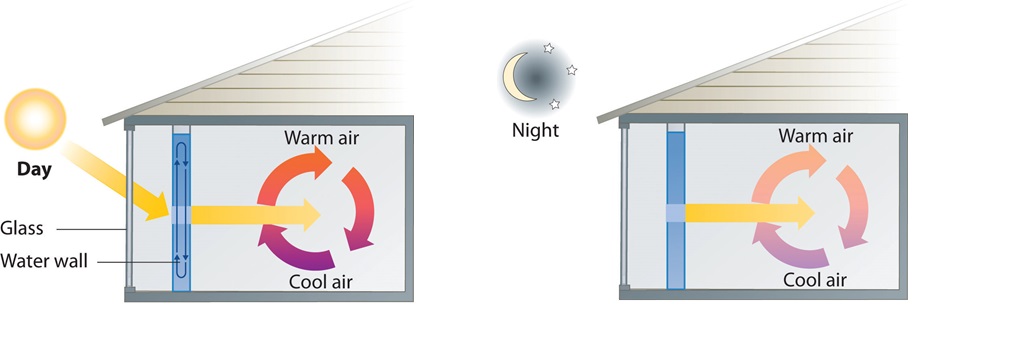
Given: volume and density of water and initial and final temperatures
Asked for: amount of energy stored
Strategy:
- Use the density of water at 22.0°C to obtain the mass of water (m) that corresponds to 400 L of water. Then compute ΔT for the water.
- Determine the amount of heat absorbed by substituting values for m, Cs, and ΔT into Equation 7.3.1.
Solution:
A The mass of water is
\[ mass \; of \; H_{2}O=400 \; \cancel{L}\left ( \dfrac{1000 \; \cancel{mL}}{1 \; \cancel{L}} \right ) \left ( \dfrac{0.998 \; g}{1 \; \cancel{mL}} \right ) = 3.99\times 10^{5}g\; H_{2}O \]
The temperature change (ΔT) is 38.0°C − 22.0°C = +16.0°C.
B From Table \(\PageIndex{1}\), the specific heat of water is 4.184 J/(g•°C). From Equation 7.3.3, the heat absorbed by the water is thus
\[ q=mC_{s}\Delta T=\left ( 3.99X10^{5} \; \cancel{g} \right )\left ( \dfrac{4.184 \; J}{\cancel{g}\cdot \cancel{^{o}C}} \right ) \left ( 16.0 \; \cancel{^{o}C} \right ) = 2.67 \times 10^{7}J = 2.67 \times 10^{4}kJ \]
Both q and ΔT are positive, consistent with the fact that the water has absorbed energy.
Some solar energy devices used in homes circulate air over a bed of rocks that absorb thermal energy from the sun. If a house uses a solar heating system that contains 2500 kg of sandstone rocks, what amount of energy is stored if the temperature of the rocks increases from 20.0°C to 34.5°C during the day? Assume that the specific heat of sandstone is the same as that of quartz (SiO2) in Table \(\PageIndex{1}\).
Answer
2.7 × 104 kJ (Even though the mass of sandstone is more than six times the mass of the water in Example \(\PageIndex{1}\), the amount of thermal energy stored is the same to two significant figures.)
When two objects at different temperatures are placed in contact, heat flows from the warmer object to the cooler one until the temperature of both objects is the same. The law of conservation of energy says that the total energy cannot change during this process:
\[q_{cold} + q_{hot} = 0 \label{7.3.4}\]
The equation implies that the amount of heat that flows from a warmer object is the same as the amount of heat that flows into a cooler object. Because the direction of heat flow is opposite for the two objects, the sign of the heat flow values must be opposite:
\[q_{cold} = −q_{hot} \label{7.3.5}\]
Thus heat is conserved in any such process, consistent with the law of conservation of energy.
The amount of heat lost by a warmer object equals the amount of heat gained by a cooler object.
Substituting for q from Equation 7.3.2 gives
\[ \left [ mC_{s} \Delta T \right ] _{cold} + \left [ mC_{s} \Delta T \right ] _{hot}=0 \label{7.3.6} \]
which can be rearranged to give
\[ \left [ mC_{s} \Delta T \right ] _{cold} = - \left [ mC_{s} \Delta T \right ] _{hot} \label{7.3.7} \]
When two objects initially at different temperatures are placed in contact, we can use Equation 7.3.7 to calculate the final temperature if we know the chemical composition and mass of the objects.
If a 30.0 g piece of copper pipe at 80.0°C is placed in 100.0 g of water at 27.0°C, what is the final temperature? Assume that no heat is transferred to the surroundings.
Given: mass and initial temperature of two objects
Asked for: final temperature
Strategy: Using Equation 7.3.7 and writing ΔT as Tfinal − Tinitial for both the copper and the water, substitute the appropriate values of m, Cs, and Tinitial into the equation and solve for Tfinal.
Solution
We can adapt Equation 7.3.7 to solve this problem, remembering that ΔT is defined as Tfinal − Tinitial:\[ \left [ mC_{s} \left (T_{final} - T_{initial} \right ) \right ] _{Cu} + \left [ mC_{s} \left (T_{final} - T_{initial} \right ) \right ] _{H_{2}O} =0 \]
Substituting the data provided in the problem and Table \(\PageIndex{1}\) gives
\[ \left [ \left (30 \; g \right ) \left (0.385 \; J \right ) \left (T_{final} - T_{initial} \right ) \right ] _{Cu} + \left [ mC_{s} \left (T_{final} - T_{initial} \right ) \right ] _{H_{2}O} =0 \]
\[ T_{final}\left ( 11.6 \; J/ ^{o}C \right ) -924 \; J + T_{final}\left ( 418.4 \; J/ ^{o}C \right ) -11,300 \; J \]
\[ T_{final}\left ( 430 \; J/\left ( g\cdot ^{o}C \right ) \right ) = -12,224 \; J \]
\[ T_{final} = -28.4 \; ^{o}C \]
If a 14.0 g chunk of gold at 20.0°C is dropped into 25.0 g of water at 80.0°C, what is the final temperature if no heat is transferred to the surroundings?
Answer: 80.0°C
A 28.0 g chunk of aluminum is dropped into 100.0 g of water with an initial temperature of 20.0°C. If the final temperature of the water is 24.0°C, what was the initial temperature of the aluminum? (Assume that no heat is transferred to the surroundings.)
Answer: 90.6°C
Conservation of Energy: The Movement of Heat between Substances: https://youtu.be/pGEYy-pNHBg
Contributors and Attributions
- Anonymous
Paul Flowers (University of North Carolina - Pembroke), Klaus Theopold (University of Delaware) and Richard Langley (Stephen F. Austin State University) with contributing authors. Textbook content produced by OpenStax College is licensed under a Creative Commons Attribution License 4.0 license. Download for free at http://cnx.org/contents/85abf193-2bd...a7ac8df6@9.110).

