HW Solutions #4
- Page ID
- 2847
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)
These homework problems are suggested and will not be turned in for review. However, answers will be available for them the following week by your class TAs. For more homework feel free to go to the Homework page.
Week 4: Coordination Chemistry 2-5, 9-13 and the below additional questions:
2. When silver nitrate is added to a solution of a substance with the empirical formula CoCl3 · 5NH3, how many moles of AgCl will be precipitated per mole of cobalt present? Why?
Cobalt(III) forms an octahedral complex, so must have six ligands. So, the five ammonia groups and one chloride are in the inner sphere, leaving two Cl- to react. So, there are 2 moles of chloride ions per 1 mole of cobalt(III) in solution. These means, assuming silver nitrate is in excess, 2 moles of silver chloride will precipitate.
Sean Gottlieb
3. Co(III) occurs in octahedral complexes compounds with the general empirical formula CoClm · nNH3. What values of n and m are possible? What are the values of n and m for the complex that precipitates 1 mole of AgCl for every mole of Co present?
Co(III) must have six ligands to form an octahedral complex. Also, it must have enough chloride ions to neutralize its 3+ charge. So, m must equal 3. With that known, it must have enough ammonia groups to achieve its octahedral structure, regardless of how many chloride ions are present. So, n can equal 6, 5, or 4 or 3.
For the second questions. n=4 and m=3. Since only the free Cl- ions will precipitate with AgCl added. The other two Cl- will be bound to the Co and will not precipitate.
Sean Gottlieb
4. How many ions per mole will you expect to find in solution when a compound with the empirical formula PtCl4- · 3NH3 is dissolved in water? What about PtCl2 · 3NH3? Draw diagrams of each of the complex cations.
PtCl4-: 3 ions [Pt(NH3)2Cl2]+ Cl- Cl- NH3
PtCl2-: 0 ions [Pt(NH3)2Cl2] NH3
5. Each of the following is dissolved in water to make a 0.001 M solution. Rank the compounds in order of decreasing conductivity of their solutions; K2PtCl6, Co(NH3)6Cl3, Cr(NH3)4Cl3, Pt(NH3)6Cl4. Rewrite each compound by using brackets to distinguish the complex ion present in aqueous solution.
Pt(NH3)6Cl4 [Pt(NH3)6]4- 4 Cl- 5 ions Most conductive
Co(NH3)6Cl3 [Co(NH3)6]3+ 3 Cl- 4 ions
K2PtCl6 2 K+ [PtCl6]2- 3 ions
Cr(NH3)4Cl3 [Cr(NH3)4Cl2]3- 1 Cl- 2 ions Least conductive
9. How many isomers are there of the compound [Cr(NH3)4Cl2]Cl? Sketch them.
2 isomers
10. Sketch all the geometrical and optical isomers of PtCl2l2(NH3)2.
11. How many geometrical and optical isomers are there of the complex ion Co(en)2Cl? Of these, how many pairs of isomers are there differing only by a mirror reflection? How many isomers have a plane of symmetry and hence do not exist in pairs of optical isomers?
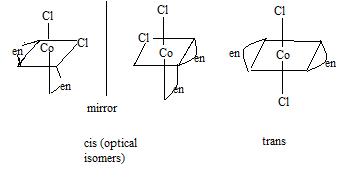
2 geometric isomers (cis and trans)
2 optical isomers (both cis) - one is mirror image of the other - this is the pair of isomers differing only by a mirror reflection
The trans isomer has a plane of symmetry containing both Cl atoms and the Co atom, so it does not exist in a pair of optical isomers.
12. Repeat Problem 11 with propylenediamine substituted for ethylenediamine. Ignore optical isomers from the propylene carbon.
13. How many different structural isomers are there of a substance with the empirical formula FeBrCl · 3NH3 · 2H2O? For each different structural isomer, how many different geometrical isomers exist? How many of these can be grouped into right-handed and left-handed pairs of optical isomers?
1. What is the definition of a transition metal?
- An element that has its s orbitals partially filled
- An element that has its p orbitals partially filled
- An element that has its d orbitals partially filled
- An element that has its f orbitals partially filled
2. Which of the following statements about transition metals is true?
- Typical transition metals have boiling points greater than 1000°C (TRUE)
- Typical transition metals have melting points greater than 1000°C
- Typical transition metals have melting points less than 1000°C
- Transition metals are less dense than Group I metals
3. What is the electronic configuration of Cr?
- [Ar] 3d3 4s2
- [Ar] 3d5 4s2
- [Ar] 3d4 4s2
- [Ar] 3d5 4s1 (TRUE)
4. Why is Mn2+ not readily oxidized to Mn3+?
- This is because the electronic configuration of Mn2+ is more stable than that of Mn3 (TRUE)
- This is because the electronic configuration of Mn2+ is less stable than that of Mn3
- This is because Mn2+ does not have any more electrons in its d orbitals
- This is because the Mn2+ cannot accept any more electrons in to its d orbital
5. In which order are the third and fourth level s, p and electrons filled in?
- 3s, 3p, 3d, 4s
- 3s, 3p, 4s, 3d
- 3s, 4s, 3p, 3d
- 4s, 3s, 3p, 3d
6. Which transition metal shows the greatest variation in possible oxidation numbers?
- V
- Cr
- Mn
- Fe
7. Why is Fe3+ more stable than Fe2+?
- The Fe3+ ion has a more stable 3d5 4s1 electronic configuration
- The Fe2+ ion has a more stable 3d5 4s1 electronic configuration
- The Fe3+ ion has a more stable 3d5 4s2 electronic configuration
- The Fe3+ ion has a more stable 3d4 4s2 electronic configuration
8. Some transition metals exhibit paramagnetism. What does this mean?
- The metal is attracted to a magnet
- The metal is repelled by a magnet
- The metal acts like the north pole of a magnet
- The metal acts like the south pole of a magnet
9. What is meant by the term 'ligand'?
- A substance that can donate a lone pair of electrons to form a dative bond
- A substance that can accept a lone pair of electrons to form a dative bond
- A substance that can accept a lone pair of electrons to form hydrogen bond
- A substance that can donate a lone pair of electrons to form a hydrogen bond
11. Write the electron configurations of the following d-block elements.
a) Vanadium
b) Manganese
c) Copper
d) Zirconium
12. Transition element and d-block are 2 terms that are easily confused..
- Using the appropriate definitions, explain why Zn and Sc are not Transition metals.
"an element whose atom has an incomplete d sub-shell, or which can give rise to cations with an incomplete d sub-shell."
Zn maintains a full d10 shell by losing s electrons.
Sc loses it's d electron very quickly to have no d shell.
Therefore Sc and Zn do not display the incomplete d character necessary for the strict definition of a transition metal.
- What would the oxidation states of the ions of Sc and Zn be?
Zn2+ Sc3+ (Zn loses two electrons from the 4s shell, Sc loses two electrons from the 4s shell as well as 1d electron)
13. What are the oxidation states of the transition metal in each of the following compounds?
- KMnO4 Mn=+7 KMnO4 : Solve for Mn; (+1)+Mn+4(-2) = 0
- Na2CrO4 Cr=+6 Na2CrO4: Solve for Cr; 2(+1)+Cr+4(-2)=0
- CrO3 Cr=+6 CrO3:Solve for Cr; Cr+3(-2)=0
- MnO2 Mn=+4 MnO2: Solve for Mn; Mn+2(-2)=0
- Na2Fe2O4 Fe=+3 Na2Fe2O4: Solve for Fe; 2(+1)+2(Fe)+4(-2)=0
- Mn2(CO)10 Mn=0 Mn2(CO)10: 2(Mn)+10(0)=0
14. Write the electronic configuration of the following atoms or ions.
- Fe [Ar] 3d64s2
- Mn2+ [Ar] 3d5
- V3+ [Ar] 3d2
- Cu [Ar] 3d104s1
- Cu+ [Ar] 3d10
- Cu2+ [Ar] 3d9
15. Describe the bonding in Co(en)33+ in terms of the simplest possible model.
Each en ligand forms two bonds with Co, so Co has a coordination number of 6, which means it forms an octahedral complex. The en ligands are strong field ligands, so they will cause a large crystal field splitting. This results in a low-spin octahedral complex.
16. List all the isomers of Cr(en)2I2+ and VO(H2O)2I2. You can ignore the structure that internal to ligands.
They will both have cis and trans isomers
Sean Gottlieb

