3.3.2: Environment- TSP, Ecological Stoichiometry, and Algal Blooms
- Page ID
- 50725
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Algal Blooms
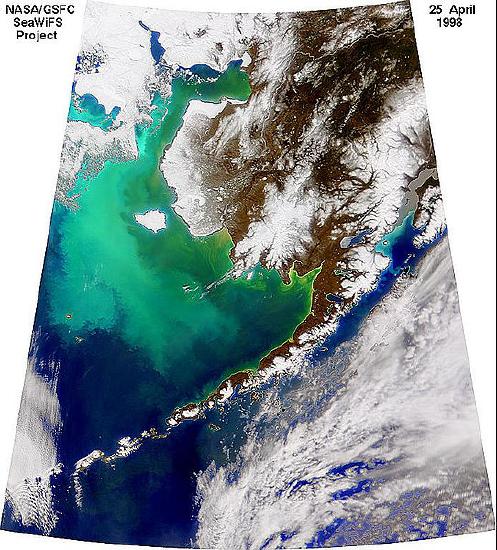
Algal blooms like the ones in the photos below may be harmful when the algae are toxic, or if they reduce the oxygen concentration enough to emperil other organisms. [1]. Blooms are visible because algae concentrations may reach millions of cells per milliliter. Blooms often result when one limiting reagent is supplied to the environment, either naturally, or through Human activities. A limiting reagent is one of several reactants that is necessary for a reaction to occur, but which is present in low concentration, so no reaction occurs even though there is an excess of all other reactants.
|
Coccolithophore algal bloom in the Bering Sea in 1998[2] |
A "red tide" which may poison seafood and cause human illness or death, caused by a dinoflagellate species.[3] |
The limiting reagent that prevents uncontrolled algae growth is often phosphorus, and it may be in low concentrations because phosphate mineral sources ("phosphate rock", like apatite) are insoluble.
Solubilizing Phosphate Rock: H3PO4
Phosphate often limits growth of foodcrops, so producing soluble phosphate is a significant sector of the fertilizer industry. Runoff from agricultural fields is often the cause of algal blooms.
Phosphate rock is solubilized for fertilizer by treatment with sulfuric acid, giving phosphoric acid and, as a byproduct, gypsum (CaSO4· 2 H2O used in Plaster of Paris and "drywall").
The reaction is:
- Ca5(PO4)3Cl + 5 H2SO4 + 10 H2O → 3 H3PO4 + 5 CaSO4 · 2 H2O + HCl

Phosphoric Acid[4]
Limiting Reagent Example 1
EXAMPLE 1 When 100.0 g of chloroapetite rock is reacted with 100.0 g of sulfuric acid to form phosphoric acid and gypsum, which is the limiting reagent? --- Solution The balanced equation
- Ca5(PO4)3Cl + 5 H2SO4 + 2 H2O → 3 H3PO4 + 5 CaSO4 · 2 H2O + HCl
tells us that according to the atomic theory, 1 mol Ca5(PO4)3Cl is required for every 5 moles of H2SO4. That is, the stoichiometric ratio S(Ca5(PO4)3Cl /H2SO4) = 1 mol Ca5(PO4)3Cl/ 5 mol H2SO4. Let us see how many moles of each we actually have
\(\begin{align} & n_{\text{Ca}_{\text{5}}\text{(PO}_{\text{4}}\text{)}_{\text{3}}\text{Cl}}=\text{100}\text{.0 g}\times \frac{\text{1 mol}}{\text{520}\text{.8 g}}=\text{0}\text{.192 mol Ca}_{\text{5}}\text{(PO}_{\text{4}}\text{)}_{\text{3}}\text{Cl} \\ & n_{\text{H}_{\text{2}}\text{SO}_{\text{4}}}=\text{100}\text{.0 g}\times \frac{\text{1 mol}_{\text{2}}}{\text{98}\text{.1 g}}=\text{1}\text{.02 mol H}_{\text{2}}\text{SO}_{\text{4}} \\ \end{align}\) If all the H2SO4 were to react, it would require 1.02 mol H2SO4 x (1 mol Ca5(PO4)3Cl / 5 mol H2SO4) = 0.204 mol Ca5(PO4)3Cl, but only 0.192 mol is present. So Ca5(PO4)3Cl is the limiting reagent, an all of the H2SO4 cannot react.
When the reaction ends, 0.960 mol H2SO4 will have reacted with 0.192 mol Ca5(PO4)3Cl and there will be (1.02 – 0.960) mol H2SO4 = 0.06 mol H2SO4 left over. Ca5(PO4)3Cl is therefore the limiting reagent.
These calculations can be organized as a table, with entries below the respective reactants and products in the chemical equation. Calculations are shown for each possible case, assuming that one reactant is completely consumed and determining if enough of the other reactants is present to consume it. If not, that scenario is discarded.
| Ca5(PO4)3Cl | + 5 H2SO4 | + 10 H2O → | 3 H3PO4 | + 5 CaSO4 · 2 H2O | + HCl | |
|---|---|---|---|---|---|---|
| m (g) | 100 | 100 | ||||
| M (g/mol) | 521 | 98.1 | 18.0 | 98.0 | 172.2 | 36.5 |
| n (mol) | 0.192 | 1.02 | -- | -- | -- | -- |
| if all Ca5(PO4)3Cl reacts | -0.192 | -0.960 | -1.92 | +0.576 | +0.960 | +0.192 |
| Actual Reaction Amounts |
0.192 | 0.960 | 1.92 | 0.576 | 0.960 | 0.192 |
| Actual Reaction Masses |
100 | 94.2 | 34.2 | 56.5 | 165.2 | 7.0 |
From this example you can begin to see what needs to be done to determine which of two reagents, X or Y, is limiting. We must compare the stoichiometric ratio S(X/Y) with the actual ratio of amounts of X and Y which were initially mixed together. In Example 1 this ratio of initial amounts \(\frac{n_{\text{Ca}_{\text{5}}\text{(PO}_{\text{4}}\text{)}_{\text{3}}\text{Cl}\text{(initial)}}}{n_{\text{H}_{\text{2}}\text{SO}_{\text{4}}}\text{(initial)}}=\frac{\text{0.192 mol Ca}_{\text{5}}\text{(PO}_{\text{4}}\text{)}_{\text{3}}\text{Cl}}{\text{1.02 mol H}_{\text{2}}\text{SO}_{\text{4}}}= \frac{\text{0.188 mol Ca}_{\text{5}}\text{(PO}_{\text{4}}\text{)}_{\text{3}}\text{Cl}}{\text{mol H}_{\text{2}}\text{SO}_{\text{4}} }\) was less than the stoichiometric ratio \(\text{S}\left( \frac{\text{Ca}_{\text{5}}\text{(PO}_{\text{4}}\text{)}_{\text{3}}\text{Cl}}{\text{H}_{\text{2}}\text{SO}_{\text{4}}} \right)=\frac{\text{1 mol Ca}_{\text{5}}\text{(PO}_{\text{4}}\text{)}_{\text{3}}\text{Cl}}{\text{5 mol H}_{\text{2}}\text{SO}_{\text{4}}}= \frac{\text{0.200 mol Ca}_{\text{5}}\text{(PO}_{\text{4}}\text{)}_{\text{3}}\text{Cl}}{\text{mol H}_{\text{2}}\text{SO}_{\text{4}} }\) This indicated that there was not enough Ca5(PO4)3Cl to react with all the H2SO4 and Ca5(PO4)3Cl was the limiting reagent. The corresponding general rule, for any reagents X and Y, is \(\begin{align} & \text{If}\frac{n_{\text{X}}\text{(initial)}}{n_{\text{Y}}\text{(initial)}}\text{is less than S}\left( \frac{\text{X}}{\text{Y}} \right)\text{, then X is limiting}\text{.} \\ & \\ & \text{If}\frac{n_{\text{X}}\text{(initial)}}{n_{\text{Y}}\text{(initial)}}\text{is greater than S}\left( \frac{\text{X}}{\text{Y}} \right)\text{, then Y is limiting}\text{.} \\ \end{align}\) (Of course, when the amounts of X and Y are in exactly the stoichiometric ratio, both reagents will be completely consumed at the same time, and neither is in excess.). This general rule for determining the limiting reagent is applied in the next example.
TriSodium phosphate, TSP
The phosphoric acid may be applied as a fertilizer solution, or converted to trisodium phosphate (TSP), a solid:
- H3PO4 + 3 NaOH → 3 Na3PO4 + 3 H2O

TSP is used as a cleaning and degreasing agent, and was used extensively in household detergents in the US until its deleterious effects on the environment were appreciated in the 1970s.[6]
Limiting Reagents and Ecological Stoichiometry
Since release of TSP and phosphoric acid may not be controlled in all countries, and in all activities, they often provide a source for phosphorus which is a limiting reagent in nature because of the low solubility of mineral phosphates.
As we explained when we discussed the significance of formulas, ecological stoichiometry examines the stoichiometric relationship between the nutritional demands of a species and the food available to the species.[7] [8]. Proponents say that "Ecological stoichiometry recognizes that organisms themselves are outcomes of chemical reactions and thus their growth and reproduction can be constrained by supplies of key chemical elements [especially carbon (C), nitrogen (N) and phosphorus (P)]".[9]
For example, by writing an approximate chemical equation for photosynthesis in oceanic algae, we can predict which nutrients (nitrogen as potassium nitrate, KNO3-, phosphorus as phosphoric acid, H3PO42-, etc.) are required for algae growth, and what products result from algal respiration.
106 CO2(g) + 16 KNO3(aq) + H3PO4(aq) + 122 H2O(l) + 16 H+(aq) ↔
C106H263O110N16P1(s) + 138 O2(g) + 16 K+ (1)
The "formula" for algae, (C106H263O110N16P1)(M = 3553.259 g/mol) does not represent a single molecule, but just the overall composition of the algae (one might call it an "average" molecular formula)[10].
Example 4 from Equations and Mass Relationships also illustrates the idea that one reactant in a chemical equation may be completely consumed without using up all of another. In the environment, inexpensive reagents like atmospheric O2 are often supplied in excess. Some portion of such a reagent will be left unchanged after the reaction. Conversely, at least one reagent is usually completely consumed. When it is gone, the other excess reactants have nothing to react with and they cannot be converted to products. The substance which is used up first is the limiting reagent.
Limiting Reagent Example 2
EXAMPLE 2 In a small scale experiment to model fertilizer runoff, water containing 10 g of H3PO4 contaminates a small pond which already contains 300 g of KNO3. If the pond contains stable algae which forms according to the equation above, and plenty of CO2 and other reactants are available, (a) what is the limiting reagent, and (b) how much algae can form as a result of the runoff?
Solution
a) The stoichiometric ratio connecting KNO3 and H3PO4 is
\(\text{S}\left( \frac{\text{KNO}_{\text{3}}}{\text{H}_{\text{3}}\text{PO}_{\text{4}}} \right)=\frac{\text{16 mol KNO}_{\text{3}}}{\text{1 mol H}_{\text{3}}\text{PO}_{\text{4}}} \) The initial amounts of KNO3 and H3PO4 are calculated using appropriate molar masses \(\begin{align} & \text{ }n_{\text{KNO}_{\text{3}}}\text{(initial)}=\text{300 g}\times \frac{\text{1 mol KNO}_{\text{3}}}{\text{101}\text{.1 g}}=\text{2.96}\text{mol KNO}_{\text{3}} \\ & \\ & n_{\text{H}_{\text{3}}\text{PO}_{\text{4}}}\text{(initial)}=\text{10}\text{.0 g}\times \frac{\text{1 mol H}_{\text{3}}\text{PO}_{\text{4}}}{\text{98.0 g}}=\text{0}\text{.102}\text{mol H}_{\text{3}}\text{PO}_{\text{4}} \\ \end{align}\) Their ratio is \(\frac{n_{\text{KNO}_{\text{3}}}\text{(initial)}}{n_{\text{H}_{\text{3}}\text{PO}_{\text{4}}}\text{(initial)}}=\frac{\text{2}\text{.96}\text{mol KNO}_{\text{3}}}{\text{0}\text{.102}\text{mol H}_{\text{3}}\text{PO}_{\text{4}}}=\frac{\text{29}\text{.0 mol KNO}_{\text{3}}}{\text{1 mol H}_{\text{3}}\text{PO}_{\text{4}}}\) Since this ratio is larger than the stoichiometric ratio, you have more than enough KNO3 to react with all the H3PO4. H3PO4 is the limiting reagent, and you will want to order more of it first since it will be consumed first. b) The amount of product formed in a reaction may be calculated via an appropriate stoichiometric ratio from the amount of a reactant which was consumed. Some of the excess reactant KNO3 will be left over, but all the initial amount of H3PO4 will be consumed. Therefore we use nH3PO4 (initial) to calculate how much algae can be obtained \(n_{\text{H}_{\text{3}}\text{PO}_{\text{4}}}\text{ }\xrightarrow{S\text{(Algae/H}_{\text{3}}\text{PO}_{\text{4}}\text{)}}\text{ }n_{\text{Algae}}\xrightarrow{M_{\text{Algae}}}\text{ }m_{\text{Algae}}\) \(m_{\text{Algae}}=\text{0.102}\text{ mol H}_{\text{3}}\text{PO}_{\text{4}}\text{ }\times \text{ }\frac{\text{1 mol Algae}}{\text{1 mol H}_{\text{3}}\text{PO}_{\text{4}}}\text{ }\times \text{ }\frac{\text{3553 g}}{\text{mol Algae}}=\text{362 g Algae}\) Note that only 10 g of H3PO4 allowed the consumption of 456 g of carbon dioxide to make 362 g of Algae!
These calculations can be organized as a table, with entries below the respective reactants and products in the chemical equation. The H+ and K+ have been omitted.
| 106 CO2 | + 16 KNO3 | + H3PO4 | + 122 H2O ↔ | C106H263O110N16P1 | + 138 O2 | |
|---|---|---|---|---|---|---|
| m (g) | 300 | 10 | ||||
| M (g/mol) | 44 | 101.1 | 98.0 | 18.0 | 3553.259 | 32 |
| n (mol) present | 2.96 | 0.102 | -- | -- | -- | |
| if all H3PO4 reacts | -1.63 | -0.102 | ||||
| Actual Reaction Amounts |
-10.81 | -1.63 | -0.102 | -12.44 | +0.102 | +14.1 |
| Actual Reaction Masses |
-476 | -165 | -10 | -224 | 362 |
450 |
As you can see from the example, in a case where there is a limiting reagent, the initial amount of the limiting reagent must be used to calculate the amount of product formed. Using the initial amount of a reagent present in excess would be incorrect, because such a reagent is not entirely consumed.
The concept of a limiting reagent was used by the nineteenth century German chemist Justus von Liebig (1807 to 1873) to derive an important biological and ecological law. Liebig’s law of the minimum states that the essential substance available in the smallest amount relative to some critical minimum will control growth and reproduction of any species of plant or animal life. When a group of organisms runs out of that essential limiting reagent, the chemical reactions needed for growth and reproduction must stop. Vitamins, protein, and other nutrients are essential for growth of the human body and of human populations. Similarly, the growth of algae in natural bodies of water such as Lake Erie can be inhibited by reducing the supply of nutrients such as phosphorus in the form of phosphates. It is for this reason that many states have regulated or banned the use of phosphates in detergents and are constructing treatment plants which can remove phosphates from municipal sewage before they enter lakes or streams.
References
- en.Wikipedia.org/wiki/Algal_bloom
- en.Wikipedia.org/wiki/Algal_bloom
- en.Wikipedia.org/wiki/Algal_bloom
- en.Wikipedia.org/wiki/Phosphoric_acid
- en.Wikipedia.org/wiki/Trisodium_phosphate
- en.Wikipedia.org/wiki/Trisodium_phosphate
- Ecological Stoichiometry: The biology of elements from Molecules to Biosphere; Robert W. Sterner and James J. Elser; Princeton University Press, Princeton, NJ, 2002.
- en.Wikipedia.org/wiki/Ecological_stoichiometry
- http://www.plosbiology.org/article/i...l.pbio.0050181
- Ecological Stoichiometry: The biology of elements from Molecules to Biosphere; Robert W. Sterner and James J. Elser; Princeton University Press, Princeton, NJ, 2002, p. 30.