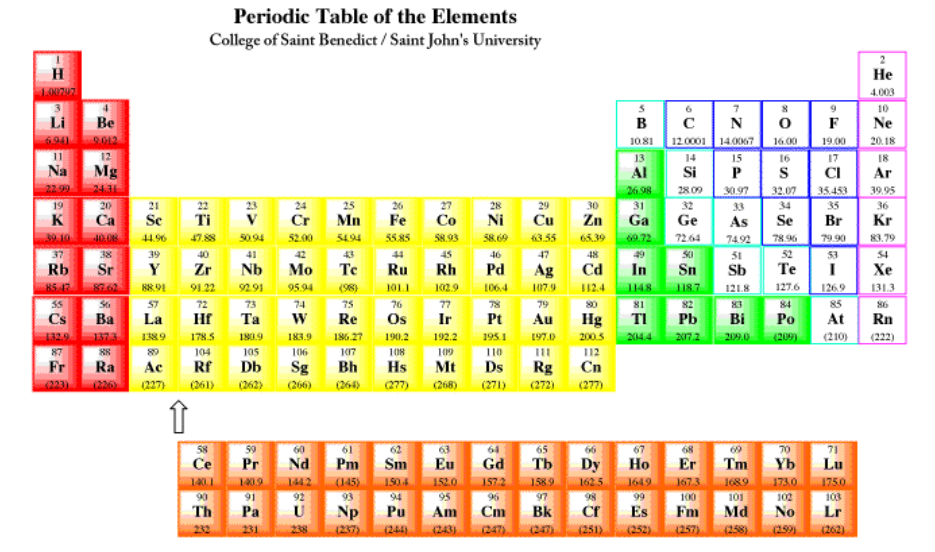
2.1: Introduction to Metals
- Page ID
- 189612
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Most of the elements found on earth are metals. A look at the periodic table shows that these elements occupy the entire left-hand stretch of the table, from the main group, through the transition metals, lanthanides, actinides, alkali and alkaline earth elements.
One element included here, hydrogen, is rarely classified as a metal. On earth, hydrogen is a gas, and it is usually classified as a non-metal, like oxygen and nitrogen. However, at very low temperatures and very high pressures, hydrogen is a solid, and under the right conditions it is expected to behave more like a metal. It is thought that gas giants, such as Jupiter and Saturn in our solar system, may have metallic hydrogen cores.
Metallic elements are not generally found as single atoms. Instead, the atoms in an element such as iron cluster together to make a larger structure. The materials formed in this way have some similar properties. Metals are shiny. Metals are malleable; they can be bent and formed into different shapes (at least when heated). Metals are good conductors of electricity.
- In metals, large groups of atoms cluster together.
- Metal atoms form large, extended arrays, with the atoms repeating in specific patterns throughout the solid.
The properties of metals are really important. The fact that metals are malleable allows them to be formed into sheets that can be used to make cars, airplanes, railway lines, cargo containers and ships, as well as more delicate items such as jewelry and surgical tools. A related property, ductility, allows metals to be stretched into long, thin wires. Together with the conductivity of metals, this property allows transmission lines to carry electricity from generating stations to people like you. Sometimes, the source of electricity is hundreds of miles away; electricity used to power a laptop in New York may come from places like La Grande Baleine or James Bay, in northwestern Quebec.
Some of these properties can be understood by thinking about the structure of metallic elements. A great deal of our structural understanding of metals and other materials comes from x-ray diffraction studies. A very focused beam of x-rays can be sent into a material, where they will bounce off the atoms and scatter in different directions. The outcome sounds chaotic, but if the solid is highly organized, the x-rays behave in very predictable ways. The result is an x-ray diffraction pattern. A diffraction pattern is a little like the pattern of ripples on a pond when a stone is thrown into calm water. The pattern can be studied and decoded mathematically to find the locations of the atoms within the material.
- X-ray diffraction can reveal the atomic-level structures of highly ordered materials such as metals.
What does x-ray diffraction tell us? Evidently, a chunk of metal is not just a mass of atoms stuck together randomly. Instead, the atoms arrange themselves in neat layers in very specific ways. These layers of atoms sit on top of each other to form a three-dimensional solid.
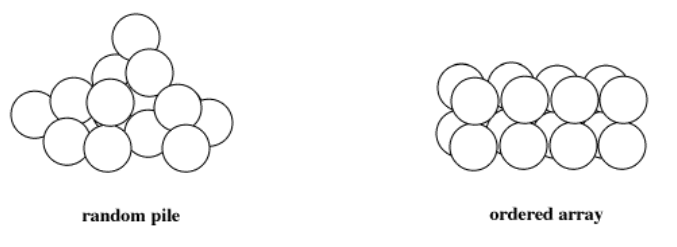
One of the properties that results from this organized arrangement of atoms is the malleability of metals. If you take a nice, soft metal such as copper, after annealing it in a fire or oven, it can be bent and shaped easily. With copper, this can be done even after the metal has cooled to room temperature. When you bend the copper, you are actually causing layers of atoms to slide over each other, until you stop bending and they come to rest in a new location.
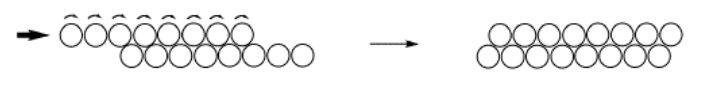
If you have ever done this, you'll know that the more you work with the copper, the harder it is to bend. That's because while you are sliding layers of atoms back and forth, occasionally an atom (or an entire row of atoms) slips out of place. It is no longer part of a smooth layer, and so other atoms can't slide past it as easily. This situation is called a defect. Once there are enough defects in the metal, it is impossible to bend the material anymore.

- Metal atoms are found in organized layers.
- Because these layers can roll over each other, metals can be worked into different shapes.
An alloy is a mixture of two metals. Steel is an alloy of iron with any of a number of other elements, such as chromium or vanadium. Alloys are often harder than metals composed of a pure element. Show how alloying introduces a defect into the metal, and how that makes the metal stronger.
- Answer
-
The second metal atom is a different size than the principle metal atom. It will not quite fit into the array of atoms. Consequently, the atoms will not be able to slide past each other as easily.
What is it that holds these metal atoms together? To answer that question, it's important to realize where metallic materials are found in the periodic table. The bottom left part of the periodic table is where the least electronegative elements are found. In fact, all of these elements lose electrons easily, and they are frequently found as cations in naturally-occurring compounds. For example, hematite is a common iron ore, containing iron cations (Fe3+) and oxygen anions (O2-). The formula of this compound is Fe2O3, meaning there is always a ratio of three oxygen anions for every two iron cations in hematite.
Show how the ratio of elements in hematite leads to a charge-balanced (overall neutral) compound.
- Answer
-
2 Fe: (3+) x 2 = (6+)
3 O: (2-) x 3 = (6-)
Fe2O3: neutral
Many of the atoms in a metallic material are present as cations. But where did their lost electrons go? Well, those electrons are still in the material, moving between the iron atoms and cations. In a piece of iron, the attraction between the iron cations and the freely moving electrons helps hold the metal together. This way of thinking about metals is sometimes called the "electron sea" model of bonding.
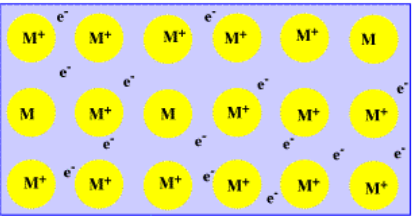
- Bonding in metals is often described through the "electron sea model".
- Metal ions are surrounded by delocalized electrons.
- Delocalized electrons are not restricted to one atom or another; they are distributed across several atoms in the solid.
Why do metals conduct electricity? Electricity is the movement of electrons through a material. But the conduction of current through a metal probably takes place through a series of events. If an electron is introduced at one end of the material, it will probably be attracted by a metal cation. It may even be captured by that metal. Sometimes, we describe this electron as moving into a "hole"; a hole, in conductivity terms, is just a positive charge that captures an electron. But remember that metals are still pretty electropositive (the opposite of electronegative), and that metal atom is likely to lose another electron. This may not be the same electron as the one you put in; it is probably another one. That electron may in turn be captured by another hole on another metal. That metal may lose another electron, and so on. Electrons will hop and skip from one metal to another throughout the material. An electric current results because these hopping electrons in the metal move away from the electrons that are being supplied at one end. They move towards the other end, instead.
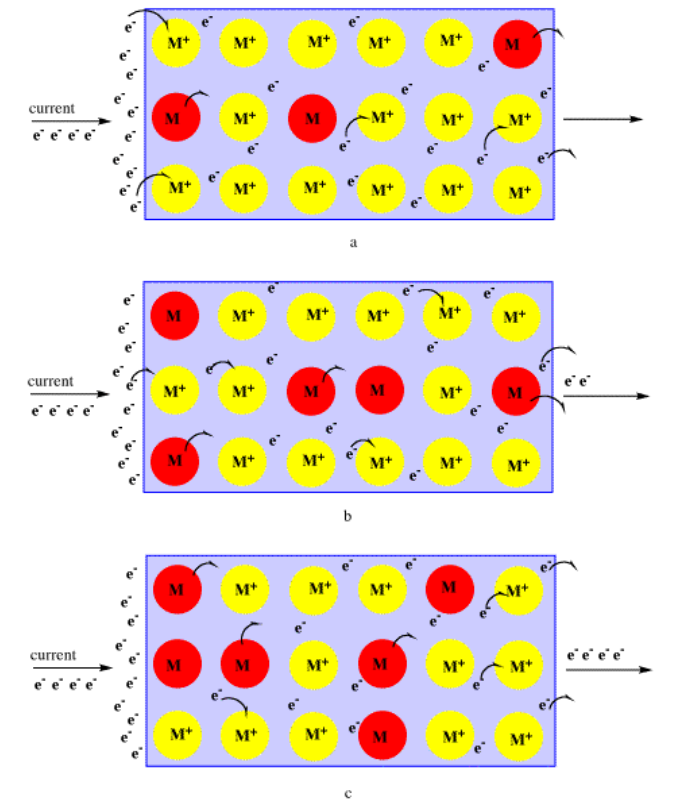
The shininess of metals is also attributed to the electron-sea aspect of metallic bonding. Collisions between incoming photons and the "free" electrons at the surface of a metal cause the photons to bounce off the surface. The reddish color of copper results from a limit on the wavelengths of visible light that bounce off the metal.
- The electron sea model is used to explain several unique properties of metals.
So far, we have looked at the electron sea model purely in terms of electrostatics: the negatively charged electron is attracted to the positively charged nucleus. However, we already saw in the discussion of quantum mechanics in the atom that kinetic energy and the wavelength of the electron is also an important factor in chemistry.

Suppose the following boxes are half-filled with water. Show the longest wavelength possible in each of the boxes.
- Answer
-
The longer the box, the longer the possible wavelength.
In the electron sea model of metallic bonding, the electrons can be delocalized. They are no longer confined to a single atom, but can be spread out over multiple atoms. As a result, the wavelength of the electron increases. Because wavelength is inversely proportional to energy, as an electron's wavelength increases, its energy goes down. As a result, spreading electrons out over a group of metals results in a decrease in energy because of the wave properties of electrons.
- Delocalization is an important concept in chemistry.
- Delocalization is related to the "particle-in-a-box" concept: the longer the container, the longer the possible wavelength.
- By spreading out over a larger group of atoms, an electron can adopt a longer wavelength.
- An electron with a longer wavelength has a lower energy.
Some of the properties of metals can be understood, given a basic outline of the structure of a metal. We are going to look in more detail at exactly how metal atoms arrange themselves into solids, before we look at some of the ways metals form compounds with other elements.


