Water Ionization

 

Water is an amphiprotic molecule, it can act as a very weak acid and a very weak base, donating protons to itself to a limited extent:

\[\text{2H}_{2}\text{O}\text{ }({l}) \rightleftharpoons \text{H}_{3}\text{O}^{+} ({aq}) + \text{OH}^{-} ({aq})\]

Individual molecules do not remain ionized for a long time, the reaction is rapid towards both sides of the equilibrium. The animation above shows the Grotthuss mechanism in which the protons tunnel from one water molecule to the next via hydrogen bonding. Auto-ionization of water occurs in a similar way with separation of ions H⁺ and OH^–, which recombine in a matter of femtoseconds. The lifetime of the separated ions is about 70 microseconds and depends on the extent of hydrogen bonding, so it is shorter at lower temperatures.^[1]

Applying the equilibrium law to the reaction above, we obtain

\[K_{c}=\dfrac{[\text{ H}_{3}\text{O}^{+}][\text{OH}^{-}]}{[\text{ H}_{2}\text{O }]^{2}}\]

However, the concentration of water has a constant value of 55.5 mol dm^–3 (the law of chemical equilibrium), and so its square can be multiplied by K_c to give a new constant K_w, called the ion-product constant of water:

\[K_{w}={K_{c}}\times{({55.5}\text{ mol dm}^{-3})^{2}}={[\text{ H}_{3}\text{O}^{+}] [\text{OH}^{-}]}\label{3}\]

Measurements of the electrical conductivity of carefully purified water indicate that at 25°C [H₃O⁺] = [OH^–] = 1.00 × 10^–7 mol dm^–3, so that

\[\begin{align}K_{w}={1.00}\times{10}^{-7}\text{ mol dm}^{-3}\times{1.00}\times{10}^{-7}\text{ mol dm}^{-3} \nonumber \\\text{ }\\\text{ }={1.00}\times{10}^{-14}\text{ mol}^2\text{dm}^{-6} \nonumber \end{align}\]

(Since the equilibrium law is not obeyed exactly, even in dilute solutions, results of most equilibrium calculations are rounded to three significant figures. Hence the value of K_w = 1.00 × 10^–14 mol² dm^–6 is sufficiently accurate for all such calculations.)

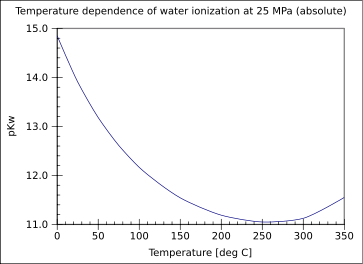
K_w depends on the strength of hydrogen bonding. Therefore, it is affected by the same factors that influence hydrogen bonding: Temperature, pressure, solute concentration and ionic strength. K_w doubles at about 100 MPa.^[1] At –35 ^oC, in undercooled water, K_w is approximately 1 x 10^–17. At high temperatures and pressures, although the K_w presents a more complex behavior, water becomes increasingly more ionized. At higher temperatures (~room temperature), one water molecule in 5.5 x 10⁸ is ionized, compared to one in 840 molecules at 1000 ^oC and 10 MPa.^[2] The following table shows the values of K_w and neutral pH in function of temperature. Taken from Harned and Owen, 1958.^[3] the figure that follows shows the dependence of pK_w on a wider range of temperature.

Table \(\PageIndex{1}\) Temperature dependence of K_w

Temperature ^oC	Kw	pKw	pH of a neutral solution [H^+]=[OH^–]
0	0.12 x 10^–14	14.93	7.47
15	0.45 x 10^–14	14.35	7.18
20	0.68 x 10^–14	14.17	7.08
25	1.01 x 10^–14	14.00	7.00
30	1.47 x 10^–14	13.83	6.92
40	2.95 x 10^–14	13.53	6.76

Ionic strength affects ionization of water, K_w reaches a maximum of 2 x 10^–14 mol² l^–2 at about 0.25 M ionic strength (trimethylammonium chloride) and decreases to 1 x 10^–14 mol² l^–2 when the ionic strength is raised to 5 M.^[1] K_w tends to increase in the presence of organic compounds.^[2]

Even though the degree of ionization is small (2.8 x 10^–9 at 37^oC), the dissociation of water has important implications in all biological systems including foods. Besides acting as lubricant, cleaning fluid, transporting media (nutrients and waste products) in living systems. The auto-ionization ability of water makes it a highly effective medium for proton exchange and participate essentially in four types of reactions: oxidation, reduction, hydrolysis, and condensation.^[2] The following sections in this chapter will show the importance of ionization of water as the major component and solvent in food systems.

The equilibrium constant K_w applies not only to pure water but to any aqueous solution at 25°C. Thus, for example, if we add 1.00 mol of the strong acid HNO₃, used in methods to determine ash content in foods, to H₂O to make a total volume of 1 dm³, essentially all the HNO₃ molecules donate their protons to H₂O:

\[\text{HNO}_{3} + \text{H}_{2}\text{O} \rightarrow \text{NO}_{3}^{-} + \text{H}_{3}\text{O}^{+}\]

and a solution in which [H₃O⁺] = 1.00 mol dm^–3 is obtained. Although this solution is very acidic, there are still hydroxide ions present. We can calculate their concentration by rearranging Eq. \(\ref{3}\) :

\[\begin{align}\text{ }[\text{OH}^{-}]=\dfrac{K_{w}}{[\text{ H}_{3}\text{O}^{+}]}=\dfrac{\text{1.00 }\times \text{ 10}^{-14}\text{ mol}^{2}\text{ dm}^{-6}}{\text{1.00 mol dm}^{-3}}\\\text{ }\\\text{ }=\text{1.00 }\times \text{ 10}^{-14}\text{ mol dm}^{-3}\end{align}\]

The addition of the HNO₃ to H₂O not only increases the hydronium-ion concentration but also reduces the hydroxide-ion concentration from an initially minute 10^–7 mol dm^–3 to an even more minute 10^–14 mol dm^–3.

Example \(\PageIndex{1}\) Ion Concentration

Calculate the hydronium-ion concentration in a solution of 0.516 M Mg(OH)₂.

Solution Since 1 mol Mg(OH)₂ produces 2 mol OH^– in solution, we have

\[[OH^-] = 2 \times 0.516 \dfrac{mol}{dm^3} = 1.032 \dfrac{mol}{dm^3}\]

Then

\[\begin{align}\text{ }[\text{ H}_{3}\text{O}^{+}]=\dfrac{K_{w}}{[\text{OH}^{-}]}=\dfrac{\text{1.00 }\times \text{ 10}^{-14}\text{ mol}^{2}\text{ dm}^{-6}}{\text{0.612 mol dm}^{-3}} \nonumber \\\text{ }\\\text{ }=\text{1.63 }\times \text{ 10}^{-14}\text{ mol dm}^{-3} \nonumber \end{align} \]

From ChemPRIME: 14.1: Ionization of Water

References

1. ↑ Chaplin, M. 2009. http://www1.lsbu.ac.uk/water/index2.html
2. ↑ Water: A matrix of life 2nd Ed. (Royal Society of Chemistry) 2000. F. Franks.
3. ↑ The Physical Chemistry of Electrolyte Solutions, 3rd Ed. 1958. Harned, H. S. and Owen, B. B.

Contributors and Attributions

• Ed Vitz (Kutztown University), John W. Moore (UW-Madison), Justin Shorb (Hope College), Xavier Prat-Resina (University of Minnesota Rochester), Tim Wendorff, and Adam Hahn.