There are many ways one can go about determining the structure of an unknown organic molecule. Although, nuclear magnetic resonance (NMR) and infrared radiation (IR) are the primary ways of determining molecular structures, calculating the degrees of unsaturation is useful information because it easily provides information about molecular structure.
Saturated and Unsaturated Molecules
Because alkanes have the maximum number of H atoms possible according to the rules of covalent bonds, alkanes are also referred to as saturated hydrocarbons. The presence of a double bond causes alkenes to have less hydrogens than an alkane with the same number of carbons. Likewise, compounds containing a carbon-to-carbon triple bonds (R–C≡C–R) called alkynes (Discussed in Chapter 9), also have fewer hydrogens than the corresponding alkane. Collectively, compounds which have fewer hydrogen atoms than an alkane with the same number of carbon atoms are called unsaturated hydrocarbons. The relationship between the number of carbons (n) and hydrogens in the molecular formula for alkanes, alkenes, and alkynes are listed below.
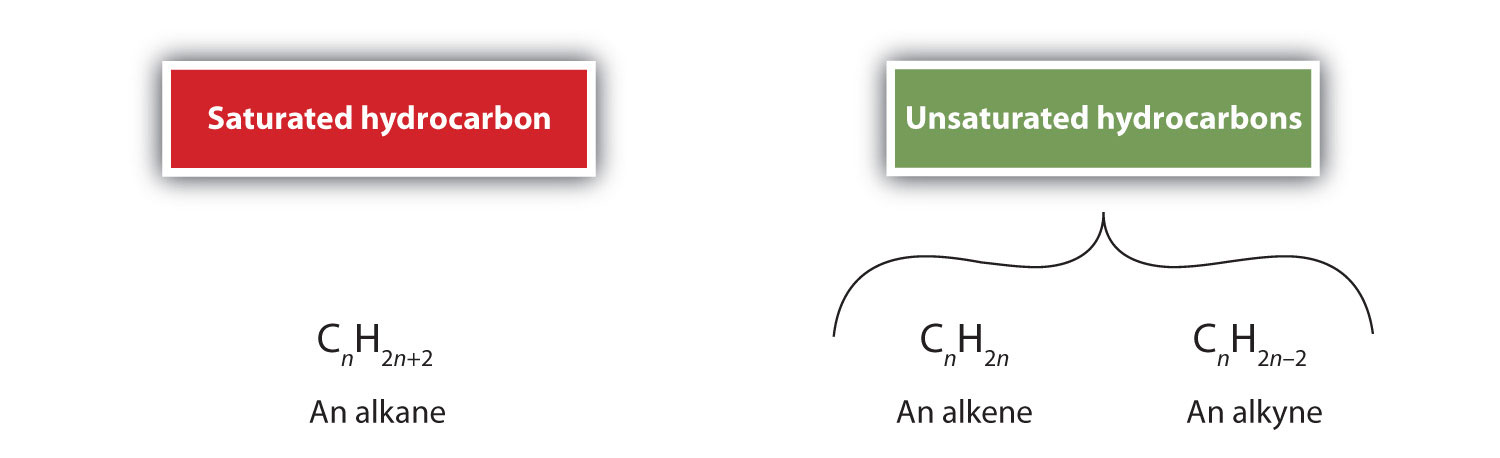
For example, the three carbon alkane, propane has the molecular formula of C3H8. While the unsaturated compounds propene (C3H6) and propyne (C3H4) both have fewer hydrogens. Also, it is important to note that cycloalkanes with one ring have a general molecular formula of CnH2n just like alkenes. Because they also have fewer than maximum number of hydrogens possible, cyclic compounds are also considered unsaturated.

Calculating the Degree of Unsaturation (DoU)
As noted above, every degree of unsaturation causes the loss of two hydrogens from a compound's molecular formula when compared to an alkane with the same number of carbons. Understanding this relationship allows for the degrees of unsaturation of a compound to be calculated from its molecular formula. First, the maximum number of hydrogens possible for a given compound (2C + 2) is calculated and then the actual number of hydrogens present in the compound (H) is subtracted. If this difference is then divided by 2 the answer will be equal to the degrees of unsaturation for the compound.
For a compound which only contains carbon and hydrogen:
DoU = (2C + 2) - H / 2
As an example, for the molecular formula C3H4 the number of actual hydrogens needed for the compound to be saturated is 8 [2C+2=(2x3)+2=8]. Because the compound only has 4 hydrogens in its molecular formula, it would have to gain 4 more hydrogens in order to be fully saturated (8-4 = 4). Degrees of unsaturation is equal to half the number of hydrogens the molecule needs to be fully saturated. This compound has 2 degrees of unsaturation (4/2 = 2).
The DoU of compounds containing elements other than carbon and hydrogen can also be calculated in a similar fashion. However, different elements can affect the formula used to calculate DoU.
For a compound which contains elements other than carbon and hydrogen:
\[ DoU= \dfrac{2C+2+N-X-H}{2} \tag{7.2.1} \]
- \(C\) is the number of carbons
- \(N\) is the number of nitrogens
- \(X\) is the number of halogens (F, Cl, Br, I)
- \(H\) is the number of hydrogens
A halogen (X) replaces a hydrogen in a compound because both form one single bond. Therefore the DoU formula subtracts the number of halogens (X) present in a compound. For instance, 1,1-dichloroethene (C2H2Cl2) has two fewer hydrogens than ethene (C2H4) yet they both have one degree of unsaturation.

Oxygen and sulfur are not included in the DoU formula because saturation is unaffected by these elements. The inclusion of an alcohol or sulfur in a compound does not change the number of hydrogens to obtain saturation. As seen in alcohols, the number of hydrogens in cyclohexanol (C6H12O) matches the number of hydrogens in cyclohexane (C6H12) and they both have one degree of unsaturation.

When a nitrogen is present in a compound one more hydrogen is required to reach saturation. Therefore, we add the number of nitrogens (N). Propyl amine (C3H9N) has one more hydrogen compared to propane (C3H8) both of which are saturated compounds with 0 DoU.

With the degrees of unsaturation comes information about the possible number of rings and multiple bonds in a given compound. Remember, the degrees of unsaturation only gives the sum of pi bonds and/or rings.
- One degree of unsaturation is equivalent to 1 ring or 1 double bond (1 \( \pi \) bond).
- Two degrees of unsaturation is equivalent to 2 double bonds, 1 ring and 1 double bond, 2 rings, or 1 triple bond (2 \( \pi \) bonds).
Example 7.3.1: Benzene
What is the Degree of Unsaturation for Benzene?
Solution
The molecular formula for benzene is C6H6. Thus,
DoU= 4, where C=6, N=0,X=0, and H=6. 1 DoB can equal 1 ring or 1 double bond. This corresponds to benzene containing 1 ring and 3 double bonds.

However, when given the molecular formula C6H6, benzene is only one of many possible structures (isomers). The following structures all have DoB of 4 and have the same molecular formula as benzene.
-hexa-1%252C3-dien-5-yne%252C_fulvene%252C_and_hexa-1%252C5-dien-3-yne.svg?revision=1)
Exercise \(\PageIndex{2}\)
Determine whether the following molecules are saturated or unsaturated.

- Answer
-
a) unsaturated (Even though the rings only contain single bonds, rings are considered unsaturated.)
b) unsaturated
c) saturated
d) unsaturated
e) unsaturated
f) saturated
Exercise \(\PageIndex{3}\)
Determine the degrees of unsaturation for each of the following compounds.

- Answer
-
If the molecular structure is given, the easiest way to solve is to count the number of double bonds, triple bonds and/or rings. However, you can also determine the molecular formula and solve for the degrees of unsaturation by using the formula.
a) 2 (2 rings)
b) 2 (one double bond and the double bond from the carbonyl)
c) 0 (no double bonds or rings)
d) 10 (2(10) + 2 + 4 - 0 - 6)/2 = 10
e) 1 (2(5) + 2 + 0 - 0 - 10)/2 = 1
f) 0 (2(6) + 2 + 0 - 2 - 12)/2 = 0
Exercise \(\PageIndex{4}\)
Calculate the degrees of unsaturation for the following molecular formulas:
a) C9H20 b) C7H8 c) C5H7Cl d) C9H9NO4
- Answer
-
Use the formula to solve (O not involved in the formula)
(a.) 0 (2(9) + 2 - 20)/2 = 0
(b.) 4 (2(7) + 2 - 8)/2 = 4
(c.) 2 (2(5) + 2 - 1 -7)/2 = 2
(d.) 6 (2(9) + 1 - 7)/2 = 6


