Types of Saturation
- Page ID
- 1616
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\dsum}{\displaystyle\sum\limits} \)
\( \newcommand{\dint}{\displaystyle\int\limits} \)
\( \newcommand{\dlim}{\displaystyle\lim\limits} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Introduction
When solid solute (substance or particles) and liquid solvent are mixed, the only possible reactions are dissolution and crystallization.
- Dissolution is the dissolving process of the solid solute.
- Crystallization is the opposite, causing the solid solute to remain undissolved.
Types of Saturation
| Kinds of Saturation | Definition |
|---|---|
| Saturated Solution | A solution with solute that dissolves until it is unable to dissolve anymore, leaving the undissolved substances at the bottom. |
| Unsaturated Solution | A solution (with less solute than the saturated solution) that completely dissolves, leaving no remaining substances. |
| Supersaturated Solution | A solution (with more solute than the saturated solution) that contains more undissolved solute than the saturated solution because of its tendency to crystallize and precipitate. |
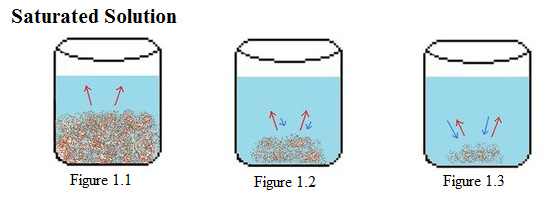
| Example 1: Saturated Solution |
|---|
|
Example 1: Above is illustrated an example of a saturated solution. In Figure 1.1-1.3, there is a constant amount of water in all the beakers. Figure 1.1 shows the start of the saturation process, in which the solid solute begins to dissolve (represented by red arrows). In the next beaker, Figure 1.2, much of the solid solute has dissolved, but not completely, because the process of crystallization (represented by blue arrows) has begun. In the last beaker, Figure 1.3, only a small amount of the solute solvent remains undissolved. In this process, the rate of the crystallization is faster than the rate of dissolution, causing the amount of dissolved to be less than the amount crystallized. |
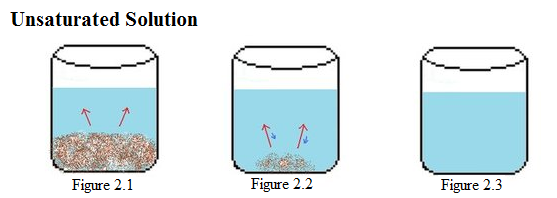
| Example 2: Unsaturated Solution |
|---|
|
Example 2: Next, an unsaturated solution is considered. In Figure 2.1-2.3, there is a constant amount of water in all the beakers. Figure 2.1 shows the start of the process, in which solid solute is beginning to dissolve (represented by red arrows). In the next beaker, shown in Figure 2.2, a large amount of solute has dissolved. The size of the red arrows are much larger than those of the blue arrows, which means that the rate of dissolution is much greater than rate of crystallization. In the last beaker, shown in Figure 2.3, the solute solvent has completely dissolved in the liquid solvent. |
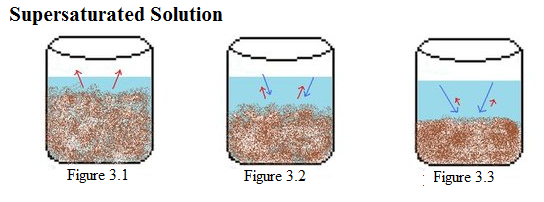
| Example 3: Supersaturated Solution |
|---|
|
Example 3: This is an example of a supersaturated solution. In Figure 3.1-3.3, there is a constant amount of water in all the beakers. Figure 3.1 shows a beaker with more solid solute than in the saturated solution (Figure 1.1) dissolving. In Figure 3.2, solid begins to crystallize as it slowly decreases the rate of dissolution. In the last picture, Figure 3.3, the solids become a crystallized form which begins to harden. |
Factors Affecting Saturation
- The solubilities of ionic solutions increase with an increase in temperature, with the exceptions of compounds containing anions.
- Finely divided solids have greater solubilities.
- In contrast to the solubility rate, which depends primarily on temperature, the rate of crystallization depends on the concentration of the solute at the crystal surface.
- In a still solution, concentration builds at the solute surface causing higher crystallization; therefore, stirring the solution prevents the build up, maximizing the net dissolving rate.
- The net dissolving rate is defined as the dissolving rate minus the crystallization rate.
- If the rates of solubility and crystallization are the same, the solution is saturated, and dynamic equilibrium is reached.
- Le Chatelier's principle predicts the responses when an equilibrium system is subjected to change in temperature, pressure or concentration. This principle states the following:
- For an increase of temperature, solubility increases which causes an endothermic reactions.
- For an decrease of temperature, solubility decreases which causes an exothermic reactions.
- Adding an inert gas to a constant-volume equilibrium mixture has no effect on the equilibrium.
- An increase in the external pressure causes a decrease in reaction volume and shifts equilibrium to the right.
Other Related Topics
Outside Links
References
- Petrucci, Harwood, Herring. General Chemistry: Principles & Modern Applications. 8th ed. Upper Saddle River, New Jersey: Pearson/Prentice Hall, 2002.
Contributors
- Mimi Lee, Victoria Chen