1.3: Work as a Mechanism to Transfer Energy
- Page ID
- 42252
- To define work
- To identify mechanical and pressure-volume work
One definition of energy is the capacity to do work. There are many kinds of work, including mechanical work, electrical work, and work against a gravitational or a magnetic field. Here we will consider only mechanical work and focus on the work done during changes in the pressure or the volume of a gas.
Mechanical Work
The easiest form of work to visualize is mechanical work (Figure \(\PageIndex{1}\)), which is the energy required to move an object a distance \(d\) when opposed by a force \(F\), such as gravity (Figure \(\PageIndex{1}\)):
\[w=F\,d \label{12.3.1}\]
with \(w\) is work, \(F\) is opposing force, and \(d\) is distance.
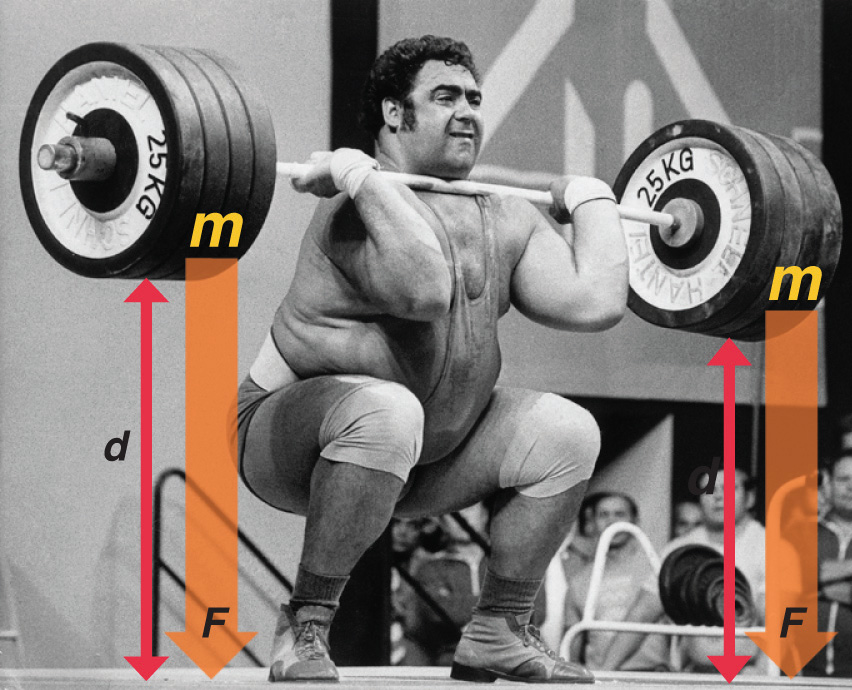
Because the force (\(F\)) that opposes the action is equal to the mass (\(m\)) of the object times its acceleration (\(a\)), Equation \(\ref{12.3.1}\) can be rewritten to:
\[w = m\,a\,d \label{12.3.2}\]
with \(w\) is work, \(m\) is mass, \(a\) is acceleration, and \(d\) is distance.
Recall from that weight is a force caused by the gravitational attraction between two masses, such as you and Earth. Hence for works against gravity (on Earth), \(a\) can be set to \(g=9.8\; m/s^2\). Consider the mechanical work required for you to travel from the first floor of a building to the second. Whether you take an elevator or an escalator, trudge upstairs, or leap up the stairs two at a time, energy is expended to overcome the opposing force of gravity. The amount of work done (w) and thus the energy required depends on three things:
- the height of the second floor (the distance \(d\));
- your mass, which must be raised that distance against the downward acceleration due to gravity; and
- your path.
Pressure-Volume (PV) Work
To describe this pressure–volume work (PV work), we will use such imaginary oddities as frictionless pistons, which involve no component of resistance, and ideal gases, which have no attractive or repulsive interactions. Imagine, for example, an ideal gas, confined by a frictionless piston, with internal pressure \(P_{int}\) and initial volume \(V_i\) (Figure \(\PageIndex{2}\)). If \(P_{ext} = P_{int}\), the system is at equilibrium; the piston does not move, and no work is done. If the external pressure on the piston (\(P_{ext}\)) is less than \(P_{int}\), however, then the ideal gas inside the piston will expand, forcing the piston to perform work on its surroundings; that is, the final volume (\(V_f\)) will be greater than \(V_i\). If \(P_{ext} > P_{int}\), then the gas will be compressed, and the surroundings will perform work on the system.
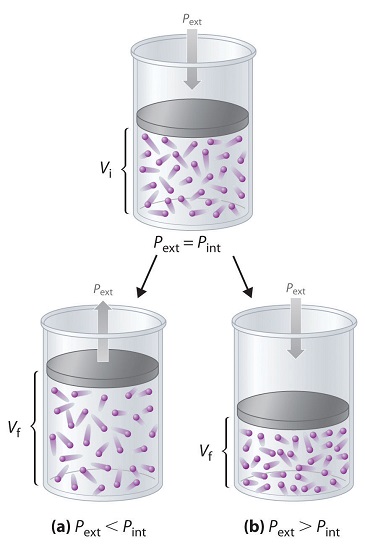
If the piston has cross-sectional area \(A\), the external pressure exerted by the piston is, by definition, the force per unit area:
\[P_{ext} = \dfrac{F}{A} \label{eq5}\]
The volume of any three-dimensional object with parallel sides (such as a cylinder) is the cross-sectional area times the height (\(V = Ah\)). Rearranging Equation \ref{eq5} to give
\[F = P_{ext}A\]
and defining the distance the piston moves (\(d\)) as \(Δh\), we can calculate the magnitude of the work performed by the piston by substituting into Equation \(\ref{12.3.1}\):
\[w = F d = P_{ext}AΔh \label{12.3.3}\]
The change in the volume of the cylinder (\(ΔV\)) as the piston moves a distance \(d\) is \(ΔV = AΔh\), as shown in Figure \(\PageIndex{3}\).
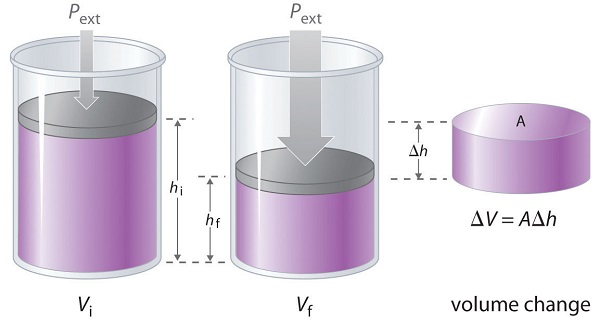
The PV work performed is thus
\[ w = P_{ext}ΔV \label{12.3.4}\]
The units of work obtained using this definition are correct for energy: pressure is force per unit area (newton/m2) and volume has units of cubic meters, so
\[w=\left(\dfrac{F}{A}\right)_{\textrm{ext}}(\Delta V)=\dfrac{\textrm{newton}}{\textrm m^2}\times \textrm m^3=\mathrm{newton\cdot m}=\textrm{joule}\]
If we use atmospheres for P and liters for V, we obtain units of L·atm for work. These units correspond to units of energy, as shown in the different values of the ideal gas constant R:
\[R=\dfrac{0.08206\;\mathrm{L\cdot atm}}{\mathrm{mol\cdot K}}=\dfrac{8.314\textrm{ J}}{\mathrm{mol\cdot K}}\]
Thus 0.08206 L·atm = 8.314 J and 1 L·atm = 101.3 J.
Whether work is defined as having a positive sign or a negative sign is a matter of convention. Heat flow is defined from a system to its surroundings as negative; using that same sign convention, we define work done by a system on its surroundings as having a negative sign because it results in a transfer of energy from a system to its surroundings. This is an arbitrary convention and one that is not universally used. Some engineering disciplines are more interested in the work done on the surroundings than in the work done by the system and therefore use the opposite convention. Because \(ΔV > 0\) for an expansion, Equation \(\ref{12.3.4}\) must be written with a negative sign to describe PV work done by the system as negative:
\[ w = −P_{ext}ΔV \label{12.3.5}\]
The work done by a gas expanding against an external pressure is therefore negative, corresponding to work done by a system on its surroundings. Conversely, when a gas is compressed by an external pressure, \(ΔV < 0\) and the work is positive because work is being done on a system by its surroundings.
For the thermochemistry
- Heat is positive when energy is transferred from the surroundings to the system
- Work is positive when energy is transferred from the surroundings to the system
A small high-performance internal combustion engine has six cylinders with a total nominal displacement (volume) of 2.40 L and a 10:1 compression ratio (meaning that the volume of each cylinder decreases by a factor of 10 when the piston compresses the air–gas mixture inside the cylinder prior to ignition). How much work in joules is done when a gas in one cylinder of the engine expands at constant temperature against an opposing pressure of 40.0 atm during the engine cycle? Assume that the gas is ideal, the piston is frictionless, and no energy is lost as heat.
Given: final volume, compression ratio, and external pressure
Asked for: work done
Strategy
Calculate the final volume of gas in a single cylinder. Then compute the initial volume of gas in a single cylinder from the compression ratio. Use Equation \(\ref{12.3.5}\) to calculate the work done in liter-atmospheres. Convert from liter-atmospheres to joules.
Solution
A To calculate the work done, we need to know the initial and final volumes. The final volume is the volume of one of the six cylinders with the piston all the way down: Vf = 2.40 L/6 = 0.400 L. With a 10:1 compression ratio, the volume of the same cylinder with the piston all the way up is Vi = 0.400 L/10 = 0.0400 L. Work is done by the system on its surroundings, so work is negative.
\[\begin{align} w &= −P_{ext}ΔV\nonumber \\[5pt] &= −(40.0\, atm)(0.400\, L − 0.0400 \,L)\nonumber \\[5pt] &= −14.4\, L·atm\nonumber \end{align}\nonumber\]
Converting from liter-atmospheres to joules,
\[\begin{align} w &=-(14.4\;\mathrm{L\cdot atm})[101.3\;\mathrm{J/(L\cdot atm)}]\nonumber \\[5pt] &= -1.46\times10^3\textrm{ J}\nonumber \end{align}\nonumber\]
In the following exercise, you will see that the concept of work is not confined to engines and pistons. It is found in other applications as well.
Breathing requires work, even if you are unaware of it. The lung volume of a 70 kg man at rest changed from 2200 mL to 2700 mL when he inhaled, while his lungs maintained a pressure of approximately 1.0 atm.
- How much work in liter-atmospheres and joules was required to take a single breath?
- During exercise, his lung volume changed from 2200 mL to 5200 mL on each in-breath.
- How much additional work in joules did he require to take a breath while exercising?
- Answer
-
−0.500 L·atm, or −50.7 J; −304 J; if he takes a breath every three seconds, this corresponds to 1.4 Calories per minute (1.4 kcal).
- How much work is done by a gas that expands from 2 liters to 5 liters against an external pressure of 750 mmHg?
- How much work is done by 0.54 moles of a gas that has an initial volume of 8 liters and expands under the following conditions: 30 oC and 1.3 atm?
- How much work is done by a gas (P=1.7 atm, V=1.56 L) that expands against an external pressure of 1.8 atm?
- Solution a
-
\[w = − PΔV\nonumber\]
\[ΔV = V_{final} - V_{Initial} = 5 \,L - 2\, L = 3 L\nonumber\]
Convert 750 mmHg to atm:
\[750 mmHg * 1/760 (atm/mmHg) = 0.9868 atm.\nonumber\]
\[W = − pΔV = -(.9868\, atm)(3\,L) = -2.96 \,L\, atm.\nonumber\]
- Solution b
-
First we must find the final volume using the ideal gas law:
\[pV = nRT\nonumber\]
or
\[\begin{align*} V &= \dfrac{nRT}{P} \\[5pt] &= \dfrac{ (0.54 \,moles)(0.082057 (L\, atm)/ (mol\, K))(303\,K)}{1.3\, atm} = 10.33\, L \end{align*}\]
\[\begin{align*} ΔV &= V_{final} - V_{initial} \\[5pt] &= 10.3\, L - 8\, L = 2.3\, L \end{align*}\]
Then from Equation \ref{12.3.4}
\[w = − pΔV = - (1.3\, atm)(2.3 \,L) = -3\, L\, \cdot atm.\nonumber\]
- Solution c
-
\[w = - p ΔV = - (1.8 \,atm )\, ΔV.\nonumber\]
Given \(p_1\), \(V_1\), and \(p_2\), find \(V_2\): \(p_1V_1=p_2V_2\) (at constant \(T\) and \(n\))
\[V_2= \dfrac{V_1 P_1}{ P_2} = (1.56 L * 1.7 atm) / 1.8 atm = 1.47 L\nonumber\]
Now,
\[ΔV = V_2 - V_1=1.47\, L - 1.56\, L = -0.09\nonumber\]
\[w = - (1.8 atm) * (-0.09 L) = 0.162 L atm.\nonumber\]
Work in Chemical Transformations
Suppose, for example, that the system under study is a mass of steam heated by the combustion of several hundred pounds of coal and enclosed within a cylinder housing a piston attached to the crankshaft of a large steam engine. The gas is not ideal, and the cylinder is not frictionless. Nonetheless, as steam enters the engine chamber and the expanding gas pushes against the piston, the piston moves, so useful work is performed. In fact, PV work launched the Industrial Revolution of the 19th century and powers the internal combustion engine on which most of us still rely for transportation.
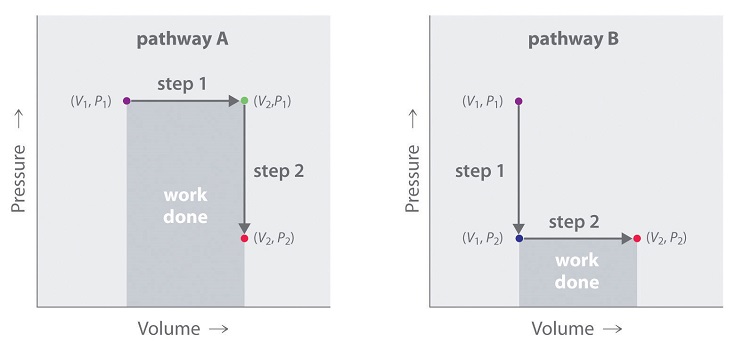
In contrast to internal energy, work is not a state function. We can see this by examining Figure \(\PageIndex{4}\), in which two different, two-step pathways take a gaseous system from an initial state to a final state with corresponding changes in temperature. In pathway A, the volume of a gas is initially increased while its pressure stays constant (step 1); then its pressure is decreased while the volume remains constant (step 2). In pathway B, the order of the steps is reversed. The temperatures, pressures, and volumes of the initial and final states are identical in both cases, but the amount of work done, indicated by the shaded areas in the figure, is substantially different. As we can see, the amount of work done depends on the pathway taken from (\(V_1\), \(P_1\)) to (\(V_2\), \(P_2\)), which means that work is not a state function.
Internal energy is a state function, whereas work is not.
Work and Chemical Reactions
Consider, for example, a reaction that produces a gas, such as dissolving a piece of copper in concentrated nitric acid. The chemical equation for this reaction is as follows:
\[\ce{Cu(s) + 4HNO3(aq) -> Cu(NO3)2(aq) + 2H2O(l) + 2NO2(g)} \label{12.3.5a}\]
If the reaction is carried out in a closed system that is maintained at constant pressure by a movable piston, the piston will rise as nitrogen dioxide gas is formed (Figure \(\PageIndex{5}\)). The system is performing work by lifting the piston against the downward force exerted by the atmosphere (i.e., atmospheric pressure). We find the amount of PV work done by multiplying the external pressure P by the change in volume caused by movement of the piston (\(ΔV\)). At a constant external pressure (here, atmospheric pressure)
\[w = −PΔV \label{12.3.6}\]
The negative sign associated with \(PV\) work done indicates that the system loses energy. If the volume increases at constant pressure (ΔV > 0), the work done by the system is negative, indicating that a system has lost energy by performing work on its surroundings. Conversely, if the volume decreases (ΔV < 0), the work done by the system is positive, which means that the surroundings have performed work on the system, thereby increasing its energy.

Enthalpy
The symbol \(U\) represents the internal energy of a system, which is the sum of the kinetic energy and potential energy of all its components. It is the change in internal energy that produces heat plus work. To measure the energy changes that occur in chemical reactions, chemists usually use a related thermodynamic quantity called enthalpy (H) (from the Greek enthalpein, meaning “to warm”). The enthalpy of a system is defined as the sum of its internal energy \(U\) plus the product of its pressure \(P\) and volume \(V\):
\[H =U + PV \label{12.3.7}\]
Because internal energy, pressure, and volume are all state functions, enthalpy is also a state function.
If a chemical change occurs at constant pressure (i.e., for a fixed \(P\), \(ΔP = 0\)), the change in enthalpy (\(ΔH\)) is
\[\begin{align} ΔH &= Δ(U + PV) \\[4pt] &= ΔU + Δ(PV) \\[4pt] &= ΔU + PΔV \label{12.3.8} \end{align}\]
Substituting \(q + w\) for \(ΔU\) (Equation \(\ref{12.3.8}\)) and \(−w\) for \(PΔV\) (Equation \(\ref{12.3.6}\)), we obtain
\[\begin{align} ΔH &= ΔU + PΔV \nonumber\\[4pt] &= q_p + \cancel{w} − \cancel{w} \nonumber\\[4pt] &= q_p \label{12.3.9} \end{align}\]
The subscript \(p\) is used here to emphasize that this equation is true only for a process that occurs at constant pressure. From Equation \(\ref{12.3.9}\) we see that at constant pressure the change in enthalpy, \(ΔH\) of the system, defined as \(H_{final} − H_{initial}\), is equal to the heat gained or lost.
\[ΔH = H_{final} − H_{initial} = q_p \label{12.3.10}\]
Just as with \(ΔU\), because enthalpy is a state function, the magnitude of \(ΔH\) depends on only the initial and final states of the system, not on the path taken. Most important, the enthalpy change is the same even if the process does not occur at constant pressure.
To find \(ΔH\) for a reaction, measure \(q_p\) under constant pressure.
Summary
All forms of energy can be interconverted. Three things can change the energy of an object: the transfer of heat, work performed on or by an object, or some combination of heat and work.
Outside Links
- Gasparro, Frances P. "Remembering the sign conventions for q and w in deltaU = q - w." J. Chem. Educ. 1976: 53, 389.
- Koubek, E. "PV work demonstration (TD)." J. Chem. Educ. 1980: 57, 374. '

