3.7: Bond Energies & Bond Enthalpies
- Page ID
- 20211
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Atoms bond together to form compounds because in doing so they attain lower energies than they possess as individual atoms. A quantity of energy, equal to the difference between the energies of the bonded atoms and the energies of the separated atoms, is released, usually as heat. That is, the bonded atoms have a lower energy than the individual atoms do. When atoms combine to make a compound, energy is always given off, and the compound has a lower overall energy.
When a chemical reaction occurs, molecular bonds are broken and other bonds are formed to make different molecules. For example, the bonds of two water molecules are broken to form hydrogen and oxygen.
\[ 2H_2O \rightarrow 2H_2 + O_2\]
Energy is always required to break a bond, which is known as bond energy. While the concept may seem simple, bond energy serves a very important purpose in describing the structure and characteristics of a molecule. It can be used to determine which Lewis Dot Structure is most suitable when there are multiple Lewis Dot Structures.
Energy is always required to break a bond. Energy is released when a bond is made.
Although each molecule has its own characteristic bond energy, some generalizations are possible. For example, although the exact value of a C–H bond energy depends on the particular molecule, all C–H bonds have a bond energy of roughly the same value because they are all C–H bonds. It takes roughly 100 kcal of energy to break 1 mol of C–H bonds, so we speak of the bond energy of a C–H bond as being about 100 kcal/mol. A C–C bond has an approximate bond energy of 80 kcal/mol, while a C=C has a bond energy of about 145 kcal/mol. We can calculate a more general bond energy by finding the average of the bond energies of a specific bond in different molecules to get the average bond energy.
| Single Bonds | Multiple Bonds | ||||||
|---|---|---|---|---|---|---|---|
| H—H |
432
|
N—H |
391
|
I—I |
149
|
C = C |
614
|
| H—F |
565
|
N—N |
160
|
I—Cl |
208
|
C ≡ C |
839
|
| H—Cl |
427
|
N—F |
272
|
I—Br |
175
|
O = O |
495
|
| H—Br |
363
|
N—Cl |
200
|
C = O* |
745
|
||
| H—I |
295
|
N—Br |
243
|
S—H |
347
|
C ≡ O |
1072
|
| N—O |
201
|
S—F |
327
|
N = O |
607
|
||
| C—H |
413
|
O—H |
467
|
S—Cl |
253
|
N = N |
418
|
| C—C |
347
|
O—O |
146
|
S—Br |
218
|
N ≡ N |
941
|
| C—N |
305
|
O—F |
190
|
S—S |
266
|
C ≡ N |
891
|
| C—O |
358
|
O—Cl |
203
|
C = N |
615
|
||
| C—F |
485
|
O—I |
234
|
Si—Si |
340
|
||
| C—Cl |
339
|
Si—H |
393
|
||||
| C—Br |
276
|
F—F |
154
|
Si—C |
360
|
||
| C—I |
240
|
F—Cl |
253
|
Si—O |
452
|
||
| C—S |
259
|
F—Br |
237
|
||||
| Cl—Cl |
239
|
||||||
| Cl—Br |
218
|
||||||
| Br—Br |
193
|
||||||
|
*C == O(CO2) = 799
|
|||||||
When a bond is strong, there is a higher bond energy because it takes more energy to break a strong bond. This correlates with bond order and bond length. When the Bond order is higher, bond length is shorter, and the shorter the bond length means a greater the Bond Energy because of increased electric attraction. In general, the shorter the bond length, the greater the bond energy.
The average bond energies in Table T3 are the averages of bond dissociation energies. For example the average bond energy of O-H in H2O is 464 kJ/mol. This is due to the fact that the H-OH bond requires 498.7 kJ/mol to dissociate, while the O-H bond needs 428 kJ/mol.
\[\dfrac{498.7\; kJ/mol + 428\; kJ/mol}{2}=464\; kJ/mol\]
When more bond energies of the bond in different molecules that are taken into consideration, the average will be more accurate. However,
- Average bonds values are not as accurate as a molecule specific bond-dissociation energies.
- Double bonds are higher energy bonds in comparison to a single bond (but not necessarily 2-fold higher).
- Triple bonds are even higher energy bonds than double and single bonds (but not necessarily 3-fold higher).
Bond Breakage and Formation
When a chemical reaction occurs, the atoms in the reactants rearrange their chemical bonds to make products. The new arrangement of bonds does not have the same total energy as the bonds in the reactants. Therefore, when chemical reactions occur, there will always be an accompanying energy change.
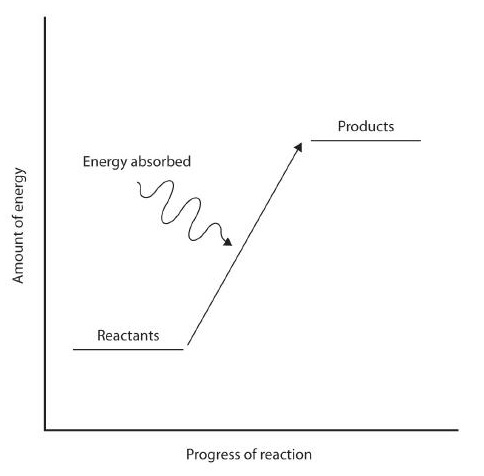.jpg?revision=1)
In some reactions, the energy of the products is lower than the energy of the reactants. Thus, in the course of the reaction, the substances lose energy to the surrounding environment. Such reactions are exothermic and can be represented by an energy-level diagram in Figure 1 (left). In most cases, the energy is given off as heat (although a few reactions give off energy as light). In chemical reactions where the products have a higher energy than the reactants, the reactants must absorb energy from their environment to react. These reactions are endothermic and can be represented by an energy-level diagrams like Figure 1 (right).
It is not uncommon that textbooks and instructors to consider heat as a independent "species" in a reaction. While this is rigorously incorrect because one cannot "add or remove heat" to a reaction as with species, it serves as a convenient mechanism to predict the shift of reactions with changing temperature. For example, if heat is a "reactant" (\(\Delta{H} > 0 \)), then the reaction favors the formation of products at elevated temperature. Similarly, if heat is a "product" (\(\Delta{H} < 0 \)), then the reaction favors the formation of reactants. A more accurate, and hence preferred, description is discussed below.
Exothermic and endothermic reactions can be thought of as having energy as either a "product" of the reaction or a "reactant." Exothermic reactions releases energy, so energy is a product. Endothermic reactions require energy, so energy is a reactant.
Is each chemical reaction exothermic or endothermic?
- \(2H_{2(g)} + O_{2(g)} \rightarrow 2H_2O_{(ℓ)} + \text{135 kcal}\)
- \(N_{2(g)} + O_{2(g)} + \text{45 kcal} \rightarrow 2NO_{(g)}\)
Solution
No calculates are required to address this question. Just look at where the "heat" is in the chemical reaction.
- Because energy is released; this reaction is exothermic.
- Because energy is absorbed; this reaction is endothermic.
If the bond energy for H-Cl is 431 kJ/mol. What is the overall bond energy of 2 moles of HCl?
Answer
Simply multiply the average bond energy of H-Cl by 2. This leaves you with 862 kJ (Table T3).
What is the enthalpy change for this reaction and is it endothermic or exothermic?
\[H_2(g)+I_2(g) \rightarrow 2HI(g)\]
SolutionFirst look at the equation and identify which bonds exist on in the reactants.
- one H-H bond and
- one I-I bond
Now do the same for the products
- two H-I bonds
Then identify the bond energies of these bonds from the table above:
- H-H bonds: 436 kJ/mol
- I-I bonds: 151 kJ/mol
The sum of enthalpies on the reaction side is:
436 kJ/mole + 151 kJ/mole = 587 kJ/mol.
This is how much energy is needed to break the bonds on the reactant side. Then we look at the bond formation which is on the product side:
- 2 mol H-I bonds: 297 kJ/mol
The sum of enthalpies on the product side is:
2 x 297 kJ/mol= 594 kJ/mol
This is how much energy is released when the bonds on the product side are formed. The net change of the reaction is therefore
587-594= -7 kJ/mol.
Since this is negative, the reaction is exothermic.
Using the bond energies given in the chart above, find the enthalpy change for the thermal decomposition of water:
\[ 2H_2O (g) \rightarrow 2H_2 + O_2 (g) \]
Is the reaction written above exothermic or endothermic? Explain.
Solution
The enthalpy change deals with breaking two mole of O-H bonds and the formation of 1 mole of O-O bonds and two moles of H-H bonds (Table T3).
- The sum of the energies required to break the bonds on the reactants side is 4 x 460 kJ/mol = 1840 kJ/mol.
- The sum of the energies released to form the bonds on the products side is
- 2 moles of H-H bonds = 2 x 436.4 kJ/mol = 872.8 kJ/mol
- 1 moles of O=O bond = 1 x 498.7 kJ/mil = 498.7 kJ/mol
which is an output (released) energy = 872.8 kJ/mol + 498.7 kJ/mol = 1371.5 kJ/mol.
Total energy difference is 1840 kJ/mol – 1371.5 kJ/mol = 469 kJ/mol, which indicates that the reaction is endothermic and that 469 kJ of heat is needed to be supplied to carry out this reaction.
This reaction is endothermic since it requires energy in order to create bonds.
Summary
Energy is released to generate bonds, which is why the enthalpy change for breaking bonds is positive. Energy is required to break bonds. Atoms are much happier when they are "married" and release energy because it is easier and more stable to be in a relationship (e.g., to generate octet electronic configurations). The enthalpy change is negative because the system is releasing energy when forming bond.
References
- Petrucci, Ralph H., Harwood, William S., Herring, F. G., and Madura Jeffrey D. General Chemistry: Principles and Modern Applications. 9th ed. Upper Saddle River: Pearson Education, Inc., 2007.
- Carruth, Gorton, Ehrlich, Eugene. "Bond Energies." Volume Library. Ed. Carruth, Gorton. Vol 1. Tennessee: Southwestern, 2002.
- For more practice problems: http://www.chalkbored.com/lessons/chemistry-11/bond-energies-worksheet.pdf
Contributors and Attributions
- Kim Song (UCD), Donald Le (UCD)

