19.1: Electrical Fundamentals
- Page ID
- 97476
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Introduction
In this chapter will introduce electrochemistry and be going over electrochemical cells, and so it seems prudent to introduce some of the fundamental concepts of electricity. This section does not have problem sets or activities, but will provide definitions you may want to refer back to as you are doing the other sections of this chapter. I am writing this section to help students who have not had physics and hope it will help make the other sections easier to understand.
Electricity refers to a number of phenomena associated with the presence and flow of electric charge. Electricity includes such diverse things as lightning, static electricity, the current generated by a battery as it discharges, and many other influences on our daily lives. The flow or movement of charge is an electric current (Figure \(\PageIndex{1}\)). Electrons or ions may carry the charge through a conductor. Wires, electrodes are electronic conductors while electrolytes are ionic conductors, and the current must flow through a circuit.

Figure \(\PageIndex{1}\): Electricity-related phenomena include lightning, accumulation of static electricity, and current produced by a battery. (credit left: modification of work by Thomas Bresson; credit middle: modification of work by Chris Darling; credit right: modification of work by Windell Oskay).
So what are the units that describe electricity? In section 1B.1.1 the 7 SI base units were introduced, and we learned that all measurable quantities can be quantified in terms of SI base units. So far in this class we have used 5 of the SI base units; mass (kg), time (s), length (m), temperature (K) and quantity (mol). We have not covered the SI units of electrical current, Ampere (A) or luminous intensity, candela (cd). This chapter uses the SI unit of the Ampere, which is a measure of electric current.
Units of Charge and Current
In May of 2019 the SI base unit definitions changed to depend on 7 immutable reference constants (defined numbers), and you should reference section 1B.1.3.1.2 of the first semester LibreText of this class. This change was initiated at the 26th meeting of Bureau International des Poids et Mesures, and the above 7 base units will be based on seven reference constants.
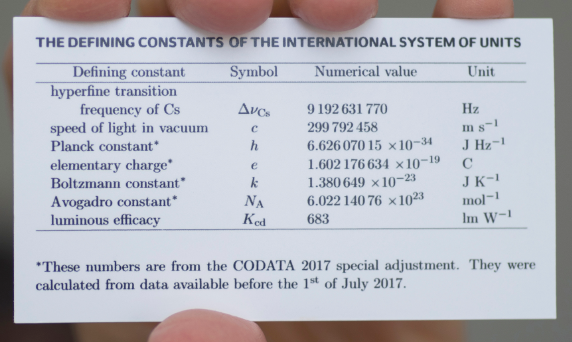
One of the 7 fundamental reference constants is the value of elementary charge, \(e = 1.602 176 634 × 10^{-19} Coulombs (C)\), which is the charge of an electron or proton: This constant is used in defining the SI base unit for charge, the ampere (A).
\[\text{Charge of Proton} = e = 1.602 176 634 × 10^{-19} Coulombs (C) \\ \text{Charge of Electron} = e = -1.602 176 634 × 10^{-19} Coulombs (C) \]
Rearranging, gives:
\[\text{1 Coulomb}= \text{charge of } 6.241509074 x 10^{18} electrons\]
Although in this class we won't need so many significant figures (and the charge of the electron is negative).
Note, the 7 reference constants are immutable constants, which either define SI base units, or enable their derivation. The SI base unit associated with electric charge is the Ampere (A), which is a unit of current flow, \[1\,A=\frac{1\,C}{s}.\] The ampere base unit can be described by the reference constants of charge (e) and of time (s), the latter of which is based on the caesium-133 atomic clock frequency of 9,192,631,770 cycles/second (Hz).
In the early 1800s Michael Faraday pioneered electrochemical studies and noted that if a current flowed into a solution with metal ions, some of the ions could become attached to the electrode that provided the electrons (Figure \(\PageIndex{3}\)). These cations migrated through the solution to the electrode and became "reduced", in that they gained electrons, and as we shall see, the electrode where reduction occurs is called the cathode. Such a technique could be used to plate silver onto a spoon as in Figure \(\PageIndex{3}\), but of interest, is that you can relate the mass gain of the spoon to the number of electrons transferred if you know the identity of the metal being plated onto the spoon.
Figure \(\PageIndex{3}\): Electroplating silver onto a spoon
In the above reaction silver cations migrate to the spoon which acts as the cathode (where the ions become reduced by electrons provided through the external source, and this reduction reaction can be described by the following equation.
\[\text{Ag}^{+} + e^{-} \rightarrow \text{Ag}\]
As the above equation relates one mol Ag+ being converted to one mol Ag(s) as one mol e– is transferred through the external circuit, the mass change can be related to the number of electrons transferred. Since the charge of an electron is defined through an SI constant (e= 1.602 176 634 × 10−19 Coulombs), the charge of a mole of electrons can be calculated, which is defined by a constant called Faraday's constant.
\[\begin{align} F & = \text{|charge of a mole of electrons|*} \nonumber \\ \nonumber \\ & = \text{(charge of one electron)(Avogadro's number)} \nonumber \\ \nonumber \\ & = \left ( 1.6022 × 10^{-19} \frac{C}{electron} \right )\left ( 6.0221 × 10^{23}\frac{electrons}{mol} \right ) \nonumber \\ \nonumber \\ &= 9.649 × 10^4 \frac{C}{mol \; electrons} \nonumber \\ \nonumber \\ F &= 96,500 \frac{C}{mol \; electrons} \end{align} \]
*Note, Faraday's constant is thus the charge of a mole of protons or electrons.
Electrical Potential
Energy is the capacity to do work or transfer heat. Translational work results when an applied force moves an object distance (\( \Delta x\)) along the axis the force is applied.
\[W=F \cdot \Delta x\]
The Joule is the unit of energy that is defined in terms of translation, with one Joule being the energy associated when a force of 1 newton moves an object 1 meter in a straight line along the axis the force is applied.
Electric work is that associated with moving a charge (q) across an electric potential gradient (E), Note: E is not energy but electric potential.
\[W=q \cdot \Delta E \]
this is commonly written as
\[W=qE\]
where E is the electric potential difference between two points and has the units of volts. The electric work is a measure of the energy (Joules) required or released as a charged particle (q) moves across the potential (volts).
One joule of work is done when one coulomb of charge moves across a potential difference of 1 volt.
\[1 \; Joule \; = \; 1 \; Coulomb \left ( 1\;Volt \right )\]
So \[1 Coulomb \;=\; \frac{1 \; Joule}{1 \;Volt}\]
Substituting for C in Faraday's constant gives
\[F=96,500 \frac{Joules}{Volt \cdot mol \; electrons}\]
So Faraday's constant can also be expressed in terms of J/Volt mole -electrons
The SI unit for electrical current is the SI base unit called the ampere (A), which is a flow rate of 1 coulomb of charge per second (1 A = 1 C/s). An electric current flows in a path, called an electric circuit. In most chemical systems, it is necessary to maintain a closed path for current to flow. The flow of charge is generated by an electrical potential difference, or potential, between two points in the circuit. Electrical potential is the ability of the electric field to do work on the charge. The SI unit of electrical potential is the volt (V). When 1 coulomb of charge moves through a potential difference of 1 volt, it gains or loses 1 joule (J) of energy. Table \(\PageIndex{1}\) summarizes some of this information about electricity.
| Quantity | Definition | Measure or Unit |
|---|---|---|
| Electric charge |
Charge of an electron |
1.602 × 10−19 C |
| Electric current (A) | The movement of charge | ampere = A = 1 C/s |
| Electric potential (E or V) | The force trying to move the charge | volt = V = J/C |
Sign Convention
Note that in electronics current flows from high potential to low potential, that is, it flows down the potential gradient. That is, the direction of flow is that of positive charge, but in reality, what flows for a conductive current are electrons, and those actually move towards the positive electrode.
Contributors and Attributions
Robert E. Belford (University of Arkansas Little Rock; Department of Chemistry). The breadth, depth and veracity of this work is the responsibility of Robert E. Belford, rebelford@ualr.edu. You should contact him if you have any concerns. This material has both original contributions, and content built upon prior contributions of the LibreTexts Community and other resources, including but not limited to:

