7.3: Symmetry Elements

A symmetry operation is an action that leaves an object looking the same after it has been carried out. For example, if we take a molecule of water and rotate it by 180° about an axis passing through the central O atom (between the two H atoms) it will look the same as before. Each symmetry operation has a corresponding symmetry element, which is the axis, plane, line or point with respect to which the symmetry operation is carried out. The symmetry element consists of all the points that stay in the same place when the symmetry operation is performed. In a rotation, the line of points that stay in the same place constitute a symmetry axis; in a reflection the points that remain unchanged make up a plane of symmetry. The symmetry elements that a molecule (and any other 3-D object) may possess are discussed below.

Symmetry Operations

A symmetry operation is a permutation of atoms such that the molecule is transformed into a state indistinguishable from the starting state.

\(E\): The Identity Symmetry

The identity operation consists of doing nothing, and the corresponding symmetry element is the entire molecule. Every molecule has at least this element. For example, the \(CHFClBr\) molecule in Figure \(\PageIndex{1}\). The identify symmetry is not indicated since all molecule exhibit this symmetry.

Figure \(\PageIndex{1}\): Example of Identify Symmetry: The \(CHFClBr\) molecule contain no other symmetry other than identity. Image created via Symmetry @ Otterbein site by Dean Johnston et al.

\(C_n\): an \(n\)-fold Axis of Rotation

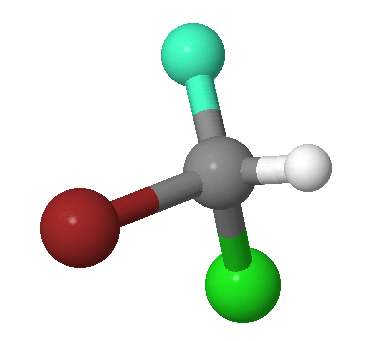
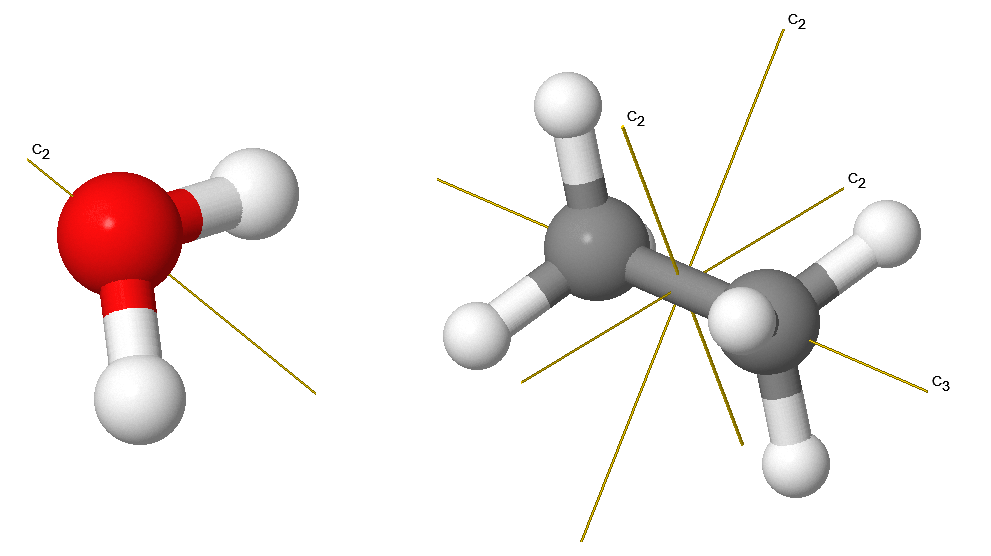
Rotation by \(360°/n\) leaves the molecule unchanged. The \(H_2O\) molecule has a \(C_2\) axis (Figure \(\PageIndex{2}\)). Some molecules have more than one \(C_n\) axis, in which case the one with the highest value of \(n\) is called the principal axis. Note that by convention rotations are counterclockwise about the axis. \(C_n\) rotations are indicated via vectors with labels as indicated below.

Figure \(\PageIndex{2}\): Examples of \(n\)-fold Axis of Rotation: (left) The water molecule contains a \(C_2\) axis. (right) Ethane contains both \(C_2\) and \(C_3\) axes. Image created via Symmetry @ Otterbein site by Dean Johnston et al.

\(\sigma\): a Plane of Symmetry

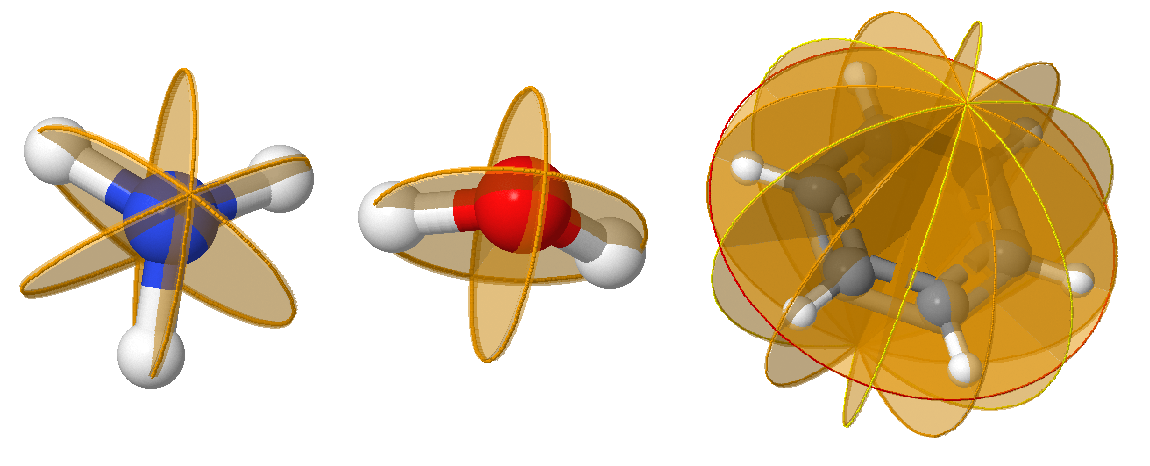
Reflection in the plane leaves the molecule looking the same. In a molecule that also has an axis of symmetry, a mirror plane that includes the axis is called a vertical mirror plane and is labeled \(\sigma_v\), while one perpendicular to the axis is called a horizontal mirror plane and is labeled \(\sigma_h\). A vertical mirror plane that bisects the angle between two \(C_2\) axes is called a dihedral mirror plane, \(\sigma_d\). \(\sigma\) symmetry is indicated as a plane on molecules; since they often bisect atoms, which should be clearly indicated.

Figure \(\PageIndex{3}\): Examples of reflection symmetry. (left) The ammonia molecule contains three identical reflection planes. All are designated as vertical symmetry planes (\(σ_v\)) because they contain the principle rotation axis.(middle) The water molecule contains two different reflection planes. (right) benzene contains a total of seven reflection planes, one horizontal plane (\(σ_h\)) and six vertical planes (\(σ_v\) and \(σ_d\)). Image created via Symmetry @ Otterbein site by Dean Johnston et al.

\(i\): a Center of Inversion Symmetry

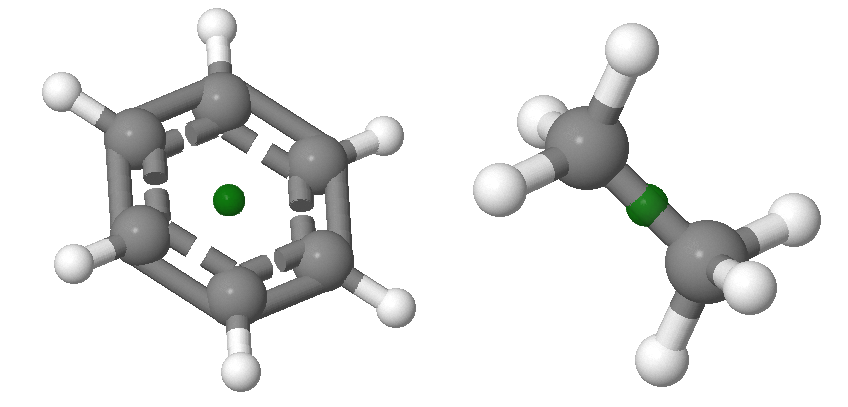
Inversion through the center of symmetry leaves the molecule unchanged. Inversion consists of passing each point through the center of inversion and out to the same distance on the other side of the molecule. Examples of molecules with centers of inversion is shown in Figure \(\PageIndex{4}\). Centers of inversion are indicated via a point, which may or may not overlap with an atoms. The centers of inversion in the examples below do not overlap with atoms.

Figure \(\PageIndex{4}\): Examples of Center of Inversion Symmetry. (left) Benzene and (right) staggard ethane have centers of inversion (green balls). Image created via Symmetry @ Otterbein site by Dean Johnston et al.

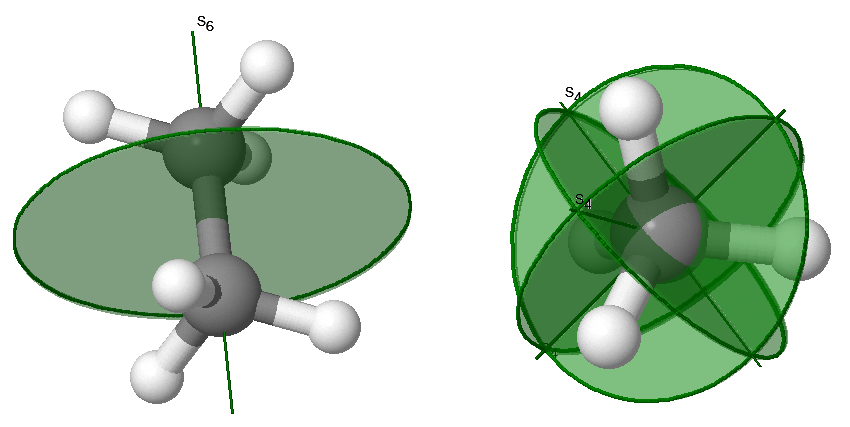
\(S_n\): an \(n\)-fold axis of improper rotation Symmetry

Improper rotations are also called a rotary-reflection axis. The rotary reflection operation consists of rotating through an angle \(360°/n\) about the axis, followed by reflecting in a plane perpendicular to the axis. Improper rotation symmetry is indicated with both an axis and a plan as demonstrated in the examples in Figure \(\PageIndex{5}\).

Figure \(\PageIndex{5}\): Examples of Improper axis of rotation. (left) Staggered ethane contains an \(S_6\) axis of improper rotation. (right) Methane contains three \(S_4\) axes of improper rotation. Image created via Symmetry @ Otterbein site by Dean Johnston et al.

Note

\(S_1\) is the same as reflection and \(S_2\) is the same as inversion.

The identity \(E\) and rotations \(C_n\) are symmetry operations that could actually be carried out on a molecule. For this reason they are called proper symmetry operations. Reflections, inversions and improper rotations can only be imagined (it is not actually possible to turn a molecule into its mirror image or to invert it without some fairly drastic rearrangement of chemical bonds) and as such, are termed improper symmetry operations. These five symmetry elements are tabulated in Table \(\PageIndex{1}\) with their corresponding operators.

Table \(\PageIndex{1}\): The five principal symmetry elements and their operators for 3D space

Symbol Elements	Description	Symbol Operator	Symbol
\(E\)	identity	\(\hat{E}\)	no change
\(C_n\)	\(n\)-fold axis of rotation	\(\hat{C}_n\)	Rotation by \(360°/n\) leaves the molecule unchanged
\(\sigma\)	plane of symmetry	\(\hat{\sigma}\)	Reflection in the plane leaves the molecule unchanged
\(i\)	center of symmetry.	\(\hat{i}\)	Inversion through the center of symmetry leaves the molecule unchanged.
\(S_n\)	\(n\)-fold improper rotation	\(\hat{S}_n\)	The rotary reflection operation consists of rotating through an angle \(360°/n\) about the axis, followed by reflecting in a plane perpendicular to the axis.

Axis Definitions

Conventionally, when imposing a set of Cartesian axes on a molecule (as we will need to do later on in the course), the \(z\) axis lies along the principal axis of the molecule, the \(x\) axis lies in the plane of the molecule (or in a plane containing the largest number of atoms if the molecule is non-planar), and the \(y\) axis makes up a right handed axis system.

Molecular Point Groups

It is only possible for certain combinations of symmetry elements to be present in a molecule (or any other object). As a result, we may group together molecules that possess the same symmetry elements and classify molecules according to their symmetry. These groups of symmetry elements are called point groups (due to the fact that there is at least one point in space that remains unchanged no matter which symmetry operation from the group is applied). There are two systems of notation for labeling symmetry groups, called the Schoenflies and Hermann-Mauguin (or International) systems. The symmetry of individual molecules is usually described using the Schoenflies notation, which is used below.

Table \(\PageIndex{2}\): Common Point Groups for Molecules

Nonaxial groups	C1	Cs	Ci	-	-	-	-	-	-
Cn groups	C2	C3	C4	C5	C6	C7	C8	-	-
Dn groups	D2	D3	D4	D5	D6	D7	D8	-	-
Cnv groups	C2v	C3v	C4v	C5v	C6v	C7v	C8v	-	-
Cnh groups	C2h	C3h	C4h	C5h	C6h	-	-	-	-
Dnh groups	D2h	D3h	D4h	D5h	D6h	D7h	D8h	-	-
Dnd groups	D2d	D3d	D4d	D5d	D6d	D7d	D8d	-	-
Sn groups	S2	-	S4	-	S6		S8	S10	S12
Cubic groups	T	Th	Td	O	Oh	I	Ih	-	-
Linear groups	C∞v	D∞h	-	-	-	-	-	-	-

Shared Names

Some of the point groups share their names with symmetry operations, so be careful you do not mix up the two. It is usually clear from the context which one is being referred to.

1. \(C_1\) - contains only the identity (a \(C_1\) rotation is a rotation by 360° and is the same as the identity operation) e.g. CHDFCl.

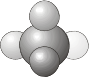
2. \(C_i\) - contains the identity \(E\) and a center of inversion \(i\).

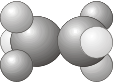
3. \(C_S\) - contains the identity \(E\) and a plane of reflection \(\sigma\).

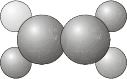
4. \(C_n\) - contains the identity and an \(n\)-fold axis of rotation.

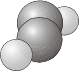
5. \(C_{nv}\) - contains the identity, an \(n\)-fold axis of rotation, and \(n\) vertical mirror planes \(\sigma_v\).

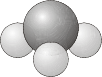
6. \(C_{nh}\) - contains the identity, an \(n\)-fold axis of rotation, and a horizontal reflection plane \(\sigma_h\) (note that in \(C_{2h}\) this combination of symmetry elements automatically implies a center of inversion).

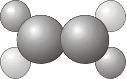
7. \(D_n\) - contains the identity, an \(n\)-fold axis of rotation, and \(n\) 2-fold rotations about axes perpendicular to the principal axis.

8. \(D_{nh}\) - contains the same symmetry elements as \(D_n\) with the addition of a horizontal mirror plane.

9. \(D_{nd}\) - contains the same symmetry elements as \(D_n\) with the addition of \(n\) dihedral mirror planes.

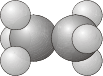
10. \(S_n\) - contains the identity and one \(S_n\) axis. Note that molecules only belong to \(S_n\) if they have not already been classified in terms of one of the preceding point groups (e.g. \(S_2\) is the same as \(C_i\), and a molecule with this symmetry would already have been classified).

The following groups are the cubic groups, which contain more than one principal axis. They separate into the tetrahedral groups (\(T_d\), \(T_h\) and \(T\)) and the octahedral groups (\(O\) and \(O_h\)). The icosahedral group also exists, but is not included below.

11. \(T_d\) - contains all the symmetry elements of a regular tetrahedron, including the identity, 4 \(C_3\) axes, 3 \(C_2\) axes, 6 dihedral mirror planes, and 3 \(S_4\) axes e.g. \(CH_4\).

12. \(T\) - as for \(T_d\) but no planes of reflection.
13. \(T_h\) - as for \(T\) but contains a center of inversion.
14. \(O_h\) - the group of the regular octahedron e.g. \(SF_6\).

15. \(O\) - as for \(O_h\), but with no planes of reflection.

The final group is the full rotation group \(R_3\), which consists of an infinite number of \(C_n\) axes with all possible values of \(n\) and describes the symmetry of a sphere. Atoms (but no molecules) belong to \(R_3\), and the group has important applications in atomic quantum mechanics. However, we won’t be treating it any further here.

Once you become more familiar with the symmetry elements and point groups described above, you will find it quite straightforward to classify a molecule in terms of its point group. In the meantime, the flowchart shown below provides a step-by-step approach to the problem.

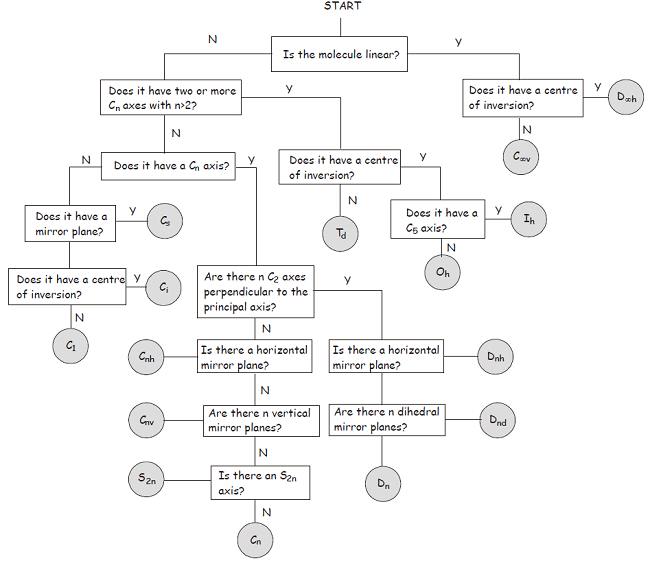
¹Though the Hermann-Mauguin system can be used to label point groups, it is usually used in the discussion of crystal symmetry. In crystals, in addition to the symmetry elements described above, translational symmetry elements are very important. Translational symmetry operations leave no point unchanged, with the consequence that crystal symmetry is described in terms of space groups rather than point groups.

Symmetry and physical properties

Carrying out a symmetry operation on a molecule must not change any of its physical properties. It turns out that this has some interesting consequences, allowing us to predict whether or not a molecule may be chiral or polar on the basis of its point group.

For a molecule to have a permanent dipole moment, it must have an asymmetric charge distribution. The point group of the molecule not only determines whether the molecule may have a dipole moment, but also in which direction(s) it may point. If a molecule has a \(C_n\) axis with \(n > 1\), it cannot have a dipole moment perpendicular to the axis of rotation (for example, a \(C_2\) rotation would interchange the ends of such a dipole moment and reverse the polarity, which is not allowed – rotations with higher values of \(n\) would also change the direction in which the dipole points). Any dipole must lie parallel to a \(C_n\) axis.

Also, if the point group of the molecule contains any symmetry operation that would interchange the two ends of the molecule, such as a \(\sigma_h\) mirror plane or a \(C_2\) rotation perpendicular to the principal axis, then there cannot be a dipole moment along the axis. The only groups compatible with a dipole moment are \(C_n\), \(C_{nv}\) and \(C_s\). In molecules belonging to \(C_n\) or \(C_{nv}\) the dipole must lie along the axis of rotation.

One example of symmetry in chemistry that you will already have come across is found in the isomeric pairs of molecules called enantiomers. Enantiomers are non-superimposable mirror images of each other, and one consequence of this symmetrical relationship is that they rotate the plane of polarized light passing through them in opposite directions. Such molecules are said to be chiral,² meaning that they cannot be superimposed on their mirror image. Formally, the symmetry element that precludes a molecule from being chiral is a rotation-reflection axis \(S_n\). Such an axis is often implied by other symmetry elements present in a group.

For example, a point group that has \(C_n\) and \(\sigma_h\) as elements will also have \(S_n\). Similarly, a center of inversion is equivalent to \(S_2\). As a rule of thumb, a molecule definitely cannot have be chiral if it has a center of inversion or a mirror plane of any type (\(\sigma_h\), \(\sigma_v\) or \(\sigma_d\)), but if these symmetry elements are absent the molecule should be checked carefully for an \(S_n\) axis before it is assumed to be chiral.

Chirality

The word chiral has its origins in the Greek word for hand (\(\chi\)\(\epsilon\)\(\rho\)\(\iota\), pronounced ‘cheri’ with a soft ch as in ‘loch’). A pair of hands is also a pair of non-superimposable mirror images, and you will often hear chirality referred to as ‘handedness’ for this reason.

Summary

All molecules can be described in terms of their symmetry or lack thereof, which may contain symmetry elements (point, line, plane). Reflection, rotation, and inversion are symmetry operations (movement of the molecules such that after the movement, all the atoms of the molecules is coincidental with equivalent atom of the molecule in original).

Contributors

• Claire Vallance (University of Oxford)