4.1.3: Molecular orbitals from d orbitals
- Page ID
- 241394
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)In transition metals and other heavier elements, the \(d\) orbitals may combine with other orbitals of compatible symmetry (and energy) to form molecular orbitals. generally, there are three types of bonding and antibonding interactions that may occur with \(d\) orbitals; \(\sigma\), \(\pi\), and \(\delta\) bonds.
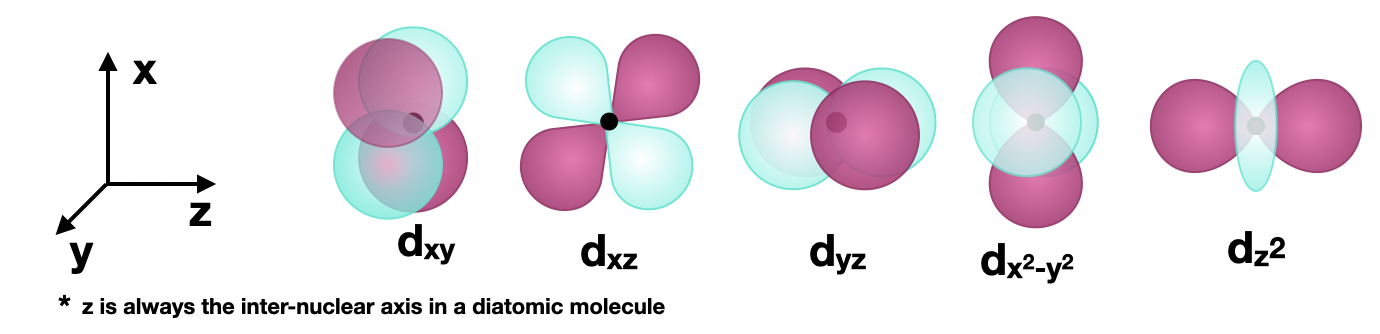 Figure \(\PageIndex{1}\): The five \(3d\) orbitals are shown. The orientation of the axes is consistent and \(z\) axis is horizontal for convinience in drawing bonding along the \(z\) axis (see examples below).
Figure \(\PageIndex{1}\): The five \(3d\) orbitals are shown. The orientation of the axes is consistent and \(z\) axis is horizontal for convinience in drawing bonding along the \(z\) axis (see examples below).
\(\sigma\) bonding with \(d\) orbitals
\(sigma\) bonds are symmetric with respect to the inter-nuclear axis (in a diatomic molecule, this is the \(z\) axis). An example of a \(\sigma\) bond formed by \(\d\) orbitals is that of two \(d_{z^2}\) orbitals (see Figure \(\PageIndex{2}\)). If a bonded atom is in a position other than on the \(z\) axis (in an octahedral geometry, for example), sigma bonds can also form. For example, two \(d_{x^2-y^2}\) orbital on atoms bonded along the \(x\) or \(y\) axes could also form a \(\sigma\) bond.
\(d\) orbitals can also form \(\sigma\) bonds with other types of orbitals with the appropriate symmetry. Examples of orbitals with appropriate symmetry are the \(s\) orbital and certain \(p\) orbitals on another atom, as shown below in Figure \(\PageIndex{2}\).
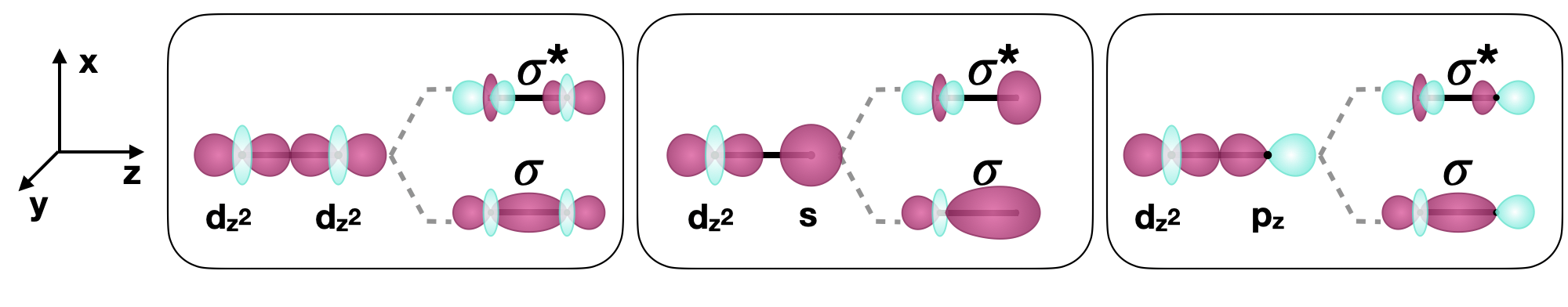 Figure \(\PageIndex{2}\): Selected examples of \(\sigma\) bonds involving \(d\) orbitals along the \(z\) internuclear axis (shown in bold horizontal line) between two atoms.
Figure \(\PageIndex{2}\): Selected examples of \(\sigma\) bonds involving \(d\) orbitals along the \(z\) internuclear axis (shown in bold horizontal line) between two atoms.
\(\pi\) bonding with \(d\) orbitals
\(\pi\) bonds are those with one node that is in-plane with the internuclear axis. \(\pi\) bonds can for between two \(d\) orbitals or between \(d\) orbitals and other types of orbitals with comparable symmetry. An example of a \(\pi\) bond between two \(d\) orbitals is that formed by two \(d_{xz}\) orbitals along the \(z\) axis (shown in Figure \(\PageIndex{3}\)). \(d\) orbitals can also form \(\pi\) bonds using \(p\) orbitals with compatible symmetry, as shown in Figure \(\PageIndex{3}\).
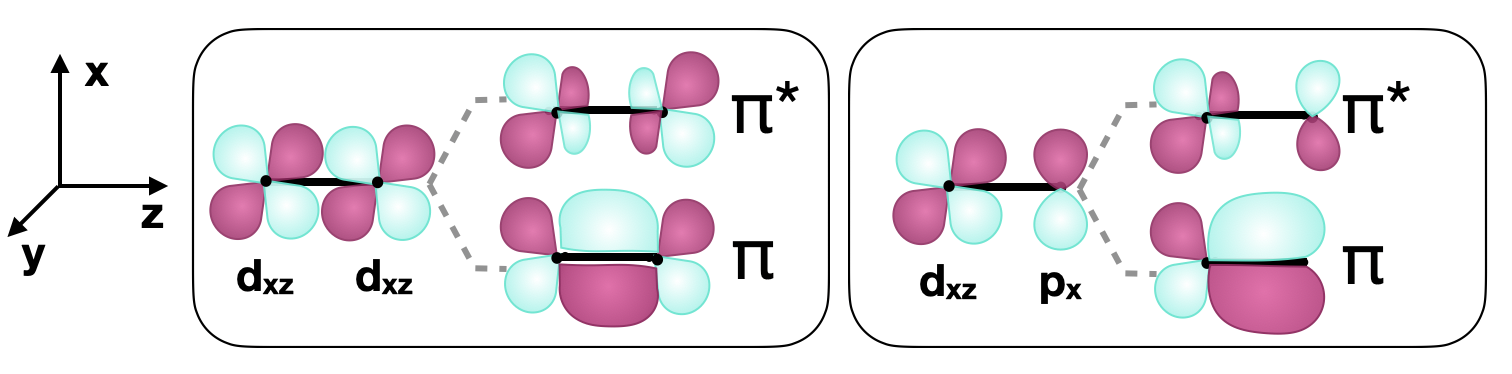
Figure \(\PageIndex{3}\): Selected examples of \(\pi\) bonds involving \(d\) orbitals along the \(z\) internuclear axis (shown in bold horizontal line) between two atoms.
\(\delta\) bonding with \(d\) orbitals
\(\delta \) bonds are those with two nodes that are in-plane with the internuclear axis. \(\delta\) bonds can form between two \(d\) orbitals with appropriate symmetry. For example, when two atoms bond along the \(z\) axis, the \(d_{xy}\) orbitals and the two \(d_{x^2-y^2}\) orbitals can form \(\delta\) bonds Figure \(\PageIndex{4}\)
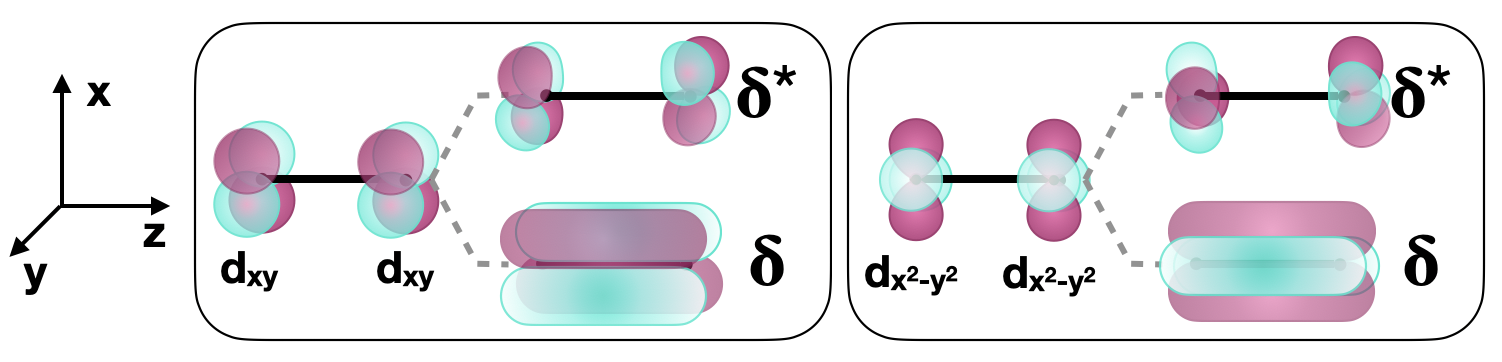 Figure \(\PageIndex{4}\): Selected examples of \(\delta\) bonds involving \(d\) orbitals along the \(z\) internuclear axis (shown in bold horizontal line) between two atoms.
Figure \(\PageIndex{4}\): Selected examples of \(\delta\) bonds involving \(d\) orbitals along the \(z\) internuclear axis (shown in bold horizontal line) between two atoms.
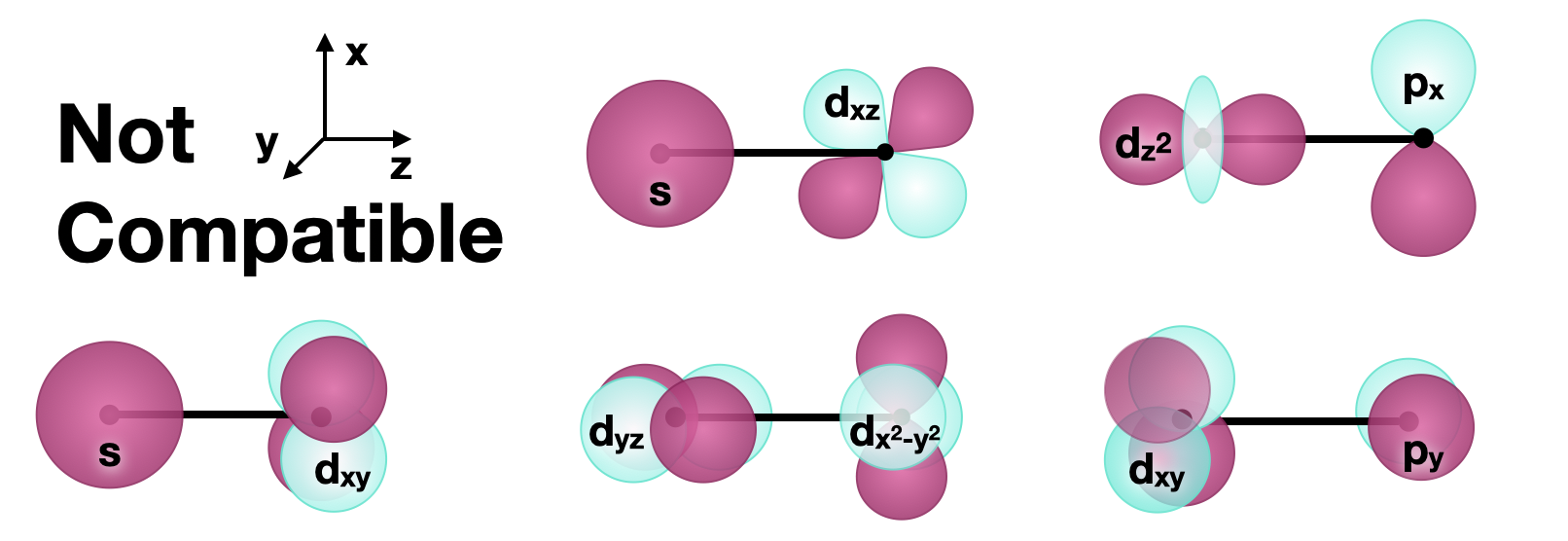
Incompatible orbitals
In the descriptions above, we focused on how bonds (and antibonds) can be formed with \(d\) orbitals. All bonding and non-bonding interactions require that orbitals have compatible symmetry to form productive interactions. It is worth mentioning that orbitals with symmetry that is incompatible with the \(d\) orbitals will not form bonding and antibonding interactions. The figure below shows several sets of orbitals that are incompatible for bonding.