9.2.2: Electronic Spectra - Ultraviolet and Visible Spectroscopy - Transition Metal Compounds and Complexes
- Page ID
- 222267
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)UV-Vis Spectroscopy
UV-Vis spectroscopy is an analytical chemistry technique used to determine the presence of various compounds, such as transition metals/transition metal ions, highly conjugated organic molecules, and more. However, due to the nature of this course, only transition metal complexes will be discussed. UV-Vis spectroscopy works by exciting a metal’s d-electron from the ground state configuration to an excited state using light. In short, when energy in the form of light is directed at a transition metal complex, a d-electron will gain energy and a UV-Vis spectrophotometer measures the abundance of transition metal atoms with excited electrons at a specific wavelength of light, from the visible light region to the UV region.
When using a UV-Vis Spectrophotometer, the solution to be analyzed is prepared by placing the sample in a cuvette then placing the cuvette inside the spectrophotometer. The machine then shines light waves from the visible and ultraviolet wavelengths and measures how much light of each wavelength the sample absorbs and then emits.
Absorbance of the sample can be calculated via Beer’s Law: A=εlc where A is the absorbance, ε is the molar absorptivity of the sample, l is the length of the cuvette used, and c is the concentration of the sample.[1] When the spectrophotometer produces the absorption graph, the molar absorptivity can then be calculated.

Figure \(\PageIndex{1}\). UV-Vis Spectrum of a Chromium(III) complex
To illustrate what this looks like, you will find a sample absorbance spectrum to the right.[2] 404-5</ref> </ref> As can be seen, the y-axis represents absorbance and the x-axis represents the wavelengths of light being scanned. This specific transition metal complex, [CrCl(NH3)5]2+, has the highest absorbance in the UV region of light, right around 250-275 nm, and two slight absorbance peaks near 400 nm and 575 nm respectively. The two latter peaks are much less pronounced than the former peak due to the electron’s transition being partially forbidden—a concept that will be discussed later in this chapter. If a transition is forbidden, not many transition metal electrons will undergo the excitation.
Theory Behind UV-Vis Spectroscopy
Splitting of the D-Orbitals
Figure \(\PageIndex{2}\). D orbitals
As is widely known, the d-orbitals contain five types of sub-orbitals: dxy, dyz, dxz, dx2-y2, and dz2 which are all shown to the right[3]. When in the absence of a magnetic field—such as when there are no electrons present—all the sub-orbitals combine together to form a degenerate spherical orbital. This singular orbital promptly differentiates back into its sub-orbitals when electrons are introduced or the transition metal is bonded to a set of ligands. 
Figure \(\PageIndex{3}\). This differentiation gives rise to the origin of color in metallic complexes.
The Origination of Color in Transition Metal Complexes
When looking at color in transition metal complexes, it is necessary to pay attention to the differentiated d-orbitals. Color in this sense originates from the excitation of d-orbital electrons from one energy level to the next. For instance, an electron in the t2g bonding orbital can be excited by light to the eg* bonding orbital and upon its descent back to the ground state, energy is released in the form of light: 
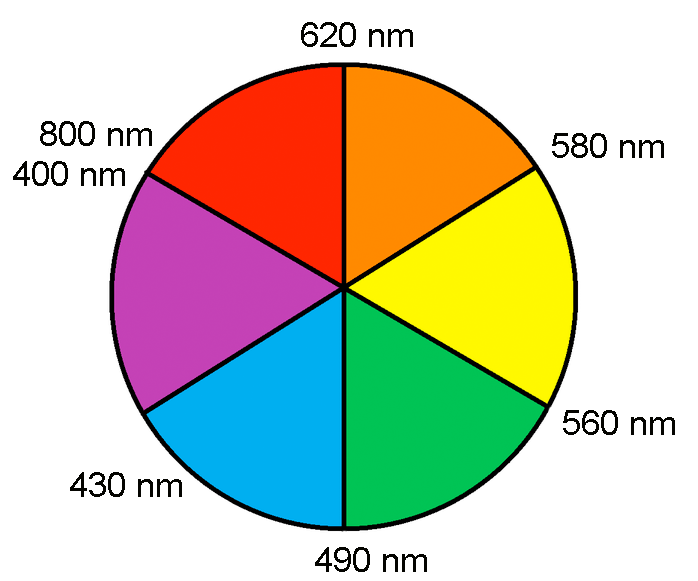
Figure \(\PageIndex{4}\). Colorwheel wavelengths
The specific wavelength of light required to excite an electron to the eg* orbital directly correlates to the color given off when the electron moves back down to the ground state. The figure on the right helps visualize the properties of transition metal color. Whichever color is absorbed, the complimentary color (directly opposite from the color in the figure) is emitted. For instance, if a metal complex emits green light we can figure out that the complex absorbed red light with a wavelength between 630 nm-750 nm in length.
Rules of Color Intensity and Forbidden Transitions
The intensity of the emitted color is based on two rules:[4]
- Spin multiplicity: the spin multiplicity of a complex cannot change when an electron is excited. Multiplicity can be calculated via the equation 2S+1 where S is calculated by (1/2)(number of unpaired d-electrons).
- If there is a center of symmetry in the molecule (i.e. center of inversion) then a g to g or u to u electron excitation is not allowed.
If a complex breaks one of these rules, we say it is a forbidden transition. If one rule is broken, it is singly forbidden. If two rules are broken, we call it double forbidden and so on. Even though the convention is to call it forbidden, this does not mean it will not happen; rather, the more rules the complex breaks, the more faded its color will be because the more unlikely the chances the transition will happen. Let’s again look at the previous example: 
If we apply the intensity rules to it:
- Multiplicity before transition=2(0.5[1 unpaired electron])+1=3, Multiplicity after transition=2(0.5[1 unpaired electron])+1=3. Both multiplicities are the same, so this transition is allowed under rule 1.
- If we assume this molecule is octahedral in symmetry, this means it has an inversion center and thus the transition of eg* to t2g is forbidden under rule 2 due to both orbitals being gerade (g).
- We are only exciting one electron and thus it is allowed under rule 3.
Based on these rules, we can see that this transition is only singly forbidden, and thus it will appear only slightly faded and light rather than a deep, rich green.
Ligand Field Theory: How Ligands Affect Color
As it turns out, the atoms bonded to a transition metal affect the wavelength that the complex needs to absorb in order to give off light; we refer to this as Ligand Field Theory. While certain transition metals like to absorb different wavelengths than other transition metals, the ligand(s) plays the most important role in determining the wavelength of light needed for electron excitation.[5]
The terms low spin and high spin are used to describe the difference in energy levels of the t2g and eg* orbitals, which correlates to the wavelength of light needed to excite an electron from t2g to eg*. When a complex is characterized as low spin, the ligands attached to the metal raise the energy of the eg* orbital so much that the ground state configuration of the complex fills the first six electrons in the t2g orbital before the eg* orbital is filled. As a result, high energy wavelengths of light—violet, blue, and green—are needed to successful excite an electron to the eg* bonding orbital. This means that the transition metal complex will emit yellow, orange, and red light, respectively. Conversely, high spin complexes have ligands which lower the energy level of the eg* orbital so that low energy light—red, orange, and yellow—or even high energy light can successfully excite an electron. Thus, a high spin complex can emit any color. High spin complexes can be thought of as all-inclusive while low spin complexes are half-exclusive in terms of the wavelengths needed to excite an electron.
To determine whether a complex is high spin or low spin:
- Look at the transition metal. First row metals will want to be high spin unless the ligand(s) forces it to be low spin. Second row metals want to be low spin unless the ligand(s) forces it high spin. Third row metals will low spin.
- Look at the ligand(s) as they will be the ultimate determining factor. If the ligand matches the transition metal in terms of high spin/low spin, then the complex’s spin will be whatever is “agreed” upon. If they differ, follow the ligand’s spin type. If the ligand is classified neither as high nor low spin, follow the transition metal’s spin type. Ligand spin types are enumerated below.
- If there are multiple ligands with differing spin types, go with whichever spin type is most abundant in the complex.
The ligands below are ranked from low spin (greatest energy difference between t2g and eg*) to high spin (lowest energy difference):[6] 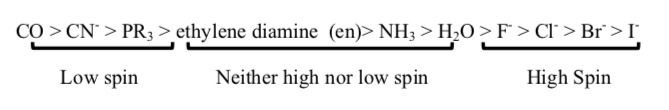
To illustrate this concept, let’s take the following complexes:
[Ni(NH3)6]2+, [Ni(CN)4]2-
The nickel complexes all have the same oxidation state on the metal (2+), and thus the same d-electron count. In the first complex, nickel wants to be high spin, while ammonia prefers neither high nor low spin. Therefore the complex will be high spin and emit blue light, which is an absorbance of orange—weak energy—light. For the second complex, nickel again wants to be high spin, but cyanide prefers low spin. As a result, the complex becomes low spin and will emit yellow light, which is an absorbance of violet—strong energy—light.
References
- Inorganic Chemistry, Miessler, Fischer, and Tarr, 2013, Pages 404 and 405
- ↑ Chemistry LibreTexts, Electronic Spectroscopy: Interpretation, https://chem.libretexts.org/Core/Physical_and_Theoretical_Chemistry/Spectroscopy/Electronic_Spectroscopy/Electronic_Spectroscopy%3A_Interpretation
- ↑ Principles of Inorganic Chemistry, Brian William Pfennig, 2015, Page 88
- ↑ Inorganic Chemistry, Miessler, Fischer, and Tarr, 2013, Page 414
- ↑ Principles of Inorganic Chemistry, Brian William Pfennig, 2015, Page 526
- ↑ Principles of Inorganic Chemistry, Brian William Pfennig, 2015, Page 523


