7.2: Concentrations of Solutions
- Page ID
- 236760
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Skills to Develop
- Define the concentration units of mass percentage, volume percentage, mass-volume percentage, parts-per-million (ppm), and parts-per-billion (ppb)
- Perform computations relating a solution’s concentration and its components’ volumes and/or masses using these units\
- Express concentrations of solution components using mole fraction and molality
- Describe the effect of solute concentration on various solution properties (vapor pressure, boiling point, freezing point, and osmotic pressure)
- Perform calculations using the mathematical equations that describe these various colligative effects
Last term, we introduced molarity, a very useful measurement unit for evaluating the concentration of solutions. However, molarity is only one measure of concentration. In this section, we will introduce some other units of concentration that are commonly used in various applications, either for convenience or by convention.
Mass Percentage
Percentages are commonly used to express the composition of mixtures, including solutions. The mass percentage of a solution component is defined as the ratio of the component’s mass to the solution’s mass, expressed as a percentage:
\[ \text{mass percentage} = \dfrac{\text{mass of component}}{\text{mass of solution}} \times100\% \label{3.5.1}\]
We are generally most interested in the mass percentages of solutes, but it is also possible to compute the mass percentage of solvent.
Mass percentage is also referred to by similar names such as percent mass, percent weight, weight/weight percent, and other variations on this theme. The most common symbol for mass percentage is simply the percent sign, %, although more detailed symbols are often used including %mass, %weight, and (w/w)%. Use of these more detailed symbols can prevent confusion of mass percentages with other types of percentages, such as volume percentages (to be discussed later in this section).
Mass percentages are popular concentration units for consumer products. The label of a typical liquid bleach bottle (Figure \(\PageIndex{1}\)) cites the concentration of its active ingredient, sodium hypochlorite (NaOCl), as being 7.4%. A 100.0-g sample of bleach would therefore contain 7.4 g of NaOCl.

Figure \(\PageIndex{1}\): Liquid bleach is an aqueous solution of sodium hypochlorite (NaOCl). This brand has a concentration of 7.4% NaOCl by mass.
Example \(\PageIndex{1}\): Calculation of Percent by Mass
A 5.0-g sample of spinal fluid contains 3.75 mg (0.00375 g) of glucose. What is the percent by mass of glucose in spinal fluid?
Solution
The spinal fluid sample contains roughly 4 mg of glucose in 5000 mg of fluid, so the mass fraction of glucose should be a bit less than one part in 1000, or about 0.1%. Substituting the given masses into the equation defining mass percentage yields:
\[\mathrm{\%\,glucose=\dfrac{3.75\;mg \;glucose \times \frac{1\;g}{1000\; mg}}{5.0\;g \;spinal\; fluid}=0.075\%}\]
The computed mass percentage agrees with our rough estimate (it’s a bit less than 0.1%).
Note that while any mass unit may be used to compute a mass percentage (mg, g, kg, oz, and so on), the same unit must be used for both the solute and the solution so that the mass units cancel, yielding a dimensionless ratio. In this case, we converted the units of solute in the numerator from mg to g to match the units in the denominator. We could just as easily have converted the denominator from g to mg instead. As long as identical mass units are used for both solute and solution, the computed mass percentage will be correct.
Exercise \(\PageIndex{1}\)
A bottle of a tile cleanser contains 135 g of HCl and 775 g of water. What is the percent by mass of HCl in this cleanser?
- Answer
-
14.8%
Example \(\PageIndex{2}\): Calculations using Mass Percentage
“Concentrated” hydrochloric acid is an aqueous solution of 37.2% HCl that is commonly used as a laboratory reagent. The density of this solution is 1.19 g/mL. What mass of HCl is contained in 0.500 L of this solution?
Solution
The HCl concentration is near 40%, so a 100-g portion of this solution would contain about 40 g of HCl. Since the solution density isn’t greatly different from that of water (1 g/mL), a reasonable estimate of the HCl mass in 500 g (0.5 L) of the solution is about five times greater than that in a 100 g portion, or \(\mathrm{5 \times 40 = 200\: g}\). To derive the mass of solute in a solution from its mass percentage, we need to know the corresponding mass of the solution. Using the solution density given, we can convert the solution’s volume to mass, and then use the given mass percentage to calculate the solute mass. This mathematical approach is outlined in this flowchart:
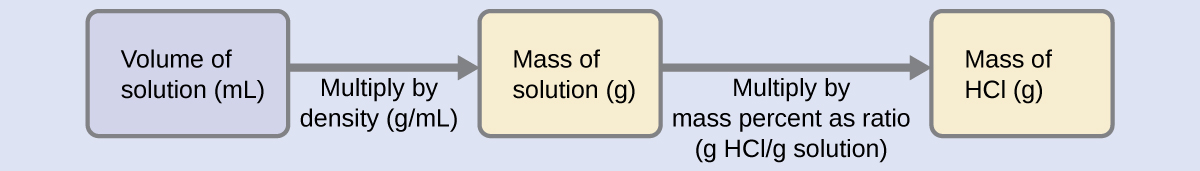
For proper unit cancellation, the 0.500-L volume is converted into 500 mL, and the mass percentage is expressed as a ratio, 37.2 g HCl/g solution:
\[ \mathrm{500\; mL\; solution \left(\dfrac{1.19\;g \;solution}{mL \;solution}\right) \left(\dfrac{37.2\;g\; HCl}{100\;g \;solution}\right)=221\;g\; HCl}\]
This mass of HCl is consistent with our rough estimate of approximately 200 g.
Exercise \(\PageIndex{2}\)
What volume of concentrated HCl solution contains 125 g of HCl?
- Answer
-
282 mL
Volume Percentage
Liquid volumes over a wide range of magnitudes are conveniently measured using common and relatively inexpensive laboratory equipment. The concentration of a solution formed by dissolving a liquid solute in a liquid solvent is therefore often expressed as a volume percentage, %vol or (v/v)%:
\[ \text{volume percentage} = \dfrac{\text{volume solute}}{\text{volume solution}} \times100\% \label{3.5.2}\]
Example \(\PageIndex{3}\): Calculations using Volume Percentage
Rubbing alcohol (isopropanol) is usually sold as a 70%vol aqueous solution. If the density of isopropyl alcohol is 0.785 g/mL, how many grams of isopropyl alcohol are present in a 355 mL bottle of rubbing alcohol?
Solution
Per the definition of volume percentage, the isopropanol volume is 70% of the total solution volume. Multiplying the isopropanol volume by its density yields the requested mass:
\[ \text {355 mL solution}(\frac{\text{70 mL isopropyl alcohol}}{\text{100 mL solution}})(\frac{\text{0.785 g isopropyl alcohol}}{\text{1 mL isopropyl alcohol}})=\text{195 g isopropyl alcohol}\]
Exercise \(\PageIndex{3}\)
Wine is approximately 12% ethanol (\(\ce{CH_3CH_2OH}\)) by volume. Ethanol has a molar mass of 46.06 g/mol and a density 0.789 g/mL. How many moles of ethanol are present in a 750-mL bottle of wine?
- Answer
-
1.5 mol ethanol
Mass-Volume Percentage
“Mixed” percentage units, derived from the mass of solute and the volume of solution, are popular for certain biochemical and medical applications. A mass-volume percent is a ratio of a solute’s mass to the solution’s volume expressed as a percentage. The specific units used for solute mass and solution volume may vary, depending on the solution. For example, physiological saline solution, used to prepare intravenous fluids, has a concentration of 0.9% mass/volume (m/v), indicating that the composition is 0.9 g of solute per 100 mL of solution. The concentration of glucose in blood (commonly referred to as “blood sugar”) is also typically expressed in terms of a mass-volume ratio. Though not expressed explicitly as a percentage, its concentration is usually given in milligrams of glucose per deciliter (100 mL) of blood (Figure \(\PageIndex{2}\)).

Figure \(\PageIndex{2}\): “Mixed” mass-volume units are commonly encountered in medical settings. (a) The NaCl concentration of physiological saline is 0.9% (m/v). (b) This device measures glucose levels in a sample of blood. The normal range for glucose concentration in blood (fasting) is around 70–100 mg/dL. (credit a: modification of work by “The National Guard”/Flickr; credit b: modification of work by Biswarup Ganguly).
Parts per Million and Parts per Billion
Very low solute concentrations are often expressed using appropriately small units such as parts per million (ppm) or parts per billion (ppb). Like percentage (“part per hundred”) units, ppm and ppb may be defined in terms of masses, volumes, or mixed mass-volume units. There are also ppm and ppb units defined with respect to numbers of atoms and molecules.
The mass-based definitions of ppm and ppb are given here:
\[\text{ppm}=\dfrac{\text{mass solute}}{\text{mass solution}} \times 10^6\; \text{ppm} \label{3.5.3A}\]
\[\text{ppb}=\dfrac{\text{mass solute}}{\text{mass solution}} \times 10^9\; \text{ppb} \label{3.5.3B}\]
Both ppm and ppb are convenient units for reporting the concentrations of pollutants and other trace contaminants in water. Concentrations of these contaminants are typically very low in treated and natural waters, and their levels cannot exceed relatively low concentration thresholds without causing adverse effects on health and wildlife. For example, the EPA has identified the maximum safe level of fluoride ion in tap water to be 4 ppm. Inline water filters are designed to reduce the concentration of fluoride and several other trace-level contaminants in tap water (Figure \(\PageIndex{3}\)).
Figure \(\PageIndex{3}\): (a) In some areas, trace-level concentrations of contaminants can render unfiltered tap water unsafe for drinking and cooking. (b) Inline water filters reduce the concentration of solutes in tap water. (credit a: modification of work by Jenn Durfey; credit b: modification of work by “vastateparkstaff”/Wikimedia commons).
Example \(\PageIndex{4}\): Parts per Million and Parts per Billion Concentrations
According to the EPA, when the concentration of lead in tap water reaches 15 ppb, certain remedial actions must be taken. What is this concentration in ppm? At this concentration, what mass of lead (μg) would be contained in a typical glass of water (300 mL)?
Solution
The definitions of the ppm and ppb units may be used to convert the given concentration from ppb to ppm. Comparing these two unit definitions shows that ppm is 1000 times greater than ppb (1 ppm = 103 ppb). Thus:
\[ \mathrm{15\; \cancel{ppb} \times \dfrac{1\; ppm}{10^3\;\cancel{ppb}} =0.015\; ppm}\]
The definition of the ppb unit may be used to calculate the requested mass if the mass of the solution is provided. However, only the volume of solution (300 mL) is given, so we must use the density to derive the corresponding mass. We can assume the density of tap water to be roughly the same as that of pure water (~1.00 g/mL), since the concentrations of any dissolved substances should not be very large. Rearranging the equation defining the ppb unit and substituting the given quantities yields:
\[\text{ppb}=\dfrac{\text{mass solute}}{\text{mass solution}} ×10^9\; \text{ppb}\]
\[\text{mass solute} = \dfrac{\text{ppb} \times \text{mass solution}}{10^9\;\text{ppb}}\]
\[\text{mass solute}=\mathrm{\dfrac{15\:ppb×300\:mL×\dfrac{1.00\:g}{mL}}{10^9\:ppb}=4.5 \times 10^{-6}\;g}\]
Finally, convert this mass to the requested unit of micrograms:
\[\mathrm{4.5 \times 10^{−6}\;g \times \dfrac{1\; \mu g}{10^{−6}\;g} =4.5\; \mu g}\]
Exercise \(\PageIndex{4}\)
A 50.0-g sample of industrial wastewater was determined to contain 0.48 mg of mercury. Express the mercury concentration of the wastewater in ppm and ppb units.
- Answer
-
9.6 ppm, 9600 ppb
The properties of a solution are different from those of either the pure solute(s) or solvent. Many solution properties are dependent upon the chemical identity of the solute. Compared to pure water, a solution of hydrogen chloride is more acidic, a solution of ammonia is more basic, a solution of sodium chloride is more dense, and a solution of sucrose is more viscous. There are a few solution properties, however, that depend only upon the total concentration of solute species, regardless of their identities. These colligative properties include vapor pressure lowering, boiling point elevation, freezing point depression, and osmotic pressure. This small set of properties is of central importance to many natural phenomena and technological applications, as will be described in this module.
Mole Fraction and Molality
Several units commonly used to express the concentrations of solution components were introduced in an earlier chapter of this text, each providing certain benefits for use in different applications. For example, molarity (M) is a convenient unit for use in stoichiometric calculations, since it is defined in terms of the molar amounts of solute species:
\[M=\dfrac{\text{mol solute}}{\text{L solution}} \label{11.5.1}\]
Because solution volumes vary with temperature, molar concentrations will likewise vary. When expressed as molarity, the concentration of a solution with identical numbers of solute and solvent species will be different at different temperatures, due to the contraction/expansion of the solution. More appropriate for calculations involving many colligative properties are mole-based concentration units whose values are not dependent on temperature. Two such units are mole fraction (introduced in the previous chapter on gases) and molality.
The mole fraction, \(X\), of a component is the ratio of its molar amount to the total number of moles of all solution components:
\[X_\ce{A}=\dfrac{\text{mol A}}{\text{total mol of all components}} \label{11.5.2}\]
Molality is a concentration unit defined as the ratio of the numbers of moles of solute to the mass of the solvent in kilograms:
\[m=\dfrac{\text{mol solute}}{\text{kg solvent}} \label{11.5.3}\]
Since these units are computed using only masses and molar amounts, they do not vary with temperature and, thus, are better suited for applications requiring temperature-independent concentrations, including several colligative properties, as will be described in this chapter module.
Example \(\PageIndex{5}\): Calculating Mole Fraction and Molality
The antifreeze in most automobile radiators is a mixture of equal volumes of ethylene glycol and water, with minor amounts of other additives that prevent corrosion. What are the (a) mole fraction and (b) molality of ethylene glycol, C2H4(OH)2, in a solution prepared from \(\mathrm{2.22 \times 10^3 \;g}\) of ethylene glycol and \(\mathrm{2.00 \times 10^3\; g}\) of water (approximately 2 L of glycol and 2 L of water)?
Solution
(a) The mole fraction of ethylene glycol may be computed by first deriving molar amounts of both solution components and then substituting these amounts into the unit definition.
\(\mathrm{mol\:H_2O=2000\:g×\dfrac{1\:mol\:H_2O}{18.02\:g\:H_2O}=111\:mol\:H_2O}\)
\(X_\mathrm{ethylene\:glycol}=\mathrm{\dfrac{35.8\:mol\:C_2H_4(OH)_2}{(35.8+111)\:mol\: total}=0.245}\)
Notice that mole fraction is a dimensionless property, being the ratio of properties with identical units (moles).
(b) To find molality, we need to know the moles of the solute and the mass of the solvent (in kg).
First, use the given mass of ethylene glycol and its molar mass to find the moles of solute:
Then, convert the mass of the water from grams to kilograms:
Finally, calculate molarity per its definition:
\ce{molality}&=\mathrm{\dfrac{mol\: solute}{kg\: solvent}}\\
\ce{molality}&=\mathrm{\dfrac{35.8\:mol\:C_2H_4(OH)_2}{2\:kg\:H_2O}}\\
\ce{molality}&=17.9\:m
\end{align}\)
Exercise \(\PageIndex{5}\)
What are the mole fraction and molality of a solution that contains 0.850 g of ammonia, NH3, dissolved in 125 g of water?
- Answer
-
7.14 × 10−3; 0.399 m
Example \(\PageIndex{6}\): Converting Mole Fraction and Molal Concentrations
Calculate the mole fraction of solute and solvent in a 3.0 m solution of sodium chloride.
Solution
Converting from one concentration unit to another is accomplished by first comparing the two unit definitions. In this case, both units have the same numerator (moles of solute) but different denominators. The provided molal concentration may be written as:
\[\mathrm{\dfrac{3.0\;mol\; NaCl}{1.0\; kg\; H_2O}} \label{11.5.X}\]
The numerator for this solution’s mole fraction is, therefore, 3.0 mol NaCl. The denominator may be computed by deriving the molar amount of water corresponding to 1.0 kg
\(\mathrm{1.0\:kg\:H_2O\left(\dfrac{1000\:g}{1\:kg}\right)\left(\dfrac{mol\:H_2O}{18.02\:g}\right)=55\:mol\:H_2O}\)
and then substituting these molar amounts into the definition for mole fraction.
\[\begin{align*}
X_\mathrm{H_2O}&=\mathrm{\dfrac{mol\:H_2O}{mol\: NaCl + mol\:H_2O}}\\
X_\mathrm{H_2O}&=\mathrm{\dfrac{55\:mol\:H_2O}{3.0\:mol\: NaCl+55\:mol\:H_2O}}\\
X_\mathrm{H_2O}&=0.95\\
X_\mathrm{NaCl}&=\mathrm{\dfrac{mol\: NaCl}{mol\: NaCl+mol\:H_2O}}\\
X_\mathrm{NaCl}&=\mathrm{\dfrac{3.0\:mol\:NaCl}{3.0\:mol\: NaCl+55\:mol\:H_2O}}\\
X_\mathrm{NaCl}&=0.052
\end{align*}\]
Exercise \(\PageIndex{6}\)
The mole fraction of iodine, \(\mathrm{I_2}\), dissolved in dichloromethane, \(\mathrm{CH_2Cl_2}\), is 0.115. What is the molal concentration, m, of iodine in this solution?
- Answer
-
1.50 m
Summary
Video \(\PageIndex{2}\): An overview of concentration units for solutions.
In addition to molarity, a number of other solution concentration units are used in various applications. Percentage concentrations based on the solution components’ masses, volumes, or both are useful for expressing relatively high concentrations, whereas lower concentrations are conveniently expressed using ppm or ppb units. These units are popular in environmental, medical, and other fields where mole-based units such as molarity are not as commonly used.
Footnotes
- A nonelectrolyte shown for comparison.
Glossary
- mass percentage
- ratio of solute-to-solution mass expressed as a percentage
- mass-volume percent
- ratio of solute mass to solution volume, expressed as a percentage
- parts per billion (ppb)
- ratio of solute-to-solution mass multiplied by 109
- parts per million (ppm)
- ratio of solute-to-solution mass multiplied by 106
- volume percentage
- ratio of solute-to-solution volume expressed as a percentage
- boiling point elevation
- elevation of the boiling point of a liquid by addition of a solute
- boiling point elevation constant
- the proportionality constant in the equation relating boiling point elevation to solute molality; also known as the ebullioscopic constant
- colligative property
- property of a solution that depends only on the concentration of a solute species
- freezing point depression
- lowering of the freezing point of a liquid by addition of a solute
- freezing point depression constant
- (also, cryoscopic constant) proportionality constant in the equation relating freezing point depression to solute molality
- ion pair
- solvated anion/cation pair held together by moderate electrostatic attraction
- molality (m)
- a concentration unit defined as the ratio of the numbers of moles of solute to the mass of the solvent in kilograms
- van’t Hoff factor (i)
- the ratio of the number of moles of particles in a solution to the number of moles of formula units dissolved in the solution
Contributors
Paul Flowers (University of North Carolina - Pembroke), Klaus Theopold (University of Delaware) and Richard Langley (Stephen F. Austin State University) with contributing authors. Textbook content produced by OpenStax College is licensed under a Creative Commons Attribution License 4.0 license. Download for free at http://cnx.org/contents/85abf193-2bd...a7ac8df6@9.110).
- Adelaide Clark, Oregon Institute of Technology
- Crash Course Chemistry: Crash Course is a division of Complexly and videos are free to stream for educational purposes.

