2.3: Atomic Orbitals and the Bohr Model
- Page ID
- 119819
Skills to Develop
- Describe the Bohr model of the hydrogen atom
- Use the Rydberg equation to calculate energies of light emitted or absorbed by hydrogen atoms
- Understand the general idea of the quantum mechanical description of electrons in an atom, and that it uses the notion of three-dimensional wave functions, or orbitals, that define the distribution of probability to find an electron in a particular part of space
The Bohr Model and Spectroscopy
Video \(\PageIndex{1}\): An introduction to the Bohr Model of the Atom.
In 1913, Niels Bohr attempted to resolve the atomic paradox by ignoring classical electromagnetism’s prediction that the orbiting electron in hydrogen would continuously emit light. Instead, he incorporated into the classical mechanics description of the atom Planck’s ideas of quantization and Einstein’s finding that light consists of photons whose energy is proportional to their frequency. Bohr assumed that the electron orbiting the nucleus would not normally emit any radiation (the stationary state hypothesis), but it would emit or absorb a photon if it moved to a different orbit. The energy absorbed or emitted would reflect differences in the orbital energies according to this equation:
\[ |ΔE|=|E_f−E_i|=h\nu=\dfrac{hc}{\lambda}\]
In this equation, h is Planck’s constant and Ei and Ef are the initial and final orbital energies, respectively. The absolute value of the energy difference is used, since frequencies and wavelengths are always positive. Instead of allowing for continuous values for the angular momentum, energy, and orbit radius, Bohr assumed that only discrete values for these could occur (actually, quantizing any one of these would imply that the other two are also quantized). Bohr’s expression for the quantized energies is:
\[E_n=−\dfrac{k}{n^2} \label{6.3.2}\]
with \(n=1,2,3, ...\)In this expression, \(k\) is a constant comprising fundamental constants such as the electron mass and charge and Planck’s constant. Inserting the expression for the orbit energies into the equation for \(ΔE\) gives
or
\[ \dfrac{1}{\lambda}=\dfrac{k}{hc} \left(\dfrac{1}{n^2_1}−\dfrac{1}{n_2^2}\right) \label{6.3.4}\]
\[ΔE=E_1−E_2=2.179 \times 10^{−18}\left(\dfrac{1}{n^2_1}−\dfrac{1}{n_2^2}\right)\]
This formula should look familiar - we talked about it in the previous section when we talked about the principle quantum number. The lowest few energy levels are shown in Figure \(\PageIndex{1}\). One of the fundamental laws of physics is that matter is most stable with the lowest possible energy. Thus, the electron in a hydrogen atom usually moves in the \(n = 1\) orbit, the orbit in which it has the lowest energy. When the electron is in this lowest energy orbit, the atom is said to be in its ground electronic state (or simply ground state). If the atom receives energy from an outside source, it is possible for the electron to move to an orbit with a higher \(n\) value and the atom is now in an excited electronic state (or simply an excited state) with a higher energy. When an electron transitions from an excited state (higher energy orbit) to a less excited state, or ground state, the difference in energy is emitted as a photon. Similarly, if a photon is absorbed by an atom, the energy of the photon moves an electron from a lower energy orbit up to a more excited one.
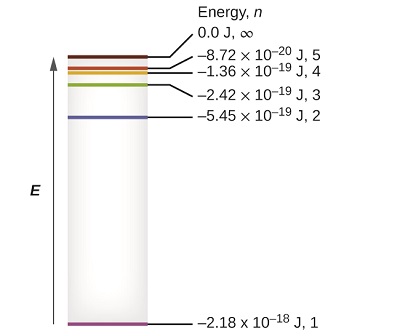
Figure \(\PageIndex{1}\): Quantum numbers and energy levels in a hydrogen atom. The more negative the calculated value, the lower the energy.
We can relate the energy of electrons in atoms to what we learned previously about energy. The law of conservation of energy says that we can neither create nor destroy energy. Thus, if a certain amount of external energy is required to excite an electron from one energy level to another, that same amount of energy will be liberated when the electron returns to its initial state (Figure \(\PageIndex{2}\)). In effect, an atom can “store” energy by using it to promote an electron to a state with a higher energy and release it when the electron returns to a lower state. The energy can be released as one quantum of energy, as the electron returns to its ground state (say, from \(n = 5\) to \(n = 1\)), or it can be released as two or more smaller quanta as the electron falls to an intermediate state, then to the ground state (say, from \(n = 5\) to \(n = 4\), emitting one quantum, then to \(n = 1\), emitting a second quantum).
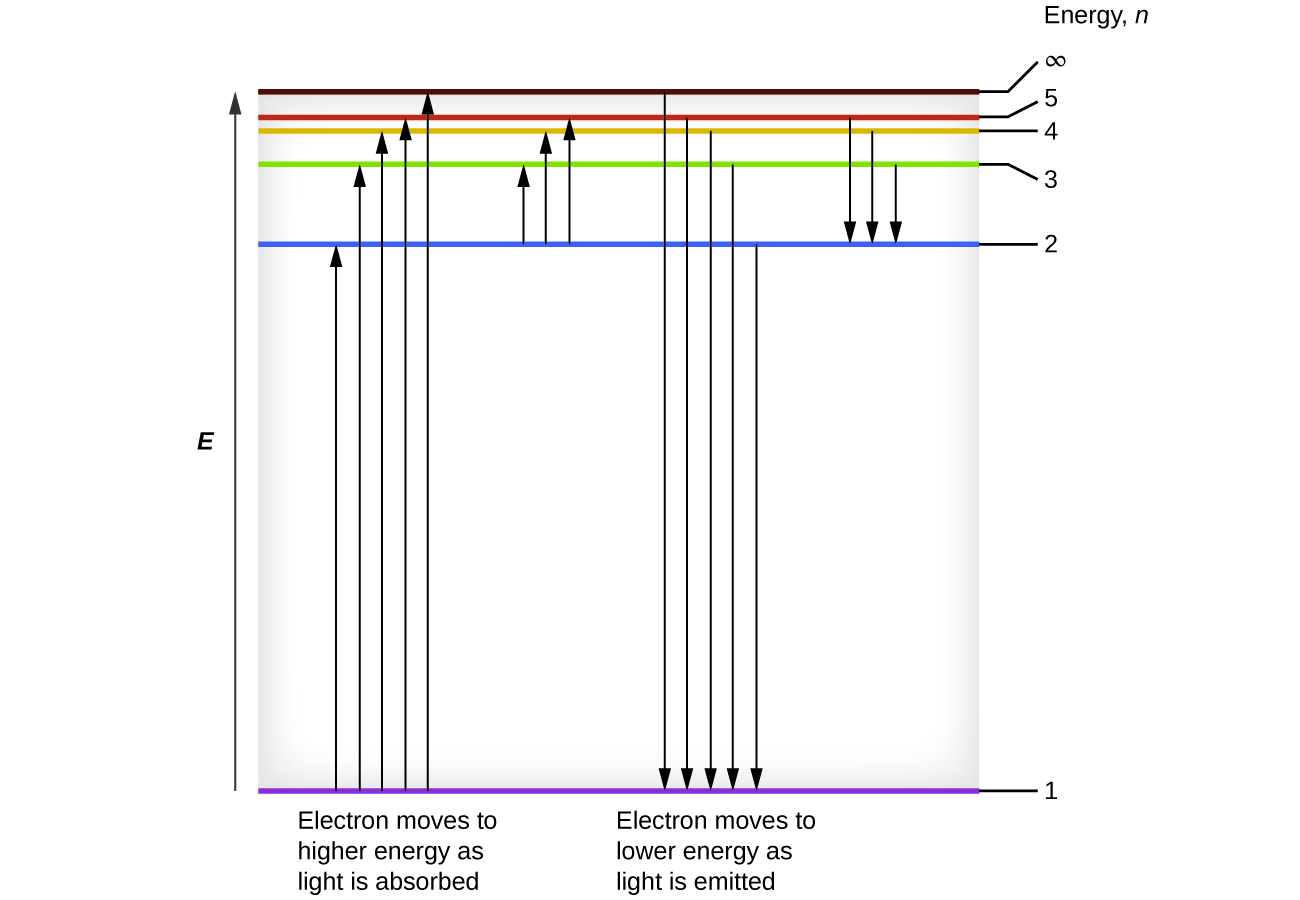
Figure \(\PageIndex{2}\): The horizontal lines show the relative energy of orbits in the Bohr model of the hydrogen atom, and the vertical arrows depict the energy of photons absorbed (left) or emitted (right) as electrons move between these orbits.
Since Bohr’s model involved only a single electron, it could also be applied to the single electron ions He+, Li2+, Be3+, and so forth, which differ from hydrogen only in their nuclear charges, and so one-electron atoms and ions are collectively referred to as hydrogen-like or hydrogenic atoms. The energy expression for hydrogen-like atoms is a generalization of the hydrogen atom energy, in which \(Z\) is the nuclear charge (+1 for hydrogen, +2 for He, +3 for Li, and so on) and \(k\) has a value of \(2.179 \times 10^{–18}\; J\).
\[ \color{red} E_n=−\dfrac{kZ^2}{n^2} \label{6.3.5}\]
The sizes of the circular orbits for hydrogen-like atoms are given in terms of their radii by the following expression, in which \(a_o\) is a constant called the Bohr radius, with a value of \(5.292 \times 10^{−11}\; m\):
\[ \color{red} r=\dfrac{n^2}{Z} a_0 \label{6.3.6}\]
The equation also shows us that as the electron’s energy increases (as \(n\) increases), the electron is found at greater distances from the nucleus. This is implied by the inverse dependence on \(r\) in the Coulomb potential, since, as the electron moves away from the nucleus, the electrostatic attraction between it and the nucleus decreases, and it is held less tightly in the atom. Note that as \(n\) gets larger and the orbits get larger, their energies get closer to zero, and so the limits \(n⟶∞\) and \(r⟶∞\) imply that \(E = 0\) corresponds to the ionization limit where the electron is completely removed from the nucleus. Thus, for hydrogen in the ground state \(n = 1\), the ionization energy would be:
\[ ΔE=E_{n⟶∞} −E_1=0+k=k \label{6.3.7}\]
With three extremely puzzling paradoxes now solved (blackbody radiation, the photoelectric effect, and the hydrogen atom), and all involving Planck’s constant in a fundamental manner, it became clear to most physicists at that time that the classical theories that worked so well in the macroscopic world were fundamentally flawed and could not be extended down into the microscopic domain of atoms and molecules. Unfortunately, despite Bohr’s remarkable achievement in deriving a theoretical expression for the Rydberg constant, he was unable to extend his theory to the next simplest atom, He, which only has two electrons. Bohr’s model was severely flawed, since it was still based on the classical mechanics notion of precise orbits, a concept that was later found to be untenable in the microscopic domain, when a proper model of quantum mechanics was developed to supersede classical mechanics.
Example \(\PageIndex{1}\): Calculating the Energy of an Electron in a Bohr Orbit
Early researchers were very excited when they were able to predict the energy of an electron at a particular distance from the nucleus in a hydrogen atom. If a spark promotes the electron in a hydrogen atom into an orbit with \(n = 3\), what is the calculated energy, in joules, of the electron?
Solution
The energy of the electron is given by Equation \(\ref{6.3.5}\):
\[ E=\dfrac{−kZ^2}{n^2}\]
The atomic number, \(Z\), of hydrogen is 1; \(k = 2.179 \times 10^{–18}\; J\); and the electron is characterized by an n value of \(3\). Thus,
\[E=\dfrac{−(2.179 \times 10^{−18}\;J)×(1)^2}{(3)^2}=−2.421 \times 10^{−19}\;J\]
Exercise \(\PageIndex{1}\)
The electron in Example \(\PageIndex{1}\) in the \(n=3\) state is promoted even further to an orbit with \(n = 6\). What is its new energy?
- Answer
-
TBD
Example \(\PageIndex{2}\): Calculating Electron Transitions in a One–electron System
What is the energy (in joules) and the wavelength (in meters) of the line in the spectrum of hydrogen that represents the movement of an electron from Bohr orbit with n = 4 to the orbit with n = 6? In what part of the electromagnetic spectrum do we find this radiation?
Solution
In this case, the electron starts out with \(n = 4\), so \(n_1 = 4\). It comes to rest in the \(n = 6\) orbit, so \(n_2 = 6\). The difference in energy between the two states is given by this expression:
\[ΔE=E_1−E_2=2.179 \times 10^{−18}\left(\dfrac{1}{n^2_1}−\dfrac{1}{n_2^2}\right)\]
\[ΔE=2.179 \times 10^{−18} \left(\dfrac{1}{4^2}−\dfrac{1}{6^2}\right)\; J\]
\[ΔE=2.179 \times 10^{−18} \left(\dfrac{1}{16}−\dfrac{1}{36}\right)\;J\]
\[ΔE=7.566 \times 10^{−20}\;J\]
This energy difference is positive, indicating a photon enters the system (is absorbed) to excite the electron from the n = 4 orbit up to the \(n = 6\) orbit. The wavelength of a photon with this energy is found by the expression \(E=hc \lambda\). Rearrangement gives:
\[ \lambda=\dfrac{hc}{E}\]
From the figure of electromagnetic radiation, we can see that this wavelength is found in the infrared portion of the electromagnetic spectrum.
Exercise \(\PageIndex{2}\)
What is the energy in joules and the wavelength in meters of the photon produced when an electron falls from the \(n = 5\) to the \(n = 3\) level in a \(He^+\) ion (\(Z = 2\) for \(He^+\))?
- Answer
-
\(6.198 \times 10^{–19}\; J\) and \(3.205 \times 10^{−7}\; m\)
Bohr’s model of the hydrogen atom provides insight into the behavior of matter at the microscopic level, but it is does not account for electron–electron interactions in atoms with more than one electron. It does introduce several important features of all models used to describe the distribution of electrons in an atom. These features include the following:
- The energies of electrons (energy levels) in an atom are quantized, described by quantum numbers: integer numbers having only specific allowed value and used to characterize the arrangement of electrons in an atom.
- An electron’s energy increases with increasing distance from the nucleus.
- The discrete energies (lines) in the spectra of the elements result from quantized electronic energies.
Of these features, the most important is the postulate of quantized energy levels for an electron in an atom. As a consequence, the model laid the foundation for the quantum mechanical model of the atom. Bohr won a Nobel Prize in Physics for his contributions to our understanding of the structure of atoms and how that is related to line spectra emissions.
Video \(\PageIndex{2}\): An overview of the Bohr Model of the Atom.
Bohr’s model explained the experimental data for the hydrogen atom and was widely accepted, but it also raised many questions. Why did electrons orbit at only fixed distances defined by a single quantum number n = 1, 2, 3, and so on, but never in between? Why did the model work so well describing hydrogen and one-electron ions, but could not correctly predict the emission spectrum for helium or any larger atoms? To answer these questions, scientists needed to completely revise the way they thought about matter.
The Quantum–Mechanical Model of an Atom
Shortly after de Broglie published his ideas that the electron in a hydrogen atom could be better thought of as being a circular standing wave instead of a particle moving in quantized circular orbits, as Bohr had argued, Erwin Schrödinger extended de Broglie’s work by incorporating the de Broglie relation into a wave equation, deriving what is today known as the Schrödinger equation. When Schrödinger applied his equation to hydrogen-like atoms, he was able to reproduce Bohr’s expression for the energy and, thus, the Rydberg formula governing hydrogen spectra, and he did so without having to invoke Bohr’s assumptions of stationary states and quantized orbits, angular momenta, and energies; quantization in Schrödinger’s theory was a natural consequence of the underlying mathematics of the wave equation. Like de Broglie, Schrödinger initially viewed the electron in hydrogen as being a physical wave instead of a particle, but where de Broglie thought of the electron in terms of circular stationary waves, Schrödinger properly thought in terms of three-dimensional stationary waves, or wavefunctions, represented by the Greek letter psi, ψ. A few years later, Max Born proposed an interpretation of the wavefunction ψ that is still accepted today: Electrons are still particles, and so the waves represented by ψ are not physical waves but, instead, are complex probability amplitudes. The square of the magnitude of a wavefunction \(∣ψ∣^2\)
\[\hat{H}ψ=Eψ\]
\(\hat{H}\) is the Hamiltonian operator, a set of mathematical operations representing the total energy of the quantum particle (such as an electron in an atom), ψ is the wavefunction of this particle that can be used to find the special distribution of the probability of finding the particle, and \(E\) is the actual value of the total energy of the particle.
Schrödinger’s work, as well as that of Heisenberg and many other scientists following in their footsteps, is generally referred to as quantum mechanics.
Video \(\PageIndex{3}\): You may also have heard of Schrödinger because of his famous thought experiment. This story explains the concepts of superposition and entanglement as related to a cat in a box with poison.
Video \(\PageIndex{4}\): What can we learn about Quantum Mechanics from the cat experiment?
Video \(\PageIndex{5}\): More on the thought experiment.
Key Equations
- \[ΔE=E_1−E_2=2.179 \times 10^{−18}\left(\dfrac{1}{n^2_1}−\dfrac{1}{n_2^2}\right)\]
Summary
Bohr incorporated Planck’s and Einstein’s quantization ideas into a model of the hydrogen atom that resolved the paradox of atom stability and discrete spectra. The Bohr model of the hydrogen atom explains the connection between the quantization of photons and the quantized emission from atoms. Bohr described the hydrogen atom in terms of an electron moving in a circular orbit about a nucleus. He postulated that the electron was restricted to certain orbits characterized by discrete energies. Transitions between these allowed orbits result in the absorption or emission of photons. When an electron moves from a higher-energy orbit to a more stable one, energy is emitted in the form of a photon. To move an electron from a stable orbit to a more excited one, a photon of energy must be absorbed. Using the Bohr model, we can calculate the energy of an electron and the radius of its orbit in any one-electron system.
Glossary
- Bohr’s model of the hydrogen atom
- structural model in which an electron moves around the nucleus only in circular orbits, each with a specific allowed radius; the orbiting electron does not normally emit electromagnetic radiation, but does so when changing from one orbit to another.
- excited state
- state having an energy greater than the ground-state energy
- ground state
- state in which the electrons in an atom, ion, or molecule have the lowest energy possible
- line spectrum
- electromagnetic radiation emitted at discrete wavelengths by a specific atom (or atoms) in an excited state
- quantum number
- integer number having only specific allowed values and used to characterize the arrangement of electrons in an atom
Contributors
Paul Flowers (University of North Carolina - Pembroke), Klaus Theopold (University of Delaware) and Richard Langley (Stephen F. Austin State University) with contributing authors. Textbook content produced by OpenStax College is licensed under a Creative Commons Attribution License 4.0 license. Download for free at http://cnx.org/contents/85abf193-2bd...a7ac8df6@9.110).
- Adelaide Clark, Oregon Institute of Technology
- Crash Course Physics: Crash Course is a division of Complexly and videos are free to stream for educational purposes.
- TED-Ed’s commitment to creating lessons worth sharing is an extension of TED’s mission of spreading great ideas. Within TED-Ed’s growing library of TED-Ed animations, you will find carefully curated educational videos, many of which represent collaborations between talented educators and animators nominated through the TED-Ed website.