13.7: The Born-Haber Cycle

We can measure the strength of a covalent bond, because we can usually break a covalently-bonded molecule into fragments and measure the energy required to do so. For example, if we want to measure the bond energy in HCl, we can carry out the reaction:

\(\ce{HCl(g) -> H(g) + Cl(g)}\)

However, we cannot measure the strength of an ionic bond this way, because ionic compounds do not break apart into gaseous ions:

NaCl(s) \(\ce{->}\) Na⁺(g) + Cl^–(g)

Born-Haber cycles are used to estimate the strength of “ionic bonds” in compounds such as NaCl.

An Application of Hess's Law

In a Born-Haber cycle, we carry out the following sequence of reactions:

Elements (in their standard states)

\(\ce{->}\)

Gaseous atoms

\(\ce{->}\)

Gaseous ions

\(\ce{->}\)

Compound

The energy of each step except the last one can be measured experimentally. In addition, we can measure the energy of the single-step reaction below (this is the “heat of formation” of the compound):

Elements (in their standard states) \(\ce{->}\) Compound

By Hess’s Law, the energies of the reactions in the first sequence must add up to the heat of formation (the energy of the single-step reaction).

The basic concept is not hard. The difficult is in keeping the details straight. Ionic compounds contain two (or more) elements, each of which must be converted to gaseous atoms and then to gaseous ions. For example, here are the steps required to convert elemental calcium (a solid at room temperature) to gaseous calcium ions:

Sublime the solid calcium (convert it to a gas): Ca(s) \(\ce{->}\) Ca(g)

Remove one electron from each atom: Ca(g) \(\ce{->}\) Ca⁺(g) + e^–

Remove a second electron from each atom: Ca⁺(g) \(\ce{->}\) Ca²⁺(g) + e^–

Nonmetals often form covalent molecules. If so, you must break the covalent bond as part of this process. Here are the steps required to convert elemental bromine (a diatomic liquid at room temperature) to gaseous bromide ions:

Vaporize the liquid bromine (convert it to a gas): Br₂(l) \(\ce{->}\) Br₂(g)

Break the covalent bond in Br₂: Br₂(g) \(\ce{->}\) 2 Br(g)

Add an electron to each atom: Br(g) + e^– \(\ce{->}\) Br^–(g)

Your job in sorting out a Born-Haber cycle has two parts. The first is to be able to figure out exactly what reactions must occur when you convert the original element to a monatomic gas. The second is to know how to identify the energy of each reaction type.

The reaction types that you might see in a Born-Haber cycle

1) Heat of sublimation (\( \ce{ \Delta H_{sub} } \)):this is the energy required to convert a solid to a gas. For metals and most solid nonmetals, sublimation produces a monatomic gas. Heats of sublimation are always positive numbers. For iodine (which is diatomic), sublimation produces I₂(g).

Na(s) \(\ce{->}\) Na(g) S(s) \(\ce{->}\) S(g) I₂(s) \(\ce{->}\) I₂(g)

2) Heat of vaporization (\( \ce{ \Delta H_{vap} } \)): this is the energy required to convert a liquid to a gas. Heats of vaporization are always positive numbers. The only elements for which this will come into play are bromine and mercury, which are liquids at room temperature.

Hg(l) \(\ce{->}\) Hg(g) Br₂(l) \(\ce{->}\) Br₂(g)

3) Bond dissociation energy (BDE, or \( \ce{ \Delta H_{BDE} } \)): this is the energy required to break a covalent bond. Bond dissociation energies are always positive numbers. Bond dissociation energies only come into play for the diatomic nonmetals (H₂, N₂, O₂, F₂, Cl₂, Br₂, I₂ and At₂). In these cases, sublimation or vaporization gives us diatomic molecules, not individual atoms, so we must also break the covalent bonds in the gaseous form.

H₂(g) \(\ce{->}\) 2 H(g) N₂(g) \(\ce{->}\) 2 N(g)

4) Ionization energy (IE, or \( \ce{ \Delta H_{IE} } \)): this is the energy required to remove one electron from a gaseous atom. Since many ionic compounds contain metals that have lost two or more electrons, we often need to consider two or more successive ionization energies. For example, if we need to make aluminum ions, we must remove three electrons from Al(g), so we must consider the first three ionization energies of aluminum:

Al(g) \(\ce{->}\) Al⁺(g) + e^– \( \ce{ \Delta H_{IE1} } \) = “first ionization energy” (IE1)

Al⁺(g) \(\ce{->}\) Al²⁺(g) + e^– \( \ce{ \Delta H_{IE2} } \) = “second ionization energy” (IE2)

Al²⁺(g) \(\ce{->}\) Al³⁺(g) + e^– \( \ce{ \Delta H_{IE3} } \) = “third ionization energy” (IE3)

Ionization energies are always positive numbers, and they increase as you remove more electrons (so IE1 < IE2 < IE3 for any given element).

5) Electron affinity (EA, or \( \ce{ \Delta H_{EA} } \)): this is the energy absorbed or released when you add one electron to a gaseous atom. In general, only the first electron affinity can be measured directly, because negative ions repel electrons. However, the second (and third, if necessary) electron affinity can be estimated using a variation on the Born-Haber cycle. Here are the reactions that must be considered if you need to make oxide ions.

O(g) + e^– \(\ce{->}\) O^–(g) \( \ce{ \Delta H_{EA1} } \) = “first electron affinity” (EA1, or simply EA)

O^–(g) + e^– \(\ce{->}\) O^2–(g) \( \ce{ \Delta H_{EA2} } \) = “second electron affinity” (EA2)

The first electron affinity is usually a negative number (a few elements have positive EA’s). The second EA (and beyond) is always positive.

6) Solution energy (\( \ce{ \Delta H_{soln} } \)): this is the energy released or absorbed for the solution process of an ionic compound. Here is the reaction that must be considered for the solution of AlF₃(s).

AlF₃(s) \(\ce{->}\) Al³⁺(aq) + 3 F^–(aq)

7) Hydration energy (HE, or \( \ce{ \Delta H_{HE} } \)): this is the energy released for the solution of gaseous ions of a compound. The hydration energy is a measure of all the ion-dipole attractions between the ions on the water solvent. Here is the reaction that must be considered for the hydration of the ions of AlF₃(s).

Al³⁺(g) + 3 F^–(g) \(\ce{->}\) Al³⁺(aq) + 3 F^–(aq)

8) Crystal lattice energy (CLE, or \( \ce{ \Delta H_{CLE} } \)): this is the energy released when gaseous ions are converted into the solid ionic compound. For example, the lattice energy of aluminum fluoride corresponds to the following reaction:

Al³⁺(g) + 3 F^–(g) \(\ce{->}\) AlF₃(s)

Lattice energies are always large negative numbers, ranging from around –600 to –13,000 kJ/mol.

9) Heat of formation (\( \ce{ \Delta H_{f} } \))): this is the energy absorbed or released when you make an ionic compound from its constituent elements (as they normally appear at room temperature and 1 atm pressure). For aluminum fluoride, the corresponding reaction is:

Al(s) + ³/₂ F₂(g) \(\ce{->}\) AlF₃(s)

Heats of formation are virtually always negative numbers for ionic compounds.

Putting the reactions together:

Here is an example of how these reactions can be fitted together to make the complete Born-Haber cycle for LiF. We start with solid Li and gaseous F₂, the standard states of these elements at room temperature and 1 atm pressure.

Energy “bookkeeping”: \( \ce{ \Delta H_{sub} } \) + IE1 + ¹/₂·BDE + EA + CLE = \( \ce{ \Delta H_{f} } \)

For CaI₂, the energy bookkeeping would look like this (see if you can figure out why). Note that there are two heats of sublimation involved, because both calcium and iodine are solids at room temperature.

\( \ce{ \Delta H_{sub} } \)(Ca) + IE1 + IE2 + BDE + \( \ce{ \Delta H_{sub} } \)(I₂) + 2·EA + CLE = \( \ce{ \Delta H_{f} } \)

For Al₂O₃, the energy bookkeeping would look like this. Again, see if you can figure out why. Note that in this case, we must include two electron affinities for oxygen, because the charge on oxygen is –2 in this compound.

2·\( \ce{ \Delta H_{sub} } \) + 2·IE1 + 2·IE2 + 2·IE3 + ³/₂·BDE + 3·EA1 + 3·EA2 + CLE = \( \ce{ \Delta H_{f} } \)

These problems can also be approached in terms of an energy diagram (as shown in the following example problem).

Example \(\PageIndex{1}\)

Given the partial Born-Haber energy level diagram below, along with the ∆H values provided, answer the questions that follow.

a. Determine ∆H_sublimation [Fe]

b. Determine ∆H_CLE [Fe₂O₃]

c. Determine ∆H_EA1 [O(g)]

d. Determine ∆H_EA2 [O(g)]

e. What is the value for the sum (∆H_IE1 [Fe(g)] + ∆H_IE2 [Fe(g)] + ∆H_IE3 [Fe(g)])?

Solution

To solve this problem, we can begin by using the energies given below the diagram to draw a couple of additional energy levels….

• In any Born-Haber diagram, the energy of the elements in their standard states (Fe(s) + ³/₂ O₂(g) in this case) is zero.
• The ∆H_f of Fe₂O₃(s) is -826 kJ/mol, so the energy of Fe₂O₃(s) is -826 kJ.
• The bond dissociation energy of O₂(g) is 495 kJ/mol, so converting ³/₂ of a mole of O₂(g) into 3 moles of O(g) requires ³/₂(495 kJ) = 742.5 kJ. On the diagram in the problem, the lowest level that contains 3 O(g) is the one at the bottom (labeled 2 Fe(g) + 3 O(g)). Therefore, the level corresponding to 2 Fe(g) + ³/₂ O₂(g) must be 742.5 kJ below this. 1537.5 kJ – 742.5 kJ = 795 kJ.

Below, we’ve redrawn the Born-Haber diagram with these additional energy values.

Now we can use this diagram to answer the questions. For each question, the following diagram shows the relevant ∆H value. The substances that change are shown in red, and substances that are spectators during the reaction are shown in green.

a) Comparing the levels at E = 0 and E = 795 kJ, we see that the only change is 2 Fe(s) -> 2 Fe(g). This is the reaction for the sublimation of Fe(s). Therefore, ∆H = 795 kJ – 0 kJ = 795 kJ for this reaction. Since this energy corresponds to the sublimation of 2 moles of Fe, the enthalpy of sublimation is 795 kJ/2 mol = 397.5 kJ/mol.

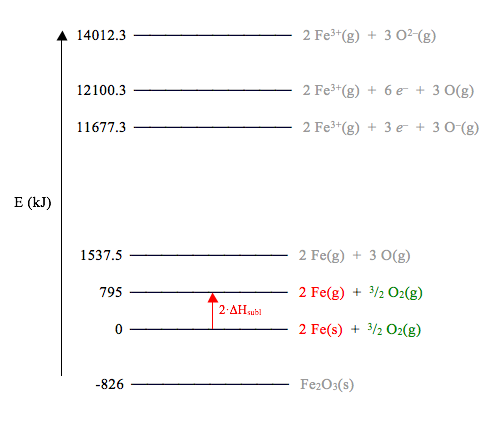
b) The crystal lattice energy is the energy change on going from the gaseous ions to the solid compound: 2 Fe³⁺(g) + 3 O^2–(g) -> Fe₂O₃(s). From the diagram, we can calculate this energy change: ∆H = (-826 kJ) – 14012.3 kJ = -14838.3 kJ. Since this corresponds to the formation of one mole of Fe₂O₃, the crystal lattice energy is -14838.3 kJ/mol.

c) EA1 for oxygen is the energy change for the reaction O(g) -> O^–(g). Looking at the diagram, we see that going from the 12100.3 kJ level to the 11677.3 kJ level corresponds to the reaction 3 O(g) -> 3 O^–(g), which is the right reaction, just multiplied by 3. (The iron remains in the same state, so it is a spectator here. Therefore, ∆H = 11677.3 kJ – 12100.3 kJ = -423 kJ for this reaction. Since this energy corresponds to the ionization of three moles of O, the EA1 is -423 kJ/3 mol = -141 kJ/mol.

d) EA2 for oxygen is the energy change for the reaction O^–(g) -> O^2–(g). Looking at the diagram, we see that going from the 11677.3 kJ level to the 14012.3 kJ level corresponds to the reaction 3 O^–(g) -> 3 O^2–(g), which is the right reaction, just multiplied by 3. (Again, the iron remains in the same state.) Therefore, ∆H = 14012.3 kJ - 11677.3 kJ = 2335 kJ for this reaction. Since this energy corresponds to the ionization of three moles of O, the EA2 is 2335 kJ/3 mol = 778.3 kJ/mol.

e) The sum of the three IE’s for iron is the total energy required to convert a mole of Fe(g) into a mole of Fe³⁺(g), corresponding to the reaction Fe(g) ® Fe³⁺(g) + 3 e^–. Looking at the diagram, we see that going from the 1537.5 kJ level to the 12100.3 kJ level corresponds to the reaction 2 Fe(g) ® 2 Fe³⁺(g) + 6 e^–, which is the right reaction, just multiplied by 2. Note that we had to go to the 12100.3 kJ level in order to keep the oxygen in the same state, 3 O(g). Therefore, ∆H = 12100.3 kJ – 1537.5 kJ = 10562.8 kJ for this reaction. Since this energy corresponds to the ionization of two moles of Fe, the correct answer is 10562.8 kJ/2 mol = 5281.4 kJ/mol.