6.2: Intermolecular Forces in Action
- Page ID
- 221368
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)
Boiling points and melting point in covalent compounds
As described previously, whether a covalent substance is a solid, a liquid, or a gas at room temperature depends on the relative strength of its intermolecular forces (IMFs) compared to the kinetic energies (KE) of its molecules at room temperature. When the IMFs are stronger than the KE, the molecules tend to be close together, with very restricted ability to move, then the substance is likely to be a solid. When the IMF and the KE are similar in magnitude, the molecules can move, bet their movement is limited to short distances, so the covalent compound is likely to be liquid. When the IMFs are very weak compared to the KE, the attraction between molecules is minimal, so the molecules can move freely. Therefore the substance is most likely to be a gas.
Figure \(\PageIndex{1}\): In a gas, the kinetic energy of the molecules is higher than the intermolecular forces. In a liquid, intermolecular forces and kinetic energy have comparable values. In a solid, intermolecular forces are stronger than the kinetic energy of the molecules. Image by Yelod - Wikimedia Commons * Yelod - Wikipedia (En) * ילוד - ויקיפדיה העברית, CC BY-SA 3.0, via Wikimedia Commons
Since the kinetic energy of the molecules increases with the temperature, if a covalent compound is in solid state and its temperature is increased gradually, there will be a temperature value at which the molecular kinetic energy will overcome the intermolecular forces, and the solid will melt into a liquid. This temperature is known as the melting point. Similarly, is the liquid is further heated, there will be a temperature value at which the molecular kinetic energy will be much higher than the intermolecular forces and the liquid will vaporize into a gas. This temperature is known as the boiling point. We can predict that the melting point and boiling point values for a given substance depend on the intensity of its intermolecular forces: the stronger the intermolecular forces, the higher the melting point and boiling point values.
In the case of ionic compounds, metallic substances, and network covalent solids, the melting and boiling processes involve the separation of particles within crystal structures, and therefore, chemical bonds must be broken. As a consequence, ionic compounds, metallic substances, and network covalent solid substances always exhibit very high melting and boiling points values compared to covalent compounds. This is because the melting and boiling processes for covalent compounds do not involve breaking the covalent bond, but rather separating the molecules by overcoming the acting intermolecular forces.
Table \(\PageIndex{1}\) shows the boiling point and melting for some substances and the forces that must be overcome in each case.
Table \(\PageIndex{1}\). Boiling points and melting points compared to intermolecular forces |
|||
| Substance | Melting point (ºC) | Boiling point (ºC) | Forces that must be overcome |
| molecular oxygen O2 | −218 | −183 | London dispersion forces |
| hydrogen sulfide, H2S | -82 | -60 | Dipole-Dipole |
| water, H2O | 0 | 100 | Hydrogen bonding |
| metallic aluminum, Al | 660 | 2743 | metallic bond |
| Sodium Chloride, NaCl | 800 | 1465 | ionic bond |
| Carbon in diamond, C | 4027 | 4830 | covalent bonds in a network covalent solid |
We can see that breaking chemical bonds requires much more energy than overcoming intermolecular forces. There a few places in the universe in which the temperature is high enough to have ionic or metallic compounds in gas or liquid phase. Some examples, the magma produced by volcanos have a high content of Fe2O3 in a liquid state, while the sun contains some metals in gas state (iron, magnesium, etc)
Figure \(\PageIndex{2}\). Volcano in Hawaii producing lava. Lava contains a high proportion of melted ionic compounds such as Fe2O3, Al2O3, and FeO. Image by Hawaii Volcano Observatory (DAS), Public domain, via Wikimedia Commons
Solubility
The ability of a given substance to dissolve in a given solvent depends on the strength of the intermolecular forces that can act between solute and solvent molecules. In general, polar substances will dissolve in polar solvents, while non-polar substances will dissolve in non-polar solvents. This is because non-polar substances can interact very well through London Dispersion forces, while polar substances can interact well through dipole-dipole or hydrogen bonding interactions. Therefore, the general rule is "like dissolves like".
Table \(\PageIndex{2}\) shows the solubility values for a series of substances in water, a polar solvent
Table \(\PageIndex{2}\) shows the solubility values for a series of substances in water at 25ºC and 1 atm |
||
| Substance | Solubility in H2O (g/100 mL, at 25ºC) | Acting intermolecular forces |
| Oxygen, O2 | 0.0041 | LDF |
| Sulfur dioxide, SO2 | 9.4 | Dipole-Dipole |
| Ammonia, NH3 | 31 | Hydrogen bonding |
| Sodium chloride, NaCl | 36 | Ion-Dipole |
As we can see, the solubility values increase when the intermolecular interactions between the solvent and solute molecule become stronger. Switching the solvent into a non-polar one, such as carbon tetrachloride (CCl4), carbon disulfide (CS2) or hexane (C6H6) will certainly show the opposite trend in solubility for ten above listed substances.
Viscosity
The viscosity of a liquid is a measure of its resistance to flow. It is similar to the concept of "thickness". For example, maple syrup has a higher viscosity than sweet tea. Viscosity can be measured in units of millipascal-second (mPa·s). As the intensity of intermolecular forces increases, so does the viscosity. Table \(\PageIndex{3}\) shows the viscosity values for a series of liquid substances.
Table \(\PageIndex{3}\): viscosity values for a series of liquid substances at 25ºC |
||
| Substance | Viscosity (mPa·s) at 25ºC | Intermolecular forces |
| Benzene, C6H6 | 0.604 | LDF |
| Water, H2O | 0.89 | Hydrogen bonding |
| Mercury, Hg | 1.526 | Metallic bond |
Surface Tension
The next time you are by a still body of water, take a close look at what is scooting along on the surface. You may see insects seemingly floating on top of the water. These creatures are known by a variety of names including water skaters, water striders, pond skaters, and other equally descriptive names. They take advantage of a property called surface tension to stay above the water and not sink. The force they exert downward is less than the forces exerted among the water molecules on the surface of the pond, so the insect does not penetrate beneath the surface of the water.
Molecules within a liquid are pulled equally in all directions by intermolecular forces. However, molecules at the surface are pulled downwards and sideways by other liquid molecules, but not upwards away from the surface. The overall effect is that the surface molecules are pulled into the liquid, creating a surface that is tightened like a film (Figure \(\PageIndex{3A}\)). The surface tension of a liquid is a measure of the elastic force in the liquid's surface. Liquids that have strong intermolecular forces, like the hydrogen bonding in water, exhibit the greatest surface tension. Surface tension allows objects that are denser than water, such as the paper clip shown in B in the figure below, to nonetheless float on its surface. It is also responsible for the beading up of water droplets on a freshly waxed car because there are no attractions between the polar water molecules and the nonpolar wax.
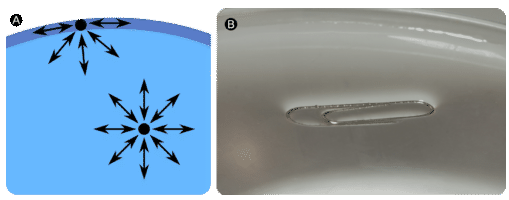
Other liquids, such as diethyl ether, do not demonstrate strong surface tension interactions. The intermolecular forces for the ether are the relatively weak dipole-dipole interactions that do not draw the molecules together as tightly as hydrogen bonds would.
Summary
- The surface tension of a liquid is a measure of the elastic force in the liquid's surface.
- Liquids with strong intermolecular forces have higher surface tensions than liquids with weaker forces.
Contributions & Attributions
Solubility and Structure. (2019, June 5). Retrieved May 19, 2021, from https://chem.libretexts.org/@go/page/42100
Wikipedia contributors. (2021, May 5). Viscosity. In Wikipedia, The Free Encyclopedia. Retrieved 19:41, May 19, 2021, from https://en.wikipedia.org/w/index.php?title=Viscosity&oldid=1021646585
This page was constructed from content via the following contributor(s) and edited (topically or extensively) by the LibreTexts development team to meet platform style, presentation, and quality:
Henry Agnew (UC Davis)



