Redox Titration
- Page ID
- 221875
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Analytical titrations using redox reactions were introduced shortly after the development of acid–base titrimetry. The earliest Redox titration took advantage of the oxidizing power of chlorine. In 1787, Claude Berthollet introduced a method for the quantitative analysis of chlorine water (a mixture of Cl2, HCl, and HOCl) based on its ability to oxidize indigo, a dye that is colorless in its oxidized state. In 1814, Joseph Gay-Lussac developed a similar method for determining chlorine in bleaching powder. In both methods the end point is a change in color. Before the equivalence point the solution is colorless due to the oxidation of indigo. After the equivalence point, however, unreacted indigo imparts a permanent color to the solution.
The number of redox titrimetric methods increased in the mid-1800s with the introduction of MnO4–, Cr2O72–, and I2 as oxidizing titrants, and of Fe2+ and S2O32– as reducing titrants. Even with the availability of these new titrants, redox titrimetry was slow to develop due to the lack of suitable indicators. A titrant can serve as its own indicator if its oxidized and reduced forms differ significantly in color. For example, the intensely purple MnO4– ion serves as its own indicator since its reduced form, Mn2+, is almost colorless. Other titrants require a separate indicator. The first such indicator, diphenylamine, was introduced in the 1920s. Other redox indicators soon followed, increasing the applicability of redox titrimetry.
Redox Titration Curves
To evaluate a redox titration we need to know the shape of its titration curve. In an acid–base titration or a complexation titration, the titration curve shows how the concentration of H3O+ (as pH) or Mn+ (as pM) changes as we add titrant. For a redox titration it is convenient to monitor the titration reaction’s potential instead of the concentration of one species.
You may recall from Chapter 6 that the Nernst equation relates a solution’s potential to the concentrations of reactants and products participating in the redox reaction. Consider, for example, a titration in which a titrand in a reduced state, Ared, reacts with a titrant in an oxidized state, Box.
\[A_\textrm{red}+B_\textrm{ox} \rightleftharpoons B_\textrm{red}+A_\textrm{ox}\]
where Aox is the titrand’s oxidized form, and Bred is the titrant’s reduced form. The reaction’s potential, Erxn, is the difference between the reduction potentials for each half-reaction.
\[E_\textrm{rxn}=E_{B_\mathrm{\Large ox}/B_\mathrm{\Large red}}-E_{A_\mathrm{\Large ox}/A_\mathrm{\Large red}}\]
After each addition of titrant the reaction between the titrand and the titrant reaches a state of equilibrium. Because the potential at equilibrium is zero, the titrand’s and the titrant’s reduction potentials are identical.
\[E_{B_\mathrm{\Large ox}/B_\mathrm{\Large red}}=E_{A_\mathrm{\Large ox}/A_\mathrm{\Large red}}\]
This is an important observation because we can use either half-reaction to monitor the titration’s progress.
Before the equivalence point the titration mixture consists of appreciable quantities of the titrand’s oxidized and reduced forms. The concentration of unreacted titrant, however, is very small. The potential, therefore, is easier to calculate if we use the Nernst equation for the titrand’s half-reaction
\[E_\textrm{rxn}= E^o_{A_\mathrm{\Large ox}/A_\mathrm{\Large red}}-\dfrac{RT}{nF}\ln\dfrac{[A_\textrm{red}]}{[A_\textrm{ox}]}\]
Although the Nernst equation is written in terms of the half-reaction’s standard state potential, a matrix-dependent formal potential often is used in its place. See Appendix 13 for the standard state potentials and formal potentials for selected half-reactions.
After the equivalence point it is easier to calculate the potential using the Nernst equation for the titrant’s half-reaction.
\[E_\textrm{rxn}= E^o_{B_\mathrm{\Large ox}/B_\mathrm{\Large red}}-\dfrac{RT}{nF}\ln\dfrac{[B_\textrm{red}]}{[B_\textrm{ox}]}\]
Calculating the Titration Curve
Let’s calculate the titration curve for the titration of 50.0 mL of 0.100 M Fe2+ with 0.100 M Ce4+ in a matrix of 1 M HClO4. The reaction in this case is
\[\textrm{Fe}^{2+}(aq)+\textrm{Ce}^{4+}(aq)\rightleftharpoons \textrm{Ce}^{3+}(aq)+\textrm{Fe}^{3+}(aq)\tag{9.15}\]
In 1 M HClO4, the formal potential for the reduction of Fe3+ to Fe2+ is +0.767 V, and the formal potential for the reduction of Ce4+ to Ce3+ is +1.70 V.
Because the equilibrium constant for reaction 9.15 is very large—it is approximately 6 × 1015—we may assume that the analyte and titrant react completely.
Step 1
Calculate the volume of titrant needed to reach the equivalence point.
The first task is to calculate the volume of Ce4+ needed to reach the titration’s equivalence point. From the reaction’s stoichiometry we know that
\[\textrm{moles Fe}^{2+}=\textrm{moles Ce}^{4+}\]
\[M_\textrm{Fe}\times V_\textrm{Fe} = M_\textrm{Ce}\times V_\textrm{Ce}\]
Solving for the volume of Ce4+ gives the equivalence point volume as
\[V_\textrm{eq} = V_\textrm{Ce} = \dfrac{M_\textrm{Fe}V_\textrm{Fe}}{M_\textrm{Ce}}=\dfrac{\textrm{(0.100 M)(50.0 mL)}}{\textrm{(0.100 M)}}=\textrm{50.0 mL}\]
Step 2:
alculate the potential before the equivalence point by determining the concentrations of the titrand’s oxidized and reduced forms, and using the Nernst equation for the titrand’s reduction half-reaction.
Before the equivalence point, the concentration of unreacted Fe2+ and the concentration of Fe3+ are easy to calculate. For this reason we find the potential using the Nernst equation for the Fe3+/Fe2+ half-reaction.
\[E = E^o_\mathrm{\large Fe^{3+}/Fe^{2+}} - \dfrac{RT}{nF}\log\dfrac{[\mathrm{Fe^{2+}}]}{[\mathrm{Fe^{3+}}]}=+0.767\textrm V - 0.05916\log\dfrac{[\mathrm{Fe^{2+}}]}{[\mathrm{Fe^{3+}}]}\tag{9.16}\]
For example, the concentrations of Fe2+ and Fe3+ after adding 10.0 mL of titrant are
\[\begin{align}
[\textrm{Fe}^{2+}]&=\dfrac{\textrm{initial moles Fe}^{2+} - \textrm{moles Ce}^{4+}\textrm{ added}}{\textrm{total volume}}=\dfrac{M_\textrm{Fe}V_\textrm{Fe} - M_\textrm{Ce}V_\textrm{Ce}}{V_\textrm{Fe}+V_\textrm{Ce}}\\
&\mathrm{= \dfrac{(0.100\;M)(50.0\;mL)-(0.100\;M)(10.0\;mL)}{50.0\;mL+10.0\;mL} = 6.67\times10^{-2}\;M}
\end{align}\]
\[\begin{align}
[\mathrm{Fe^{3+}}]&=\mathrm{\dfrac{moles\;Ce^{4+}\;added}{total\;volume}}=\dfrac{M_\textrm{Ce}V_\textrm{Ce}}{V_\textrm{Fe} + V_\textrm{Ce}}\\
&=\dfrac{\textrm{(0.100 M)(10.0 mL)}}{\textrm{50.0 mL + 10.0 mL}}=1.67\times10^{-2}\textrm{ M}
\end{align}\]
\[E = +0.767\textrm{ V} - 0.05916 \log\dfrac{6.67\times10^{-2}\textrm{ M}}{1.67\times10^{-2}\textrm{ M}}=+0.731\textrm{ V}\]
Step 3:
Calculate the potential after the equivalence point by determining the concentrations of the titrant’s oxidized and reduced forms, and using the Nernst equation for the titrant’s reduction half-reaction.
After the equivalence point, the concentration of Ce3+ and the concentration of excess Ce4+ are easy to calculate. For this reason we find the potential using the Nernst equation for the Ce4+/Ce3+ half-reaction.
\[E=E^o_\mathrm{\large{Ce^{4+}/Ce^{3+}}}-\dfrac{RT}{nF}\log\mathrm{\dfrac{[Ce^{3+}]}{[Ce^{4+}]}}=+ 1.70\textrm{ V} - 0.05916 \log\mathrm{\dfrac{[Ce^{3+}]}{[Ce^{4+}]}}\tag{9.17}\]
\[\begin{align}
[\textrm{Ce}^{3+}]&={\dfrac{\textrm{initial moles Fe}^{2+}}{\textrm{total volume}}}=\dfrac{M_\textrm{Fe}V_\textrm{Fe}}{V_\textrm{Fe}+V_\textrm{Ce}}\\
&=\dfrac{\textrm{(0.100 M)(50.0 mL)}}{\textrm{50.0 mL + 60.0 mL}}=4.55\times10^{-3}\textrm{ M}
\end{align}\]
\[\begin{align}
[\textrm{Ce}^{4+}]&=\dfrac{\textrm{moles Ce}^{4+}\textrm{ added} - \textrm{initial moles Fe}^{2+}}{\textrm{total volume}}=\dfrac{M_\textrm{Ce}V_\textrm{Ce}-M_\textrm{Fe}V_\textrm{Fe}}{V_\textrm{Fe}+V_\textrm{Ce}}\\
&=\dfrac{\textrm{(0.100 M)(60.0 mL)}-\textrm{(0.100 M)(50.0 mL)}}{\textrm{50.0 mL + 60.0 mL}}=9.09\times10^{-3}\textrm{ M}
\end{align}\]
\[E=+1.70\textrm{ V}-0.05916\log\dfrac{4.55\times10^{-2}\textrm{ M}}{9.09\times10^{-3}\textrm{ M}}=+1.66\textrm{ V}\]
Step 4
Calculate the potential at the equivalence point.
At the titration’s equivalence point, the potential, Eeq, in equation 9.16 and equation 9.17 are identical. Adding the equations together to gives
\[2E_\textrm{eq}= E^o_\mathrm{\large Fe^{3+}/Fe^{2+}}+E^o_\mathrm{\large Ce^{4+}/Ce^{3+}}-0.05916\log\dfrac{\mathrm{[{Fe}^{2+}][Ce^{3+}]}}{\mathrm{[Fe^{3+}][Ce^{4+}]}}\]
Because [Fe2+] = [Ce4+] and [Ce3+] = [Fe3+] at the equivalence point, the log term has a value of zero and the equivalence point’s potential is
\[E_\textrm{eq}=\dfrac{E^o_\mathrm{\large Fe^{3+}/Fe^{2+}} + E^o_\mathrm{\large Ce^{4+}/Ce^{3+}}}{2}=\dfrac{\textrm{0.767 V + 1.70 V}}{2}=1.23\textrm{ V}\]
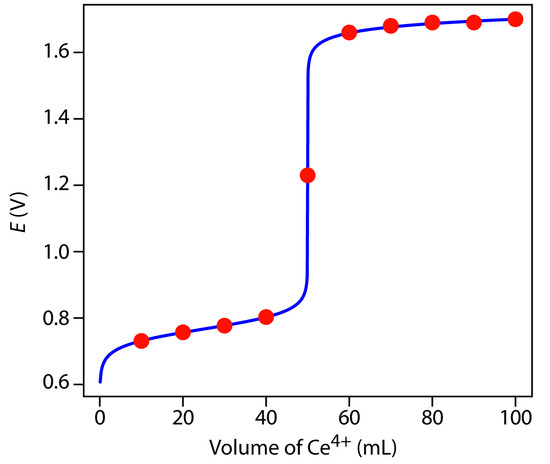
Additional results for this titration curve are shown in Table 9.15 and Figure 9.36.
| Volume of Ce4+ (mL) | E (V) | Volume Ce4+ (mL) | E (V) |
|---|---|---|---|
| 10.0 | 0.731 | 60.0 | 1.66 |
| 20.0 | 0.757 | 70.0 | 1.68 |
| 30.0 | 0.777 | 80.0 | 1.69 |
| 40.0 | 0.803 | 90.0 | 1.69 |
| 50.0 | 1.23 | 100.0 | 1.70 |
Figure 9.36 Titration curve for the titration of 50.0 mL of 0.100 M Fe2+ with 0.100 M Ce4+. The red points correspond to the data in Table 9.15. The blue line shows the complete titration curve.
Exercise \(\PageIndex{1}\)
Calculate the titration curve for the titration of 50.0 mL of 0.0500 M Sn2+ with 0.100 M Tl3+. Both the titrand and the titrant are 1.0 M in HCl. The titration reaction is
\[\textrm{Sn}^{2+}(aq)+\textrm{Tl}^{3+}(aq)\rightarrow \textrm{Sn}^{4+}(aq)+\textrm{Tl}^+(aq)\]
Click here to review your answer to this exercise.
Sketching a Redox Titration Curve
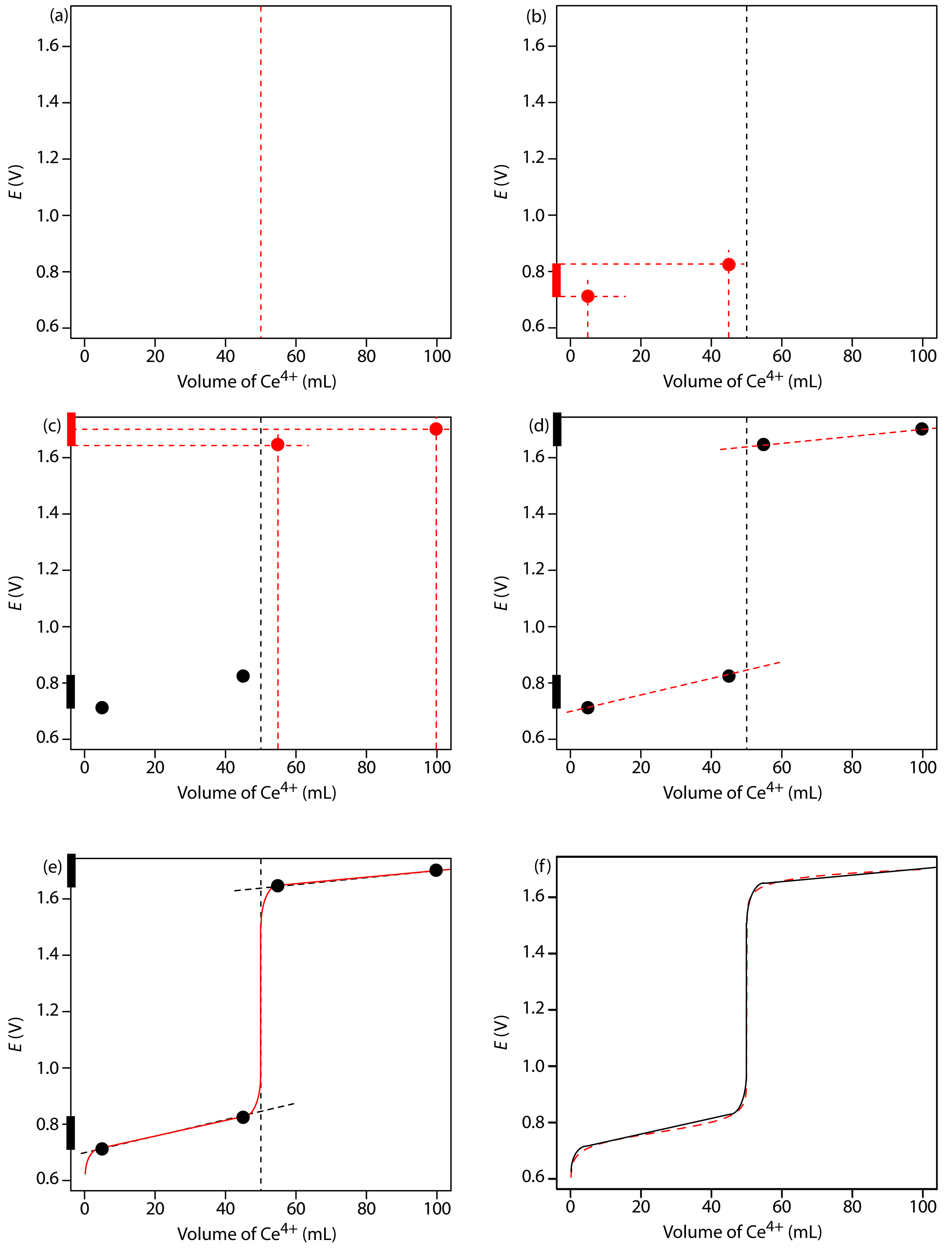
To evaluate the relationship between a titration’s equivalence point and its end point we need to construct only a reasonable approximation of the exact titration curve. In this section we demonstrate a simple method for sketching a redox titration curve. Our goal is to sketch the titration curve quickly, using as few calculations as possible. Let’s use the titration of 50.0 mL of 0.100 M Fe2+ with 0.100 M Ce4+ in a matrix of 1 M HClO4.
This is the same example that we used in developing the calculations for a redox titration curve. You can review the results of that calculation in Table 9.15 and Figure 9.36.
We begin by calculating the titration’s equivalence point volume, which, as we determined earlier, is 50.0 mL. Next, we draw our axes, placing the potential, E, on the y-axis and the titrant’s volume on the x-axis. To indicate the equivalence point’s volume, we draw a vertical line corresponding to 50.0 mL of Ce4+. Figure 9.37a shows the result of the first step in our sketch.
Before the equivalence point, the potential is determined by a redox buffer of Fe2+ and Fe3+. Although we can easily calculate the potential using the Nernst equation, we can avoid this calculation by making a simple assumption. You may recall from Chapter 6 that a redox buffer operates over a range of potentials that extends approximately ±(0.05916/n) unit on either side of EoFe3+/Fe2+. The potential is at the buffer’s lower limit
\[\textrm E=E^o_\mathrm{\large Fe^{3+}/Fe^{2+}}-0.05916\]
when the concentration of Fe2+ is 10× greater than that of Fe3+. The buffer reaches its upper potential
\[\textrm E=E^o_\mathrm{\large Fe^{3+}/Fe^{2+}}+0.05916\]
when the concentration of Fe2+ is 10× smaller than that of Fe3+. The redox buffer spans a range of volumes from approximately 10% of the equivalence point volume to approximately 90% of the equivalence point volume.
Figure 9.37b shows the second step in our sketch. First, we superimpose a ladder diagram for Fe2+ on the y-axis, using its EoFe3+/Fe2+ value of 0.767 V and including the buffer’s range of potentials. Next, we add points representing the pH at 10% of the equivalence point volume (a potential of 0.708 V at 5.0 mL) and at 90% of the equivalence point volume (a potential of 0.826 V at 45.0 mL).
We used a similar approach when sketching the acid–base titration curve for the titration of acetic acid with NaOH.
The third step in sketching our titration curve is to add two points after the equivalence point. Here the potential is controlled by a redox buffer of Ce3+ and Ce4+. The redox buffer is at its lower limit of E = EoCe4+/Ce3+ – 0.05916 when the titrant reaches 110% of the equivalence point volume and the potential is EoCe4+/Ce3+ when the volume of Ce4+ is 2×Veq.
Figure 9.37c shows the third step in our sketch. First, we add a ladder diagram for Ce4+, including its buffer range, using its EoCe4+/Ce3+ value of 1.70 V. Next, we add points representing the potential at 110% of Veq (a value of 1.66 V at 55.0 mL) and at 200% of Veq (a value of 1.70 V at 100.0 mL).
We used a similar approach when sketching the complexation titration curve for the titration of Mg2+ with EDTA.
Next, we draw a straight line through each pair of points, extending the line through the vertical line representing the equivalence point’s volume (Figure 9.37d). Finally, we complete our sketch by drawing a smooth curve that connects the three straight-line segments (Figure 9.37e). A comparison of our sketch to the exact titration curve (Figure 9.37f) shows that they are in close agreement.
Figure 9.37: Illustrations showing the steps in sketching an approximate titration curve for the titration of 50.0 mL of 0.100 M Fe2+ with 0.100 M Ce4+ in 1 M HClO4: (a) locating the equivalence point volume; (b) plotting two points before the equivalence point; (c) plotting two points after the equivalence point; (d) preliminary approximation of titration curve using straight-lines; (e) final approximation of titration curve using a smooth curve; (f) comparison of approximate titration curve (solid black line) and exact titration curve (dashed red line). See the text for additional details.
Exercise \(\PageIndex{2}\)
Sketch the titration curve for the titration of 50.0 mL of 0.0500 M Sn4+ with 0.100 M Tl+. Both the titrand and the titrant are 1.0 M in HCl. The titration reaction is
\[\textrm{Sn}^{2+}(aq)+\textrm{Tl}^{3+}(aq)\rightarrow\textrm{Sn}^{4+}(aq)+\textrm{Tl}^+(aq)\]
Compare your sketch to your calculated titration curve from Practice Exercise 9.17.
Click here to review your answer to this exercise.
9.4.2 Selecting and Evaluating the End point
A redox titration’s equivalence point occurs when we react stoichiometrically equivalent amounts of titrand and titrant. As is the case with acid–base and complexation titrations, we estimate the equivalence point of a complexation titration using an experimental end point. A variety of methods are available for locating the end point, including indicators and sensors that respond to a change in the solution conditions.
Where is the Equivalence Point?
For an acid–base titration or a complexometric titration the equivalence point is almost identical to the inflection point on the steeping rising part of the titration curve. If you look back at Figure 9.7 and Figure 9.28, you will see that the inflection point is in the middle of this steep rise in the titration curve, which makes it relatively easy to find the equivalence point when you sketch these titration curves. We call this a symmetric equivalence point. If the stoichiometry of a redox titration is symmetric—one mole of titrant reacts with each mole of titrand—then the equivalence point is symmetric. If the titration reaction’s stoichiometry is not 1:1, then the equivalence point is closer to the top or to bottom of the titration curve’s sharp rise. In this case we have an asymmetric equivalence point.
Example \(\PageIndex{1}\)
Derive a general equation for the equivalence point’s potential when titrating Fe2+ with MnO4–.
\[5\textrm{Fe}^{2+}(aq)+\textrm{MnO}_4^-(aq)+8\textrm H^+(aq)\rightarrow 5\textrm{Fe}^{3+}(aq)+\textrm{Mn}^{2+}(aq)+\mathrm{4H_2O}\]
(We often use H+ instead of H3O+ when writing a redox reaction.)
Solution
The half-reactions for Fe2+ and MnO4– are
\[\textrm{Fe}^{2+}(aq)\rightarrow\textrm{Fe}^{3+}(aq)+e^-\]
\[\textrm{MnO}_4^-(aq)+8\textrm H^+(aq)+5e^-\rightarrow \textrm{Mn}^{2+}(aq)+4\mathrm{H_2O}(l)\]
for which the Nernst equations are
\[E=E^o_\mathrm{\large Fe^{3+}/Fe^{2+}}-0.05916\log\dfrac{[\textrm{Fe}^{2+}]}{[\textrm{Fe}^{3+}]}\]
\[E=E^o_\mathrm{\large MnO_4^-/Mn^{2+}}-\dfrac{0.05916}{5}\log\dfrac{[\textrm{Mn}^{2+}]}{\ce{[MnO_4^- ][H^+]^8}}\]
Before adding these two equations together we must multiply the second equation by 5 so that we can combine the log terms; thus
\[6E=E^o_\mathrm{\large Fe^{3+}/Fe^{2+}}+5E^o_\mathrm{\large MnO_4^-/Mn^{2+}}-0.05916\log\mathrm{\dfrac{[Fe^{2+}][Mn^{2+}]}{[Fe^{3+}][\ce{MnO_4^-}][H^+]^8}}\]
At the equivalence point we know that
\[[\textrm{Fe}^{2+}]=5\times[\textrm{MnO}_4^-]\]
\[[\textrm{Fe}^{3+}]=5\times[\textrm{Mn}^{2+}]\]
Substituting these equalities into the previous equation and rearranging gives us a general equation for the potential at the equivalence point.
\[6E_\textrm{eq}=E^o_\mathrm{\large Fe^{3+}/Fe^{2+}}+5E^o_\mathrm{\large MnO_4^-/Mn^{2+}}-0.05916\log\mathrm{\dfrac{5[\ce{MnO_4^-}][Mn^{2+}]}{5[Mn^{2+}][\ce{MnO_4^-}][H^+]^8}}\]
\[E_\textrm{eq}=\dfrac{E^o_\mathrm{\large Fe^{3+}/Fe^{2+}} + 5E^o_\mathrm{\large MnO_4^-/Mn^{2+}}}{6}-\dfrac{0.05916}{6}\log\dfrac{1}{[\textrm H^+]^8}\]
\[E_\textrm{eq}=\dfrac{E^o_\mathrm{\large Fe^{3+}/Fe^{2+}}+5E^o_\mathrm{\large MnO_4^-/Mn^{2+}}}{6}+\dfrac{0.05916\times8}{6}\log[\textrm H^+]\]
\[E_\textrm{eq}=\dfrac{E^o_\mathrm{\large Fe^{3+}/Fe^{2+}}+5E^o_\mathrm{\large MnO_4^-/Mn^{2+}}}{6}-0.07888\textrm{pH}\]
Our equation for the equivalence point has two terms. The first term is a weighted average of the titrand’s and the titrant’s standard state potentials, in which the weighting factors are the number of electrons in their respective half-reactions. (Instead of standard state potentials, you can use formal potentials.) The second term shows that Eeq for this titration is pH-dependent. At a pH of 1 (in H2SO4), for example, the equivalence point has a potential of
\[E_\textrm{eq}=\dfrac{0.768+5\times1.51}{6}-0.07888\times1=1.31\textrm{ V}\]
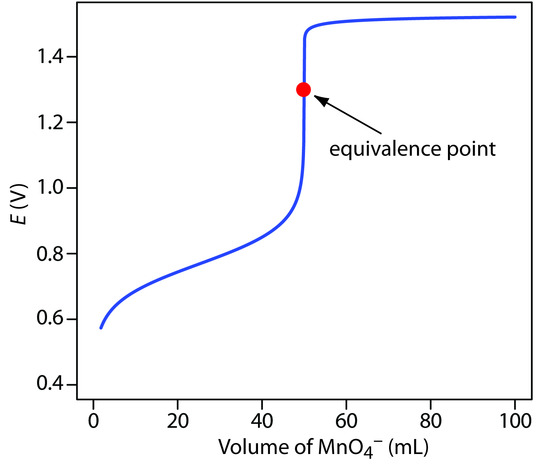
Figure 9.38 shows a typical titration curve for titration of Fe2+ with MnO4–. Note that the titration’s equivalence point is asymmetrical.
Figure 9.38: Titration curve for the titration of 50.0 mL of 0.100 M Fe2+ with 0.0200 M MnO4– at a fixed pH of 1 (using H2SO4). The equivalence point is shown by the red dot.
Exercise \(\PageIndex{3}\)
Derive a general equation for the equivalence point’s potential for the titration of U4+ with Ce4+. The unbalanced reaction is
\[\textrm{Ce}^{4+}(aq)+\textrm U^{4+}(aq)\rightarrow \textrm{UO}_2^{2+}(aq)+\textrm{Ce}^{3+}(aq)\]
What is the equivalence point’s potential if the pH is 1?
Click here to review your answer to this exercise.
Finding the End point with an Indicator
Three types of indicators are used to signal a redox titration’s end point. The oxidized and reduced forms of some titrants, such as MnO4–, have different colors. A solution of MnO4– is intensely purple. In an acidic solution, however, permanganate’s reduced form, Mn2+, is nearly colorless. When using MnO4– as a titrant, the titrand’s solution remains colorless until the equivalence point. The first drop of excess MnO4– produces a permanent tinge of purple, signaling the end point.
Some indicators form a colored compound with a specific oxidized or reduced form of the titrant or the titrand. Starch, for example, forms a dark blue complex with I3–. We can use this distinct color to signal the presence of excess I3– as a titrant—a change in color from colorless to blue—or the completion of a reaction consuming I3– as the titrand—a change in color from blue to colorless. Another example of a specific indicator is thiocyanate, SCN–, which forms a soluble red-colored complex of Fe(SCN)2+ with Fe3+.
The most important class of indicators are substances that do not participate in the redox titration, but whose oxidized and reduced forms differ in color. When we add a redox indicator to the titrand, the indicator imparts a color that depends on the solution’s potential. As the solution’s potential changes with the addition of titrant, the indicator changes oxidation state and changes color, signaling the end point.
To understand the relationship between potential and an indicator’s color, consider its reduction half-reaction
\[\mathrm{In_{ox}}+ne^-\rightleftharpoons \mathrm{In_{red}}\]
where Inox and Inred are, respectively, the indicator’s oxidized and reduced forms.
For simplicity, Inox and Inred are shown without specific charges. Because there is a change in oxidation state, Inox and Inred cannot both be neutral.
The Nernst equation for this half-reaction is
\[E=E^o_\mathrm{In_{\large ox}/In_{\large red}}-\dfrac{0.05916}{n}\log\mathrm{\dfrac{[In_{red}]}{[In_{ox}]}}\]
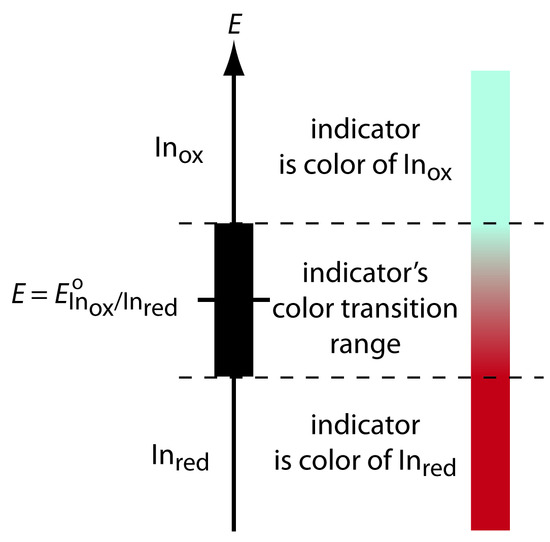
As shown in Figure 9.39, if we assume that the indicator’s color changes from that of Inox to that of Inred when the ratio [Inred]/[Inox] changes from 0.1 to 10, then the end point occurs when the solution’s potential is within the range
\[E=E^o_\mathrm{In_{\large ox}/In_{\large red}}\pm\dfrac{0.05916}{n}\]
This is the same approach we took in considering acid–base indicators and complexation indicators.
Figure 9.39 Diagram showing the relationship between E and an indicator’s color. The ladder diagram defines potentials where Inred and Inox are the predominate species. The indicator changes color when E is within the range
E = EoInox/Inred ± 0.05916/n
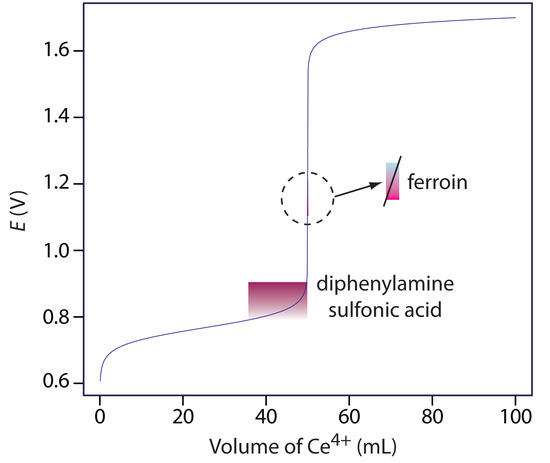
A partial list of redox indicators is shown in Table 9.16. Examples of appropriate and inappropriate indicators for the titration of Fe2+ with Ce4+ are shown in Figure 9.40.
| Indicator | Color of Inox | Color of Inred | EoInox/Inred |
|---|---|---|---|
| indigo tetrasulfate | blue | colorless | 0.36 |
| methylene blue | blue | colorless | 0.53 |
| diphenylamine | violet | colorless | 0.75 |
| diphenylamine sulfonic acid | red-violet | colorless | 0.85 |
| tris(2,2´-bipyridine)iron | pale blue | red | 1.120 |
| ferroin | pale blue | red | 1.147 |
| tris(5-nitro-1,10-phenanthroline)iron | pale blue | red-violet | 1.25 |
Figure 9.40: Titration curve for the titration of 50.0 mL of 0.100 M Fe2+ with 0.100 M Ce4+. The end point transitions for the indicators diphenylamine sulfonic acid and ferroin are superimposed on the titration curve. Because the transition for ferroin is too small to see on the scale of the x-axis—it requires only 1–2 drops of titrant—the color change is expanded to the right.
Other Methods for Finding the End point
Another method for locating a redox titration’s end point is a potentiometric titration in which we monitor the change in potential while adding the titrant to the titrand. The end point is found by visually examining the titration curve. The simplest experimental design for a potentiometric titration consists of a Pt indicator electrode whose potential is governed by the titrand’s or titrant’s redox half-reaction, and a reference electrode that has a fixed potential. A further discussion of potentiometry is found in Chapter 11. Other methods for locating the titration’s end point include thermometric titrations and spectrophotometric titrations.
The best way to appreciate the theoretical and practical details discussed in this section is to carefully examine a typical redox titrimetric method. Although each method is unique, the following description of the determination of the total chlorine residual in water provides an instructive example of a typical procedure. The description here is based on Method 4500-Cl B as published in Standard Methods for the Examination of Water and Wastewater, 20th Ed., American Public Health Association: Washington, D. C., 1998.
Representative Method 9.3: Determination of Total Chlorine Residual
Description of the Method
The chlorination of public water supplies produces several chlorine-containing species, the combined concentration of which is called the total chlorine residual. Chlorine may be present in a variety of states, including the free residual chlorine, consisting of Cl2, HOCl and OCl–, and the combined chlorine residual, consisting of NH2Cl, NHCl2, and NCl3. The total chlorine residual is determined by using the oxidizing power of chlorine to convert I– to I3–. The amount of I3– formed is then determined by titrating with Na2S2O3 using starch as an indicator. Regardless of its form, the total chlorine residual is reported as if Cl2 is the only source of chlorine, and is reported as mg Cl/L.
Procedure
Select a volume of sample requiring less than 20 mL of Na2S2O3 to reach the end point. Using glacial acetic acid, acidify the sample to a pH of 3–4, and add about 1 gram of KI. Titrate with Na2S2O3 until the yellow color of I3– begins to disappear. Add 1 mL of a starch indicator solution and continue titrating until the blue color of the starch–I3– complex disappears (Figure 9.41). Use a blank titration to correct the volume of titrant needed to reach the end point for reagent impurities.
Questions
1. Is this an example of a direct or an indirect analysis?
This is an indirect analysis because the chlorine-containing species do not react with the titrant. Instead, the total chlorine residual oxidizes I– to I3–, and the amount of I3– is determined by titrating with Na2S2O3.
2. Why does the procedure rely on an indirect analysis instead of directly titrating the chlorine-containing species using KI as a titrant?
Because the total chlorine residual consists of six different species, a titration with I– does not have a single, well-defined equivalence point. By converting the chlorine residual to an equivalent amount of I3–, the indirect titration with Na2S2O3 has a single, useful equivalence point.
Even if the total chlorine residual is from a single species, such as HOCl, a direct titration with KI is impractical. Because the product of the titration, I3–, imparts a yellow color, the titrand’s color would change with each addition of titrant, making it difficult to find a suitable indicator.
3. Both oxidizing and reducing agents can interfere with this analysis. Explain the effect of each type of interferent has on the total chlorine residual.
An interferent that is an oxidizing agent converts additional I– to I3–. Because this extra I3– requires an additional volume of Na2S2O3 to reach the end point, we overestimate the total chlorine residual. If the interferent is a reducing agent, it reduces back to I– some of the I3– produced by the reaction between the total chlorine residual and iodide. As a result. we underestimate the total chlorine residual.
Figure 9.41 Endpoint for the determination of the total chlorine residual. (a) Acidifying the sample and adding KI forms a brown solution of I3–. (b) Titrating with Na2S2O3 converts I3– to I– with the solution fading to a pale yellow color as we approach the end point. (c) Adding starch forms the deep purple starch–I3– complex. (d) As the titration continues, the end point is a sharp transition from a purple to a colorless solution. The change in color from (c) to (d) typically takes 1–2 drops of titrant.
9.4.3 Quantitative Applications
Although many quantitative applications of redox titrimetry have been replaced by other analytical methods, a few important applications continue to be relevant. In this section we review the general application of redox titrimetry with an emphasis on environmental, pharmaceutical, and industrial applications. We begin, however, with a brief discussion of selecting and characterizing redox titrants, and methods for controlling the titrand’s oxidation state.
Adjusting the Titrand’s Oxidation State
If a redox titration is to be used in a quantitative analysis, the titrand must initially be present in a single oxidation state. For example, iron can be determined by a redox titration in which Ce4+ oxidizes Fe2+ to Fe3+. Depending on the sample and the method of sample preparation, iron may initially be present in both the +2 and +3 oxidation states. Before titrating, we must reduce any Fe3+ to Fe2+. This type of pretreatment can be accomplished using an auxiliary reducing agent or oxidizing agent.
A metal that is easy to oxidize—such as Zn, Al, and Ag—can serve as an auxiliary reducing agent. The metal, as a coiled wire or powder, is added to the sample where it reduces the titrand. Because any unreacted auxiliary reducing agent will react with the titrant, it must be removed before beginning the titration. This can be accomplished by simply removing the coiled wire, or by filtering.
An alternative method for using an auxiliary reducing agent is to immobilize it in a column. To prepare a reduction column an aqueous slurry of the finally divided metal is packed in a glass tube equipped with a porous plug at the bottom. The sample is placed at the top of the column and moves through the column under the influence of gravity or vacuum suction. The length of the reduction column and the flow rate are selected to ensure the analyte’s complete reduction.
Two common reduction columns are used. In the Jones reductor the column is filled with amalgamated zinc, Zn(Hg), prepared by briefly placing Zn granules in a solution of HgCl2. Oxidation of zinc
\[\textrm{Zn(Hg)}(s)\rightarrow \textrm{Zn}^{2+}(aq)+\textrm{Hg}(l)+2e^-\]
provides the electrons for reducing the titrand. In the Walden reductor the column is filled with granular Ag metal. The solution containing the titrand is acidified with HCl and passed through the column where the oxidation of silver
\[\textrm{Ag}(s)+\textrm{Cl}^-(aq)\rightarrow \textrm{AgCl}(s)+e^-\]
provides the necessary electrons for reducing the titrand. Table 9.17 provides a summary of several applications of reduction columns.
| Oxidized Titrand | Walden Reductor | Jones Reductor |
|---|---|---|
| Cr3+ | — | Cr3+(aq) + e− → Cr2+(aq) |
| Cu2+ | Cu2+(aq) +e− → Cu+(aq) | Cu2+(aq) + 2e− → Cr(s) |
| Fe3+ | Fe3+(aq) + e− → Fe2+(aq) | Fe3+(aq) + e− → Fe2+(aq) |
| TiO2+ | — |
TiO2+(aq) + 2H+(aq) + e− → Ti3+(aq) + H2O(l)
|
| MoO22+ | MoO22+(aq) + e−→ MoO2+(aq) | MoO22+(aq) + 4H+(aq) + 3e−→ Mo3+(aq) + 2H2O(l) |
| VO2+ | VO2+(aq) + 2H+(aq) + e− → VO2+(aq) + H2O(l) | VO2+(aq) + 4H+(aq) + 3e− → V2+(aq) + 2H2O(l) |
Several reagents are commonly used as auxiliary oxidizing agents, including ammonium peroxydisulfate, (NH4)2S2O8, and hydrogen peroxide, H2O2. Peroxydisulfate is a powerful oxidizing agent
\[\mathrm{S_2O_8^{2-}}(aq)+2e^-\rightarrow\mathrm{2SO_4^{2-}}(aq)\]
capable of oxidizing Mn2+ to MnO4–, Cr3+ to Cr2O72–, and Ce3+ to Ce4+. Excess peroxydisulfate is easily destroyed by briefly boiling the solution. The reduction of hydrogen peroxide in acidic solution
\[\mathrm{H_2O_2}(aq)+\mathrm{2H^+}(aq)+2e^-\rightarrow\mathrm{2H_2O}(l)\]
provides another method for oxidizing a titrand. Excess H2O2 is destroyed by briefly boiling the solution.
Selecting and Standardizing a Titrant
If it is to be used quantitatively, the titrant’s concentration must remain stable during the analysis. Because a titrant in a reduced state is susceptible to air oxidation, most redox titrations use an oxidizing agent as the titrant. There are several common oxidizing titrants, including MnO4–, Ce4+, Cr2O72–, and I3–. Which titrant is used often depends on how easy it is to oxidize the titrand. A titrand that is a weak reducing agent needs a strong oxidizing titrant if the titration reaction is to have a suitable end point.
The two strongest oxidizing titrants are MnO4– and Ce4+, for which the reduction half-reactions are
\[\ce{MnO_4^-}(aq)+\mathrm{8H^+}(aq)+5e^-\rightleftharpoons \mathrm{Mn^{2+}}(aq)+\mathrm{4H_2O}(l)\]
\[\textrm{Ce}^{4+}(aq)+e^-\rightleftharpoons \textrm{Ce}^{3+}(aq)\]
Solutions of Ce4+ usually are prepared from the primary standard cerium ammonium nitrate, Ce(NO3)4•2NH4NO3, in 1 M H2SO4. When prepared using a reagent grade material, such as Ce(OH)4, the solution is standardized against a primary standard reducing agent such as Na2C2O4 or Fe2+ (prepared using iron wire) using ferroin as an indicator. Despite its availability as a primary standard and its ease of preparation, Ce4+ is not as frequently used as MnO4– because it is more expensive.
Note
The standardization reactions are
\[\mathrm{Ce^{4+}}(aq)+\mathrm{Fe^{2+}}(aq)\rightarrow \mathrm{Ce^{3+}}(aq)+\mathrm{Fe^{3+}}(aq)\]
\[\mathrm{2Ce^{4+}}(aq)+\mathrm{H_2C_2O_4}(aq)\rightarrow \mathrm{2Ce^{3+}}(aq)+\mathrm{2CO_2}(g)+\mathrm{2H^+}(aq)\]
Solutions of MnO4– are prepared from KMnO4, which is not available as a primary standard. Aqueous solutions of permanganate are thermodynamically unstable due to its ability to oxidize water.
\[\ce{4MnO_4^-}(aq)+\mathrm{2H_2O}(l)\rightleftharpoons\mathrm{4MnO_2}(s)+\mathrm{3O_2}(g)+\mathrm{4OH^-}(aq)\]
This reaction is catalyzed by the presence of MnO2, Mn2+, heat, light, and the presence of acids and bases. A moderately stable solution of permanganate can be prepared by boiling it for an hour and filtering through a sintered glass filter to remove any solid MnO2 that precipitates. Standardization is accomplished against a primary standard reducing agent such as Na2C2O4 or Fe2+ (prepared using iron wire), with the pink color of excess MnO4– signaling the end point. A solution of MnO4– prepared in this fashion is stable for 1–2 weeks, although the standardization should be rechecked periodically.
Note
The standardization reactions are
\[\ce{MnO_4^-}(aq)+\mathrm{5Fe^{2+}}(aq)+\mathrm{8H^+}(aq)\rightarrow \mathrm{Mn^{2+}}(aq)+\mathrm{5Fe^{3+}}(aq)+\mathrm{4H_2O}(l)\]
\[\ce{2MnO_4^-}(aq)+\mathrm{5H_2C_2O_4}(aq)+\mathrm{6H^+}(aq)\rightarrow\mathrm{2Mn^{2+}}(aq)+\mathrm{10CO_2}(g)+\mathrm{8H_2O}(l)\]
Potassium dichromate is a relatively strong oxidizing agent whose principal advantages are its availability as a primary standard and the long term stability of its solutions. It is not, however, as strong an oxidizing agent as MnO4– or Ce4+, which makes it less useful when the titrand is a weak reducing agent. Its reduction half-reaction is
\[\mathrm{Cr_2O_7^{2-}}(aq)+\mathrm{14H^+}(aq)+6e^-\rightleftharpoons \mathrm{2Cr^{3+}}(aq)+\mathrm{7H_2O}(l)\]
Although a solution of Cr2O72– is orange and a solution of Cr3+ is green, neither color is intense enough to serve as a useful indicator. Diphenylamine sulfonic acid, whose oxidized form is red-violet and reduced form is colorless, gives a very distinct end point signal with Cr2O72–.
Iodine is another important oxidizing titrant. Because it is a weaker oxidizing agent than MnO4–, Ce4+, and Cr2O72–, it is useful only when the titrand is a stronger reducing agent. This apparent limitation, however, makes I2 a more selective titrant for the analysis of a strong reducing agent in the presence of a weaker reducing agent. The reduction half-reaction for I2 is
\[\textrm I_2(aq) + 2e^-\rightleftharpoons 2\textrm I^-(aq)\]
Because iodine is not very soluble in water, solutions are prepared by adding an excess of I–. The complexation reaction
\[\textrm I_2(aq)+\textrm I^-(aq)\rightleftharpoons\textrm I_3^-(aq)\]
increases the solubility of I2 by forming the more soluble triiodide ion, I3–. Even though iodine is present as I3– instead of I2, the number of electrons in the reduction half-reaction is unaffected.
\[\textrm I_3^-(aq)+2e^-\rightleftharpoons 3\textrm I^-(aq)\]
Solutions of I3– are normally standardized against Na2S2O3 using starch as a specific indicator for I3–.
Note
The standardization reaction is
\[\mathrm I_3^-(aq)+\mathrm{2S_2O_3^{2-}}(aq)\rightarrow 3\textrm I^-(aq)+\mathrm{2S_4O_6^{2-}}(aq)\]
An oxidizing titrant such as MnO4–, Ce4+, Cr2O72–, and I3–, is used when the titrand is in a reduced state. If the titrand is in an oxidized state, we can first reduce it with an auxiliary reducing agent and then complete the titration using an oxidizing titrant. Alternatively, we can titrate it using a reducing titrant. Iodide is a relatively strong reducing agent that could serve as a reducing titrant except that a solution of I– is susceptible to the air-oxidation of I– to I3–.
\[3\textrm I^-(aq)\rightleftharpoons \mathrm I_3^-(aq)+2e^-\]
Note
A freshly prepared solution of KI is clear, but after a few days it may show a faint yellow coloring due to the presence of I3–.
Instead, adding an excess of KI reduces the titrand, releasing a stoichiometric amount of I3–. The amount of I3– produced is then determined by a back titration using thiosulfate, S2O32–, as a reducing titrant.
\[\mathrm{2S_2O_3^{2-}}(aq)\rightleftharpoons\mathrm{2S_4O_6^{2-}}(aq)+2e^-\]
Solutions of S2O32– are prepared using Na2S2O3•5H2O, and must be standardized before use. Standardization is accomplished by dissolving a carefully weighed portion of the primary standard KIO3 in an acidic solution containing an excess of KI. The reaction between IO3– and I–
\[\textrm{IO}_3^-(aq)+8\textrm I^-(aq)+6\textrm H^+(aq)\rightarrow \ce{3I_3^-}(aq)+\mathrm{3H_2O}(l)\]
liberates a stoichiometric amount of I3–. By titrating this I3– with thiosulfate, using starch as a visual indicator, we can determine the concentration of S2O32– in the titrant.
Note
The standardization titration is
\[\mathrm I_3^-(aq)+\mathrm{2S_2O_3^{2-}}(aq)\rightarrow 3\textrm I^-(aq)+\mathrm{2S_4O_6^{2-}}(aq)\]
which is the same reaction used to standardize solutions of I3−. This approach to standardizing solutions of S2O32−. is similar to the determination of the total chlorine residual outlined in Representative Method 9.3.
Although thiosulfate is one of the few reducing titrants that is not readily oxidized by contact with air, it is subject to a slow decomposition to bisulfite and elemental sulfur. If used over a period of several weeks, a solution of thiosulfate should be restandardized periodically. Several forms of bacteria are able to metabolize thiosulfate, which also can lead to a change in its concentration. This problem can be minimized by adding a preservative such as HgI2 to the solution.
Another useful reducing titrant is ferrous ammonium sulfate, Fe(NH4)2(SO4)2•6H2O, in which iron is present in the +2 oxidation state. A solution of Fe2+ is susceptible to air-oxidation, but when prepared in 0.5 M H2SO4 it remains stable for as long as a month. Periodic restandardization with K2Cr2O7 is advisable. The titrant can be used to directly titrate the titrand by oxidizing Fe2+ to Fe3+. Alternatively, ferrous ammonium sulfate is added to the titrand in excess and the quantity of Fe3+ produced determined by back titrating with a standard solution of Ce4+ or Cr2O72–.
Inorganic Analysis
One of the most important applications of redox titrimetry is evaluating the chlorination of public water supplies. Representative Method 9.3, for example, describes an approach for determining the total chlorine residual by using the oxidizing power of chlorine to oxidize I– to I3–. The amount of I3– is determined by back titrating with S2O32–.
The efficiency of chlorination depends on the form of the chlorinating species. There are two contributions to the total chlorine residual—the free chlorine residual and the combined chlorine residual. The free chlorine residual includes forms of chlorine that are available for disinfecting the water supply. Examples of species contributing to the free chlorine residual include Cl2, HOCl and OCl–. The combined chlorine residual includes those species in which chlorine is in its reduced form and, therefore, no longer capable of providing disinfection. Species contributing to the combined chlorine residual are NH2Cl, NHCl2 and NCl3.
When a sample of iodide-free chlorinated water is mixed with an excess of the indicator N,N-diethyl-p-phenylenediamine (DPD), the free chlorine oxidizes a stoichiometric portion of DPD to its red-colored form. The oxidized DPD is then back titrated to its colorless form using ferrous ammonium sulfate as the titrant. The volume of titrant is proportional to the free residual chlorine.
Having determined the free chlorine residual in the water sample, a small amount of KI is added, catalyzing the reduction monochloramine, NH2Cl, and oxidizing a portion of the DPD back to its red-colored form. Titrating the oxidized DPD with ferrous ammonium sulfate yields the amount of NH2Cl in the sample. The amount of dichloramine and trichloramine are determined in a similar fashion.
The methods described above for determining the total, free, or combined chlorine residual also are used to establish a water supply’s chlorine demand. Chlorine demand is defined as the quantity of chlorine needed to completely react with any substance that can be oxidized by chlorine, while also maintaining the desired chlorine residual. It is determined by adding progressively greater amounts of chlorine to a set of samples drawn from the water supply and determining the total, free, or combined chlorine residual.
Another important example of redox titrimetry, which finds applications in both public health and environmental analyses is the determination of dissolved oxygen. In natural waters, such as lakes and rivers, the level of dissolved O2 is important for two reasons: it is the most readily available oxidant for the biological oxidation of inorganic and organic pollutants; and it is necessary for the support of aquatic life. In a wastewater treatment plant dissolved O2 is essential for the aerobic oxidation of waste materials. If the concentration of dissolved O2 falls below a critical value, aerobic bacteria are replaced by anaerobic bacteria, and the oxidation of organic waste produces undesirable gases, such as CH4 and H2S.
One standard method for determining the dissolved O2 content of natural waters and wastewaters is the Winkler method. A sample of water is collected without exposing it to the atmosphere, which might change the concentration of dissolved O2. The sample is first treated with a solution of MnSO4, and then with a solution of NaOH and KI. Under these alkaline conditions the dissolved oxygen oxidizes Mn2+ to MnO2.
\[\mathrm{2Mn^{2+}}(aq)+\mathrm{4OH^-}(aq)+\mathrm O_2(g)\rightarrow \mathrm{2MnO_2}(s)+\mathrm{2H_2O}(l)\]
After the reaction is complete, the solution is acidified with H2SO4. Under the now acidic conditions I– is oxidized to I3– by MnO2.
\[\mathrm{MnO_2}(s)+\mathrm{3I^-}(aq)+\mathrm{4H^+}(aq)\rightarrow \mathrm{Mn^{2+}}+\ce{I_3^-}(aq)+\mathrm{2H_2O}(l)\]
The amount of I3– formed is determined by titrating with S2O32– using starch as an indicator. The Winkler method is subject to a variety of interferences, and several modifications to the original procedure have been proposed. For example, NO2– interferes because it can reduce I3– to I– under acidic conditions. This interference is eliminated by adding sodium azide, NaN3, reducing NO2– to N2. Other reducing agents, such as Fe2+, are eliminated by pretreating the sample with KMnO4, and destroying the excess permanganate with K2C2O4.
Another important example of redox titrimetry is the determination of water in nonaqueous solvents. The titrant for this analysis is known as the Karl Fischer reagent and consists of a mixture of iodine, sulfur dioxide, pyridine, and methanol. Because the concentration of pyridine is sufficiently large, I2 and SO2 react with pyridine (py) to form the complexes py•I2 and py•SO2. When added to a sample containing water, I2 is reduced to I– and SO2 is oxidized to SO3.
\[\textrm{py}\bullet\textrm I_2+\textrm{py}\bullet\mathrm{SO_2}+\textrm{py}+\mathrm{H_2O}\rightarrow 2\textrm{py}\bullet\textrm{HI}+\textrm{py}\bullet\mathrm{SO_3}\]
Methanol is included to prevent the further reaction of py•SO3 with water. The titration’s end point is signaled when the solution changes from the product’s yellow color to the brown color of the Karl Fischer reagent.
Organic Analysis
Redox titrimetry also is used for the analysis of organic analytes. One important example is the determination of the chemical oxygen demand (COD) of natural waters and wastewaters. The COD provides a measure of the quantity of oxygen necessary to completely oxidize all the organic matter in a sample to CO2 and H2O. Because no attempt is made to correct for organic matter that can not be decomposed biologically, or for slow decomposition kinetics, the COD always overestimates a sample’s true oxygen demand. The determination of COD is particularly important in managing industrial wastewater treatment facilities where it is used to monitor the release of organic-rich wastes into municipal sewer systems or the environment.
A sample’s COD is determined by refluxing it in the presence of excess K2Cr2O7, which serves as the oxidizing agent. The solution is acidified with H2SO4 using Ag2SO4 to catalyze the oxidation of low molecular weight fatty acids. Mercuric sulfate, HgSO4, is added to complex any chloride that is present, preventing the precipitation of the Ag+ catalyst as AgCl. Under these conditions, the efficiency for oxidizing organic matter is 95–100%. After refluxing for two hours, the solution is cooled to room temperature and the excess Cr2O72– is determined by back titrating using ferrous ammonium sulfate as the titrant and ferroin as the indicator. Because it is difficult to completely remove all traces of organic matter from the reagents, a blank titration must be performed. The difference in the amount of ferrous ammonium sulfate needed to titrate the sample and the blank is proportional to the COD.
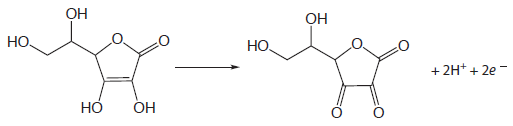
Iodine has been used as an oxidizing titrant for a number of compounds of pharmaceutical interest. Earlier we noted that the reaction of S2O32– with I3– produces the tetrathionate ion, S4O62–. The tetrathionate ion is actually a dimer consisting of two thiosulfate ions connected through a disulfide (–S–S–) linkage. In the same fashion, I3– can be used to titrate mercaptans of the general formula RSH, forming the dimer RSSR as a product. The amino acid cysteine also can be titrated with I3–. The product of this titration is cystine, which is a dimer of cysteine. Triiodide also can be used for the analysis of ascorbic acid (vitamin C) by oxidizing the enediol functional group to an alpha diketone
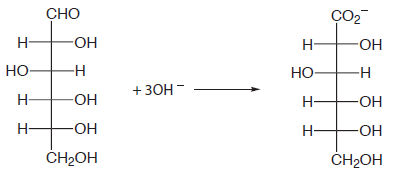
and for the analysis of reducing sugars, such as glucose, by oxidizing the aldehyde functional group to a carboxylate ion in a basic solution.
An organic compound containing a hydroxyl, a carbonyl, or an amine functional group adjacent to an hydoxyl or a carbonyl group can be oxidized using metaperiodate, IO4–, as an oxidizing titrant.
\[\ce{IO_4^-}(aq)+\mathrm{H_2O}(l)+2e^-\rightleftharpoons \ce{IO_3^-}(aq)+\mathrm{2OH^-}(aq)\]
A two-electron oxidation cleaves the C–C bond between the two functional groups, with hydroxyl groups being oxidized to aldehydes or ketones, carbonyl functional groups being oxidized to carboxylic acids, and amines being oxidized to an aldehyde and an amine (ammonia if a primary amine). The analysis is conducted by adding a known excess of IO4– to the solution containing the analyte, and allowing the oxidation to take place for approximately one hour at room temperature. When the oxidation is complete, an excess of KI is added, which converts any unreacted IO4– to IO3– and I3–.
\[\ce{IO_4^-}(aq)+3\mathrm I^-(aq)+\mathrm{H_2O}(l)\rightarrow \ce{IO_3^-}(aq)+\textrm I_3^-(aq)+\mathrm{2OH^-}(aq)\]
The I3– is then determined by titrating with S2O32– using starch as an indicator.
Quantitative Calculations
The quantitative relationship between the titrand and the titrant is determined by the stoichiometry of the titration reaction. If you are unsure of the balanced reaction, you can deduce the stoichiometry by remembering that the electrons in a redox reaction must be conserved.
Example 9.11
The amount of Fe in a 0.4891-g sample of an ore was determined by titrating with K2Cr2O7. After dissolving the sample in HCl, the iron was brought into the +2 oxidation state using a Jones reductor. Titration to the diphenylamine sulfonic acid end point required 36.92 mL of 0.02153 M K2Cr2O7. Report the ore’s iron content as %w/w Fe2O3.
(Although we can deduce the stoichiometry between the titrant and the titrand without balancing the titration reaction, the balanced reaction
\[\mathrm{K_2Cr_2O_7}(aq)+\mathrm{6Fe^{2+}}(aq)+\mathrm{14H^+}(aq)\rightarrow \mathrm{2Cr^{3+}}(aq)+\mathrm{2K^+}(aq)+\mathrm{6Fe^{3+}}(aq)+\mathrm{7H_2O}(l)\]
does provide useful information. For example, the presence of H+ reminds us that the reactionfs feasibility is pH-dependent.)
Solution
Because we have not been provided with the titration reaction, let’s use a conservation of electrons to deduce the stoichiometry. During the titration the analyte is oxidized from Fe2+ to Fe3+, and the titrant is reduced from Cr2O72– to Cr3+. Oxidizing Fe2+ to Fe3+ requires only a single electron. Reducing Cr2O72–, in which each chromium is in the +6 oxidation state, to Cr3+ requires three electrons per chromium, for a total of six electrons. A conservation of electrons for the titration, therefore, requires that each mole of K2Cr2O7 reacts with six moles of Fe2+.
The moles of K2Cr2O7 used in reaching the end point is
\[\mathrm{(0.02153\;M\;K_2Cr_2O_7)\times(0.03692\;L\;K_2Cr_2O_7)=7.949\times10^{-4}\;mol\;K_2Cr_2O_7}\]
which means that the sample contains
\[\mathrm{7.949\times10^{-4}\;mol\;K_2Cr_2O_7\times\dfrac{6\;mol\;Fe^{2+}}{mol\;K_2Cr_2O_7}=4.769\times10^{-3}\;mol\;Fe^{2+}}\]
Thus, the %w/w Fe2O3 in the sample of ore is
\[\mathrm{4.769\times10^{-3}\;mol\;Fe^{2+}\times\dfrac{1\;mol\;Fe_2O_3}{2\;mol\;Fe^{2+}}\times\dfrac{159.69\;g\;Fe_2O_3}{mol\;Fe_2O_3}=0.3808\;g\;Fe_2O_3}\]
\[\mathrm{\dfrac{0.3808\;g\;Fe_2O_3}{0.4891\;g\;sample}\times100=77.86\%\;w/w\;Fe_2O_3}\]
Practice Exercise 9.20
The purity of a sample of sodium oxalate, Na2C2O4, is determined by titrating with a standard solution of KMnO4. If a 0.5116-g sample requires 35.62 mL of 0.0400 M KMnO4 to reach the titration’s end point, what is the %w/w Na2C2O4 in the sample.
Click here to review your answer to this exercise.
As shown in the following two examples, we can easily extend this approach to an analysis that requires an indirect analysis or a back titration.
Example 9.12
A 25.00-mL sample of a liquid bleach was diluted to 1000 mL in a volumetric flask. A 25-mL portion of the diluted sample was transferred by pipet into an Erlenmeyer flask containing an excess of KI, reducing the OCl– to Cl–, and producing I3–. The liberated I3– was determined by titrating with 0.09892 M Na2S2O3, requiring 8.96 mL to reach the starch indicator end point. Report the %w/v NaOCl in the sample of bleach.
Solution
To determine the stoichiometry between the analyte, NaOCl, and the titrant, Na2S2O3, we need to consider both the reaction between OCl– and I–, and the titration of I3– with Na2S2O3.
First, in reducing OCl– to Cl–, the oxidation state of chlorine changes from +1 to –1, requiring two electrons. The oxidation of three I– to form I3– releases two electrons as the oxidation state of each iodine changes from –1 in I– to –⅓ in I3–. A conservation of electrons, therefore, requires that each mole of OCl– produces one mole of I3–.
Second, in the titration reaction, I3–. is reduced to I– and S2O32– is oxidized to S4O62–. Reducing I3– to 3I– requires two elections as each iodine changes from an oxidation state of –⅓ to –1. In oxidizing S2O32– to S4O62–, each sulfur changes its oxidation state from +2 to +2.5, releasing one electron for each S2O32–. A conservation of electrons, therefore, requires that each mole of I3– reacts with two moles of S2O32–.
Finally, because each mole of OCl– produces one mole of I3–, and each mole of I3– reacts with two moles of S2O32–, we know that every mole of NaOCl in the sample ultimately results in the consumption of two moles of Na2S2O3.
The balanced reactions for this analysis are:
\[\mathrm{OCl^-}(aq)+\mathrm{3I^-}(aq)+\mathrm{2H^+}(aq)\rightarrow \ce{I_3^-}(aq)+\mathrm{Cl^-}(aq)+\mathrm{H_2O}(l)\]
\[\mathrm I_3^-(aq)+\mathrm{2S_2O_3^{2-}}(aq)\rightarrow \mathrm{S_4O_6^{2-}}(aq)+\mathrm{3I^-}(aq)\]
The moles of Na2S2O3 used in reaching the titration’s end point is
\[\mathrm{(0.09892\;M\;Na_2S_2O_3)\times(0.00896\;L\;Na_2S_2O_3)=8.86\times10^{-4}\;mol\;Na_2S_2O_3}\]
which means the sample contains
\[\mathrm{8.86\times10^{-4}\;mol\;Na_2S_2O_3\times\dfrac{1\;mol\;NaOCl}{2\;mol\;Na_2S_2O_3}\times\dfrac{74.44\;g\;NaOCl}{mol\;NaOCl}=0.03299\;g\;NaOCl}\]
Thus, the %w/v NaOCl in the diluted sample is
\[\mathrm{\dfrac{0.03299\;g\;NaOCl}{25.00\;mL}\times100=0.132\%\;w/v\;NaOCl}\]
Because the bleach was diluted by a factor of 40 (25 mL to 1000 mL), the concentration of NaOCl in the bleach is 5.28% (w/v).
Example 9.13
The amount of ascorbic acid, C6H8O6, in orange juice was determined by oxidizing the ascorbic acid to dehydroascorbic acid, C6H6O6, with a known amount of I3–, and back titrating the excess I3– with Na2S2O3. A 5.00-mL sample of filtered orange juice was treated with 50.00 mL of 0.01023 M I3–. After the oxidation was complete, 13.82 mL of 0.07203 M Na2S2O3 was needed to reach the starch indicator end point. Report the concentration ascorbic acid in mg/100 mL.
Solution
For a back titration we need to determine the stoichiometry between I3– and the analyte, C6H8O6, and between I3– and the titrant, Na2S2O3. The later is easy because we know from Example 9.12 that each mole of I3– reacts with two moles of Na2S2O3.
The balanced reactions for this analysis are:
\[\mathrm{C_6H_8O_6}(aq)+\ce{I_3^-}(aq)\rightarrow \mathrm{3I^-}(aq)+\mathrm{C_6H_6O_6}(aq)+\mathrm{2H^+}(aq)\]
\[\ce{I_3^-}(aq)+\mathrm{2S_2O_3^{2-}}(aq)\rightarrow \mathrm{S_4O_6^{2-}}(aq)+\mathrm{3I^-}(aq)\]
In oxidizing ascorbic acid to dehydroascorbic acid, the oxidation state of carbon changes from +⅔ in C6H8O6 to +1 in C6H6O6. Each carbon releases ⅓ of an electron, or a total of two electrons per ascorbic acid. As we learned in Example 9.12, reducing I3– requires two electrons; thus, a conservation of electrons requires that each mole of ascorbic acid consumes one mole of I3–.
The total moles of I3– reacting with C6H8O6 and with Na2S2O3 is
\[\mathrm{(0.01023\;M\;\ce{I_3^-})\times(0.05000\;L\;\ce{I_3^-})=5.115\times10^{-4}\;mol\;\ce{I_3^-}}\]
The back titration consumes
\[\mathrm{0.01382\;L\;Na_2S_2O_3\times\dfrac{0.07203\;mol\;Na_2S_2O_3}{L\;Na_2S_2O_3}\times\dfrac{1\;mol\;\ce{I_3^-}}{2\;mol\;Na_2S_2O_3}=4.977\times10^{-4}\;mol\;\ce{I_3^-}}\]
Subtracting the moles of I3– reacting with Na2S2O3 from the total moles of I3– gives the moles reacting with ascorbic acid.
\[\mathrm{5.115\times10^{-4}\;mol\;\ce{I_3^-} - 4.977\times10^{-4}\;mol\;\ce{I_3^-}=1.38\times10^{-5}\;mol\;\ce{I_3^-}}\]
The grams of ascorbic acid in the 5.00-mL sample of orange juice is
\[\mathrm{1.38\times10^{-5}\;mol\;\ce{I_3^-}\times\dfrac{1\;mol\;C_6H_8O_6}{mol\;\ce{I_3^-}}\times\dfrac{176.13\;g\;C_6H_8O_6}{mol\;C_6H_8O_6}=2.43\times10^{-3}\;g\;C_6H_8O_6}\]
There are 2.43 mg of ascorbic acid in the 5.00-mL sample, or 48.6 mg per 100 mL of orange juice.
Practice Exercise 9.21
A quantitative analysis for ethanol, C2H6O, can be accomplished by a redox back titration. Ethanol is oxidized to acetic acid, C2H4O2, using excess dichromate, Cr2O72–, which is reduced to Cr3+. The excess dichromate is titrated with Fe2+, giving Cr3+ and Fe3+ as products. In a typical analysis, a 5.00-mL sample of a brandy is diluted to 500 mL in a volumetric flask. A 10.00-mL sample is taken and the ethanol is removed by distillation and collected in 50.00 mL of an acidified solution of 0.0200 M K2Cr2O7. A back titration of the unreacted Cr2O72– requires 21.48 mL of 0.1014 M Fe2+. Calculate the %w/v ethanol in the brandy.
Click here to review your answer to this exercise.
9.4.4 Evaluation of Redox Titrimetry
The scale of operations, accuracy, precision, sensitivity, time, and cost of a redox titration are similar to those described earlier in this chapter for acid–base or a complexation titration. As with acid–base titrations, we can extend a redox titration to the analysis of a mixture of analytes if there is a significant difference in their oxidation or reduction potentials. Figure 9.42 shows an example of the titration curve for a mixture of Fe2+ and Sn2+ using Ce4+ as the titrant. A titration of a mixture of analytes is possible if their standard state potentials or formal potentials differ by at least 200 mV.
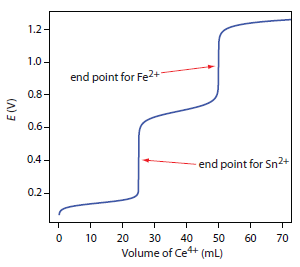
Figure 9.42 Titration curve for the titration of 50.0 mL of 0.0125 M Sn2+ and 0.0250 M Fe2+ with 0.050 M Ce4+. Both the titrand and the titrant are 1M in HCl.