20.2 Structure and Properties of Carboxylic Acids
- Page ID
- 90982
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Objectives
After completing this section, you should be able to
- describe the geometry and electronic structure of a simple carboxylic acid; for example, acetic acid.
- describe the hydrogen bonding that occurs between carboxylic acid molecules, and hence account for the relatively high boiling points of these compounds.
- write an expression for the dissociation constant of a given carboxylic acid, and use it to calculate dissociation constants, percentage dissociation, etc.
- identify carboxylic acids as being weaker acids than mineral acids, such as hydrochloric acid, but stronger acids than alcohols.
- use the concept of resonance to explain why carboxylic acids are stronger acids than alcohols.
- draw an orbital picture of a carboxylate anion to show the equivalence of the two oxygen atoms.
- explain why the two carbon‑oxygen bond lengths are identical in sodium carboxylate, but different in carboxylic acid.
- write an equation for the reaction of a carboxylic acid with a base, such as sodium hydroxide.
You might wish to review Sections 2.7, “Acids and Bases: The Brønsted‑Lowry Definition” and 6.7, “Describing a Reaction: Equilibria, Rates, and Energy Changes” in conjunction with this section.
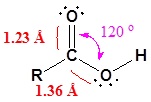
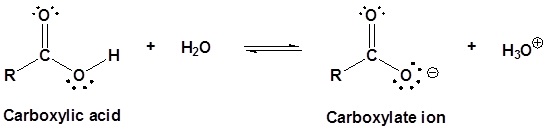
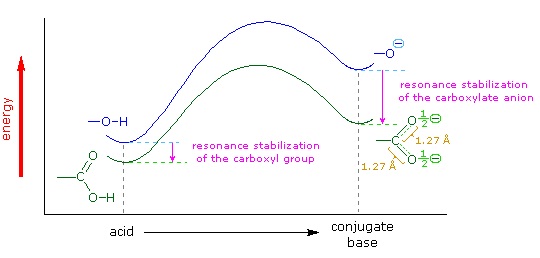
In the reading, the discussion of the role of resonance in the acidity of a carboxylic acid explains that the two carbon‑oxygen bonds in the delocalized carboxylate anion are identical (both 1.27 Å). However, in the structure of a carboxylic acid the C$\ce{-}$O bond (1.20 Å) is shorter than the C$\ce{-}$OH bond (1.34 Å).
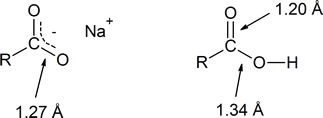
Structure of the carboxyl acid group
Carboxylic acids are organic compounds which incorporate a carboxyl functional group, CO2H. The name carboxyl comes from the fact that a carbonyl and a hydroxyl group are attached to the same carbon.
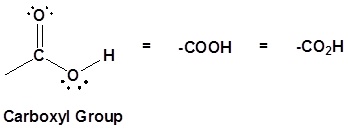
The carbon and oxygen in the carbonyl are both sp2 hybridized which give a carbonyl group a basic trigonal shape. The hydroxyl oxygen is also sp2 hybridized which allows one of its lone pair electrons to conjugate with the pi system of the carbonyl group. This make the carboxyl group planar an can represented with the following resonance structure.
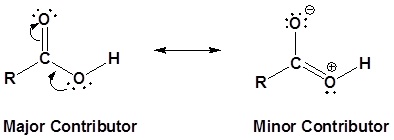
Carboxylic acids are named such because they can donate a hydrogen to produce a carboxylate ion. The factors which affect the acidity of carboxylic acids will be discussed later.
Physical Properties of Some Carboxylic Acids
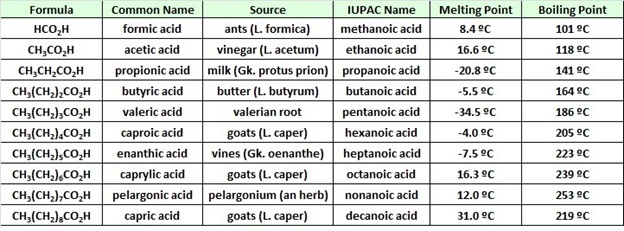
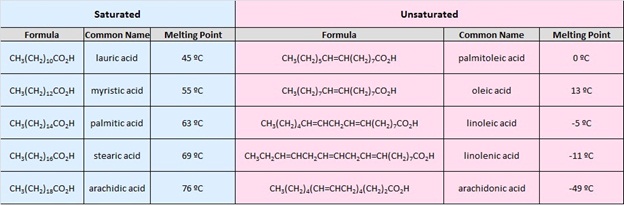
The table at the beginning of this page gave the melting and boiling points for a homologous group of carboxylic acids having from one to ten carbon atoms. The boiling points increased with size in a regular manner, but the melting points did not. Unbranched acids made up of an even number of carbon atoms have melting points higher than the odd numbered homologs having one more or one less carbon. This reflects differences in intermolecular attractive forces in the crystalline state. In the table of fatty acids we see that the presence of a cis-double bond significantly lowers the melting point of a compound. Thus, palmitoleic acid melts over 60º lower than palmitic acid, and similar decreases occur for the C18 and C20 compounds. Again, changes in crystal packing and intermolecular forces are responsible.
The factors that influence the relative boiling points and water solubilities of various types of compounds were discussed earlier. In general, dipolar attractive forces between molecules act to increase the boiling point of a given compound, with hydrogen bonds being an extreme example. Hydrogen bonding is also a major factor in the water solubility of covalent compounds To refresh your understanding of these principles Click Here. The following table lists a few examples of these properties for some similar sized polar compounds (the non-polar hydrocarbon hexane is provided for comparison).
|
Formula |
IUPAC Name |
Molecular Weight |
Boiling Point |
Water Solubility |
|---|---|---|---|---|
| CH3(CH2)2CO2H | butanoic acid | 88 | 164 ºC | very soluble |
| CH3(CH2)4OH | 1-pentanol | 88 | 138 ºC | slightly soluble |
| CH3(CH2)3CHO | pentanal | 86 | 103 ºC | slightly soluble |
| CH3CO2C2H5 | ethyl ethanoate | 88 | 77 ºC | moderately soluble |
| CH3CH2CO2CH3 | methyl propanoate | 88 | 80 ºC | slightly soluble |
| CH3(CH2)2CONH2 | butanamide | 87 | 216 ºC | soluble |
| CH3CON(CH3)2 | N,N-dimethylethanamide | 87 | 165 ºC | very soluble |
| CH3(CH2)4NH2 | 1-aminobutane | 87 | 103 ºC | very soluble |
| CH3(CH2)3CN | pentanenitrile | 83 | 140 ºC | slightly soluble |
| CH3(CH2)4CH3 | hexane | 86 | 69 ºC | insoluble |
The first five entries all have oxygen functional groups, and the relatively high boiling points of the first two is clearly due to hydrogen bonding. Carboxylic acids have exceptionally high boiling points, due in large part to dimeric associations involving two hydrogen bonds. A structural formula for the dimer of acetic acid is shown here. When the mouse pointer passes over the drawing, an electron cloud diagram will appear. The high boiling points of the amides and nitriles are due in large part to strong dipole attractions, supplemented in some cases by hydrogen bonding.
Comparing the strengths of weak acids
The strengths of weak acids are measured on the pKa scale. The smaller the number on this scale, the stronger the acid is. Three of the compounds we shall be looking at, together with their pKa values are:
Remember - the smaller the number the stronger the acid. Comparing the other two to ethanoic acid, you will see that phenol is very much weaker with a pKa of 10.00, and ethanol is so weak with a pKa of about 16 that it hardly counts as acidic at all! The pKa of ethanol is about 17, while the pKa of acetic acid is about 5: this is a 1012-fold difference in the two acidity constants. In both compounds, the acidic proton is bonded to an oxygen atom. How can they be so different in terms of acidity? We begin by considering the conjugate bases.
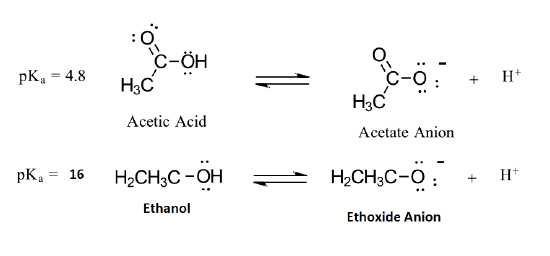
In both species, the negative charge on the conjugate base is held by an oxygen, so periodic trends cannot be invoked. For acetic acid, however, there is a key difference: a resonance contributor can be drawn in which the negative charge is localized on the second oxygen of the group. The two resonance forms for the conjugate base are equal in energy. What this means is that the negative charge on the acetate ion is not located on one oxygen or the other: rather it is shared between the two. Chemists use the term ‘delocalization of charge’ to describe this situation. In the ethoxide ion, by contrast, the negative charge is ‘locked’ on the single oxygen – it has nowhere else to go.
Recall the findamental idea that electrostatic charges, whether positive or negative, are more stable when they are ‘spread out’ than when they are confined to one atom. Here, a charge is being ‘spread out’ (in other words, delocalized) by resonance, rather than simply by the size of the atom involved.
The delocalization of charge by resonance has a very powerful effect on the reactivity of organic molecules, enough to account for the difference of over 12 pKa units between ethanol and acetic acid. The acetate ion is that much more stable than the ethoxide ion, all due to the effects of resonance delocalization.
Acidity of Carboxylic Acids
The pKa 's of some typical carboxylic acids are listed in the following table. When we compare these values with those of comparable alcohols, such as ethanol (pKa = 16) and 2-methyl-2-propanol (pKa = 19), it is clear that carboxylic acids are stronger acids by over ten powers of ten! Furthermore, electronegative substituents near the carboxyl group act to increase the acidity.
|
Compound |
pKa |
Compound |
pKa |
|
|---|---|---|---|---|
| HCO2H | 3.75 | CH3CH2CH2CO2H | 4.82 | |
| CH3CO2H | 4.74 | ClCH2CH2CH2CO2H | 4.53 | |
| FCH2CO2H | 2.65 | CH3CHClCH2CO2H | 4.05 | |
| ClCH2CO2H | 2.85 | CH3CH2CHClCO2H | 2.89 | |
| BrCH2CO2H | 2.90 | C6H5CO2H | 4.20 | |
| ICH2CO2H | 3.10 | p-O2NC6H4CO2H | 3.45 | |
| Cl3CCO2H | 0.77 | p-CH3OC6H4CO2H | 4.45 |
Why should the presence of a carbonyl group adjacent to a hydroxyl group have such a profound effect on the acidity of the hydroxyl proton? To answer this question we must return to the nature of acid-base equilibria and the definition of pKa , illustrated by the general equations given below.
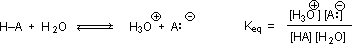
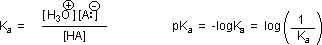
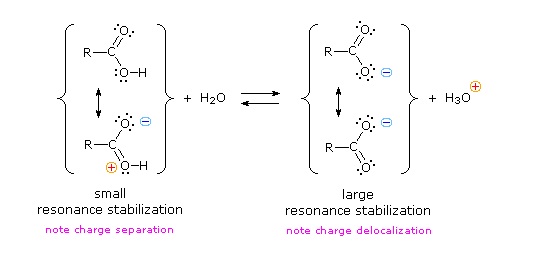
We know that an equilibrium favors the thermodynamically more stable side, and that the magnitude of the equilibrium constant reflects the energy difference between the components of each side. In an acid base equilibrium the equilibrium always favors the weaker acid and base (these are the more stable components). Water is the standard base used for pKa measurements; consequently, anything that stabilizes the conjugate base (A:(–)) of an acid will necessarily make that acid (H–A) stronger and shift the equilibrium to the right. Both the carboxyl group and the carboxylate anion are stabilized by resonance, but the stabilization of the anion is much greater than that of the neutral function, as shown in the following diagram. In the carboxylate anion the two contributing structures have equal weight in the hybrid, and the C–O bonds are of equal length (between a double and a single bond). This stabilization leads to a markedly increased acidity, as illustrated by the energy diagram displayed by clicking the "Toggle Display" button.
Exercises
Use acid-base chemistry and differences in water solubility to separate 1-octanol from octanoic acid using the following solutions: 1 M NaOH, ether, and 6 M HCl and any lab equipment.
- Answer
-

What percentage of propanoic acid (CH3CH2COOH) is dissociated into its conjugate base in a 0.20 M aqueous solution? Ka = 1.32 x 10⁻⁵
- Answer
-
From the acid equilibrium expression we get that:
[CH₃CH₂COO⁻][H3O⁺]/[CH₃CH₂COOH] = Ka = 1.32 x 10⁻⁵
[X][X]/[0.20 M - X] = 1.32 x 10⁻⁵
Assume X is small compared to 0.20 M
[X]2/[0.20 M] = 1.32 x 10⁻⁵
X = [CH₃CH₂COO⁻] = 1.62 x 10⁻3 M
[CH₃CH₂COO⁻] / [CH₃CH₂COOH] X 100 = %Disassociation
1.62 x 10⁻3 M / 0.20 M X 100 = 0.812%
Contributors and Attributions
Dr. Dietmar Kennepohl FCIC (Professor of Chemistry, Athabasca University)
Prof. Steven Farmer (Sonoma State University)
William Reusch, Professor Emeritus (Michigan State U.), Virtual Textbook of Organic Chemistry

