20.7: The Microstates of a Given Population Set
- Page ID
- 152763
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Thus far, we have considered only the probabilities associated with the assignments of distinguishable molecules to the allowed energy levels. In Section 20.2, we introduce the hypothesis that all of the \(g_i\) degenerate quantum states with energy \(\epsilon_i\) are equally probable, so that the probability that a molecule has energy \(\epsilon _i\) is \(P_i=P\left(\epsilon _i\right)=g_i\rho \left(\epsilon _i\right)\). Making this substitution, the total probability sum becomes
\[\begin{align*} 1&=\left(P_1+P_2+\dots +P_i+\dots \right)^N \\[4pt] &=\sum_{\left\{N_i\right\}}{\frac{N!}{N_1!N_2!\dots N_i!\dots }}P^{N_1}_1P^{N_2}_2\dots P^{N_i}_i\dots \\[4pt] &=\sum_{\left\{N_i\right\}}{\frac{N!g^{N_1}_1g^{N_2}_2\dots g^{N_i}_i\dots }{N_1!N_2!\dots N_i!\dots }}{\rho \left(\epsilon _1\right)}^{N_1}{\rho \left(\epsilon _2\right)}^{N_2}\dots {\rho \left(\epsilon _i\right)}^{N_i}\dots . \\[4pt] &=\sum_{\left\{N_i\right\}}{N!} \prod^{\infty}_{i=1} \left(\frac{g^{N_i}_i}{N_i!}\right) \rho \left(\epsilon_i\right)^{N_i} \\[4pt] &=\sum_{\left\{N_i\right\}} W\prod^{\infty }_{i=1}\rho \left(\epsilon _i\right)^{N_i} \end{align*} \nonumber \]
where we use the notation
\[a_1\times a_2\times \dots a_i\times \dots a_{\omega }\times =\prod^{\omega }_{i=1}{a_i} \nonumber \]
for extended products and introduce the function
\[\begin{align*} W &= W\left(N_i,g_i\right) \\[4pt] &=W\left(N_1,g_1,N_2,g_2,\dots ,N_i,g_i,\dots .\right) \\[4pt] &=N!\prod^{\infty }_{i=1}{\left(\frac{g^{N_i}_i}{N_i!}\right)} \\[4pt] &= C\left(N_1,N_2,\dots ,N_i,\dots \right)\prod^{\infty }_{i=1}{g^{N_i}_i} \end{align*} \nonumber \]
For reasons that become clear later, \(W\) is traditionally called the thermodynamic probability. This name is somewhat unfortunate, because \(W\) is distinctly different from an ordinary probability.
In Section 20.5, we note that \(P^{N_1}_1P^{N_2}_2\dots P^{N_i}_i\) is the probability that \(N_i\) molecules occupy each of the energy levels \(\epsilon _i\) and that \({N!}/{\left(N_1!N_2!\dots N_i!\dots \right)}\) is the number of combinations of distinguishable molecules that arise from the population set \(\{N_1,N_2,\dots ,N_i,\dots \}\). Now we observe that the extended product
\[{\rho \left(\epsilon _1\right)}^{N_1}{\rho \left(\epsilon _2\right)}^{N_2}\dots {\rho \left(\epsilon _i\right)}^{N_i}\dots . \nonumber \]
is the probability of any one assignment of the distinguishable molecules to quantum states such that \(N_i\) molecules are in quantum states whose energies are \(\epsilon _i\). Since a given molecule of energy \(\epsilon _i\) can be in any of the \(g_i\) degenerate quantum states, the probability that it is in the energy level \(\epsilon _i\) is \(g_i\)-fold greater that the probability that it is in any one of these quantum states.
Microstates
We call a particular assignment of distinguishable molecules to the available quantum states a microstate. For any population set, there are many combinations. When energy levels are degenerate, each combination gives rise to many microstates. The factor \({\rho \left(\epsilon _1\right)}^{N_1}{\rho \left(\epsilon _2\right)}^{N_2}\dots {\rho \left(\epsilon _i\right)}^{N_i}\dots .\) is the probability of any one microstate of the population set \(\{N_1,N_2,\dots ,N_i,\dots \}\). Evidently, the thermodynamic probability
\[W=N!\prod^{\infty }_{i=1}{\left(\frac{g^{N_i}_i}{N_i!}\right)} \label{micro} \]
is the total number of microstates of that population set.
To see directly that the number of microstates is dictated by Equation \ref{micro}, let us consider the number of ways we can assign \(N\) distinguishable molecules to the quantum states when the population set is \(\{N_1,N_2,\dots ,N_i,\dots \}\) and energy level \(\epsilon _i\) is \(g_i\)-fold degenerate. We begin by assigning the \(N_1\) molecules in energy level \(\epsilon _1\). We can choose the first molecule from among any of the \(N\) distinguishable molecules and can choose to place it in any of the \(g_1\) quantum states whose energy is \(\epsilon _1\). The number of ways we can make these choices is \({Ng}_1\). We can choose the second molecule from among the \(N-1\) remaining distinguishable molecules. In Boltzmann statistics, we can place any number of molecules in any quantum state, so there are again \(g_1\) quantum states in which we can place the second molecule. The total number of ways we can place the second molecule is \(\left(N-1\right)g_1\).
The number of ways the first and second molecules can be chosen and placed is therefore \(N\left(N-1\right)g^2_1\). We find the number of ways that successive molecules can be placed in the quantum states of energy \(\epsilon _1\) by the same argument. The last molecule whose energy is \(\epsilon _1\) can be chosen from among the \(\left(N-N_1+1\right)\) remaining molecules and placed in any of the \(g_1\) quantum states. The total number of ways of placing the \(N_1\) molecules in energy level \(\epsilon _1\) is \(N\left(N-1\right)\left(N-2\right)\dots \left(N-N_1+1\right)g^{N_1}_1\).
This total includes all possible orders for placing every set of \(N_1\) distinguishable molecules into every possible set of quantum states. However, the order doesn’t matter; the only thing that affects the state of the system is which molecules go into which quantum state. (When we consider all of the ways our procedure puts all of the molecules into any of the quantum states, we find that any assignment of molecules \(A\), \(B\), and \(C\) to any particular set of quantum states occurs six times. Selections in the orders \(A\),\(B\),\(C\); \(A\),\(C\),\(B\); \(B\),\(A\),\(C\); \(B\),\(C\),\(A\); \(C\),\(A\),\(B\); and \(C\),\(B\),\(A\) all put the same molecules in the same quantum states.) There are \(N_1!\) orders in which our procedure chooses the \(N_1\) molecules; to correct for this, we must divide by \(N_1!\), so that the total number of assignments we want to include in our count is
\[N\left(N-1\right)\left(N-2\right)\dots \left(N-N_1+1\right)g^{N_1}_1/N_1! \nonumber \]
The first molecule that we assign to the second energy level can be chosen from among the \(N-N_1\) remaining molecules and placed into any of the \(g_2\) quantum states whose energy is \(\epsilon _2\). The last one can be chosen from among the remaining \(\left(N-N_1-N_2+1\right)\) molecules. The number of assignments of the \(N_2\) molecules to \(g_2\)-fold degenerate quantum states whose energy is \(\epsilon _2\) is
\[\left(N-N_1\right)\left(N-N_1-1\right)\dots \left(N-N_1-N_2+1\right)g^{N_2}_2/N_2! \nonumber \]
When we consider the number of assignments of molecules to quantum states with energies \(\epsilon _1\) and \(\epsilon _2\) we have
\[N\left(N-1\right)\dots \left(N-N_1+1\right)\left(N-N_1\right)\left(N-N_1-1\right)\dots \nonumber \] \[\times \left(N-N_1-N_2+1\right)\left(\frac{g^{N_1}_1}{N_1!}\right)\left(\frac{g^{N_2}_2}{N_2!}\right) \nonumber \]
Let the last energy level to contain any molecules be \(\epsilon _{\omega }\). The number of ways that the \(N_{\omega }\) molecules can be assigned to the quantum states with energy \(\epsilon _{\omega }\) is \(N_{\omega }\left(N_{\omega }-1\right)\dots \left(1\right)g^{N_{\omega }}_{\omega }/N_{\omega }!\) The total number of microstates for the population set \(\{N_1,N_2,\dots ,N_i,\dots \}\) becomes
\[N\left(N-1\right)\dots \left(N-N_1\right)\left(N-N_1-1\right)\dots \nonumber \] \[\times \left(N_{\omega }\right)\left(N_{\omega }-1\right)\dots \left(1\right)\prod^{\infty }_{i=1}{\left(\frac{g^{N_i}_i}{N_i!}\right)}=N!\prod^{\infty }_{i=1}{\left(\frac{g^{N_i}_i}{N_i!}\right)} \nonumber \]
When we consider Fermi-Dirac and Bose-Einstein statistics, it is no longer true that the molecules are distinguishable. For Fermi-Dirac statistics, no more than one molecule can be assigned to a particular quantum state. For a given population set, Boltzmann, Fermi-Dirac, and Bose-Einstein statistics produce different numbers of microstates.
It is helpful to have notation that enables us to specify different combinations and different microstates. If \(\epsilon _i\) is the energy associated with the wave equation that describes a particular molecule, it is convenient to say that the molecule is in energy level \(\epsilon _i\); that is, its quantum state is one of those that has energy \(\epsilon _i\). Using capital letters to represent molecules, we indicate that molecule \(A\) is in energy level \(\epsilon _i\) by writing \(\epsilon _i\left(A\right)\). To indicate that \(A\), \(B\), and \(C\) are in \(\epsilon _i\), we write \(\epsilon _i\left(A,B,C\right)\). Similarly, to indicate that molecules \(D\) and \(E\) are in \(\epsilon _k\), we write \(\epsilon _k\left(D,E\right)\). For this system of five molecules, the assignment \(\epsilon _i\left(A,B,C\right)\epsilon _k\left(D,E\right)\) represents one of the possible combinations. The order in which we present the molecules that have a given energy is immaterial: \(\epsilon _i\left(A,B,C\right)\epsilon _k\left(D,E\right)\) and \(\epsilon _i\left(C,B,A\right)\epsilon _k\left(E,D\right)\) represent the same combination. When any one molecule is distinguishable from others of the same substance, assignments in which a given molecule has different energies are physically different and represent different combinations. The assignments \(\epsilon _i\left(A,B,C\right)\epsilon _k\left(D,E\right)\) and \(\epsilon _i\left(D,B,C\right)\epsilon _k\left(A,E\right)\) represent different combinations. In Figure 2, we represent these assignments more schematically.
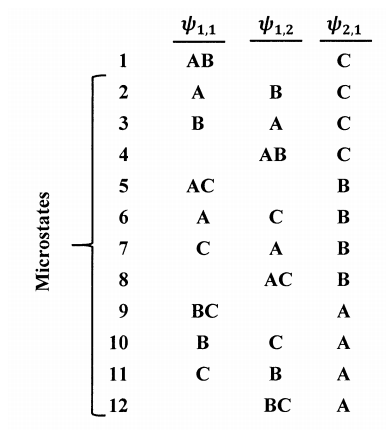
Any two assignments in which a particular molecule occupies different quantum states give rise to different microstates. If the \(i^{th}\) energy level is three-fold degenerate, a molecule in any of the quantum states \({\psi }_{i,1}\), \({\psi }_{i,2}\), or \({\psi }_{i,3}\) has energy \(\epsilon _i\). Let us write
\[{\psi }_{i,1}\left(A,B\right){\psi }_{i,2}\left(C\right){\psi }_{k,1}\left(DE\right) \nonumber \]
to indicate the microstate arising from the combination \(\epsilon _i\left(A,B,C\right)\epsilon _k\left(D,E\right)\) in which molecules \(A\) and \(B\) occupy \({\psi }_{i,1}\), molecule \(C\) occupies \({\psi }_{i,2}\), and molecules \(D\) and \(E\) occupy \({\psi }_{k,1}\). Then,
\[{\psi }_{i,1}\left(A,B\right){\psi }_{i,2}\left(C\right){\psi }_{k,1}\left(DE\right) \nonumber \] \[{\psi }_{i,1}\left(B,C\right){\psi }_{i,2}\left(A\right){\psi }_{k,1}\left(DE\right) \nonumber \] \[{\psi }_{i,1}\left(A\right){\psi }_{i,2}\left(B,C\right){\psi }_{k,1}\left(DE\right) \nonumber \]
are three of the many microstates arising from the combination \(\epsilon _i\left(A,B,C\right)\epsilon _k\left(D,E\right)\). Figure 3 shows all of the microstates possible for the population set \(\{2,1\}\) when the quantum states of a molecule are \({\psi }_{1,1}\), \({\psi }_{1,2}\), and \({\psi }_{2,1}\).