17.16: The Nernst Equation for Half-cells
- Page ID
- 152733
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)If the S.H.E. is one of the half-cells, the corresponding Nernst equation can be viewed as a description of the other half-cell. Using the cell in which the silver–silver ion electrode opposes the S.H.E., as in the preceding example, the cell potential is the algebraic sum of the potential of the silver terminal and the potential of the platinum terminal. We can represent the potential of the silver–silver ion electrode as \({\mathcal{E}}_{Ag\mid {Ag}^+}\). Since the S.H.E. is always at standard conditions, its potential, which we can represent as \({\mathcal{E}}^o_{Pt\mid H_2\mid H^+}\), is zero by definition. The cell potential is
\[\mathcal{E}={\mathcal{E}}_{Ag\mid {Ag}^+}+{\mathcal{E}}^o_{Pt\mid H_2\mid H^+} \nonumber \]
The potential of the cell with both half-cells at standard conditions is
\[{\mathcal{E}^o={\mathcal{E}}^o_{Ag\mid {Ag}^+}+\mathcal{E}}^o_{Pt\mid H_2\mid H^+} \nonumber \]
and, again since the S.H.E. is at standard conditions, \({\tilde{a}}_{H^+}=1\) and \(P_{H_2}=1\). Substituting into the Nernst equation for the full cell, we have
\[\mathcal{E}_{Ag\mid {Ag}^+}+ \mathcal{E}^o_{Pt\mid H_2\mid H^+}= \mathcal{E}^o_{Ag\mid Ag^+}+\mathcal{E}^o_{Pt\mid H_2\mid H^+}-\frac{RT}{\mathcal{F}} \ln \frac{1}{\tilde{a}_{Ag}^+} \nonumber \]
or
\[\mathcal{E}_{Ag\mid {Ag}^+}= \mathcal{E}^o_{Ag\mid {Ag}^+}-\frac{RT}{\mathcal{F}} \ln \frac{1}{\tilde{a}_{Ag^+}} \nonumber \]
where the algebraic signs of \(\mathcal{E}_{Ag\mid {Ag}^+}\) and \(\mathcal{E}^o_{Ag\mid {Ag}^+}\) correspond to writing the half-reaction in the direction \(Ag^++e^-\to Ag^0\). Note that this is precisely the equation that we would obtain by writing out the Nernst equation corresponding to the chemical equation \(Ag^++e^-\to Ag^0\).
To see how these various conventions work together, let us consider the oxidation of hydroquinone \(\left(H_2Q\right)\) to quinone \(\left(Q\right)\) by ferric ion in acidic aqueous solutions:
\[2\ {Fe}^{3+}+H_2Q\rightleftharpoons \ 2\ {Fe}^{2+}+Q+2H^+ \nonumber \]
The quinone–hydroquinone couple is
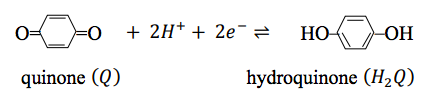
and the ferric ion–ferrous ion couple is
\[Fe^{3+}+e^-\rightleftharpoons Fe^{2+} \nonumber \]
The standard electrode potentials are \(\mathcal{E}_{Pt\mid Q,H_2Q,H^+}=+0.699\ \mathrm{v}\) and \(\mathcal{E}_{Pt\mid Fe^{3+},Fe^{2+}}=+0.783\ \mathrm{v}\). In each case, the numerical value is the potential of a full cell in which the other electrode is the S.H.E. The algebraic sign of the half-cell potential is equal to the sign of the half-cell’s electrical potential when it operates versus the S.H.E.
To carry out this reaction in an electrochemical cell, we can use a salt bridge to join a \(Pt\mid Fe^{3+},Fe^{2+}\) cell to a \(Pt\mid Q,H_2Q,H^+\) cell. To construct a standard \(Pt\mid Fe^{3+},Fe^{2+}\) cell, we need only insert a platinum wire into a solution containing ferric and ferrous ions, both at unit activity. To construct a standard \(Pt\mid Q,H_2Q,H^+\) cell, we insert a platinum wire into a solution containing quinone, hydroquinone, and hydronium ion, all at unit activity. For standard half-cells, the cathode and anode reactions are
\[Fe^{3+}+e^-\rightleftharpoons Fe^{2+} \nonumber \]
and
\[H_2Q\rightleftharpoons Q+2H^++2e^- \nonumber \]
We can immediately write the Nernst equation for each of these half-reactions as
\[\mathcal{E}_{Pt\mid Fe^{3+},Fe^{2+}}=\mathcal{E}^o_{Pt\mid Fe^{3+},Fe^{2+}}-\frac{RT}{\mathcal{F}} \ln \frac{\tilde{a}_{Fe^{2+}}}{\tilde{a}_{Fe^{3+}}} \nonumber \]
and
\[\left(-\mathcal{E}_{Pt\mid Q,H_2Q,H^+}\right)=\left(- \mathcal{E}^o_{Pt\mid Q,H_2Q,H^+}\right)-\frac{RT}{\mathrm{2}\mathcal{F}} \ln \frac{\tilde{a}_Q \tilde{a}^2_{H^+}}{\tilde{a}_{H_2Q}} \nonumber \]
If we add the equations for these half-reactions, the result does not correspond to the original full-cell reaction, because the number of electrons does not cancel. This can be overcome by multiplying the ferric ion–ferrous ion half-reaction by two. What do we then do about the corresponding half-cell Nernst equation? Clearly, the values of \({\mathcal{E}}_{Pt\mid {Fe}^{3+},{Fe}^{2+}}\) and \({\mathcal{E}}^o_{Pt\mid {Fe}^{3+},{Fe}^{2+}}\) do not depend on the stoichiometric coefficients in the half-reaction equation. However, the activity terms in the logarithm’s argument do, as does the number of electrons taking part in the half-reaction. We have
\[2Fe^{3+}+2e^-\rightleftharpoons 2Fe^{2+} \nonumber \]
with
\[\begin{aligned} \mathcal{E}_{Pt\mid Fe^{3+},Fe^{2+}} & = \mathcal{E}^o_{Pt\mid Fe^{3+},Fe^{2+}}-\frac{RT}{\mathrm{2}\mathcal{F}} \ln \frac{\tilde{a}^2_{Fe^{2+}}}{\tilde{a}^2_{Fe^{3+}}} \\ ~ & =\mathcal{E}^o_{Pt\mid Fe^{3+},Fe^{2+}}-\frac{RT}{\mathcal{F}} \ln \frac{\tilde{a}_{Fe^{2+}}}{\tilde{a}_{Fe^{3+}}} \end{aligned} \nonumber \]
We see that we can apply any factor we please to the half-reaction. The Nernst equation gives the same dependence of the half-cell potential on reagent concentrations no matter what factor we choose. This is true also of the Nernst equation for any full-cell reaction. In the present example, adding the appropriate half-cell equations and their corresponding Nernst equations gives
\[2\ Fe^{3+}+H_2Q\rightleftharpoons \ 2\ Fe^{2+}+Q+2H^+ \nonumber \]
and
\[ \begin{aligned} \mathcal{E} & = \mathcal{E}_{Pt\mid Fe^{3+},Fe^{2+}}- \mathcal{E}_{Pt\mid Q,H_2Q,H^+} \\ ~ & = \mathcal{E}^o_{Pt\mid Fe^{3+},Fe^{2+}}- \mathcal{E}^o_{Pt\mid Q,H_2Q,H^+}-\frac{RT}{\mathrm{2}\mathcal{F}} \ln \frac{\tilde{a}^2_{Fe^{2+}}}{\tilde{a}^2_{Fe^{3+}}} -\frac{RT}{\mathrm{2}\mathcal{F}} \ln \frac{\tilde{a}_Q \tilde{a}^2_{H^+}}{\tilde{a}_{H_2Q}} \\ ~ & =\mathcal{E}^0-\frac{RT}{\mathrm{2}\mathcal{F}} \ln \frac{\tilde{a}_Q \tilde{a}^2_{H^+} \tilde{a}^2_{Fe^{2+}}}{\tilde{a}_{H_2Q} \tilde{a}^2_{Fe^{3+}}} \end{aligned} \nonumber \]


