Particle on a Ring
- Page ID
- 1727
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)- To be familiar with a quantum system with angular symmetry.
- To be introduced to quantum angular momentum
The case of a quantum particle confined a one-dimensional ring is similar to the particle in a 1D box. Consider a variant of the one-dimensional particle in a box problem in which the x-axis is bent into a ring of radius \(R\). We can write the same Schrödinger equation
\[ \dfrac{-\hbar^2}{2m} \dfrac{d^2 \psi(x)}{dx^2} = E \psi(x) \label{1} \]
There are no boundary conditions in this case since the x-axis closes upon itself. A more appropriate independent variable for this problem is the angular position on the ring given by, \( \phi = x {/} R \). The Schrödinger equation would then read
\[ -\dfrac{\hbar^2}{2mR^2} \dfrac{d^2 \psi (\phi)} {d (\phi)^2} = E \psi (\phi) \label{2} \]
The kinetic energy of a body rotating in the xy-plane can be expressed as
\[ E = \dfrac{L_z^2}{2I} \label{3} \]
where \(I = mR^2\) is the moment of inertia and \( L_z\), the z-component of angular momentum. (Since \( L = r \times p\), if r and p lie in the xy-plane, L points in the z-direction.) The structure of Equation \ref{2} suggests that this angular-momentum operator is given by
\[ \hat{L_z} = -{i} \hbar \dfrac{\partial}{\partial \phi} \label{4} \]
This result will follow from a more general derivation elsewhere. The Schrödinger Equation (Equation \ref{2}) can now be written more compactly as
\[ \psi \prime \prime \ (\phi) + m^2 \psi (\phi) = 0 \label{5} \]
where
\[m^2 \equiv 2IE/ \hbar^2\label{6} \]
Do not confuse the variable \(m\) with the mass of the particle!
Possible solutions to Equation \ref{5} are
\[ \psi (\phi) = \text{const}\, e^{\pm{i}m\phi} \label{7} \]
For this wavefunction to be physically acceptable, it must be single-valued. Since \(\phi \) increased by any multiple of 2\(\pi \) represents the same point on the ring, we must have
\[ \psi (\phi + 2\pi ) = \psi (\phi) \label{8} \]
and therefore
\[e^{{i}m (\phi + 2\pi)} = e^{{i}m \phi} \label{9} \]
This requires that
\[e^{2\pi {i}m} = 1 \label{10} \]
which is true only if m is an integer:
\[ m = 0, \pm 1, \pm 2... \nonumber \]
Using Equation \ref{6}, this gives the quantized energy values
\[E_m = \dfrac{\hbar^2}{2I} m^2 \nonumber \]
In contrast to the particle in a box, the eigenfunctions corresponding to \(+m \) and \(-m \) (Equation \ref{7}) are linearly independent, so both must be accepted. Therefore all eigenvalues, except \(E_0 \), are two-fold (or doubly) degenerate. The eigenfunctions can all be written in the form const \( e^{{i}m \phi} \), with \(m\) allowed to take either positive and negative values (or 0), as in Equation \ref{10}. The normalized eigenfunctions are
\[ {\psi _{m}} (\phi) = \dfrac{1}{\sqrt{2 \pi}} e^{im\phi} \nonumber \]
and can be verified to satisfy the normalization condition containing the complex conjugate
\[ \int\limits_{0}^{2\pi} {\psi_{m}^*} (\phi) {\psi _{m}} (\phi) d\phi = 1 \nonumber \]
where we have noted that \( {\psi_{m}^*} (\phi) = (2\pi)^{-1/2} e^{-{i}m\phi} \). The mutual orthogonality of the functions also follows easily, for
\[ \begin{align} \int\limits_{0}^{2\pi} {\psi_{m^\prime}^*} {\psi _{m}} (\phi) d\phi &= \dfrac{1}{2\pi} \int\limits_{0}^{2\pi} e^{{i}(m-m^\prime) \phi} d\phi \\[4pt] &= \dfrac{1}{2\pi} \int\limits_{0}^{2\pi} [\cos(m-m^\prime)\phi + {i} \sin(m-m^\prime)\phi] d\phi =0 \end{align} \nonumber \]
for \( m^\prime \neq m \).
The solutions of Equation \ref{2} are also eigenfunctions of the angular momentum operator (Equation \ref{4}), with
\[ \hat{L_z} \psi_{m} (\phi) = m\hbar \psi_{m} (\phi), m = 0, \pm 1, \pm 2... \nonumber \]
This is a instance of a fundamental result in quantum mechanics, that any measured component of orbital angular momentum is restricted to integral multiples of \( \hbar \). The Bohr theory of the hydrogen atom can be derived from this principle alone.
The benzene molecule consists of a ring of six carbon atoms around which six delocalized pi-electrons can circulate. A variant of the FEM for rings predicts the ground-state electron configuration which we can write as \( 1\pi^{2} 2\pi^{4} \), as shown here:
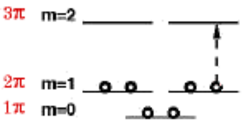
The enhanced stability the benzene molecule can be attributed to the complete shells of \(\pi \)-electron orbitals, analogous to the way that noble gas electron configurations achieve their stability. Naphthalene, apart from the central C-C bond, can be modeled as a ring containing 10 electrons in the next closed-shell configuration\( 1\pi^{2} 2\pi^{4} 3\pi^{4} \). These molecules fulfill Hückel's "4N+2 rule" for aromatic stability. The molecules cyclobutadiene \( {(1\pi^{2} 2\pi^{2})} \) and cyclooctatetraene\( {(1\pi^{2} 2\pi^{4} 3\pi^{2})} \) , even though they consist of rings with alternating single and double bonds, do not exhibit aromatic stability since they contain partially-filled orbitals.
The longest wavelength absorption in the benzene spectrum can be estimated according to this model as
\[ \dfrac{hc}{\lambda} = E_2 - E_1 = \dfrac{\hbar^2}{2mR^2} {(2^2 -1^2)} \nonumber \]
The ring radius R can be approximated by the C-C distance in benzene, 1.39 Å. We predict \( \lambda \approx \) 210 nm, whereas the experimental absorption has \( \lambda_{max} \approx \) 268 nm.
Angular Momentum
One type of rotational motion in quantum mechanics is a particle in a ring. An important aspect of this is the angular momentum J which includes a vector with a direction that shows axis of rotation1. The particle’s magnitude of angular momentum that is traveling along a circular path of radius \(r\) is classified as \(J=p \times r\) where \(p\) is the linear momentum at any moment. When the particle of mass m travels in the horizontal radius \(r\), the particle has purely kinetic energy since potential energy is constant and is set to zero everywhere. The energy with regards of angular momentum can be expressed as:
\[E = \dfrac{J^2_z}{2mr^2} \label{1C} \]
- \(J\) is the angular momentum in z-axis and
- \(mr^2\) is the particle's moment of inertia I on the z-axis.
A particle has a moment of inertia I when traveling along a circular path. I is defined by m (mass) multiplied by \(r^2\) (radius squared). The heavier particle in the top picture has a large moment of inertia on the central point while the lighter particle in the lower picture has a smaller moment of inertia while traveling on the path of the same radius1. For the heavier particle, the I is large and therefore, the particle's energy can be expressed by:
\[E = \dfrac{J^2_z}{2I} \label{2C} \]
We then use the de Broglie equation to quantize the energy of rotation. This is done by expressing the angular momentum in wavelengths:
\[J_z = pr = \dfrac{hr}{\lambda} \label{3C} \]
where p is the linear momentum and h is Planck's constant (\(6.626 \times 10^{-34}~ \textrm{J s}\)). It can also be written:
\[\lambda = \dfrac{h}{p} \nonumber \]
With this equation, de Broglie postulated that there is a wave correlated with the electron via wavelength. He had done this to explain Bohr's model of the Hydrogen atom, in which the electron is only allowed permitted to orbit from the nucleus at certain distances.
References
- Atkins, Peter, and Julio de Paula. Physical Chemistry for the Life Sciences. New York. Oxford University Press. 2006, p 358.

