The Effect of Changing Conditions
- Page ID
- 3842
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\dsum}{\displaystyle\sum\limits} \)
\( \newcommand{\dint}{\displaystyle\int\limits} \)
\( \newcommand{\dlim}{\displaystyle\lim\limits} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)This page looks at the relationship between equilibrium constants and Le Chatelier's Principle. Students often get confused about how it is possible for the position of equilibrium to change as you change the conditions of a reaction, although the equilibrium constant may remain the same.
Changing concentrations
Equilibrium constants are not changed if you change the concentrations of things present in the equilibrium. The only thing that changes an equilibrium constant is a change of temperature. The position of equilibrium is changed if you change the concentration of something present in the mixture. According to Le Chatelier's Principle, the position of equilibrium moves in such a way as to tend to undo the change that you have made.
Suppose you have an equilibrium established between four substances A, B, C and D.
\[ A + 2B \rightleftharpoons C + D\]
According to Le Chatelier's Principle, if you decrease the concentration of C, for example, the position of equilibrium will move to the right to increase the concentration again.
Explanation in terms of the constancy of the equilibrium constant
The equilibrium constant, \(K_c\) for this reaction looks like this:
\[ K_c = \dfrac{[C][D]}{[A][B]^2}\]
If you have moved the position of the equilibrium to the right (and so increased the amount of \(C\) and \(D\)), why hasn't the equilibrium constant increased? This is actually the wrong question to ask! We need to look at it the other way round.
Let's assume that the equilibrium constant must not change if you decrease the concentration of \(C\) - because equilibrium constants are constant at constant temperature. Why does the position of equilibrium move as it does?
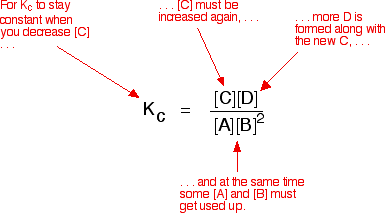
If you decrease the concentration of \(C\), the top of the \(K_c\) expression gets smaller. That would change the value of \(K_c\). In order for that not to happen, the concentrations of \(C\) and \(D\) will have to increase again, and those of \(A\) and \(B\) must decrease. That happens until a new balance is reached when the value of the equilibrium constant expression reverts to what it was before. The position of equilibrium moves - not because Le Chatelier says it must - but because of the need to keep a constant value for the equilibrium constant.
If you decrease the concentration of \(C\):
Changing pressure
This only applies to systems involving at least one gas. Equilibrium constants are not changed if you change the pressure of the system. The only thing that changes an equilibrium constant is a change of temperature. The position of equilibrium may be changed if you change the pressure. According to Le Chatelier's Principle, the position of equilibrium moves in such a way as to tend to undo the change that you have made.
That means that if you increase the pressure, the position of equilibrium will move in such a way as to decrease the pressure again - if that is possible. It can do this by favoring the reaction which produces the fewer molecules. If there are the same number of molecules on each side of the equation, then a change of pressure makes no difference to the position of equilibrium.
Case 1: Differing Numbers of Gaseous Species on each side of the Equation
Let's look at the same equilibrium we've used before. This one would be affected by pressure because there are three molecules on the left, but only two on the right. An increase in pressure would move the position of equilibrium to the right.
\[A_{(g)} + 2B_{(g)} \rightleftharpoons C_{(g)} + D_{(g)} \]
Because this is an all-gas equilibrium, it is much easier to use \(K_p\):
\[K_p = \dfrac{P_c\;P_D}{P_A\;P_B^2} \label{EqC1}\]
Once again, it is easy to suppose that, because the position of equilibrium will move to the right if you increase the pressure, \(K_p\) will increase as well. Not so! To understand why, you need to modify the \(K_p\) expr ession. Remember the relationship between partial pressure, mole fraction and total pressure?
\[ P_A = (\text{mole fraction of A} )( \text{total pressure})\]
\[P_A = \chi_A P_{tot}\]
Replacing all the partial pressure terms in \(\ref{EqC1}\) by mole fractions\( \chi_A \) and total pressure (\(P_{tot}\)) g ives you this:
\[ K_p \dfrac{(\chi_C P_{tot} )( \chi_D P_{tot} )}{(\chi_A P_{tot} )(\chi_B P_{tot})^2}\]
Most of the "P"s cancel out, with one left at the bottom of the expression.
\[ K_p \dfrac{\chi_C \chi_D}{\chi_A\chi_B^2 P_{tot}}\]
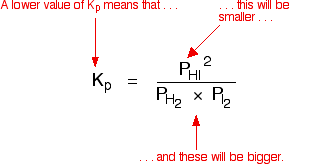
Now, remember that \(K_p\) has got to stay constant because the temperature is unchanged. How can that happen if you increase P? To compensate, you would have to increase the terms on the top, \(\chi_C\) and \(\chi_D\), and decrease the terms on the bottom, \(\chi_A\) and \(\chi_B\).
Increasing the terms on the top means that you have increased the mole fractions of the molecules on the right-hand side. Decreasing the terms on the bottom means that you have decreased the mole fractions of the molecules on the left. That is another way of saying that the position of equilibrium has moved to the right - exactly what Le Chatelier's Principle predicts. The position of equilibrium moves so that the value of \(K_p\) is kept constant.
Case 2: Same Numbers of Gaseous Species on each side of the Equation
There are the same numbers of molecules on each side of the equation. In this case, the position of equilibrium is not affected by a change of pressure. Why not?
\[A_{(g)} + B_{(g)} \rightleftharpoons C_{(g)} + D_{(g)} \]
Let's go through the same process as in Case 1:
\[ K_p = \dfrac{ P_C P_D}{P_A P_B}\]
Substituting mole fractions and total pressure:
\[ K_p = \dfrac{ (\chi_C P_{tot}) (\chi_D P_{tot}) }{ (\chi_A P_{tot}) (\chi_B P_{tot})}\]
Cancelling out as far as possible:
\[ K_p = \dfrac{ \chi_C \chi_D }{ \chi_A \chi_B }\]
There is not a single \(P_{tot}\) left in the expression so changing the pressure makes no difference to the \(K_p\) expression. The position of equilibrium doesn't need to move to keep \(K_p\) constant.
Changing temperature
Equilibrium constants are changed if you change the temperature of the system. \(K_c\) or \(K_p\) are constant at constant temperature, but they vary as the temperature changes. Look at the equilibrium involving hydrogen, iodine and hydrogen iodide:
\[ H_{2(g)} + I_{2(g)} \rightleftharpoons 2HI_{(g)} \label{EqHI}\]
with \(\Delta H = -10.4\; kJ/mol\). The \(K_p\) expression is:
\[ P_p =\dfrac{P_{HI}^2}{P_{H_2}P_{(I_2)}}\]
Two values for \(K_p\) a re:
| temperature | Kp |
|---|---|
| 500 K | 160 |
| 700 K | 54 |
You can see that as the temperature increases, the value of \(K_p\) falls. This is typical of what happens with any equilibrium where the forward reaction is exothermic. Increasing the temperature decreases the value of the equilibrium constant. Where the forward reaction is endothermic, increasing the temperature increases the value of the equilibrium constant.
The position of equilibrium also changes if you change the temperature. According to Le Chatelier's Principle, the position of equilibrium moves in such a way as to tend to undo the change that you have made. If you increase the temperature, the position of equilibrium will move in such a way as to reduce the temperature again. It will do that by favoring the reaction which absorbs heat. In the equilibrium we've just looked at (\(\ref{EqHI}\), that will be the back reaction because the forward reaction is exothermic.
So, according to Le Chatelier's Principle the position of equilibrium will move to the left with increasing temperature. Less hydrogen iodide will be formed, and the equilibrium mixture will contain more unreacted hydrogen and iodine. That is entirely consistent with a fall in the value of the equilibrium constant.
Equilibrium constants are not changed if you add (or change) a catalyst. The only thing that changes an equilibrium constant is a change of temperature. The position of equilibrium is not changed if you add (or change) a catalyst. A catalyst speeds up both the forward and back reactions by exactly the same amount. Dynamic equilibrium is established when the rates of the forward and back reactions become equal. If a catalyst speeds up both reactions to the same extent, then they will remain equal without any need for a shift in position of equilibrium.
Contributors and Attributions
Jim Clark (Chemguide.co.uk)


