Kc
- Page ID
- 3840
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)This page defines the equilibrium constant and introduces the equilibrium constant expressed in terms of concentrations, Kc. It assumes familiarity with the concept of dynamic equilibrium, as well as the terms "homogeneous" and "heterogeneous" as applied to chemical reactions. The two types of dynamic equilibria (homogeneous and heterogeneous) are discussed separately below, because the equilibrium constants are defined differently.
- A homogeneous equilibrium is one in which all species are present in the same phase. Common examples include gas-phase or solution reactions.
- A heterogeneous equilibrium is one in which species exist in more than one phase. Common examples include reactions involving solids and gases, or solids and liquids.
Homogeneous equilibria
This is the more straightforward case. It applies where everything in the equilibrium mixture is in the same phase. An example of a gaseous homogeneous equilibrium is the conversion of sulfur dioxide to sulfur trioxide at the heart of the Contact Process:
\[2SO_{2(g)} + O_{2(g)} \rightleftharpoons 2SO_{3(g)} \tag{1}\]
A commonly used liquid example is the esterification reaction between an organic acid and an alcohol, as in the example below:
\[ CH_3COOH_{(l)} + CH_3CH_2OH_{(l)} \rightleftharpoons CH_3COOCH_2CH_{3(l)} + H_2O_{(l)}\tag{2}\]
Writing an expression for \(K_c\)
Consider the general equilibrium reaction:
\[ aA + bB \rightleftharpoons cC + dD\tag{3}\]
No state symbols are specified, but the reaction is assumed to be homogeneous here. The measured equilibrium concentrations of the reactants and products are used to calculate the equilibrium constant, as shown in the figure below. The equilibrium constant always has the same value (provided the temperature does not change), irrespective of the initial concentrations of A, B, C and D. It is also unaffected by a change in pressure or the presence (or absence) of a catalyst.
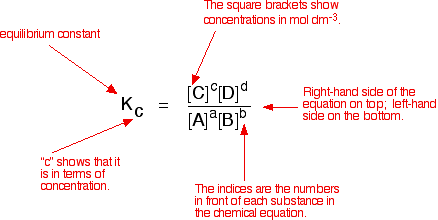
Compare this with the chemical equation for the equilibrium. The convention is that the substances on the right side of the equation are written in the numerator of the Kc expression, and those on the left side in the denominator. The exponents are the coefficients of the reactants and products from the equation.
A typical esterification reaction is given below:
\[ CH_3COOCH_2CH_{3(l)} + H_2O_{(l)} \rightleftharpoons CH_3COOH_{(l)} + CH_3CH_2OH_{(l)}\]
There is only one molecule of each reactant or product involved in the reaction, so all the exponents in the equilibrium constant expression are "1," and need not be included in the \(K_c\) ex pression, shown below:
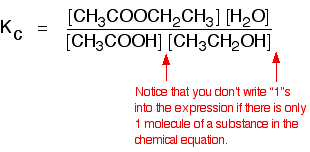
As long as the temperature is held constant, \(K_c\) has a constant value regardless of the proportions of acid and alcohol used. At room temperature, this value is approximately 4 for this reaction.
This is the reverse of the previous esterification reaction:
\[ CH_3COOCH_2CH_{3(l)} + H_2O_{(l)} \rightleftharpoons CH_3COOH_{(l)} + CH_3CH_2OH_{(l)}\]
The \(K_c\) ex pression is written as follows:
\[ K_c = \dfrac{[CH_3COOH][CH_3CH_2OH]}{[CH_3COOCH_2CH_3][H_2O]}\]
Compared with the previous \(K_c\), this expressio n is simply inverted. Its value at room temperature is approximately 1/4 (0.25).
It is helpful to write down the chemical equation for a equilibrium reaction whenever discussing an equilibrium constant, to ensure the expression is written correctly (products over reactants).
Recall that the equation for this process is as follows:
\[ 2SO_{2(g)} + O_2 {(g)} \rightleftharpoons 2SO_{3(g)} \tag{4}\]
In this case, the \(K_c\) expressio n includes some nontrivial exponents:
\[ K_c = \dfrac{[SO_3]^2}{[SO_2]^2[O_2]} \tag{5}\]
Although everything is present as a gas, because \(K_c\) is used he re, the activities are measured in mol dm-3. There is another equilibrium constant, \(K_p\), that i s more frequently used for gases.
The chemical equation for the Haber process is given below:
\[ N_{2(g)} + 3H_{2(g)} \rightleftharpoons 2NH_{3(g)} \tag{6}\]
The \(K_c\) expression is as follows:
\[ K_c = \dfrac{[NH_3]^2}{[N_2][H_2]^3} \tag{7}\]
\(K_c\) in heterogeneous Equilibria
An examples of a heterogeneous equilibrium is the equilibrium established if steam is in contact with red hot carbon. In this reaction, a gas reacts with a solid:
\[ H_2O_{(g)} + C_{(s)} \rightleftharpoons H_{2(g)} + CO_{(g)} \tag{8}\]
Another heterogeneous equilibrium involves shaking copper with silver nitrate solution; this reaction involves solids and aqueous ions:
\[ Cu_{(s)} + 2Ag^+_{(aq)} \rightleftharpoons Cu^{2+}_{(aq)} + 2Ag_{(s)} \tag{9}\]
The important consideration for a heterogeneous equilibrium is that there are no solid terms in an equilibrium constant expression; therefore, not every reactant or product will be accounted in \(K_c\).
\[ H_2O_{(g)} + C_{(s)} \rightleftharpoons H_{2(g)} + CO_{(g)}\]
The equilibrium constant expression is written the same way as in previous examples, omitting the solid carbon term:
\[ K_c = \dfrac{[H_2][CO]}{[H_2O]}\]
Consider the redox reaction between solid copper and silvir ions in solution:
\[ Cu_(s)+ + 2Ag^+_{(aq)} \rightleftharpoons Cu^{2+}_{(aq)} + 2Ag_{(s)}\]
Both the copper on the left-hand side and the silver on the right are solids. Both are left out of the equilibrium constant expression:
\[ K_c = \dfrac{[Cu_{2+}]}{[Ag^+]^2}\]
This equilibrium is only established if calcium carbonate is heated in a closed system, preventing carbon dioxide from escaping:
\[CaCO_{3(s)} \rightleftharpoons CaO_{(s)} + CO_{2(g)} \]
The only nonsolid species in this system is carbon dioxide, so it is the only term in the equilibrium constant expression:
\[ K_c = [CO_2]\]
Contributors and Attributions
Jim Clark (Chemguide.co.uk)


