9.8: Acid-Base Equilibria
- Page ID
- 84556
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)A great many processes involve proton transfer, or acid-base types of reactions. As many biological systems depend on carefully controlled pH, these types of processes are extremely important. The pH is defined by
\[ pH \equiv -\log a(\ce{H^{+}}) \approx -\log [\ce{H^{+}}] \label{eq1} \]
where \(a\) is the activity of hydronium ions and \([\ce{H^{+}}]\) is the true concentration of hydronium ions (both in mol/L).
The dissociation of a weak acid in water is governed by the equilibrium defined by
\[HA(aq) \rightleftharpoons H^+(aq) + A^-(aq) \nonumber \]
The equilibrium constant for such a reaction, \(K_a\), takes the form
\[K_a = \dfrac{[H^+][A^-]}{[HA]} \label{eq3} \]
As is the case for all thermodynamic equilibrium constants, the concentrations are replaced by activities and the equilibrium constant is unitless. However, if all species behave ideally (have unit activity coefficients) the units can be used as a very useful guide in solving problems.
What is the pH of a 0.200 M HOAc (acetic acid) solution? (Ka = 1.8 x 10-5 M)
Solution
An ICE table will come in very handy here!
| \([HOAc]\) | \([H^+]\) | \([OAc^-]\) | |
|---|---|---|---|
| Initial | 0.200 M | 0 | 0 |
| Change | -x | +x | +x |
| Equilibrium | 0.200 M - x | x | x |
The equilibrium problem can then be set up as
\[ K_a = \dfrac{[H^+][OAc^-]}{[HOAc]} \nonumber \]
Substituting the values that are known
\[ 1.8 \times 10^{-5} =\dfrac{ x^2}{0.200 \, M -x} \nonumber \]
This produces a quadratic equation, and thus two values of \(x\) which satisfy the relationship.
\[x_1 = -0.001906 \,M \nonumber \]
\[x_2 = 0.001888 \,M \nonumber \]
The negative root is not physically meaningful since the concentrations of \(H^+\) and \(OAc^-\) cannot be negative. Using the value of \(x_2\) as \([H^+]\), the pH is then calculated (via Equation \ref{eq1}) to be
\[ pH \approx -\log_{10} (0.001888) = 2.72 \nonumber \]
The Auto-ionization of Water
Water is a very important solvent as water molecules have large dipole moments which create favorable interactions with ionic compounds. Water also has a large dielectric constant which damps the electric field generated by ions in solutions, making the comparative interactions with water more favorable than with other ions in solution in many cases. But water also dissociates into ions through the reaction
\[H_2O(l) \rightleftharpoons H^+(aq) + OH^-(aq) \label{eqA} \]
The equilibrium constant governing this dissociation is highly temperature dependent. The data below are presented by Bandura and Lvov (Bandura & Lvov, 2006)
| T (°C) | 0 | 25 | 50 | 75 | 100 |
|---|---|---|---|---|---|
| pKw | 14.95 | 13.99 | 13.26 | 12.70 | 12.25 |
From these data, a van’t Hoff plot can be constructed.
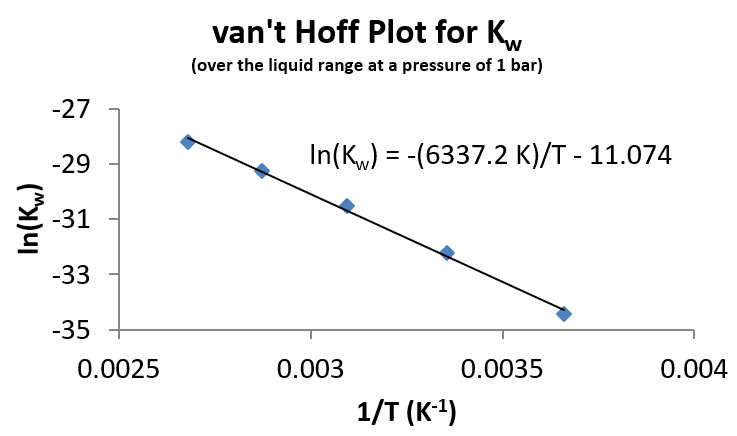
There is some curvature to the line, suggesting some (albeit small) temperature dependence for \(\Delta H_{rxn}\) for Equation \ref{eqA}. However, from the fit of these data, a value of \(\Delta H_{rxn}\) can be determined to be 52.7 kJ/mol. Of particular note is that the dissociation is endothermic, so increases in temperature will lead to a greater degree of dissociation.
What is the pH of neutral water at 37 °C (normal human body temperature)? Neutral water no excess of \([H^+]\) over \([OH^-]\) or vice versa.
Solution
From the best-fit line in the van’t Hoff plot of Figure \(\PageIndex{1}\), the value of \(K_w\) can be calculated:
\[ \ln (K_w) = - \dfrac{6338 \,K}{310\,K} - 11.04 \nonumber \]
\[ K_w= 2.12 \times 10^{-14}\,M^2 \nonumber \]
Since \(K_w\) gives the product of \([H^+]\) and \([OH^-]\) (which must be equal in a neutral solution),
\[H^+] = \sqrt{2.12 \times 10^{-14}\,M^2} = 1.456 \times 10^{-7}\,M \nonumber \]
And the pH is given by (Equation \ref{eq1}):
\[ pH = -\log_{10} (1.456 \times 10^{-7}) = 6.84 \nonumber \]
Note: This is slightly less than a pH of 7.00, which is normally considered to be “neutral.” But a pH of 7.00 is only neutral at 25 °C! At higher temperatures, neutral pH is a lower value due to the endothermic nature of the auto-ionization water. While it has a nigher \([H^+]\) concentration, it also has a higher \([OH^-]\) and at the same level, so it is still technically neutral.
The Hydrolysis of a Weak Base
Hydrolysis is defined as a reaction with water that splits a water molecule. The hydrolysis of a weak base defines the equilibrium constant Kb.
\[A^- + H_2O \rightleftharpoons HA + OH^- \nonumber \]
For this reaction, the equilibrium constant \(K_b\) is given by
\[ K_b = \dfrac{[HA][OH^-]}{[A^-]} \nonumber \]
The concentration (or activity) of the pure compound H2O is not included in the equilibrium expression because, being a pure compound in its standard state, it has unit activity throughout the process of establishing equilibrium. Further, it should be noted that when Kb is combined with the expression for Ka for the weak acid HA (Equation \ref{eq3}),
\[K_a K_b = \left( \dfrac{[H^+][A^-]}{[HA]} \right) \left( \dfrac{[HA][OH^-]}{[A^-]} \right) = [H^+][OH^-] = K_w \nonumber \]
As a consequence, if one knows \(K_a\) for a weak acid, one also knows \(K_b\) for its conjugate base, since the product results in \(K_w\).
What is the pH of a 0.150 M solution of KF? (For HF, pKa = 3.17 at 25 °C)
Solution
The problem involves the hydrolysis of the conjugate base of HF, F-. The hydrolysis reaction is
\[F^- + H_2O \rightleftharpoons HF + OH^- \nonumber \]
An ICE table is in order here.
| \(F^-\) | \(HF\) | \(OH^-\) | |
|---|---|---|---|
| Initial | 0.150 M | 0 | 0 |
| Change | -x | +x | +x |
| Equilibrium | 0.150 M - x | x | x |
So the expression for \(K_b\) is
\[ K_b = \dfrac{K_w}{K_a} = \dfrac{1.0 \times 10^{-14} M^2}{10^{3.17} M} = \dfrac{x^2}{1.50 \,M-x} \nonumber \]
In this case, the small value of \(K_b\) insures that the value of x will be negligibly small compared to 0.150 M. In this limit, the value of \(x\) (which is equal to [OH-])
\[ x = [OH^-] = 1.49 \times 10^{-6}\,M \nonumber \]
So \([H^+]\) is given by
\[ [H^+] =\dfrac{K_w}{[OH^-]} = \dfrac{10^{-14} M^2}{1.49 \times 10^{-6} M} = 6.71 \times 10^{-9} \,M \nonumber \]
And the pH is given by (Equation \ref{eq1}):
\[ pH = -\log_{10} (6.71 \times 10^{-9}) = 8.17 \nonumber \]
Note: The pH of this salt solution is slightly basic. This is to be expected as KF can be thought of being formed in the reaction of a weak acid (HF) with a strong base (KOH). In the competition to control the pH, the strong base ends up winning the battle.


