11.1: Definitions
- Page ID
- 106869
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Mathematical Operators
A mathematical operator is a symbol standing for a mathematical operation or rule that transforms one object (function, vector, etc) into another object of the same type. For example, when the derivative operator \(d/dx\), also denoted by \(\hat{D}_x\), operates on a function \(f(x)\), the result is the function \(df/dx\).
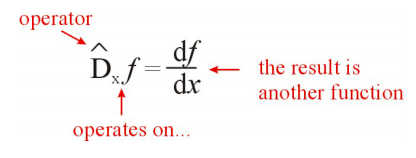
We can apply the operator \(\hat{D}_x\) to any function. For example, let’s consider the function \(g(x) = 2 \cos x+e^x\):
\[\hat{D}_xg(x)=-2 \sin x + e^x \nonumber \]
In physical chemistry, most operators involve either differentiation or multiplication. For instance, the multiplication operator denoted by \(\hat x\) means “multiply by \(x\)”. Using the previous example, when \(\hat x\) operates on \(g(x)\) we get
\[\hat{x}g(x)=2x \cos x +x e^x \nonumber \]
Before discussing what operators are good for, lets go over a few more examples. First, notice that we denote operators with a “hat”. Let’s define an operator \(\hat A\) (read as “A hat”) as \(\hat x + \dfrac{d}{dx}\)
\[\label{1} \hat A=\hat x + \dfrac{d}{dx} \]
This reads as “multiply the function by \(x\) and add the result to the first derivative of the function with respect to \(x\)”. The second term is equivalent to the operator we defined before, \(\hat {D}_x\), and using one or the other is a matter of preference. Notice that the expression \(\dfrac{d}{dx}\) does not require a “hat” because it is unambiguous. In the case of \(x\), we need to use the “hat” to be sure we distinguish the operator (multiply by \(x\)) from the variable \(x\). In the case of \(\dfrac{d}{dx}\), the expression clearly needs to be applied to a function, so it is obviously an operator. When \(\hat A\) operates on the function \(g(x)\) (defined above), we obtain:
\[\hat A g(x)=\hat x g(x) + \dfrac{d g}{dx}=-2 \sin x + e^x +2x \cos x +x e^x \nonumber \]
Linear Operators
In quantum mechanics we deal only with linear operators. An operator is said to be linear if
\[\hat A(c_1 f_1 (x)+c_2 f_2 (x))=\hat A c_1 f_1 (x)+\hat A c_2 f_2 (x) \nonumber \]
where \(c_1\) and \(c_2\) are constants (real or complex).
For instance, the \(\dfrac{d}{dx}\) operator is linear:
\[\dfrac{d}{dx}(c_1 f_1 (x)+c_2 f_2 (x))= \dfrac{d}{dx} c_1 f_1 (x)+\dfrac{d}{dx} c_2 f_2 (x) \nonumber \]
If we define the operator \(\hat B\) as the “square” operator (take the square of...), we notice that \(\hat B\) is not linear because
\[\hat B(c_1 f_1 (x)+c_2 f_2 (x))=(c_1 f_1 (x))^2+(c_2 f_2 (x))^2+2c_1 f_1 (x)c_2 f_2 (x) \nonumber \]
which is clearly different from
\[\hat B(c_1 f_1 (x))+\hat B (c_2 f_2 (x))= (c_1 f_1 (x))^2+(c_2 f_2 (x))^2 \nonumber \]
Eigenfunctions and Eigenvalues
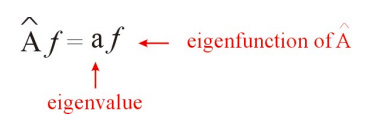
A common problem in quantum mechanics is finding the functions (\(f\)) and constants (\(a\)) that satisfy
\[\label{eigenfunction} \hat A f = a f \]
We will discuss the physical meaning of these functions and these constants later. For now, we will define the concept of eigenfunction and eigenvalue as follows:
If the result of operating on a function is the same function multiplied by a constant, the function is called an eigenfunction of that operator, and the proportionality constant is called an eigenvalue.
We can test whether a particular function is an eigenfunction of a given operator or not. For instance, let’s consider the operator \(-\dfrac{d^2}{dx^2}\) and the function \(g(x)\) defined in page . Is \(g(x)\) an eigenfunction of \(-\dfrac{d^2}{dx^2}\)? In lay terms: if we take the second derivative of \(g(x)\) and change the sign of the result, do we get a function that can be expressed as \(g(x)\) times a constant?
Let’s try it:
\[-\dfrac{d^2g(x)}{dx^2} = 2 \cos x - e^x \nonumber \]
The result cannot be expressed as a constant times \(g(x)\):
\[2 \cos x - e^x \neq a(2 \cos x+ e^x) \nonumber \]
so \(g(x)\) is not an eigenfunction of the operator \(-\dfrac{d^2}{dx^2}\).
Let’s consider another function: \(h(x) = 2sin(bx)\), where \(b\) is a constant. Is \(h(x)\) and eigenfunction of the operator \(-\dfrac{d^2}{dx^2}\)? We’ll take the second derivative of \(g(x)\), multiply by \(-1\), and check whether the result can be expressed as a constant times \(h(x)\):
\[-\dfrac{d^2h(x)}{dx^2} = 2b^2 \sin (bx) \nonumber \]
Notice that the result is \(b^2\) times the function \(h(x)\), so the conclusion is that \(h(x)\) is an eigenfunction of the operator \(-\dfrac{d^2}{dx^2}\), and that the corresponding eigenvalue is \(b^2\). A common mistake is to conclude that the eigenvalue is \(2b^2\). Be sure you understand why this is wrong.. Also, notice that \(b^2\) is a constant because it does not involve the variable \(x\). Another common mistake is to write eigenvalues that are not constants, but contain the independent variable.
So far we have learned how to test whether a given function is an eigenfunction of a given operator or not. How can we calculate the eigenfunctions of a given operator? In general, this involves solving a differential equation. For instance, the eigenfunctions of the operator \(-\dfrac{d^2}{dx^2}\) satisfy the equation
\[-\dfrac{d^2f(x)}{dx^2}=a f(x), \nonumber \]
where \(a\) is the eigenvalue. This is an ordinary second order differential equation with constant coefficients, so it can be solved with the methods we learned in previous chapters. Can you solve it and find the eigenfunctions of the operator \(-\dfrac{d^2}{dx^2}\)?
The eigenfunctions and eigenvalues of an operator play a central role in quantum mechanics. Before moving on, we’ll introduce an important property that you will use often in your physical chemistry course:
If two functions \(f_1(x)\) and \(f_2(x)\) are both eigenfunctions of an operator with the same eigenvalue, the linear combination \(c_1f_1(x)+c_2f_2(x)\) will also be an eigenfunction with the same eigenvalue.
For instance, the functions \(e^{ax}\) and \(e^{-ax}\) are both eigenfunctions of the operator \(\dfrac{d^2}{dx^2}\) with eigenvalue \(a^2\). Therefore, any linear combination \(c_1e^{ax}+c_2e^{-ax}\) will be an eigenfunction of this operator with eigenvalue \(a^2\), regardless of the values of \(c_1\) and \(c_2\). To prove it, take the second derivative of the function \(c_1e^{ax}+c_2e^{-ax}\) and prove that it equals \(a^2\) times \(c_1e^{ax}+c_2e^{-ax}\).
The function \(\cos(ax)\) is also an eigenfunction of \(\dfrac{d^2}{dx^2}\). However, the function \(c_1e^{ax}+c_2\cos(ax)\) is not. What went wrong?


