2.3: Ideal Gases
- Page ID
- 41405
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)In an ideal gas, there are no interactions between the particles, hence, the particles do not exert forces on each other. However, particles do experience a force when they collide with the walls of the container. Let us assume that each collision with a wall is elastic. Let us assume that the gas is in a cubic box of length \(a\) and that two of the walls are located at \(x = 0\) and at \(x = a\). Thus, a particle moving along the \(x\) direction will eventually collide with one of these walls and will exert a force on the wall when it strikes it, which we will denote as \(F_x\). Since every action has an equal and opposite reaction, the wall exerts a force \(-F_x\) on the particle. According to Newton’s second law, the force \(-F_x\) on the particle in this direction gives rise to an acceleration via
\[-F_x = ma_x = m\dfrac{\Delta v_x}{\Delta t} \label{2.1}\]
Here, \(t\) represents the time interval between collisions with the same wall of the box. In an elastic collision, all that happens to the velocity is that it changes sign. Thus, if \(v_x\) is the velocity in the \(x\) direction before the collision, then \(-v_x\) is the velocity after, and \(\Delta v_x = -v_x - v_x = -2v_x\), so that
\[-F_x = -2m\dfrac{v_x}{\Delta t} \label{2.2}\]
In order to find \(\Delta t\), we recall that the particles move at constant speed. Thus, a collision between a particle and, say, the wall at \(x = 0\) will not change the particle’s speed. Before it strikes this wall again, it will proceed to the wall at \(x = a\) first, bounce off that wall, and then return to the wall at \(x = 0\). The total distance in the \(x\) direction traversed is \(2a\), and since the speed in the \(x\) direction is always \(v_x\), the interval \(\Delta t = \dfrac{2a}{v_x}\). Consequently, the force is
\[-F_x = -\dfrac{mv_x^2}{a} \label{2.3}\]
Thus, the force that the particle exerts on the wall is
\[F_x = \dfrac{mv_x^2}{a} \label{2.4}\]
The mechanical definition of pressure is
\[P = \dfrac{\langle F \rangle}{A} \label{2.5}\]
where \(\langle F \rangle\) is the average force exerted by all \(N\) particles on a wall of the box of area \(A\). Here \(A = a^2\). If we use the wall at \(x = 0\) we have been considering, then
\[P = \dfrac{N \langle F_x \rangle}{a^2} \label{2.6}\]
because we have \(N\) particles hitting the wall. Hence,
\[P = \dfrac{N m \langle v_x^2 \rangle}{a^3} \label{2.7}\]
from our study of the Maxwell-Boltzmann distribution, we found that
\[\langle v_x ^2 \rangle = \dfrac{k_B T}{m} \label{2.8}\]
Hence, since \(a^3 = V\),
\[P = \dfrac{N k_B T}{V} = \dfrac{n R T}{V} \label{2.9}\]
which is the ideal gas law.
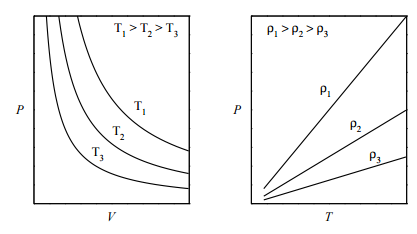
The ideal gas law is an example of an equation of state, which was introduced in Lecture 1. One way to visualize any equation of state is to plot the so-called isotherms, which are graphs of \(P\) vs. \(V\) at fixed values of \(T\) . For the ideal-gas equation of state \(P = nRT/V\) , some of the isotherms are shown in the figure below (left panel): If we plot \(P\) vs. \(T\) at fixed volume (called the isochores), we obtain the plot in the right panel. What is important to note, here, is that an ideal gas can exist only as a gas. It is not possible for an ideal gas to condense into some kind of “ideal liquid”. In other words, a phase transition from gas to liquid can be modeled only if interparticle interactions are properly accounted for.
Note that the ideal-gas equation of state can be written in the form
\[\dfrac{P V}{n R T} = \dfrac{P \bar{V}}{R T} = \dfrac{P}{\rho R T} = 1 \label{2.10}\]
where \(\bar{V} = V/n\) is called the molar volume. Unlike \(V\) , which increases as the number of moles increases (an example of what is called an extensive quantity in thermodynamics), \(\bar{V}\) does not exhibit this dependence and, therefore, is called intensive. The quantity
\[Z = \dfrac{P V}{n R T} = \dfrac{P \bar{V}}{R T} = \dfrac{P}{\rho R T} \label{2.11}\]
is called the compressibility of the gas. In an ideal gas, if we “compress” the gas by increasing \(P\) , the density \(\rho\) must increase as well so as to keep \(Z =1\). For a real gas, \(Z\), therefore, gives us a measure of how much the gas deviates from ideal-gas behavior.
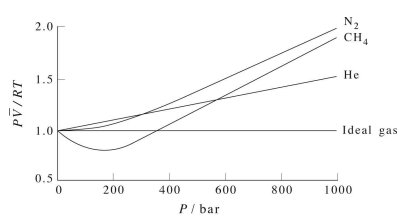
Figure 2.2 shows a plot of \(Z\) vs. \(P\) for several real gases and for an ideal gas. The plot shows that for sufficiently low pressures (hence, low densities), each gas approaches ideal-gas behavior, as expected.

