10.E: Polyatomic Bonding (Exercises)
- Page ID
- 51149
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)These are homework exercises to accompany Chapter 10 of McQuarrie and Simon's "Physical Chemistry: A Molecular Approach" Textmap.
Q10.8
Show that the four \(sp^3\) orbitals are orthonormal.
S10.8
This means showing that each pair of \(|sp^3 \rangle \) hybrid orbitals meets the criteria: \( \langle sp^3_i | sp^3_j \rangle = \delta_{ij} \)
Designating the four sp3 orbitals as:
\( | 1 \rangle = \dfrac{1}{2}( | s \rangle + | p_x \rangle + | p_y \rangle + | p_z \rangle \))
\( | 2 \rangle = \dfrac{1}{2}( | s \rangle - | p_x \rangle - | p_y \rangle + | p_z \rangle \))
\( | 3 \rangle = \dfrac{1}{2}( | s \rangle + | p_x \rangle - | p_y \rangle - | p_z \rangle \))
\( | 4 \rangle = \dfrac{1}{2}( | s \rangle - | p_x \rangle + | p_y \rangle - | p_z \rangle \))
Normality:
\[ \langle 1 | 1 \rangle = \left(\dfrac{1}{2}( \langle s| + \langle p_x | + \langle p_y| + \langle p_z|)\right) \left(\dfrac{1}{2}( |s \rangle + | p_x \rangle + |p_y \rangle + | p_z \rangle) \right) \]
\[= \dfrac{1}{4} \left(\langle s|s \rangle + \langle s| p_x \rangle + \langle s| p_y \rangle + \langle s| p_z \rangle + \langle p_x| s \rangle + \langle p_x| p_x \rangle + \langle p_x| p_y \rangle + \langle p_x | p_z \rangle + \langle p_y| s \rangle + \langle p_y | p_x \rangle + \langle p_y | p_y \rangle + \langle p_y| p_z \rangle + \langle p_z| s \rangle + \langle p_z | p_x \rangle + \langle p_z | p_y \rangle + \langle p_z| p_z \rangle \right) \]
\[= \dfrac{1}{4}( 1+0+0+0+0+1+0+0+0+0+1+0+0+0+0+1) = 1 \]
\[ \langle 2 | 2 \rangle = \left(\dfrac{1}{2}( \langle s| - \langle p_x | - \langle p_y| + \langle p_z|)\right) \left(\dfrac{1}{2}( |s \rangle - | p_x \rangle - |p_y \rangle + | p_z \rangle)\right) \]
\[= \dfrac{1}{4} \left(\langle s|s \rangle - \langle s| p_x \rangle - \langle s| p_y \rangle + \langle s| p_z \rangle - \langle p_x| s \rangle + \langle p_x| p_x \rangle + \langle p_x| p_y \rangle - \langle p_x | p_z \rangle - \langle p_y| s \rangle + \langle p_y | p_x \rangle + \langle p_y | p_y \rangle - \langle p_y| p_z \rangle + \langle p_z| s \rangle - \langle p_z | p_x \rangle - \langle p_z | p_y \rangle + \langle p_z| p_z \rangle \right) \]
\[= \dfrac{1}{4}( 1+0+0+0+0+1+0+0+0+0+1+0+0+0+0+1) = 1 \]
\[ \langle 3 | 3 \rangle = \left(\dfrac{1}{2}( \langle s| + \langle p_x | - \langle p_y| - \langle p_z|) \right) \left(\dfrac{1}{2}( |s \rangle + | p_x \rangle - |p_y \rangle - | p_z \rangle) \right) \]
\[= \dfrac{1}{4} \left(\langle s|s \rangle + \langle s| p_x \rangle - \langle s| p_y \rangle - \langle s| p_z \rangle + \langle p_x| s \rangle + \langle p_x| p_x \rangle - \langle p_x| p_y \rangle - \langle p_x | p_z \rangle - \langle p_y| s \rangle - \langle p_y | p_x \rangle + \langle p_y | p_y \rangle + \langle p_y| p_z \rangle - \langle p_z| s \rangle - \langle p_z | p_x \rangle + \langle p_z | p_y \rangle + \langle p_z| p_z \rangle \right)\]
\[= \dfrac{1}{4}( 1+0+0+0+0+1+0+0+0+0+1+0+0+0+0+1) = 1 \]
\[ \langle 4 | 4 \rangle = \left(\dfrac{1}{2}( \langle s| - \langle p_x | + \langle p_y| - \langle p_z|)\right) \left(\dfrac{1}{2}( |s \rangle - | p_x \rangle + |p_y \rangle - | p_z \rangle)\right) \]
\[= \dfrac{1}{4}(\langle s|s \rangle - \langle s| p_x \rangle + \langle s| p_y \rangle - \langle s| p_z \rangle - \langle p_x| s \rangle + \langle p_x| p_x \rangle - \langle p_x| p_y \rangle + \langle p_x | p_z \rangle + \langle p_y| s \rangle - \langle p_y | p_x \rangle + \langle p_y | p_y \rangle - \langle p_y| p_z \rangle - \langle p_z| s \rangle + \langle p_z | p_x \rangle - \langle p_z | p_y \rangle + \langle p_z| p_z \rangle)\]
\[= \dfrac{1}{4}( 1+0+0+0+0+1+0+0+0+0+1+0+0+0+0+1) = 1 \]
Orthogonality:
For the longer wavefunctions, only surviving terms in the dot product are included:
\[ \langle 1 | 2 \rangle = \langle 2 | 1 \rangle = \left(\dfrac{1}{2}( \langle s| - \langle p_x | - \langle p_y| + \langle p_z|)\right) \left(\dfrac{1}{2}( |s \rangle + | p_x \rangle + |p_y \rangle + | p_z \rangle)\right) \]
\[= \dfrac{1}{4}(\langle s|s \rangle - \langle p_x| p_x \rangle - \langle p_y | p_y \rangle + \langle p_z| p_z \rangle) = \dfrac{1}{4}( 1-1-1+1) = 0 \]
\[ \langle 1 | 3 \rangle = \langle 3 | 1 \rangle = \left(\dfrac{1}{2}( \langle s| + \langle p_x | - \langle p_y| - \langle p_z|)\right) \left(\dfrac{1}{2}( |s \rangle + | p_x \rangle + |p_y \rangle + | p_z \rangle)\right) \]
\[= \dfrac{1}{4}(\langle s|s \rangle + \langle p_x| p_x \rangle - \langle p_y | p_y \rangle - \langle p_z| p_z \rangle) = \dfrac{1}{4}( 1+1-1-1) = 0 \]
\[ \langle 1 | 4 \rangle = \langle 4 | 1 \rangle = \left(\dfrac{1}{2}( \langle s| - \langle p_x | + \langle p_y| - \langle p_z|)\right) \left(\dfrac{1}{2}( |s \rangle + | p_x \rangle + |p_y \rangle + | p_z \rangle)\right)\]
\[= \dfrac{1}{4}(\langle s|s \rangle - \langle p_x| p_x \rangle + \langle p_y | p_y \rangle - \langle p_z| p_z \rangle) = \dfrac{1}{4}( 1-1+1-1) = 0 \]
\[ \langle 2 | 3 \rangle = \langle 3 | 2 \rangle = \left(\dfrac{1}{2}( \langle s| + \langle p_x | - \langle p_y| - \langle p_z|)\right) \left(\dfrac{1}{2}( |s \rangle - | p_x \rangle - |p_y \rangle + | p_z \rangle)\right)\]
\[ = \dfrac{1}{4}(\langle s|s \rangle - \langle p_x| p_x \rangle + \langle p_y | p_y \rangle - \langle p_z| p_z \rangle) = \dfrac{1}{4}( 1-1+1-1) = 0 \]
\[ \langle 2 | 4 \rangle = \langle 4 | 2 \rangle = \left(\dfrac{1}{2}( \langle s| - \langle p_x | + \langle p_y| - \langle p_z|) \right) \left(\dfrac{1}{2}( |s \rangle - | p_x \rangle - |p_y \rangle + | p_z \rangle)\right) \]
\[= \dfrac{1}{4}(\langle s|s \rangle + \langle p_x| p_x \rangle - \langle p_y | p_y \rangle - \langle p_z| p_z \rangle) = \dfrac{1}{4}( 1+1-1-1) = 0 \]
\[ \langle 3 | 4 \rangle = \langle 4 | 3 \rangle = (\dfrac{1}{2}( \langle s| - \langle p_x | + \langle p_y| - \langle p_z|)) (\dfrac{1}{2}( |s \rangle + | p_x \rangle - |p_y \rangle - | p_z \rangle))\]
\[= \dfrac{1}{4}(\langle s|s \rangle - \langle p_x| p_x \rangle - \langle p_y | p_y \rangle + \langle p_z| p_z \rangle) = \dfrac{1}{4}( 1-1-1+1) = 0 \]
Q10.9
The \(sp^{3}d^{2}\) hybrid orbitals are given by:
\(\chi_1 (r)=\dfrac{1}{\sqrt{6}}\psi_{3s}(r)-\dfrac{1}{\sqrt{2}}\psi_{3p_x}(r)-\dfrac{1}{\sqrt{12}}\psi_{3d_{z^{2}}}+\dfrac{1}{\sqrt{4}}\psi_{3d_{x^{2}-y^{2}}}\)
\(\chi_2 (r)=\dfrac{1}{\sqrt{6}}\psi_{3s}(r)+\dfrac{1}{\sqrt{2}}\psi_{3p_x}(r)-\dfrac{1}{\sqrt{12}}\psi_{3d_{z^{2}}}+\dfrac{1}{\sqrt{4}}\psi_{3d_{x^{2}-y^{2}}}\)
\(\chi_3 (r)=\dfrac{1}{\sqrt{6}}\psi_{3s}(r)-\dfrac{1}{\sqrt{2}}\psi_{3p_y}(r)-\dfrac{1}{\sqrt{12}}\psi_{3d_{z^{2}}}-\dfrac{1}{\sqrt{4}}\psi_{3d_{x^{2}-y^{2}}}\)
\(\chi_4 (r)=\dfrac{1}{\sqrt{6}}\psi_{3s}(r)+\dfrac{1}{\sqrt{2}}\psi_{3p_y}(r)-\dfrac{1}{\sqrt{12}}\psi_{3d_{z^{2}}}-\dfrac{1}{\sqrt{4}}\psi_{3d_{x^{2}-y^{2}}}\)
\(\chi_5 (r)=\dfrac{1}{\sqrt{6}}\psi_{3s}(r)-\dfrac{1}{\sqrt{2}}\psi_{3p_z}(r)+\dfrac{1}{\sqrt{12}}\psi_{3d_{z^{2}}}\)
\(\chi_6 (r)=\dfrac{1}{\sqrt{6}}\psi_{3s}(r)+\dfrac{1}{\sqrt{2}}\psi_{3p_z}(r)+\dfrac{1}{\sqrt{12}}\psi_{3d_{z^{2}}}\)
Determine the angles of \(SF_{6}\) using the vector approach (dot product formula).
S10.9
The \(s\) orbitals are spherical and therefor do not contribute to the directional vectors for this problem. The \(d_{z^2}\) orbital only has \(z\) directionality and the \(d_{x^{2}-y^{2}}\) orbital has equal parts \(x\) and \(y\) directionality. The equations can be rewritten:
\[\psi_{1}=\left( -\dfrac{1}{\sqrt{2}} + \dfrac{1}{\sqrt{4}} \right) \textbf{i} + \dfrac{1}{\sqrt{4}}\textbf{j} - \dfrac{1}{\sqrt{12}} \textbf{k} \hspace{1cm} \psi_{2}=\left( \dfrac{1}{\sqrt{2}} + \dfrac{1}{\sqrt{4}} \right) \textbf{i} + \dfrac{1}{\sqrt{4}}\textbf{j} - \dfrac{1}{\sqrt{12}} \textbf{k} \]
\[\psi_{3}=-\dfrac{1}{\sqrt{4}}\textbf{i} + \left( -\dfrac{1}{\sqrt{2}} - \dfrac{1}{\sqrt{4}} \right) \textbf{j} - \dfrac{1}{\sqrt{12}} \textbf{k} \hspace{1cm} \psi_{4}= -\dfrac{1}{\sqrt{4}}\textbf{i} + \left( \dfrac{1}{\sqrt{2}} - \dfrac{1}{\sqrt{4}} \right) \textbf{j} - \dfrac{1}{\sqrt{12}} \textbf{k}\]
\[\psi_{5}= \left( -\dfrac{1}{\sqrt{2}} + \dfrac{1}{\sqrt{12}} \right) \textbf{k}\hspace{1cm} \psi_{6}= \left( \dfrac{1}{\sqrt{2}} + \dfrac{1}{\sqrt{12}} \right) \textbf{k} \]
For any two \(\psi\) the angel can be calculated using:
\[ \left( \sqrt{A^{2}_{x}+A^{2}_{y}+A^{2}_{z}} \right) \left( \sqrt{B^{2}_{x}+B^{2}_{y}+B^{2}_{z}} \right) cos\theta = A_{x}B_{x}+A_{y}B_{y}+A_{z}B_{z}\]
So, choosing \(\psi_{5}\) and \(\psi_{6}\) we get:
\[\left( -\dfrac{1}{\sqrt{2}} + \dfrac{1}{\sqrt{12}} \right) \left( \dfrac{1}{\sqrt{2}} + \dfrac{1}{\sqrt{12}} \right) cos\theta = \left( -\dfrac{1}{\sqrt{2}} + \dfrac{1}{\sqrt{12}} \right) \left( \dfrac{1}{\sqrt{2}} + \dfrac{1}{\sqrt{12}} \right) \]
\[-\dfrac{5}{12} cos \theta = -\dfrac{5}{12}\]
\[ cos\theta = 0\]
\[ \theta = 90^{\circ} \]
Q10.10
Given \[\xi_1 = \dfrac{1}{\sqrt{4}}2s + \sqrt{\dfrac{3}{4}}2p_z\]
\[\xi_2 = \dfrac{1}{\sqrt{4}}2s + \sqrt{\dfrac{2}{3}}2p_x - \dfrac{1}{\sqrt{12}}2p_z\]
what is the angle between \(\xi_1\) and \(\xi_2\)?
What is the purpose of the square root constants before the orbitals?
S10.10
Since we know that
\[(\sqrt{A_x^2 + A_y^2 + A_z^2})(\sqrt{B_x^2 + B_y^2 + B_z^2})\cos{\theta} = A_xB_x + A_yB_y + A_zB_z\]
When we plug in our constants in the x, y, and z directions for the two equations we get
\[(\sqrt{\dfrac{2}{3} + \dfrac{1}{12}})(\sqrt{\dfrac{3}{4}})\cos{\theta} = - \sqrt{\dfrac{1}{12}}\sqrt{\dfrac{3}{4}}\]
isolating the theta term we get
\[\theta = \arccos(- \sqrt{\dfrac{1}{12}}/\sqrt{\dfrac{12}{9}}\]
yielding
\[\theta=109.47^{\circ}\]
The square root constants are the necessary normalization constants.
Q10.11
Given that \[ \psi_{1} = 0.71j + 0.55k\] and \[ \psi_{2} = -0.71j + 0.55k\] Find the bond angle between \(\psi_1\) and \(\psi_2\) using the vector approach.
S10.11
Using this equation below:
\[ \left(\sqrt{A_{x}^{2} + A_{y}^{2} + A_{z}^{2}} \right) \left(\sqrt{B_{x}^{2} + B_{y}^{2} + B_{z}^{2}} \right) cos\theta = A_{x}B_{x} + A_{y}B_{y} + A_{z}B_{z} \]
use to find the angle between the orbitals,
\[ (0.71^{2} + 0.55^{2})^{0.5} (0.71^{2} + 0.55^{2})^{0.5} cos\theta = -0.71^{2} + 0.55^{2} \]
\[ cos\theta = -0.25 \]
\[\theta = 104.5^{\circ}\]
Q10.12
Assuming a water molecule sits in the yz-plane, show that two bonding hybrid atomic orbitals on the oxygen atom can be expressed as
\[\psi_{1} = N[\gamma 2s + (sin[\theta]2p_{y} + cos[\theta]2p_{z}]\]
and
\[\psi_{2} = N[\gamma 2s - (sin[\theta]2p_{y} + cos[\theta]2p_{z}].\]
Additionally, find \(\gamma\) assuming the bond angles to be \(104.5°\).
S10.12
Because the molecules are in the yz plane, the \(p_{x}\) orbital can be neglected because it is completely orthogonal. The hybrid orbitals are a linear combination of the \(2s,\:2p_{y},\text{ and }2p_{z}\) orbitals.
\[\psi_{bonding} = N[\gamma2_{s} + c_{1}2p_{y} + c_{2}2p_{z}]\]
\(c_{1}\) and \(c_2\) can be found by thinking about how the bonds are oriented. The two bonds both have \(cos[\theta]\) character in the z-direction and have \(\pm sin[\theta]\) in the y-direction. The two functions can therefore be written as
\[\psi_{1} = N[\gamma 2s + (sin[\theta]2p_{y} + cos[\theta]2p_{z})\]
and
\[\psi_{2} = N[\gamma 2s - (sin[\theta]2p_{y} + cos[\theta]2p_{z})\]
when \(c_{1}\) and \(c_{2}\) are replaced with the sin and cos functions above.
The two functions when integrated will equal zero due to orthogonality.
\[\langle\psi_{1}|\psi_{2}\rangle = 0\]
\[N^{2}(\gamma^{2} + cos^{2}[\theta] - sin^{2}[\theta])\]
\[\gamma^{2} = sin^{2}[\theta] - cos^{2}[\theta]\]
The bond angles in water are 104.5° which can be substituted into \(\theta\).
\[\gamma = 0.5\]
Q10.13
The lone pair wave functions of H2O can be described as:
$$\psi_{l1} = 0.54(2s) - 0.44(2pz) + 0.72(2px)$$
$$\psi_{l2} = 0.54(2s) - 0.44(2pz) - 0.72(2px)$$
Confirm the orthonormality of these wave functions.
S10.13
The two wave functions must be normalized and orthogonal to each other.
$$\int d\tau \psi_{l1}^{*} \psi_{l1} = 1$$
$$= (0.54)^2(2s) + (-0.44)^2(2pz) + (0.72)^2(2px) =1$$
$$\int d\tau \psi_{l2}^{*} \psi_{l2} = 1$$
$$= (0.54)^2(2s) + (-0.44)^2(2pz) + (-0.72)^2(2px) =1$$
$$\int d\tau \psi_{l1}^{*} \psi_{l2} = 0$$
$$= (0.54)^2(2s) + (-0.44)^2(2pz) - (0.72)^2(2px) =0$$
The atomic orbitals within the linear combination of the lone pair wave functions are normalized and orthogonal, zeroing out cross products and leaving only the squares of the coefficients.
10.14
Molecular orbitals for a linear $$XY_{2}$$ molecule can be represented as
Draw a schematic representation for the $$3\sigma_{g}, 4\sigma_{g}, 1\pi_{g}, 2\sigma_{g}$$ orbitals
Which has the highest energy?
S10.14
\[ 3\sigma_{g}\]
NOTE:
\[3\sigma_g\]
$$4\sigma_{g}$$
$$1\pi_{g}$$
$$2\sigma_{g}$$

$$4\sigma_{g}$$ has the highest energy
The \[4\sigma_g\] moleclar orbital has the highest energy; as expected since it more nodes.
Q10.18
Use the given Walsh diagram to predict the geometry of the following molecules:
- \(H_{2}O\)
- \(H_{2}S\)
- \(H_{2}Be\)
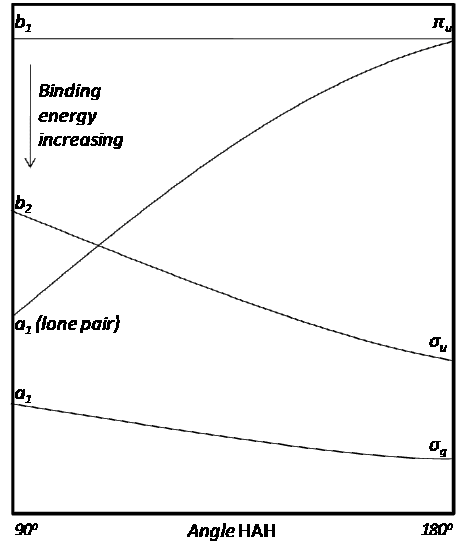
Walsh Diagram of an HAH molecule.Public Domain
S10.18
The Walsh Diagram predicts the geometry of a molecule by assigning its valence electrons to the appropriate energy levels. In general, the lowest energy configuration is preferred.
- \(H_{2}O\) has 8 valence electrons, which corresponds to the 4th highest orbital on the diagram. The bent configuration (90º) is lower in energy in this case.
- \(H_{2}S\) has 8 valence electrons as well, because sulfur and oxygen are in the same periodic group. Therefore, the bent configuration will be favored.
- \(H_{2}Be\) has 4 valence electrons, which corresponds to the 2nd highest orbital on the diagram. The linear configuration (180º) is lower in energy in this case.
Q10.19
Use the Walsh diagram for the valence electrons of a XY2 molecule to predict whether the following molecules are linear or bent:
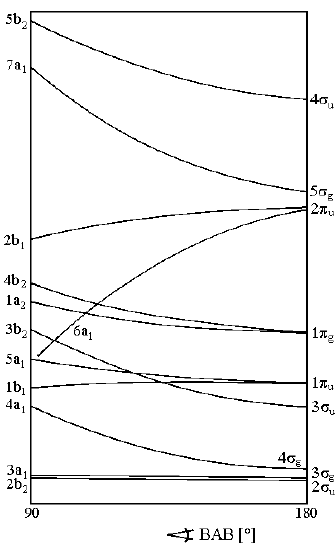
a. (CO2) b. (CO2+) c. (CO2-) d. (SO2-) b. (CF2+)
S10.19
| Valence Electrons | Geometry |
|---|---|
| 2-16 | linear |
| 17-20 | bent |
| 21-24 | linear |
- CO2 16 valence electrons Linear
- CO2+ 15 valence electrons Linear
- CO2- 17 valence electrons Bent
- SO2- 19 valence electrons Bent
- CF2+ 18 valence electrons Bent
Q10.20
Walsh correlation diagrams can be used to predict the shapes of polyatomic molecules that contain more than three atoms. In this and the following three problems we consider molecules that have the general formula XH3. We will restrict our discussion to XH3 molecules, where all the H—X—H bond angles are the same. If the molecule is planar, then the H—X—H bond angle is 120°. A nonplanar XH3 molecule, then, has an H—X—H bond angle that is less than 120°. Figure 10.26 shows the Walsh correlation diagram that describes how the energies of the molecular orbitals for an XH3 molecule change as a function of the H—X—H bond angle. Note that because XH3 is not linear, the labels used to describe the orbitals on the two sides of the correlation diagram do not have designations such as ![]() and
and ![]() . We see that the lowest-energy molecular orbital is insensitive to the H—X—H bond angle. Which atomic orbital(s) contribute to the lowest-energy molecular orbital? Explain why the energy of this molecular orbital is insensitive to changes in the H—X—H bond angle.
. We see that the lowest-energy molecular orbital is insensitive to the H—X—H bond angle. Which atomic orbital(s) contribute to the lowest-energy molecular orbital? Explain why the energy of this molecular orbital is insensitive to changes in the H—X—H bond angle.
S10.20
The lowest energy molecular orbital is the 1s orbital, which is a core atomic orbital instead of a bonding atomic orbital.
Q10.21
Consider the BH2 where the general Walsh diagram for a XH2 is shown below. What is the geometric preference of the molecule in ground and excited state?
S10.21
The BH2 molecule has the same geometric shape as water, it is bent where the HOMO is \({\pi_u}\). The first excited state relies on the degree of bending, and the 2a1 is unoccupied, and the next 1b2 is the highest occupied where the preferred geometry is linear, so at the first excited state will be linear.
\(BH_{2}\) is a linear molecule. It has 4 valance electrons which fill 2 of the lines in the Walsh diagram. This second line has lower energy towards linear conformation and \(1 \sigma_{u}\).
Q10.24
Solve for \(\psi_\pi \) corresponding to the energy \(E = \alpha + \beta \) for ethene.
S10.24
The bonding Huckel molecular orbitals is
\[ \psi_\pi = c_1 2p_{z1} + c_2 2p_{z2}\]
the relationship of the coefficients can be defined as the following from the secular determinate:
\[c_1 (\alpha - E) + c_2 \beta = 0 \]
\[c_1 \beta+ c_2 (\alpha - E) = 0 \]
Substituting \(E = \alpha + \beta \) into these expressions and solving gives
\[-c_1\beta+c_2\beta = 0 \]
\[c_1 = c_2 \]
Then plugging back into the original equation gives
\[ \psi_\pi = c_1 (2p_{zA} + 2p_{zB} ) \]
Now we can solve for \(c_1\) by normalizing the wavefunction
\[c^2_1(1 + 2S + 1) = 1 \]
where S = 0 so solving yields
\[c_1 = \frac{1}{\sqrt{2}}\].
The final wave function can be written as
\[\psi_\pi = \frac{1}{\sqrt{2}} (2p_{z1} + 2p_{z2})\]
Q10.25
Generalize the molecular orbital treatment of propene allyl cation. Find the energies and wave function of this molecule.
S10.25
The Huckel secular determinant for propene is \[\left. \begin{vmatrix} \alpha - E & \beta & 0 \\\ \beta & \alpha - E & \beta \\ 0 & \beta & \alpha - E \end{vmatrix} \right. = 0 \]
making a substitution for \( x = \frac{\alpha - E }{\beta} \) the secular determinant becomes \[\left. \begin{vmatrix} x & 1 & 0 \\ 1 & x & 1 \\ 0 & 1 & x \end{vmatrix} \right. = 0 \]
Solving the determinant yields a cubic polynomial \(x^3 - 2x = 0\) the roots of this polynomial are \( x = 0, \pm \sqrt{2} \)
Replacing x with the previous substitution made it is found that \[E = \alpha \pm \sqrt{2} \beta \] and \[E = 0\]
To find the wave function of propene you must find the constants \[\left. \begin{vmatrix} c_{1}(x) & c_{2}& 0 \\ c_{1} & c_{2}(x) & c_{3} \\ 0 & c_{2}& c_{3}(x) \end{vmatrix} \right. = 0 \]
solving the determinant yields \[c_{1} = c_{3} = \frac{1}{2}\] \[c_{2} = \frac{1}{\sqrt{2}}\]
therefore the wave function is \[ \psi = \frac{1}{2} 1s + \frac{1}{\sqrt{2}}2s + \frac{1}{2} 2p_{z} \]
Q10.26
Show that the six molecular orbitals for Benzene consturcted from the \(2Pp_x\) atomic orbital on each of the six carbon atoms:
\[\psi_{i}=\sum_{j=1}^{6} c_{ij}2p_{xj}\]
leads to a secular determinant.
S10.26
The above equation for benzene is :
\[\psi_{i} = c_{i1}2p_{z1}+c_{i2}2p_{z2}+c_{i3}2p_{z3}+c_{i4}2p_{z4}+c_{i5}2p_{z5}+c_{i6}2p_{z6}\]
The secular determinant for the benzene is :
\[\begin{vmatrix}H_{11}-ES_{11}&H_{12}-ES_{12}&H_{13}-ES_{13}&H_{14}-ES_{14}&H_{15}-ES_{15}&H_{16}-ES_{16}\\H_{12}-ES_{12}&H_{22}-ES_{22}&H_{23}-ES_{23}&H_{24}-ES_{24}&H_{25}-ES_{25}&H_{26}-ES_{26}\\H_{13}-ES_{13}&H_{23}-ES_{23}&H_{33}-ES_{33}&H_{34}-ES_{34}&H_{35}-ES_{35}&H_{36}-ES_{36}\\H_{14}-ES_{14}&H_{24}-ES_{24}&H_{34}-ES_{34}&H_{44}-ES_{44}&H_{45}-ES_{45}&H_{46}-ES_{46}\\H_{15}-ES_{15}&H_{25}-ES_{25}&H_{35}-ES_{35}&H_{45}-ES_{45}&H_{55}-ES_{55}&H_{56}-ES_{56}\\H_{16}-ES_{16}&H_{26}-ES_{26}&H_{36}-ES_{36}&H_{46}-ES_{46}&H_{56}-ES_{56}&H_{66}-ES_{66} \end{vmatrix}=0\]
\(H_{11}=H_{22}=H_{33}=H_{44}=H_{55}=H_{66}=\alpha\)
\(H_{12}=H_{23}=H_{34}=H_{45}=H_{56}=\beta\) The \(H_{ij}=H_{ji}\) is a hermitian operator when \(i\) and \(j\) are neighbors
\(H_{ij}=0\) when \(i\) and \(j\) are not neighbors
\(S_{11}=S_{22}=S_{33}=S_{44}=S_{55}=S_{66}= 1\)
\(S_{ij}=0\)

