Determination of Virial Coefficients for Argon Gas at 323 K
- Page ID
- 96234
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\(\newcommand{\ket}[1]{\left| #1 \right>} \)
\( \newcommand{\bra}[1]{\left< #1 \right|} \)
\( \newcommand{\braket}[2]{\left< #1 \vphantom{#2} \right| \left. #2 \vphantom{#1} \right>} \)
\( \newcommand{\qmvec}[1]{\mathbf{\vec{#1}}} \)
\( \newcommand{\op}[1]{\hat{\mathbf{#1}}}\)
\( \newcommand{\expect}[1]{\langle #1 \rangle}\)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Learning Objectives
- Goals: Pressure-volume data is presented for argon gas at 323.15 K. The compression factor (Z) is calculated for each P-V data pair and a virial equation of state is subsequently fitted to the data, yielding the virial coefficients.
- Prerequisites: A knowledge of virial equations of state for describing non-ideal gases.
- Resources you will need: This exercise should be carried out within a quantitative analysis software environment that is capable of fitting an arbitrary user-defined function to an x-y data set. You will also be graphing the data along with the fitted function.
The pressure (\(P\)), volume (\(V\)), and temperature (\(T\)) of a given amount of gas can be used to calculate the compression factor (\(Z\)),
\[ Z = \dfrac{PV_m}{RT} \label{1}\]
where \(V_m\) is the molar volume of the gas. \(Z\) is exactly equal to 1 for an ideal gas at all pressures. Deviations away from \(Z=1\) arise from intermolecular interactions.
A plot of \(Z\) versus pressure for an arbitrary gas is shown in Figure \ref{1}. Three regimes can be identified in this graph;
- The value of \(Z\) tends toward 1 as pressure approaches 0, where all gases tend toward ideal behavior,
- The value of Z is less than 1 at intermediate pressures because forces of attraction cause the pressure (or volume) in the numerator of Equation \ref{1} to be less than their ideal value, and
- The value of \(Z\) is greater than 1 and ultimately tends toward infinity at high pressures because repulsive forces cause the pressure (or volume) to be greater than their ideal values.
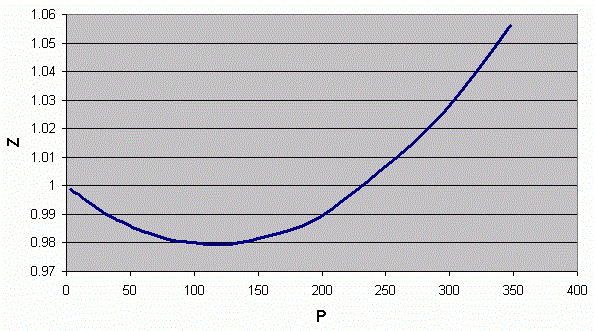
Figure \(\PageIndex{1}\): \(Z\) versus pressure for an arbitrary gas at a constant temperature.
The variation in the compression factor as a function of pressure can be expressed as a power series according to the expression
\[ Z = 1 +B'p + C'p^2 + D'p^3 ... \label{2}\]
where \(B’\), \(C’\), and \(D’\) are the second, third, and fourth virial coefficients, respectively. These coefficients have a characteristic value for each gas and are temperature dependent. When applying Equation \ref{2} to a gas from low to moderate pressures, one generally neglects higher order terms in the series. Equation \ref{2} is called a virial equation, and is one of the most accurate equations of state for a gas, provided one retains a sufficient number of terms in the expansion.
For some problems, it is more convenient to write the virial equation as a power series in reciprocal molar volume
\[ Z = 1 + B \dfrac{1}{V_m} +C \dfrac{1}{V_m^2} +D \dfrac{1}{V_m^3} \label{3}\]
where by convention the primes (') are normally dropped from the virial coefficients to distinguish them from the coefficients that are present in Equation \ref{2}.
This exercise will involve downloading a set of pressure-volume data for Argon gas at 323.15 K and analyzing the data to determine the virial coefficients \(B\), \(C\), and \(D\) in Equation \ref{3}.
Experimental Data
Click on the following link and save the data to an appropriate folder. The data was obtained from Blancett, A.L. and Hall, K.R., Physica, 47, 75-91 (1970). The first column of numbers is the pressure of argon gas in units of atmospheres and the second column of numbers is the corresponding molar volume of the gas in units of Liters/mol. The temperature of the gas was held constant throughout data collection at 323.15 Kelvin.
P-V Data for Argon at 323.15 K
Exercise
- Import the data into an appropriate quantitative analysis software package. How well does the data conform to Boyle's law? HINT: to address this question, you might investigate how well the data is represented by the equation \[ P = a \left( \dfrac{1}{V_m} \right) \label{4}\] where \(a\) is a constant.
- Calculate the compression factor (\(Z\)) for each pressure-volume data pair. Generate a plot of \(Z\) versus \(P\). Estimate the pressure where the gaseous system transitions from the attractive regime to the repulsive regime.
- You are now going to fit the data to a modified form of Equation \ref{3} from above. Generate two new columns of data from the original data; one corresponding to \(1/V_m\) and the other to (\(Z-1\)). \(1/V_m\) will represent the x-data set and (\(Z-1\)) will represent the y-data set in our fit. Using the appropriate procedures for your software environment, fit to this data to a function of the form \[y = Bx + C x^2 \label{5}\] Record the values of \(B\) and \(C\) from your fit; these are the second and third virial coefficients from Equation \ref{3}. Now generate a plot of the experimental and theoretical data compression factors versus reciprocal molar volume. Is the agreement reasonable?
- Repeat the fitting procedure from step (2), but add the term \(Dx^3\) to your fitting function. Again, record the values of \(B\), \(C\), and \(D\) and generate a plot of the experimental data with the new theoretical results. Does adding the forth virial coefficient to your fitting function improve the agreement between the experimental and theoretical data?


