12.4: General Properties of Time Correlation Functions
- Page ID
- 5288
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
Define a time correlation function between two quantities \(A (x) \) and \(B (x) \) by
\[\begin{align*} C_{AB} (t) &= \langle A(0)B(t)\rangle \\[4pt] &= \int d{\rm x}f({\rm x})A({\rm x})e^{iLt}B({\rm x}) \end{align*} \]
The following properties follow immediately from the above definition:
Property 1
\[\langle A(0)B(t)\rangle = \langle A(-t)B(0)\rangle \nonumber \]
Property 2
\[C_{AB}(0) = \langle A({\rm x})B({\rm x})\rangle \nonumber \] Thus, if \(A = B \), then
\[C_{AA}(t) = \langle A(0)A(t)\rangle \nonumber \]
known as the autocorrelation function of \(A\), and
\[C_{AA}(0) = \langle A^2\rangle \nonumber \]
If we define \(\delta A = A - \langle A \rangle \), then
\[C_{\delta A\delta A}(0) = \langle (\delta A)^2\rangle =\langle ( A - \langle A \rangle )^2 \rangle = \langle A^2\rangle - \langle A\rangle^2 \nonumber \]
which just measures the fluctuations in the quantity \(A\).
Property 3
A time correlation function may be evaluated as a time average, assuming the system is ergodic. In this case, the phase space average may be equated to a time average, and we have
\[C_{AB}(t) = \lim_{T\rightarrow\infty}{1 \over T-t}\int_0^{T-t}ds A({\rm x}(s))B({\rm x}(t+s)) \nonumber \]
which is valid for \( t<<T \). In molecular dynamics simulations, where the phase space trajectory is determined at discrete time steps, the integral is expressed as a sum
\[ C_{AB}(k\Delta t) = {1 \over N-k}\sum_{j=1}^{N-k}A({\rm x}_k)B({\rm x}_{k+j})\;\;\;\;\;\;\;\;\;\;k=0,1,2,...,N_c \nonumber \]
where \(N\) is the total number of time steps, \(\Delta t \) is the time step and \(N_c << N \).
Property 4: Onsager regression hypothesis
In the long time limit, \(A \) and \(B\) eventually become uncorrelated from each other so that the time correlation function becomes
\[ C_{AB}(t) = \langle A(0)B(t)\rangle \rightarrow \langle A\rangle\langle B\rangle \nonumber \]
For the autocorrelation function of \(A\), this becomes
\[ C_{AA}(t)\rightarrow \langle A\rangle^2 \nonumber \]
Thus, \(C_{AA} (t) \) decays from \(\langle A^2 \rangle \) at \( t = 0\) to \( \langle A^2 \rangle \) as \(t \rightarrow \infty \).
An example of a signal and its time correlation function appears in Figure \(\PageIndex{1}\). In this case, the signal is the magnitude of the velocity along the bond of a diatomic molecule interacting with a Lennard-Jones bath. Its time correlation function is shown beneath the signal:
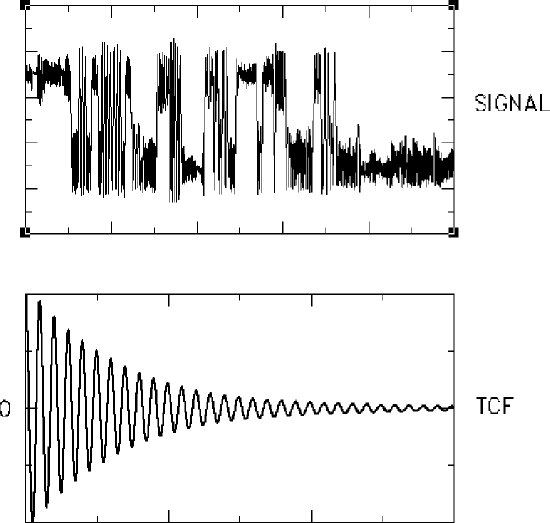
Over time, it can be seen that the property being autocorrelated eventually becomes uncorrelated with itself.


