5.3: Electrolytes
( \newcommand{\kernel}{\mathrm{null}\,}\)
What is an electrolyte?
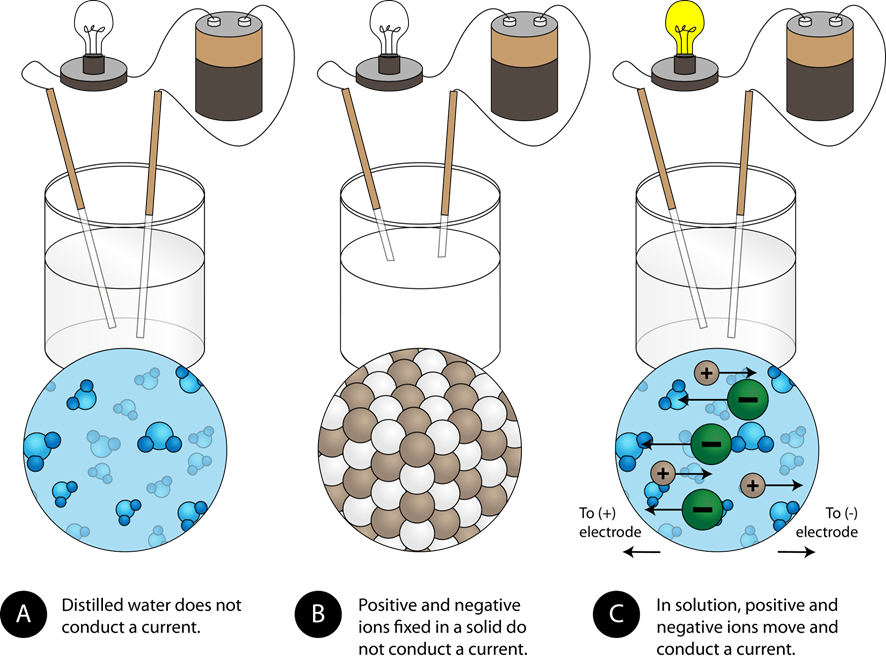
Electric current is defined as the movement of electric charges. The substances through which an electric current can flow are called electrical conductors, and the others are electrical nonconductors. Metals are electrical conductors because valence electrons of metal atoms can move around in a piece of metal. Ionic compounds are composed of cations and anions, but the ions in a solid can not move around. Therefore, solid ionic compounds are electrical nonconductors. Pure water does not have a sufficient concentration of ions in it and is an electrical nonconductor. The ionic compounds dissociate into ions when dissolved in water. The solution of ionic compounds in water is an electrical conductor because the ions can move around in the solution, as illustrated in Fig. 5.3.1.
Substances that produce electrically conducting solution when dissolved in water (or in another polar solvent) are called electrolytes.
All ionic compounds, acids, and bases produce ions in water and are classified are electrolytes.
Substances that produce an electrically nonconducting solution when dissolved in water are called nonelectrolytes.
Molecular compounds other than acids and bases, such as methanol, acetone, sugar, and glucose, remain neutral molecules when dissolved in water. The molecular solutes, other than acids and bases, are nonelectrolytes.
Strong and weak electrolytes
Substances that almost 100% dissociate into ions when dissolved in water are strong electrolytes.
- All ionic compounds that are soluble in water are strong electrolytes.
- Strong acids are strong electrolytes.
- Strong bases are ionic compounds and strong electrolytes.
A strong electrolyte does not mean that it is necessarily highly soluble in water. It means that the portion of the solute that dissolves, it also dissociates 100% into ions in water, e.g., all ionic compounds. The solubility Ca(OH)2 is only 0.16 g Ca(OH)2/100 g water at 20 oC, but all the dissolved Ca(OH)2 dissociates into Ca2+ and OH- ions.
Strong bases are hydroxides of alkali metals, i.e., LiOH, NaOH, KOH, RbOH, and CsOH, and hydroxides of heavy alkaline earth metals, i.e., Ca(OH)2, Sr(OH)2 and Ba(OH)2, which are strong electrolytes. Strong acids, i.e., HCl, HBr, HI, HClO4, HNO3, and H2SO4, are molecular compounds but are strong electrolytes because they dissociate almost 100% into ions when dissolved in water. For example, HCl almost wholly dissociates into ions when dissolved in water.
HCl(g)+H2O(l)→H3O1+(aq)+Cl1−(aq)
Substances that partially dissociate into ions when dissolved in water are weak electrolytes. Weak acids and weak bases are weak electrolytes.
Week acids and week bases, like acetic acid (CH3COOH) and ammonia (NH3), are soluble in water, but partially dissociate into ions. For example, if 1-mole acetic acid or 1-mole of ammonia is dissolved in 1 liter of water at room temperature, they establish the following equilibrium between dissolved molecules and dissociated ions:
CH3COOH(l) Water ⟶CH3COOH(aq)+H2O(l)→⟵CH3COO1−(aq)+H3O1+(aq),NH3( g) Water ⟶NH3(aq)+H2O(l)→⟵NH41+(aq)+OH1−(aq),
where only about 0.4% of the dissolved molecules dissociate into ions, the remaining about 99.6% of molecules remain neutral. Weak acids and weak bases are weak electrolytes.
Fig. 5.3.2 illustrates the difference between nonelectrolytes, strong electrolytes, and weak electrolytes.
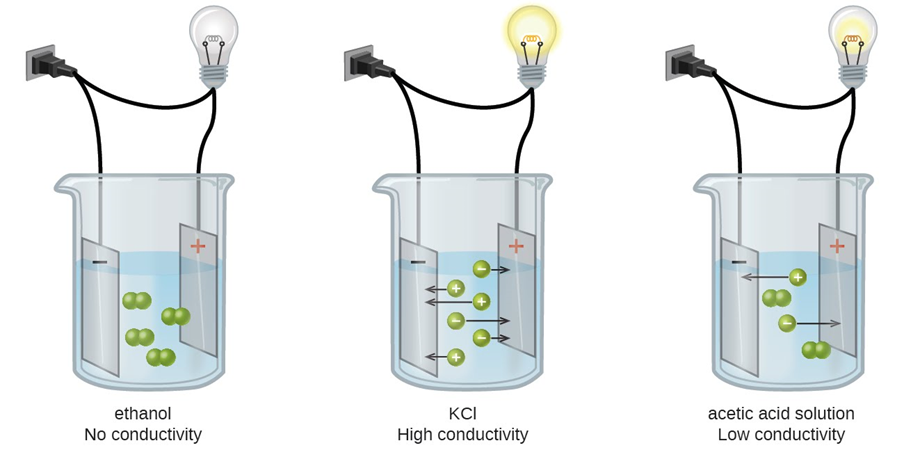
Figure 5.3.2: Illustration of a nonelectrolyte that does not conduct electricity, a strong electrolyte that has high electrical conductivity, and a weak electrolyte that has low electrical conductivity. Source: Karishma50/ (https;//creativecommons.org/licences/by-sa/4.0)
Equivalent
The amount of molecules and atoms is usually measured in moles. Ionic compounds are composed of ions but are overall neutral because the +ve charge is balanced by the –ve charge. Therefore, the mole ratio of cations to anions is not always one to one. For example, NaCl has a one-to-one mole ratio of Na+ and Cl-, but CaCl2 has a one-to-two ratio of Ca2+ and Cl-. A new unit, called equivalent (officially abbreviated as Equiv but commonly abbreviated as Eq), is introduced, to differentiate between a mole of ion and a mole of charge on the ion.
The equivalent is the amount of a substance needed to react with or supply one mole of hydrogen ions (H+) in an acid-base reaction, or react with or supply one mole of electrons in a redox reaction. In other words, the number of equivalents of a given ion in a solution is equal to the number of moles of that ion multiplied by its valence.
1 mol Na+=1 Eq Na+, but 1 mol Ca2+=2 Eq Ca2+, and 1 mol Al3+=3 Ea Al3+
Similarly,
1 mol Cl−=1 Eq Cl−, but1 mol CO32−=2 Eq CO32− and 1 mol PO43−=3 Ea PO43−
The solutions of electrolytes are overall electrically neutral, i.e., the number of equivalents of cations is equal to the number of equivalents of anions in the solution.
For example, if 1 mole of NaCl is dissolved in water, there is 1 Eq of Na+ ions and 1 Eq of Cl- ions in the solution. If one mole of CaCl2 is dissolved in the water there is 2 Eq of Ca2+ ions and 2 Eq of Cl- ions in the solution. Similarly, if 1 mole of NaCl and 1 mole of AlCl3 are dissolved in the water there is 1 Eq of Na+ ions, 3 Eq of Al3+ ions, and 4 Eq of Cl- ions to balance cations in the water.
Equality gives two conversion factors. For example, the equality 1 mol Ca2+ = 2 Eq Ca2+ gives the following two conversion factors:
1 mol Ca2+2 Eq Ca2+
and
2 Ea Ca2+1 mol Ca2+
The first conversion factor converts the given amount in equivalents to moles, and the second converts the given amount in moles to equivalents of the ion as explained in the following examples.
a) Calculate mEq of Fe3+ in 0.0200 mol of Fe3+? b) if chloride ion is the only anion in the solution, how many mEq of Cl- are present in the solution?
Solution
a) Given 0.0200 mol Fe3+. Desired mEq Fe3+.
The equality: 1 mol Fe3+ = 3 Eq Fe3+ gives the conversion factor 3 Eq Fe3+1 mol Fe3+ that converts the given amount in moles to Eq of Fe3+. Another equality: 1 Eq Fe3+ = 1000 mEq Fe3+ gives the conversion factor 1000 mEq Fe3+1 Eq Fe3+, that converts the Eq of Fe3+ to mEq of Fe3+.
Calculations: 0.0200 mol Fe3+×3 Eq Fe3+1 mol Fe3+×1000 mEq Fe3+1 Eq Fe3+=60 mEq Fe3+
An intervenous saline solution contains 145 mEq/L of Na+. How many moles of Na+ are in 0.500 L of the solution?
Solution
a) Given: 145 mEq Na+ /L solution and 0.500 L solution, Desired: ? mol Na+
Conversion factors needed: The concentration in mEq/L is the first factor for L to mEq conversion, the equality 1 mEq Na+ = 1 mmol Na+ givens the second conversion needed for mEq to mmol conversion, and the equality 1000 mmol Na+ = 1 mol Na+ given the third conversion factor needed needed for mEq to mol conversion. The three conversion factors are applied one after the other in a single row in the following calculation:
0.500 L solution ×145 mEq Na1L solution ×1 mmol Na+1 mEq Na+×1 mol Na+1000 mmol Na+=0.0725 mol Na+
Note how the units of the numerator in one fraction are canceled by the units of the denumerator of the following fraction leaving only the desired units uncancelled that become the units of the answer number.
Electrolytes in body fluids
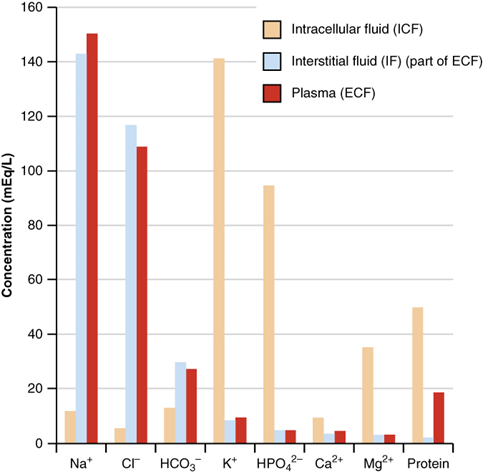
Fig. 5.3.3 shows the electrolytes and their concentrations commonly found in body fluids. They play an essential role in cell and body functions. For example, sodium ions regulate the water content and play a role in electrical impulse transmission in the nervous system. Potassium ions play a role in maintaining a regular heartbeat and also play a part in electrical impulse transmission. Chloride ions are there to balance the charge. Carbonate ions are involved in maintaining the pH of the blood.
Concentrations of electrolytes in body fluids are not high enough to be reported in a mole or equivalent units. The electrolytes in the body fluids are usually reported in millimoles (mmol) or milliequivalent (mEq) units, where: 1000 mmol=1 mol and 1000 mEq=1 Eq.
The overall concentration of electrolytes in intravenous fluids given to patients is about the same as of electrolytes in the body fluids. For example, Ringer’s lactate solution contains about: 130 mEq/L Na+, 4 mEq/L K+, 3 mEq/L Ca2+, 109 mEq/L Cl- , and 28 mEq/L lactate-. Note that the overall +ve charge (130+4+3= 137 mEq/L) is equal to the overall –ve charge (109+28 = 137 mEq/L).