19.E: Transition Metals and Coordination Chemistry (Exercises)
- Page ID
- 44125
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)19.1: Occurrence, Preparation, and Properties of Transition Metals and Their Compounds
Q19.1.1
Write the electron configurations for each of the following elements:
- Sc
- Ti
- Cr
- Fe
- Ru
S19.1.1
The electron configuration of an atom is the representation of the arrangement of electrons distributed among the orbital shells and sub-shells. The electron configuration of each element is unique to its position on the periodic table where the energy level is determined by the period and the number of electrons is given by the atomic number of the element. There are four different types of orbitals (s, p, d, and f) which have different shapes and each orbital can hold a maximum of 2 electrons, but the p, d and f orbitals have different sub-levels, meaning that they are able to hold more electrons.
The periodic table is broken up into groups which we can use to determine orbitals and thus, write electron configurations:
Group 1 & 2: S orbital
Group 13 - 18: P orbital
Group 3 - 12: D orbital
Lanthanide & Actinides: F orbital
Each orbital (s, p, d, f) has a maximum number of electrons it can hold. An easy way to remember the electron maximum of each is to look at the periodic table and count the number of periods in each collection of groups.
Group 1 & 2: 2 (2 electrons total = 1 orbital x max of 2 electrons = 2 electrons)
Group 13 - 18: 6 (6 electrons total = 3 orbitals x 2 electrons max = 6 electrons)
Group 3 - 12: 10 (10 electrons total = 5 orbitals x 2 electrons max = 10 electrons)
Lanthanide & Actinides: 14 (14 electrons total = 7 orbitals x 2 electrons max = 14 electrons)
Electron fills the orbitals in a specific pattern that affects the order in which the long-hand versions are written:
Electron filling pattern: 1s, 2s, 2p, 3s, 3p, 4s, 3d, 4p, 5s, 4d, 5p, 6s, 4f, 5d, 6p, 7s, 5f
An easier and faster way to write electron configurations is to use noble gas configurations as short-cuts. We are able to do this because the electron configurations of the noble gases always have all filled orbitals.
He: 1s22s2
Ne: 1s22s22p6
Ar: 1s22s22p63s23p6
Kr: 1s22s22p63s23p64s23d104p6
Xe: 1s22s22p63s23p64s23d104p65s24d105p6
Rn: 1s22s22p63s23p64s23d104p65s24d105p66s24f145d106p6
The most common noble gas configuration used is Ar. When you want to use the noble gas configuration short-cut, you place the noble gas's symbol inside of brackets:
[Ar]
and then write it preceding the rest of the configuration, which is solely the orbitals the proceed after that of the noble gas.
a. Sc
Let's start off by identifying where Scandium sits on the periodic table: row 4, group 3. This identification is the critical basis we need to write its electron configuration.
By looking at Scandium's atomic number, 21, it gives us both the number of protons and the number of electrons. At the end of writing its electron configuration, the electrons should add up to 21.
At row 4, group 3 Sc, is a transition metal; meaning that its electron configuration will include the D orbital.
Now, we can begin to assign the 21 electrons of Sc to orbitals. As you assign electrons to their orbitals, you move right across the periodic table.
Its first 2 electrons are in the 1s orbital which is denoted as
1s2
where the "1" preceding the s denotes the fact that it is of row one, and it has an exponent of 2 because it fulfills the s orbital's maximum electron number. Now we have 21-2=19 more electrons to assign.
Its next 2 electrons are in the 2s orbital which is denoted as
2s2
where the "2" preceding the s indicates that it is of row two, and it has an exponent of 2 because it fulfills the s orbital's maximum electron number. Now we have 19-2=17 more electrons to assign.
Its next 6 electrons are in the 2p orbital which is denoted as
2p6
where the "2" preceding the p indicates that it is of row two, and it has an exponent of 6 because it fulfills the p orbital's maximum electron number. Now we have 17-6=11 more electrons to assign.
Its next 2 electrons are in the 3s orbital which is denoted as
3s2
where the "3" preceding the s indicates that it is of row three, and it has an exponent of 2 because it fulfills the s orbital's maximum electron number. Now we have 11-2=9 more electrons to assign.
Its next 6 electrons are in the 3p orbital which is denoted as
3p6
where the "3" preceding the p indicates that it is of row three, and it has an exponent of 6 because it fulfills the p orbital's maximum electron number. Now we have 9-6=3 more electrons to assign.
Its next 2 electrons are in the 4s orbital which is denoted as
4s2
where the "4" preceding the s indicates that it is of row four, and it has an exponent of 2 because it fulfills the s orbital's maximum electron number. Now we have 3-2=1 more electron to assign.
Its last electron would be alone in the 3 d orbital which is denoted as
3d1
where the "3" preceding the d indicates that, even though it is technically of row 4, by disregarding the first row of H and He, this is the third row and it has an exponent of 1 because there is only 1 electron to be placed in the d orbital. Now we have assigned all of the electrons to the appropriate orbitals and sub-orbitals, so that the final, entire electron configuration is written as:
1s22s22p63s23p64s23d1
This is the long-hand version of its electron configuration.
So for Sc, its short-hand version of its electron configuration would therefore be:
[Ar] 4s23d1
b. Ti
Start off by identifying where Titanium sits on the periodic table: row 4, group 4, meaning it has 22 electrons total. Titanium is one element to the right of the previous problem's Sc, so we will basically use the same method except, in the end, there will be 2 electrons remaining, so therefore the final orbital will be denoted as:
3d2
If needed, look above to the exact steps for how to do it in detail again; the long-hand electron configuration for Titanium will be:
1s22s22p63s23p64s23d2
So for Ti, its short-hand version of its electron configuration would therefore be:
[Ar] 4s23d2
c. Cr
Start off by identifying where Chromium sits on the periodic table: row 4, group 6, that means it has a total of 24 electrons. But first, Cr, along with Mo, Nb, Ru, Rh, Pd, Cu, Sg, Pt and Au, is a special case. You would think that since it has 24 electrons that its configuration would look like:
1s22s22p63s23p64s23d4
which is how we learned it earlier. However, this electron configuration is very unstable because of the fact that there are 4 electrons in its 3 d orbital. The most stable configurations are half-filled (d5) and full orbitals (d10), so the elements with electrons resulting in ending with the d4 or d9 are so unstable that we write its stable form instead, where an electron from the preceding s orbital will be moved to fill the d orbital, resulting in a stable orbital.
If needed, look above to the exact steps for how to do the beginning of the configuration in detail again. However we have to apple the new rule to attain stability so that the long-hand electron configuration for Chromium will be:
1s22s22p63s23p64s13d5
So for Cr, its short-hand version of its electron configuration would therefore be:
[Ar] 4s13d5
d. Fe
Start off by identifying where Iron sits on the periodic table: row 4, group 8, meaning it has 26 electrons total. This is 5 elements to the right of the previous problem's Sc, so we will basically use the same method except, in the end, there will be 6 electrons remaining, so therefore the final orbital will be denoted as:
3d6
If needed, look above to the exact steps for how to do it in detail again; the long-hand electron configuration for Iron will be:
1s22s22p63s23p64s23d6
So for Fe, its short-hand version of its electron configuration would therefore be:
[Ar] 4s23d6
e. Ru
Start off by identifying where Ruthenium sits on the periodic table: row 5, group 8, that means it has a total of 44 electrons. But first, as stated earlier, Ru, along with Cr, Mo, Nb, Rh, Pd, Cu, Sg, Pt and Au, is a special case. You would think that since it has 44 electrons that its configuration would look like:
1s22s22p63s23p64s23d104p65s2 4d6
which is how we learned it earlier. However, this electron configuration is very unstable because of the fact that, even though there are 4 paired electrons, there are also 4 electrons unpaired. This results in a very unstable configuration, so to restore stability, we have to use a configuration that has the most paired electrons, which would be to take an electron from the s orbital and place it in the d orbital to create:
5s14d7
If needed, look above to the exact steps for how to do the beginning of the configuration in detail again. However we have to apple the new rule to attain stability so that the long-hand electron configuration for Ru will be:
1s22s22p63s23p64s23d104p65s14d7
So for Cr, its short-hand version of its electron configuration would therefore be:
[Kr] 5s14d7
A19.1.1
- Sc: [Ar]4s23d1
- Ti: [Ar]4s23d2
- Cr: [Ar]4s13d5
- Fe: [Ar]4s23d6
- Ru: [Kr]5s14d7 (anomalous configuration)
Q19.1.2
Write the electron configurations for each of the following elements and its ions:
- Ti
- Ti2+
- Ti3+
- Ti4+
S19.1.2
Electrons are distributed into molecular orbitals, the \(s, p, d, and f\) blocks. An orbital will have a number in front of it and a letter that corresponds to the block. The s block holds two electrons, the p block holds six, the d block holds ten, and the f block holds fourteen. So, based on the number of electrons an atom has, the molecular orbitals are filled up in a certain way. The order of the orbitals is \(1s, 2s, 2p, 3s, 3p, 4s, 3d, 4p, 5s, 4d, 5p, 6s, 4f, 5d, 6p, 7s, 5f, 6d, 7p\). An exponent will be put after the letter for each orbital to signify how many electrons are in that orbital. Noble gas notation can also be used by putting the noble gas prior to the element you are writing the configuration for, and then proceed by writing the orbitals filled after the noble gas. Metal ions of the d-block will have the two electrons removed from the s block prior to any electrons being removed from the proceeding d-block.
Solutions:
1. \(Ti\)
Titanium has an atomic number of 22, meaning it has 22 electrons. The noble gas prior to Titanium is Argon. Looking at row 4 of the periodic table, Titanium still has 4 electrons to be placed in orbitals since Argon has 18 electrons that are already placed. The remaining electrons will fill the \(4s\) orbital and the remaining two electrons will go into the \(3d\) orbital. [Ar]4s23d2
2. \(Ti^{+2}\)
This is an ion with a plus 2 charge, meaning 2 electrons have been removed. The electrons will be removed from the \(4s\) orbital and the 2 remaining electrons will be placed in the \(3d\) orbital. Like number 1, the prior noble gas is Argon. [Ar]3d2
3. \(Ti^{+3}\)
This is an ion with a plus 3 charge, meaning 3 electrons have been removed. The first 2 electrons will be removed from the \(4s\) orbital, and the third will be taken from the \(3d\) orbital, and the 1 remaining electron will be placed in the 3d orbital. Like number 1, the prior noble gas is Argon. [Ar]3d1
4. \(Ti^{+4}\)
This is an ion with a plus 4 charge, meaning 4 electrons have been removed. The first 2 electrons will be removed from the \(4s\) orbital and the second 2 will be removed from the \(3d\) orbital. This results in the ion having the same electron configuration as Argon. [Ar]
Answers:
- \([Ar]4s^23d^2\)
- \([Ar]3d^2\)
- \([Ar]3d^1\)
- \([Ar]\)
A19.1.2
- \([Ar]4s^2 3d^2\)
- \([Ar]3d^2\)
- \([Ar]3d^1\)
- \([Ar]\)
Q19.1.3
Write the electron configurations for each of the following elements and its 3+ ions:
- La
- Sm
- Lu
S19.1.3
In order to write the electron configuration, we begin by finding the element on the periodic table. Since La, Sm, and Lu are all a period below the noble gas Xenon, we can abbreviate \({1s^2}{2s^2}{2p^6}{3s^2}{3p^6}{3d^{10}}{4s^2}{4p^6}{4d^{10}}{5s^2}{5p^6}\) as [Xe] when writing the orbital configurations. We then find the remaining of the orbital configurations using the Aufbau Principle. For other elements not just those in period 6, the shorthand notation using noble gases would be the noble gas in the period above the given element.
1. La has three additional electrons. Two of them fill the 6s shell and the other single electron is placed on the 5d shell.
\(La:\) [Xe] \({6{s}^2} {5{d}^1}\)
2. Sm has eight more electrons. The 6s orbital is filled as previously and the 4f orbital receives 6 electrons because pairing electrons requires lower energy on the 4f shell than on the 5d shell.
\(Sm:\) [Xe] \({6{s}^2} {4{f}^6}\)
3. Lu has seventeen more electrons. Two electrons fill the 6s orbital, 14 electrons fill the 4f orbital, and extra single electron goes to the 5d orbital .
\(Lu:\) [Xe] \({6s^2}{4f^{14}}{5d^1}\)
To find the 3+ ion electron configuration, we remove 3 electrons from the neutral configuration, starting with the 6s orbital.
1. The ionization of La3+ removes the three extra electrons. So it reverts back to the stable Xenon configuration.
\({La^{3+}:}\) [Xe]
2. The ionization of Sm3+ removes two electrons from the 6s shell and one from the outermost (4f) shell
\({Sm^{3+}}:\) [Xe] \({4f^5}\)
3. The ionization of Lu3+ removes its two 6s shell and one from the outermost (5d) shell, leaving only a full 4f shell
\(Lu^{3+}:\) [Xe] \(4f^{14}\)
A19.1.3
La: [Xe]6s 25d 1, La3+: [Xe]; Sm: [Xe]6s 24f 6, Sm3+: [Xe]4f 5; Lu: [Xe]6s 24f 145d 1, Lu3+: [Xe]4f 14
Q19.1.4
Why are the lanthanoid elements not found in nature in their elemental forms?
A19.1.4
Lanthanides are rarely found in their elemental forms because they readily give their electrons to other more electronegative elements, forming compounds instead of staying in a pure elemental form. They have very similar chemical properties with one another, are often found deep within the earth, and difficult to extract. They are the inner transition elements and have partially filled d orbitals that can donate electrons. Because of this, they are very reactive and electropositive.
Q19.1.5
Which of the following elements is most likely to be used to prepare La by the reduction of La2O3: Al, C, or Fe? Why?
S19.1.5
An activity series is a list of elements in decreasing order of their reactivity. Elements on the top of the list are good reducing agents because they easily give up an electron, and elements on the bottom of the series are good oxidizing agents because they are highly electronegative would really want to accept an electron.
Step 1: Compare Aluminum, Carbon, and Iron on an activity series. Many activity series include carbon and hydrogen as references. An activity series can be found here
The activity series goes in the order (from top to bottom): Aluminum, Carbon, and Iron.
Step 2: Identify which element is the best reducing agent.
Elements on the top of the list are the best reducing agents, because they give up electrons the best.
Aluminum is the best reducing agent of the options available.
Therefore aluminum will be the best reducing agent to prepare La by the reduction of La2O3 because it is the most reactive in the series amongst the three elements.
A19.1.5
Al is used because it is the strongest reducing agent and the only option listed that can provide sufficient driving force to convert La(III) into La.
Q19.1.6
Which of the following is the strongest oxidizing agent: \(\ce{VO4^{3-}}\), \(\ce{CrO4^2-}\), or \(\ce{MnO4-}\)?
S19.1.6
Oxidizing agents oxidize other substances. In other words, they gain electrons or become reduced. These agents should be in their highest oxidation state. In order to determine, the strength of the compounds above as oxidizing agents, determine the oxidation numbers of each constituent elements.
\(\\\mathrm{VO_4^{3-}}\)
We know that \(\mathrm{O}\) has a -2 oxidation state and the overall charge of the ion is -3. We just need to determine Vanadate's oxidation number in this compound.
\(\\\mathrm{V} + \mathrm{-2(4)} = \mathrm{-3}\)
\(\\\mathrm{V} = \mathrm{+5}\)
Vanadate has an oxidation number of +5, which is its highest possible oxidation state.
\(\\\mathrm{CrO_4^{2-}}\)
Like in the previous calculation, \(\mathrm{O}\) has a -2 oxidation state. The overall charge is -2. So calculate for chromium.
\(\\\mathrm{Cr} + \mathrm{-2(4)} = \mathrm{-2}\)
\(\\\mathrm{Cr} = \mathrm{+6}\)
Chromium is in its highest possible oxidation state of +6 in this compound.
\(\\\mathrm{MnO_4^-}\)
\(\mathrm{O}\) has a -2 oxidation state and the overall charge is -1.
\(\\\mathrm{Mn} + \mathrm{-2(4)} = \mathrm{-1}\)
\(\\\mathrm{Mn} = \mathrm{+7}\)
Manganese is also in its highest oxidation state, +7.
An oxidizing agent has to be able to gain electrons which, in turn, reduces its oxidation state. Here manganese has the greatest oxidation state which allows it to experience a greater decrease in its oxidation state if needed, meaning it can gain the most electrons. So among the three compounds, \(\mathrm{MnO_4^-}\) is the strongest oxidizing agent. This method assumes the metals have similar electronegativities.
Alternatively, check a redox table.
A19.1.6
\(MnO_4^-\)
Q19.1.7
Which of the following elements is most likely to form an oxide with the formula MO3: Zr, Nb, or Mo?
S19.1.7
Mo because Zr has an oxidation state of +4 and Nb has a oxidation state of +5 and those would not balance out the charge of 3 oxygens in the state of -2 which creates a charge of -6. Mo however has multiple oxidation states, the most common being +6 which balances out the -6 charge created by 3 oxygen ions. This is why its most likely to form an oxide with the formula MO3 or \(\ce{MoO3}\).
A19.1.7
Mo
Q19.1.8
The following reactions all occur in a blast furnace. Which of these are redox reactions?
- \(\ce{3Fe2O3}(s)+\ce{CO}(g)⟶\ce{2Fe3O4}(s)+\ce{CO2}(g)\)
- \(\ce{Fe3O4}(s)+\ce{CO}(g)⟶\ce{3FeO}(s)+\ce{CO2}(g)\)
- \(\ce{FeO}(s)+\ce{CO}(g)⟶\ce{Fe}(l)+\ce{CO2}(g)\)
- \(\ce{C}(s)+\ce{O2}(g)⟶\ce{CO2}(g)\)
- \(\ce{C}(s)+\ce{CO2}(g)⟶\ce{2CO}(g)\)
- \(\ce{CaCO3}(s)⟶\ce{CaO}(s)+\ce{CO2}(g)\)
- \(\ce{CaO}(s)+\ce{SiO2}(s)⟶\ce{CaSiO3}(l)\)
S19.1.8
o identify redox reaction, we have to determine if have to see if the equation is an oxidation-reduction reaction-meaning that the species are changing oxidation states during the reaction, which involves the transfer of electrons between two species. If a species is losing electrons, then that species is being oxidized. If a species is gaining electrons, then that species is being reduced. A way to remember this is using the acronyms OIL RIG. Oxidation Is Loss, and Reduction Is Gain, referring to electrons. Both of these must occur for an equation to be a redox reaction. Let's see if these equations are redox reactions or not:
a. In the reactants side \(\ce{Fe2O3}\), Fe is has an oxidation number of +3. In the product \(\ce{Fe3O4}\), Fe has an oxidation number of +2.67. Since Fe changed from +3 to +2.67, we can say that Fe had gained electrons and therefore reduced. In the reactant, CO, carbon has an oxidation number of +2, and in \(\ce{CO2}\) (product) carbon has an oxidation number of +4. Therefore, carbon has lost electrons and it has been oxidized. Since there is oxidation and reduction of species- we can conclude that this is a redox reaction.
b. In the reactant, \(\ce{Fe3O4}\), Fe has an oxidation number of +2.67. In the product, FeO, Fe has an oxidation number of +2. Since the oxidation of Fe has changed from +2.67 to +2, electrons have been added therefore Fe has been reduced. In the reactant, CO, carbon has an oxidation number of +2, and in \(\ce{CO2}\) (product) carbon has an oxidation number of +4. Therefore, carbon has lost electrons and it has been oxidized. Since there is oxidation and reduction of species- we can conclude that this is a redox reaction.
c. In the reactant side, in FeO, Fe has an oxidation number of +2 and in the products side Fe has an oxidation number of 0. Since the oxidation number of Fe changed from +2 to 0, electrons have been gained and therefore Fe has been reduced. In the reactant, CO, carbon has an oxidation number of +2, and in \(\ce{CO2}\) (product) carbon has an oxidation number of +4. Therefore, carbon has lost electrons and it has been oxidized. Since there is oxidation and reduction of species- we can conclude that this is a redox reaction.
d. In the reactants C has an oxidation number of 0, and in the products side in \(\ce{CO2}\), C has an oxidation number of +4. Since the oxidation number of C has changed from 0 to +4, we can say that C has been oxidized. In the reactants, in \(\ce{O2}\) oxygen has an oxidation number of 0, and in the products CO2, oxygen has an oxidation number of -2. Since the oxidation number of oxygen has changed from 0 to -2, oxygen has been reduced. Since there is oxidation and reduction of species- we can conclude that this is a redox reaction.
e. In the reactants \(\ce{CO2 }\) has an oxidation number of +4, and in the products side in CO, C has an oxidation number of +2. Since carbon went from +4 to +2, carbon has been reduced. In the reactants, in \(\ce{CO2 }\) oxygen has an oxidation number of -4 and in the products CO carbon has an oxidation number of -2. Since oxygen went from -4 to -2, it has been oxidized. Since there is oxidation and reduction of species- we can conclude that this is a redox reaction.
f. In the reactants, \(\ce{CaCO3}\) Ca has an oxidation number of +2, and in products CaO Ca has an oxidation number of +2. Since the oxidization number doesn't change- we can conclude that this equation is not a redox reaction.
g. In the products CaO Ca has an oxidation number of +2, and in the products \(\ce{CaSiO3}\) Ca has an oxidation number of +2. Since the oxidization number doesn't change- we can conclude that this equation is not a redox reaction.
A19.1.8
a, b, c, d, e
Q19.1.9
Why is the formation of slag useful during the smelting of iron?
S19.1.9
Slag is a substance formed as a byproduct of iron ore or iron pellets melting together in a blast furnace. Slag is also the byproduct that is formed when a desired metal has been separated from its raw ore. It is important to note that slag from steel mills is created in a manner that reduces the loss of the desired iron ore. The \(\ce{CaSiO3}\)slag is less dense than the molten iron, so it can easily be separated. Also, the floating slag layer creates a barrier that prevents the molten iron from exposure to \(\ce{O2}\), which would oxidize the \(\ce{Fe}\) back to \(\ce{Fe2O3}\). Since Fe has a low reduction potential of -0.440 this means it has a high oxidation potential so it would easily oxidize in the presence of O2. Creating a barrier between iron and oxygen allows the maximum product of iron to be obtained in the end of smelting.
A19.1.9
The CaSiO3 slag is less dense than the molten iron, so it can easily be separated. Also, the floating slag layer creates a barrier that prevents the molten iron from exposure to O2, which would oxidize the Fe back to Fe2O3.
Q19.1.10
Would you expect an aqueous manganese(VII) oxide solution to have a pH greater or less than 7.0? Justify your answer.
S19.1.10
Manganese(VII) oxide, can be written as Mn2O7.
In relation to the Lewis acid-base theory, a Lewis acid accepts lone pair electrons, and is also known as the electron pair acceptor. Based on this theory, acidity can be measured by the element's ability to accept electron pairs. By doing the math, we find that Manganese has an oxidation state of +7 (Oxygen has an oxidation state of -2, and 2x-7=-14 or this can be shown as \(-7(2)+2(x)=0\) and \(x=7\) since the whole compound has a charge of zero, in order to balance the ion's charge, Mn must be +7). Therefore Mn has high capability of accepting electrons due to its high positive charge. For most metals, as the oxidation number increases, so does its acidity, because of its increased ability to accept electrons.
A19.1.10
In relation to the Lewis acid-base theory, the Lewis acid accepts lone pair electrons; thus, it is also known as the electron pair acceptor. This may be any chemical species. Acids are substances that must be lower than 7. Therefore, oxides of manganese is most likely going to become more acidic in (aq) solutions if the oxidation number increases.
Q19.1.11
Iron(II) can be oxidized to iron(III) by dichromate ion, which is reduced to chromium(III) in acid solution. A 2.5000-g sample of iron ore is dissolved and the iron converted into iron(II). Exactly 19.17 mL of 0.0100 M Na2Cr2O7 is required in the titration. What percentage of the ore sample was iron?
S19.1.11
To answer this question, we must first identify the net ionic equation from the given half-reactions. We can write the oxidation and reduction half-reactions:
\[ \text{ oxidation:} \text{ Fe}^\text{ 2+} \rightarrow \text{ Fe}^\text{ 3+} \]
\[ \text{ reduction: }\ce{Cr2O7^2-} \rightarrow \text{ Cr}^\text{ 3+} \]
We can quickly balance the oxidation half-reaction by adding the appropriate number of electrons to get
\[ \text{ Fe}^\text{ 2+} \rightarrow \text{ Fe}^\text{ 3+}+\text{ e}^- \]
The first step in balancing the reduction half-reaction is to balance elements in the equation other than O and H. In doing so, we get
\[\ce{Cr2O7^2-} \rightarrow 2 \text{ Cr}^\text{ 3+} \]
The second step would be to add enough water molecules to balance the oxygen.
\[ \ce{Cr2O7^2-} \rightarrow 2 \text{ Cr}^\text{ 3+}+ \ce{7H2O} \]
Next, we add the correct amount of H+ to balance the hydrogen atoms.
\[ \ce{Cr2O7^2-}+14 \text{ H}^+ \rightarrow 2 \text{ Cr}^\text{ 3+}+ \ce{7H2O} \]
Finally, we add enough electrons to balance charge.
\[ \ce{Cr2O7^2-}+14 \text{ H}^++6 \text{ e}^- \rightarrow 2 \text{ Cr}^\text{ 3+}+ \ce{7H2O} \]
The electrons involved in both half-reactions must be equal in order for us to combine the two to get the net ionic equation. This can be done by multiplying each equation by the appropriate coefficient. Scaling the oxidation half-reaction by 6, we get
\[ 6 \text{ Fe}^\text{ 2+} \rightarrow 6 \text{ Fe}^\text{ 3+}+6 \text{ e}^- \]
Now we can combine both half-reactions to get
\[ 6 \text{ Fe}^\text{ 2+}+\ce{Cr2O7^2-}+14 \text{ H}^++6 \text{ e}^- \rightarrow 6 \text{ Fe}^\text{ 3+}+6 \text{ e}^-+2 \text{ Cr}^\text{ 3+}+ \ce{7H2O}\]
The electrons cancel out, so you get:
\[ 6 \text{ Fe}^\text{ 2+}+\ce{Cr2O7^2-}+14 \text{ H}^+ \rightarrow 6 \text{ Fe}^\text{ 3+}+2 \text{ Cr}^\text{ 3+}+ \ce{7H2O}\]
From this we can see that the mole ratio of Cr2O72- to Fe2+ is 1:6. Given that 19.17 mL (or 0.01917 L) of 0.01 M Na2Cr2O7 was needed for titration we know that
\[ 0.01917\text{ L} \times 0.01 \text{ M} = 1.917 \times 10^{-4} \text{ mol} \]
of Na2Cr2O7 reacted. Also, since any number of moles of Na2Cr2O7 produces the same number of moles of Cr2O72- in solution
\[ 1.917 \times 10^{-4} \text{ mol} \text{ of } \ce{Na2Cr2O7} =1.917 \times 10^{-4} \text{ mol} \text{ of } \ce{Cr2O7^2-}\]
We can use the mole ratio of Cr2O72- to Fe2+ to determine how many moles of iron (ii) was in the solution. The number of moles of iron (ii) is the same as the number of moles of pure iron in the sample since all of the iron was converted into iron (ii).
\[ 1.917 \times 10^{-4} \text{ mol} \text{ of } \ce{Cr2O7^2-}\times \frac{6\text{ mol}\text{ of } \text{ Fe}^\text{ 2+}}{1\text{ mol}\text{ of }\ce{Cr2O7^2-}} = 0.0011502\text{ mol}\text{ of } \text{ Fe}^\text{ 2+} \]
\[ 0.0011502\text{ mol}\text{ of } \text{ Fe}^\text{ 2+} = 0.0011502\text{ mol}\text{ of } \text{ Fe} \]
Now we can find the number of grams of iron that were present in the 2.5 g iron ore sample.
\[ 0.0011502\text{ mol}\text{ of } \text{ Fe}\times\frac{55.847\text { g}}{1\text{ mol}} = 0.0642352194\text{ g}\text{ of }\text{ Fe} \]
Finally, we can answer the question and find what percentage of the ore sample was iron.
\[ \frac{0.0642352194\text{ g}}{2.5\text{ g}} \times 100 \approx 2.57\text{%} \]
So 2.57% of the ore sample was iron.
A19.1.11
2.57%
Q19.1.12
How many cubic feet of air at a pressure of 760 torr and 0 °C is required per ton of Fe2O3 to convert that Fe2O3 into iron in a blast furnace? For this exercise, assume air is 19% oxygen by volume.
S19.1.12
This question uses a series of unit conversions and the \(PV=nRT\) equation.
The first step is to write out the balanced chemical equation for the conversion of Fe2O3 to pure iron.
\[2\;Fe_2O_3(s)\rightarrow 4\;Fe(s)+3\;O_2(g)\]
Next, we need to analyze the original question to determine the value that we need to solve for. Because the question asks for a value of cubic feet, we know we need to solve for volume. We can manipulate \(PV=nRT\) to solve for volume.
\[V={nRT}/P\]
Now determine the known variables and convert into units that will be easy to deal with.
\[n = 2000\:lbs\; Fe_2O_3\frac{453.592\: grams\: Fe_2O_3}{1\: lb \:Fe_2O_3}\frac{1\: mole \:Fe_2O_3}{159.69\: grams \:Fe_2O_3}\frac{3 \;moles\: O_2}{2\; moles \:Fe_2O_3}\]
\[n=8521\: moles\: of \:O_2\]
Convert to atm for easier calculations
\[R=\frac{.0821\:L\:atm}{mol\:K}\]
\[T=0^{\circ}C=273\:K\]
\[P=760 \:torr= 1 \:atm\]
Now plug the numbers into the manipulated gas law to get to an answer for V.
\[V=190991.8\: liters \:of\: O_2\]
From here we convert liters to cubic feet.
use the conversion
\[1\;L=.0353 ft^3\]
thus we have 6744.811 ft3 of O2
We then refer back to the initial question and remember that this value is only 19% of the volume of the total air. So use a simple equation to determine the total volume of air in cubic feet.
\[6744.811ft^3=.19x\]
x=35499 ft3 of air
A19.1.12
35499 ft3 of air
Q19.1.13
Find the potentials of the following electrochemical cell:
Cd | Cd2+ (M = 0.10) ‖ Ni2+ (M = 0.50) | Ni
S19.1.13
Step 1 Write out your two half reactions and identify which is oxidation and which is reduction using the acronym OIL RIG to remember that oxidation is loss of electrons and reduction is gain of electrons
Cd(s)⟶Cd2+(aq)+2e- (oxidation)
Ni2+(aq)+2e-⟶Ni(s) (reduction)
Step 2 Write out complete balanced equation
Cd(s)+ Ni2+(aq)⟶Cd2+(aq)+Ni(s)
Step 3 Find Eocell
Eocell = Ecathode-Eanode
oxidation: Cd(s)⟶Cd2+(aq)+2e- Eo=-0.40V
reduction: Ni2+(aq)+2e-⟶Ni(s) Eo=-0.26V
* E values come from standard reduction potentials table given above. Also, remember anode is where oxidation happens, and cathode is where reduction happens.
Eocell=-0.26-(-.40)
Eocell=0.14V
Step 4 Find Q
Q=[products]/[reactants] (look at complete balanced equation) (remember that [x] means the concentration of x typically given in molarity and that we ignore solids or liquids)
Q=[Cd2+]/[Ni2+]
Q=0.10/0.50
Q=0.2
Step 5 Calculate E using E= Eocell-(.0592/n)logQ (n is number of moles of electrons transferred and in our case the balanced reaction transfers 2 electrons)
E= 0.14-(.0592/2)log(0.2)
E= 0.14-(-.207)
E=0.16 V
A19.1.13
0.16 V
Q19.1.14
A 2.5624-g sample of a pure solid alkali metal chloride is dissolved in water and treated with excess silver nitrate. The resulting precipitate, filtered and dried, weighs 3.03707 g. What was the percent by mass of chloride ion in the original compound? What is the identity of the salt?
S19.1.14
A 2.5624-g sample of a pure solid alkali metal chloride is dissolved in water and treated with excess silver nitrate. The resulting precipitate, filtered and dried, weighs 3.03707 g. What was the percent by mass of chloride ion in the original compound? What is the identity of the salt?
Assuming that metal chloride is XCl
The balance equation for the reaction would be:
\[XCl(aq)+AgNO_{3}(aq)\rightarrow XNO_{3}(aq) +AgCl(s)\]
The mass of AgCl = 3.03707g
To find the moles of AgCl present:
Next, we can determine the moles of AgCl present in the reaction since 1) the mass of the precipitate is given to us and 2) this value can help us determine the moles of alkali metal chloride compound present. Given the mass of AgCl is 3.03707g in the problem and the molecular mass of AgCl per mole is 143.32g, we can solve for how many moles of AgCl is in the reaction:
\[moles of Agcl=\tfrac{3.03707g}{143.32g/mol}=0.0211 mol\]
Since the molar ratio of the compounds are 1:1 so the number of moles of XCl used = 0.0211 mol
We can calculate the weight of Cl- with the equation:
\[0.0211 mol \times 35.5g/mol = 0.7490g\]
the amount of metal present in the original compound is the weight of the compound subtracted by weight of the Cl ion:
\[(2.5624- 0.7490)g= 1.8134g\]
And the percentage can be calculate by
\[\frac{0.7490}{2.5624}\times 100= 29.23 \%\]
the molar ratio of XCl is 1:1 so then
Atomic mass of metal = \(=\frac{1.8134\;g\; metal}{0.0211\; mol\; RbCl}=85.943g/mol\)
So the atomic mass is 85.943 g/mol which is of Rb hence the identity of the salt is RbCl
Q19.1.15
The standard reduction potential for the reaction \(\ce{[Co(H2O)6]^3+}(aq)+\ce{e-}⟶\ce{[Co(H2O)6]^2+}(aq)\) is about 1.8 V. The reduction potential for the reaction \(\ce{[Co(NH3)6]^3+}(aq)+\ce{e-}⟶\ce{[Co(NH3)6]^2+}(aq)\) is +0.1 V. Calculate the cell potentials to show whether the complex ions, [Co(H2O)6]2+ and/or [Co(NH3)6]2+, can be oxidized to the corresponding cobalt(III) complex by oxygen.
S19.1.15
To calculate the cell potential, we need to know the potentials for each half reaction. After doing so, we need to determine which one is being oxidized and which one is being reduced. The one that is oxidized is the anode and the one that is reduced is the cathode. To find the cell potential, you use this formula and the reduction potential values found in a reduction potential table. If E°cell is positive, \(\Delta\)G is negative and the reaction is spontaneous.
E°cell= E°cathode - E°anode
Because it states that \([Co(H_{2}O)_{6}]^{3+}\) will be oxidized, this means it is the anode.
\(O_{2}(g) + 4 H^{+}(aq) + 4 e^{-} \rightarrow 2 H_{2}O\) +1.229 V
\(O_{2}\) is being reduced, so it is the cathode.
1.229V - 1.8V= -.571 V, or -0.6 V using significant figures. This cannot happen spontaneously because E°cell is negative.
For \([Co(NH_{3})_{6}]^{3+}\), it is again being oxidized, meaning it’s the anode.
1.229-.1= 1.129 V or 1.1 V using significant figures. This reaction is spontaneous because E°cell is positive.
A19.1.15
a) E ° = −0.6 V, E ° is negative so this reduction is not spontaneous.
b) E ° = +1.1 V, E ° is positive so this reduction is spontaneous.
Q19.1.16
Predict the products of each of the following reactions. (Note: In addition to using the information in this chapter, also use the knowledge you have accumulated at this stage of your study, including information on the prediction of reaction products.)
- \(\ce{MnCO3}(s)+\ce{HI}(aq)⟶\)
- \(\ce{CoO}(s)+\ce{O2}(g)⟶\)
- \(\ce{La}(s)+\ce{O2}(g)⟶\)
- \(\ce{V}(s)+\ce{VCl4}(s)⟶\)
- \(\ce{Co}(s)+xs\ce{F2}(g)⟶\)
- \(\ce{CrO3}(s)+\ce{CsOH}(aq)⟶\)
S19.1.16
There is a myriad of reactions that can occur, which include: single replacement, double replacement, combustion, acid-base/neutralization, decomposition or synthesis. The first step to determine the products of a reaction is to identify the type of reaction. From then on, the next steps you take to predict the products will vary based on the reaction type.
- This reaction is a double displacement reaction, in which the cations and anions of the reactants switch places to form new compounds. Writing out the equation in terms of it's aqueous ions will help you visualize what exactly is getting moved around:
\[2H^{+}(aq)+2I^{-}(aq)\rightarrow 2H^{+}(aq)+CO_{3}^{2-}(aq)+Mn^{2+}(aq)+2I^{-}(aq)\]
In this case, the hydrogen cations will recombine with carbonate anions whilst manganese cations will recombine with iodide anions giving us the following equation:
\[\ce{MnCO3}(s)+\ce{2HI}(aq)⟶\ce{MnI2}(aq)+\ce{H2CO3}(aq)\]
This is still not the final answer however, as carbonic acid is unstable and decomposes to carbon dioxide and water under standard conditions. Taking this into account, our final equation is:
\[\ce{MnCO3}(s)+\ce{2HI}(aq)⟶\ce{MnI2}(aq)+\ce{CO2}(g)+\ce{H2O}(l)\] - This reaction is a synthesis reaction, in which two or more reactants combine to form a more complex compound. In this case we are also reacting a metal oxide with oxygen which would result in another metal oxide as the product. The resulting product would be the mixed valence oxide Co3O4 in which one cobalt atom has a +2 oxidation state whilst the other two have a +3 oxidation state. Now all is left is to balance the equation:
\[\ce{6CoO}(s)+\ce{O2}(g)⟶\ce{2Co3O4}(s)\] - Like equation 2, this reaction is also a synthesis reaction involving a metal and oxygen which should result in the formation of a metal oxide. It is a matter now of balancing the oxidation states to attain a neutral compound. Oxygen will always hold a -2 oxidation state in compounds whilst lanthanum will always exhibit a +3 oxidation state. As such, a combination of 2 lanthanum atoms with a +3 oxidation state and 3 oxygen atoms with a -2 oxidation state will give us a molecule with an overall charge of 0 (3(-2)+2(+3)=0). We know our product now, La2O3, and now just need to balance the overall equation, giving us:
\[\ce{4La}(s)+\ce{3O2}(g)⟶\ce{2La2O3}(s)\] - This reaction is slightly harder to define as it encapsulates both the properties of synthesis and decomposition reactions, wherein vanadium reacts with vanadium tetrachloride to produce vanadium trichloride. This reaction however is primarily a synthesis reaction since we are combining two reactants to produce one complex compound. With vanadium trichloride as our product, we can balance the equation:
\[\ce{V}(s)+\ce{3VCl4}(s)⟶\ce{4VCl3}(s)\] - This is another synthesis reaction combining cobalt and fluorine. This equation includes the "xs" notation in front of fluorine which is short for 'excess', meaning more fluorine than actually required is present in the reactants, ensuring the reaction goes to completion. Finding the products if a simple matter of combining the cobalt and fluorine into one molecule, which already leaves us with a balanced equation:
\[\ce{Co}(s)+xs\ce{F2}(g)⟶\ce{CoF2}(s)\] - It may not be obvious here, but the reaction we've been given here is actually an acid-base/neutralization reaction, with chromium trioxide acting as the acid and cesium hydroxide as the base. Chromium trioxide is referred to as an acidic oxide which means that it will react with water to form an acid. Note that this reaction can still proceed even if the reactants aren't in the same phases. The basic rule for acid-base/neutralization reactions is they form a salt (salt being the general term for any ionic compound formed from acid-base reactions) and water. Since we know water is one of our products, our other product must be a salt composed of cesium, chromium and oxygen. Thus, our other product should be cesium chromate, and you can now balance the equation accordingly:
\[\ce{CrO3}(s)+\ce{2CsOH}(aq)⟶\ce{Cs2CrO4}(aq)+\ce{H2O}\]
A19.1.16
- \(\ce{MnCO3}(s)+\ce{2HI}(aq)⟶\ce{MnI2}(aq)+\ce{CO2}(g)+\ce{H2O}(l)\)
- \(\ce{6CoO}(s)+\ce{O2}(g)⟶\ce{2Co3O4}(s)\)
- \(\ce{4La}(s)+\ce{3O2}(g)⟶\ce{2La2O3}(s)\)
- \(\ce{V}(s)+\ce{3VCl4}(s)⟶\ce{4VCl3}(s)\)
- \(\ce{Co}(s)+xs\ce{F2}(g)⟶\ce{CoF2}(s)\)
- \(\ce{CrO3}(s)+\ce{2CsOH}(aq)⟶\ce{Cs2CrO4}(aq)+\ce{H2O}\)
Q19.1.17
Predict the products of each of the following reactions. (Note: In addition to using the information in this chapter, also use the knowledge you have accumulated at this stage of your study, including information on the prediction of reaction products.)
- \(\ce{Fe}(s)+\ce{H2SO4}(aq)⟶\)
- \(\ce{FeCl3}(aq)+\ce{NaOH}(aq)⟶\)
- \(\ce{Mn(OH)2}(s)+\ce{HBr}(aq)⟶\)
- \(\ce{Cr}(s)+\ce{O2}(g)⟶\)
- \(\ce{Mn2O3}(s)+\ce{HCl}(aq)⟶\)
- \(\ce{Ti}(s)+xs\ce{F2}(g)⟶\)
S19.1.17
Predict the products of each of the following reactions.
- Fe(s)+H2SO4(aq)⟶ ?
Whenever a metal reacts with an acid, the products are salt and hydrogen. Because Fe is lower on the activity series, we know that when it reacts with an acid it will result in the formation of Hydrogen gas. To simplify the equation is:
\[Metal + Acid ⟶ Salt + Hydrogen\]
The salt produced will depend on the metal and in this case, the metal is iron (Fe) so the resulting equation would be:
\[\ce{Fe}(s)+\ce{H2SO4}(aq)⟶ \ce{FeSO4}(aq) + \ce{H2}(g)\]
This equation works out as the H2 is removed from H2SO2, resulting in a SO42- ion where Fe will take on an oxidation state of Fe+2 to form FeSO4 which will be the salt in this example.
But since FeSO4 and H2SO4 are aqueous, the reactants and products can also be written as its ions where the overall equation can be:
\[\ce{Fe}(s)+\ce{2H3O+}(aq)+\ce{SO2^{−4}}(aq)⟶\ce{Fe2+}(aq)+\ce{SO2^{−4}}(aq)+\ce{H2}(g)+\ce{2H2O}(l)\]
- FeCl3(aq)+NaOH(aq)⟶ ?
In this case, adding a metal hydroxide (NaOH) to a solution with a transition metal ion (Fe) will form a transition metal hydroxide (XOH). As iron is bonded to three chlorine atoms in the reactants side, it has the oxidation state of +3 where three hydroxide ions (OH-) are needed to balance out the charges when they are bonded in the products. The remaining ions are Na+ and Cl- where they bond together in a 1:1 ratio where there are 3 molecules of NaCl once the reaction is balanced.
The overall reaction will be:
\[\ce{FeCl3}(aq)+\ce{NaOH}(aq)⟶ \ce{Fe(OH)3}(s) + \ce{3NaCl}(aq)\]
NOTE: Fe(OH)3(s) is a solid as it is a rule that all all transition metal hydroxides are insoluble and a precipitate is formed.
Since NaOH(aq) and NaCl(aq) are aqueous, we can write them out in their ion forms:
\[\ce{FeCl3}(aq)+\ce{3Na+}(aq)+\ce{3OH^{−}}(aq)+\ce{Fe(OH)3}(s)+\ce{3Na+}(aq)+\ce{3Cl+}(aq)\]
- Mn(OH)2(s)+HBr(aq)⟶ ?
This is an example of a metal hydroxide reacting with an acid where a metal salt and water will always be formed:
\[Metal Hydroxide + Acid ⟶ Metal Salt + Water\]
When this rule is applied to this equation, we will get the following:
\[\ce{Mn(OH)2}(s)+\ce{HBr}(aq)⟶ \ce{MnBr2}(aq)+\ce{2H2O}(l)\]
But to follow through with this question, the aqueous solutions such as HBr(aq) and MnBr2(aq) can be re-written as:
\[\ce{Mn(OH)2}(s)+\ce{2H3O+}(aq)+\ce{2Br-}(aq)⟶\ce{Mn2+}(aq)+\ce{2Br-}(aq)+\ce{4H2O}(l)\]
- Cr(s)+O2(g)⟶ ?
This is the general reaction of a metal reacting with oxygen which will always result in a metal oxide. However, the metal oxide is determined by the oxidation state of the metal so there may be several outcomes of this reaction such as:
\[\ce{4Cr}(s)+\ce{3O2}(g)⟶\ce{2Cr2O3}(s)\]
\[\ce{Cr}(s)+\ce{O2}(g)⟶\ce{2CrO}(s)\]
\[\ce{Cr}(s)+\ce{O2}(g)⟶\ce{CrO2}(s)\]
\[\ce{2Cr}(s)+\ce{3O2}(g)⟶\ce{CrO3}(s)\]
However, Cr2O3 is the main oxide of chromium so it can be assumed that this is the general product of this reaction.
- Mn2O3(s)+HCl(aq)⟶?
This follows the general reaction of a metal oxide and an acid will always result in a salt and water
\[Metal Oxide + Acid ⟶ Salt + Water\]
Using this general reaction, similar to the general reactions above, the reaction will result in:
\[\ce{Mn2O3}(s)+\ce{HCl}(aq)⟶\ce{2MnCl3}(s)+\ce{9H2O}(l)\]
However, since HCl is an aqueous solution, the overall equation can also be re-written as:
\[\ce{Mn2O3}(s)+\ce{6H3O+}(aq)+\ce{6Cl-}(aq)⟶\ce{2MnCl3}(s)+\ce{9H2O}(l)\]
- Ti(s)+xsF2(g)⟶?
Titanium is able to react with the halogens where there are two oxidation state that titanium can be: +3 and +4. The following reactions follow each oxidation state accordingly:
\[\ce{2Ti}(s)+\ce{3F2}(g)⟶ \ce{2TiF3}(s)\]
\[\ce{Ti}(s)+\ce{2F2}(g)⟶\ce{TiF4}(s)\]
However, since there is the symbol "xs", this indicates that F2 is added in excess so the second reaction is favored more as it drives the reaction to completion.
OVERALL:
\[\ce{Ti}(s)+\ce{xsF2}(g)⟶\ce{TiF4}(g)\]
A19.1.17
- \(\ce{Fe}(s)+\ce{2H3O+}(aq)+\ce{SO4^2-}(aq)⟶\ce{Fe^2+}(aq)+\ce{SO4^2-}(aq)+\ce{H2}(g)+\ce{2H2O}(l)\);
- \(\ce{FeCl3}(aq)+\ce{3Na+}(aq)+\ce{3OH-}(aq)+\ce{Fe(OH)3}(s)+\ce{3Na+}(aq)+\ce{3Cl+}(aq)\);
- \(\ce{Mn(OH)2}(s)+\ce{2H3O+}(aq)+\ce{2Br-}(aq)⟶\ce{Mn^2+}(aq)+\ce{2Br-}(aq)+\ce{4H2O}(l)\);
- \(\ce{4Cr}(s)+\ce{3O2}(g)⟶\ce{2Cr2O3}(s)\);
- \(\ce{Mn2O3}(s)+\ce{6H3O+}(aq)+\ce{6Cl-}(aq)⟶\ce{2MnCl3}(s)+\ce{9H2O}(l)\);
- \(\ce{Ti}(s)+xs\ce{F2}(g)⟶\ce{TiF4}(g)\)
Q19.1.18
Describe the electrolytic process for refining copper.
S19.1.18
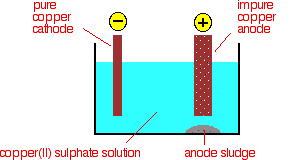
By electrolysis, copper can be refined and purely made. The reason why copper needs to remove the impurities is because it helps increase the electrical conductivity in electrical wire. You can refine copper and remove the impurities through electrolysis. Pure copper is important in making electrical wire, because it creates better electrical conductivity when transferring electricity. In order for better electrical conductivity, the impurities needs to be removed and this can be done by firing the impure copper to remove the impurities, such as sulfur, oxygen, etc. and shaping them into electrical anodes that can be used in electrolysis. Then the copper electrodes are placed into an electrical cell (into separate beakers) where electrical current can pass through the beakers and onto the electrodes. Through this process, the copper is stripped off of the anode and deposited onto the cathode. This process helps remove the impurities and refine copper because all the copper has been deposited onto the cathode all in one electrode. This process increases the weight of the cathode due to copper being deposited onto the cathode. This is a prime example of how to tell if an electrode is a cathode or an anode, as stated in Q17.2.9 above.
Q19.1.19
Predict the products of the following reactions and balance the equations.
- Zn is added to a solution of Cr2(SO4)3 in acid.
- FeCl2 is added to a solution containing an excess of \(\ce{Cr2O7^2-}\) in hydrochloric acid.
- Cr2+ is added to \(\ce{Cr2O7^2-}\) in acid solution.
- Mn is heated with CrO3.
- CrO is added to 2HNO3 in water.
- FeCl3 is added to an aqueous solution of NaOH.
S19.1.19
is added to a solution of
in acid.
- Oxidized half-reaction:
- Reduction half reaction:
- Overall reaction:
- Chromium will precipitate out of the solution because it has a higher reduction potential than Zinc; the reaction is a single replacement.
- Oxidized half-reaction:
is added to a solution containing an excess of
in hydrochloric acid.
- Dissociation reaction:
- Oxidation half-reaction:
- Reduction half-reaction:
- Overall reaction:
- The reduction potential for permanganate is larger so the reaction is still favorable even when the oxidation of
is negative.
- Dissociation reaction:
is added to
in acid solution.
- Reduction half-reaction:
- Oxidation half-reaction:
- Overall reaction:
- The reaction is favorable with a high positive
- Reduction half-reaction:
is heated with
.
- Reduction half-reaction:
- Oxidation half-reaction:
- Overall reaction:
- Heat creates a product with higher energy than both previous reactants.
- Reduction half-reaction:
is added to
in water.
- Strong acid dissociation:
- Overall reaction:
- This reaction works by exchange of electrons to yield Chromium ions.
- Strong acid dissociation:
is added to an aqueous solution of
.
- Overall reaction:
- Iron hydroxide will precipitate because the two metals will exchange anions.
- Overall reaction:
A19.1.19
- \(\ce{Cr2(SO4)3}(aq)+\ce{2Zn}(s)+\ce{2H3O+}(aq)⟶\ce{2Zn^2+}(aq)+\ce{H2}(g)+\ce{2H2O}(l)+\ce{2Cr^2+}(aq)+\ce{3SO4^2-}(aq)\);
- \(\ce{4TiCl3}(s)+\ce{CrO4^2-}(aq)+\ce{8H+}(aq)⟶\ce{4Ti^4+}(aq)+\ce{Cr}(s)+\ce{4H2O}(l)+\ce{12Cl-}(aq)\);
- In acid solution between pH 2 and pH 6, \(\ce{CrO4^2-}\) forms \(\ce{HrCO4-}\), which is in equilibrium with dichromate ion. The reaction is \(\ce{2HCrO4-}(aq)⟶\ce{Cr2O7^2-}(aq)+\ce{H2O}(l)\). At other acidic pHs, the reaction is \(\ce{3Cr^2+}(aq)+\ce{CrO4^2-}(aq)+\ce{8H3O+}(aq)⟶\ce{4Cr^3+}(aq)+\ce{12H2O}(l)\);
- \(\ce{8CrO3}(s)+\ce{9Mn}(s)\overset{Δ}{⟶}\ce{4Cr2O3}(s)+\ce{3Mn3O4}(s)\);
- \(\ce{CrO}(s)+\ce{2H3O+}(aq)+\ce{2NO3-}(aq)⟶\ce{Cr^2+}(aq)+\ce{2NO3-}(aq)+\ce{3H2O}(l)\);
- \(\ce{CrCl3}(s)+\ce{3NaOH}(aq)⟶\ce{Cr(OH)3}(s)+\ce{3Na+}(aq)+\ce{3Cl-}(aq)\)
Q19.1.20
What is the gas produced when iron(II) sulfide is treated with a nonoxidizing acid?
S19.1.20
Formula for iron(II) sulfide: \[FeS\]
Definition of non-oxidizing acid: A non-oxidizing acid is an acid that doesn't act the oxidizing agent. Its anion is a weaker oxidizing agent than H+, thus it can't be reduced. Examples of non-oxidizing acids: \[HCl, HI, HBr, H_3PO_4, H_2SO_4\]
Step 2: Choose one of the non-oxidizing acid, in this case HCl, and write the chemical reaction:
\[FeS(s)+2HCl(aq)\rightarrow FeCl_2(s)+H_2S(g)\]
The gas produced when iron (II) sulfide treated with a non-oxidizing acid, HCl, is H2S (dihydrogen sulfide) gas.
Q19.1.21
Predict the products of each of the following reactions and then balance the chemical equations.
- Fe is heated in an atmosphere of steam.
- NaOH is added to a solution of Fe(NO3)3.
- FeSO4 is added to an acidic solution of KMnO4.
- Fe is added to a dilute solution of H2SO4.
- A solution of Fe(NO3)2 and HNO3 is allowed to stand in air.
- FeCO3 is added to a solution of HClO4.
- Fe is heated in air.
S19.1.21
a. Steam is water (\(\ce{H_{2}O}\))
We can write out the reaction as:
\(\ce{Fe}\) + \(\ce{H_{2}O}\) → ?
This is a single replacement reaction, so \(\ce{Fe}\) replaces \(\ce{H_{2}}\). So, one of the products is \(\ce{Fe_{3}O_{4}}\) since it is a combination of iron(II) oxide, \(\ce{FeO}\), and iron(III) oxide, \(\ce{Fe_{2}O_{3}}\).
The \(\ce{Fe}\) is heated in an atmosphere of steam. \(\ce{H_{2}}\) becomes neutrally charged and becomes another product.
After balancing the coefficients, the final reaction is:
\(\ce{3Fe}(s)\) + \(\ce{4H_{2}O}(g)\) → \(\ce{Fe_{3}O_{4}}(s)\) + \(\ce{4H_{2}}(g)\)
b. \(\ce{NaOH}\) added to a solution of \(\ce{Fe(NO_{3})_{3}}\) is a double replacement and precipitation reaction.
We can write out the reaction as:
\(\ce{NaOH}\) + \(\ce{Fe(NO_{3})_{3}}\) → ?
The \(\ce{Na}\) and \(\ce{Fe}\) switch to form \(\ce{Fe(OH)_{3}}(s)\) and \(\ce{NaNO_{3}}(aq)\).
\(\ce{Fe(OH)_{3}}\) is solid because it is insoluble according to solubility rules.
After balancing the coefficients in the reaction, the final reaction is:
\(\ce{Fe(NO_{3})_{3}}(aq)\) + \(\ce{3NaOH}(aq)\) → \(\ce{Fe(OH)_{3}}(s)\) + \(\ce{NaNO_{3}}(aq)\)
c. For instance, the acid used to make the acidic solution is \(\ce{H_{2}SO_{4}}\), then the reaction is:
\(\ce{FeSO_{4}}\) + \(\ce{KMnO_{4}}\) + \(\ce{H_{2}SO_{4}}\) → \(\ce{Fe_{2}(SO_{4})_{3}}\) + \(\ce{MnSO_{4}}\) + \(\ce{H_{2}O}\) + \(\ce{K_{2}SO_{4}}\)
Next, the net ionic reaction has to be written to get rid of the spectator ions in the reaction, this is written as:
\(\ce{Fe^{2+}}\) + \(\ce{MnO_{4}^{-}}\) + \(\ce{H^{+}}\) → \(\ce{Fe^{3+}}\) + \(\ce{Mn^{2+}}\) + \(\ce{H_{2}O}\)
As seen in the net ionic equation above, \(\ce{Fe^{2+}}\) is oxidized to \(\ce{Fe^{3+}}\) and \(\ce{MnO_{4}^{-}}\) is reduced to \(\ce{Mn^{2+}}\). These can be written as two half reactions:
\(\ce{Fe^{2+}}\) → \(\ce{Fe^{3+}}\)
\(\ce{MnO_{4}^{-}}\) → \(\ce{Mn^{2+}}\)
To balance the oxidation half reaction, one electron as to be added to the \(\ce{Fe^{3+}}\), this is shown as:
\(\ce{Fe^{2+}}\) → \(\ce{Fe^{3+}}\) + \(\ce{e^{-}}\)
The reduction half reaction also has to be balanced, but with \(\ce{H^{+}}\) ions and \(\ce{H_{2}O}\), this is shown as:
\(\ce{MnO_{4}^{-}}\) + \(\ce{8H^{+}}\) → \(\ce{Mn^{2+}}\) + \(\ce{4H_{2}O}\)
After the charge of the \(\ce{Mn}\) atoms are balanced, the overall charge has to be balanced on both sides because on the reactants side, the charge is \(\ce{7+}\), and the charge on the products side is \(\ce{2+}\). The overall charge can be balanced by adding electrons, this is shown as:
\(\ce{MnO_{4}^{-}}\) + \(\ce{8H^{+}}\) + \(\ce{5e^{-}}\) → \(\ce{Mn^{2+}}\) + \(\ce{4H_{2}O}\)
Now since both half reactions are balanced, the electrons in both half reactions have to be equal, and then the half reactions are added together. After this is done, the reaction looks like this:
\(\ce{MnO_{4}^{-}}\) + \(\ce{8H^{+}}\) + \(\ce{5Fe^{2+}}\) + \(\ce{5e^{-}}\) → \(\ce{Mn^{2+}}\) + \(\ce{4H_{2}O}\) + \(\ce{5Fe^{3+}}\) + \(\ce{5e^{-}}\)
The \(\ce{5e^{-}}\) on both sides cancel out and the final balanced reaction is:
\(\ce{MnO_{4}^{-}}\) + \(\ce{8H^{+}}\) + \(\ce{5Fe^{2+}}\) →\(\ce{Mn^{2+}}\) + \(\ce{4H_{2}O}\) + \(\ce{5Fe^{3+}}\)
d. \(\ce{Fe}\) added to a dilute solution of \(\ce{H_{2}SO_{4}}\) is a single replacement reaction.
The \(\ce{Fe}\) is added to a dilute solution so the \(\ce{H_{2}SO_{4}}\) is written as separate ions.
We can write out the reaction as:
\(\ce{Fe}(s)\) + \(\ce{2H^+}(aq)\) + \(\ce{(SO_{4})^{2-}}(aq)\) → ?
The Fe replaces the \(\ce{H^+}\) ion, and becomes an \(\ce{Fe^{2+}}\) ion.
\(\ce{H_{2}O}\) is also a product because the solution is dilute.
Furthermore, the \(\ce{FeSO_{4}}\) also has to be separated into ions as a result of the \(\ce{Fe}\) being added to a dilute solution.
After balancing all of the coefficients, the final reaction is:
\(\ce{Fe}(s)\) + \(\ce{(2H_{3}O)^+}(aq)\) + \(\ce{(SO_{4})^{2-}}(aq)\) → \(\ce{Fe^{2+}}(aq)\) + \(\ce{SO_{4}^{2-}}(aq)\) + \(\ce{H_{2}}(g)\) + \(\ce{2H_{2}O}(l)\)
Note: \(\ce{H^+}\) can also be written as the the hydronium ion, \(\ce{(H_{3}O)^{+}}\).
e. We initially can initially write out:
\(\ce{4Fe(NO_{3})_{2}}\) + \(\ce{4HNO_{3}}\) + \(\ce{O_{2}}\) → ?
We write the oxygen term in the reactants because it is stated that the solution is allowed to stand in air.
We just have to analyze the possible products that can be formed and we can see that the hydrogen from nitric acid can combine with oxygen gas to form water and then combining everything together, we get the final reaction to be:
\(\ce{4Fe(NO_{3})_{2}}(aq)\) + \(\ce{4HNO_{3}}(aq)\) + \(\ce{O_{2}}(g)\) → \(\ce{2H_{2}O}(l)\) + \(\ce{4Fe(NO_{3})_{3}}(aq)\)
f. When \(\ce{FeCO_{3}}\) is added to \(\ce{HClO_{4}}\), a double replacement reaction occurs.
The \(\ce{Fe^{2+}}\) ion switches spots with the \(\ce{H^+}\) ion to form \(\ce{Fe(ClO_{4})_{2}}\) as a product.
When the \(\ce{H^+}\) ion is added to the \(\ce{(CO_{3})^{2-}}\) ion, \(\ce{H_{2}CO_{3}}\) is formed.
After balancing the coefficients, the final reaction is:
\(\ce{FeCO_{3}}(s)\) + \(\ce{HClO_{4}}(aq)\) → \(\ce{Fe(ClO_{4})_{2}}(aq)\) + \(\ce{H_{2}O}(l)\) + \(\ce{CO_{2}}(g)\)
g. Air is composed of oxygen gas, which is a diatomic molecule, so it is \(\ce{O_{2}}\).
Adding \(\ce{Fe}\) to \(\ce{O_{2}}\) will cause a synthesis reaction to occur forming \(\ce{Fe_{2}O_{3}}\).
After balancing coefficients, the final reaction is:
\(\ce{3Fe}(s)\) + \(\ce{2O_{2}}(g)\) → \(\ce{Fe_{2}O_{3}}(s)\)
A19.1.21
- \(\ce{3Fe}(s)+\ce{4H2O}(g)⟶\ce{Fe3O4}(s)+\ce{4H2}(g)\);
- \(\ce{3NaOH}(aq)+\ce{Fe(NO3)3}(aq)\xrightarrow{\ce{H2O}}\ce{Fe(OH)3}(s)+\ce{3Na+}(aq)+\ce{3NO3-}(aq)\);
- \(\ce{MnO_{4}^{-}}\) + \(\ce{8H^{+}}\) + \(\ce{5Fe^{2+}}\) →\(\ce{Mn^{2+}}\) + \(\ce{4H_{2}O}\) + \(\ce{5Fe^{3+}}\)
- \(\ce{Fe}(s)\) + \(\ce{(2H_{3}O)^+}(aq)\) + \(\ce{(SO_{4})^{2-}}(aq)\) → \(\ce{Fe^{2+}}(aq)\) + \(\ce{SO_{4}^{2-}}(aq)\) + \(\ce{H_{2}}(g)\) + \(\ce{2H_{2}O}(l)\)
- \(\ce{4Fe(NO_{3})_{2}}(aq)\) + \(\ce{4HNO_{3}}(aq)\) + \(\ce{O_{2}}(g)\) → \(\ce{2H_{2}O}(l)\) + \(\ce{4Fe(NO_{3})_{3}}(aq)\)
- \(\ce{FeCO_{3}}(s)\) + \(\ce{HClO_{4}}(aq)\) → \(\ce{Fe(ClO_{4})_{2}}(aq)\) + \(\ce{H_{2}O}(l)\) + \(\ce{CO_{2}}(g)\)
- \(\ce{3Fe}(s)\) + \(\ce{2O_{2}}(g)\) → \(\ce{Fe_{2}O_{3}}(s)\)
Q19.1.22
Balance the following equations by oxidation-reduction methods; note that three elements change oxidation state.
\[\ce{Co(NO3)2}(s)⟶\ce{Co2O3}(s)+\ce{NO2}(g)+\ce{O2}(g)\]
S19.1.22
Balance the following equations by oxidation-reduction methods; note that three elements change oxidation state.
\[Co(NO_3){_2(s)}⟶Co_2O{_3(s)}+NO{_2(g)}+O{_2(g)}\]
In this reaction, N changes oxidation states from +5 to +4 (reduced), Co changes oxidation states from +2 to +3 (oxidized), and O changes oxidation states from -2 to 0 (also oxidized).
First, split this reaction into an oxidation and reduction half reaction set, and balance all of the elements that are not hydrogen or oxygen (we will deal with these later):
\[Reduction: 2Co(NO_3){_2}\rightarrow Co_2O_{3}+4NO_2\]
Now, for the oxidation reaction, we are only dealing with O2 on the products side. In order to balance this, we will need to add water and hydrogen to both sides:
\[Oxidation: 2H_2O\rightarrow O_2+4H^+ \]
Balance the amount of oxygens on each side by adding the correct number of water molecules (H2O), and balance the amount of hydrogen by adding the correct number of H+ atoms:
\[Reduction: 2H^++2Co(NO_3){_2}\rightarrow Co_2O_{3}+4NO_2+H_2O\]
\[Oxidation: 2H_2O\rightarrow O_2+4H^+\]
Finally, balance the charges by adding electrons to each side of the equation. For the reduction reaction, we will add 2 electrons to balance out the 2H+, and to the oxidation reaction, we will add 4 electrons to balance out the 4H+. Remember, the goal of this step is to make sure that the charges are balanced, so we can cancel them out in the end.
\[Reduction: 2e^- + 2H^++2Co(NO_3){_2}\rightarrow Co_2O_{3}+4NO_2+H_2O\]
\[Oxidation: 2H_2O\rightarrow O_2+4H^+ +4e^-\]
Multiply the reduction reaction by two, in order to balance the charges so there are 4 electrons on each side of the reaction.
\[Reduction: 2(2e^- + 2H^++2Co(NO_3){_2}\rightarrow Co_2O_{3}+4NO_2+H_2O)\]
and combine both reactions which comes out to:
\[2H_2O + 4Co(NO_3)_2 + 4H^+ \rightarrow 2CO_2O_3 + 8NO_2 + 2H_2O + O_2 + 4H^+\]
Cancel out like terms:
\[4Co(NO_3){_2(s)} \rightarrow 2CO_2O{_3(s)} + 8NO{_2(g)} + O{_2(g)}\]
Both sides have overall charges of 0 and can be checked to see if they are balanced.
A19.1.22
\[4Co(NO_3){_2(s)} \rightarrow 2CO_2O{_3(s)} + 8NO{_2(g)} + O{_2(g)}\]
Q19.1.23
Dilute sodium cyanide solution is slowly dripped into a slowly stirred silver nitrate solution. A white precipitate forms temporarily but dissolves as the addition of sodium cyanide continues. Use chemical equations to explain this observation. Silver cyanide is similar to silver chloride in its solubility.
S19.1.23
Dilute sodium cyanide solution is slowly dripped into a slowly stirred silver nitrate solution. A white precipitate forms temporarily but dissolves as the addition of sodium cyanide continues. Use chemical equations to explain this observation. Silver cyanide is similar to silver chloride in its solubility.
A: Step 1: look at the question and begin to write out a general product to reactant formula for this reaction.
Step 2: try to reason out why a precipitate will form but only for a finite period of time before reforming in an aqueous substance.
Step 3: With step 2 you should have noticed that the reaction is a multiple step reaction and using the rough formula that you derived in step 1, you should try and see what the series of steps are that lead to the overall product of liquid AgCN2
In this reaction we see how NaCN is added to AgNO3 .A precipitate forms but then disappears with the addition of even more NaCN, this must mean that its an intermediate reaction which will not appear as the final product. The silver and the cyanide temporarily bond, but the bond is too weak to hold them together so they are pulled apart again when NaCN is added because a new, more stronger and stable compound is formed: [Ag(CN)2]- (aq).
The actual reaction equation when it is first taking place is \[AgCl(aq)+NaCN(aq)\rightarrow AgCN(s)+NaCl(aq)\]
This can be written out in the following way: as CN− is added, the silver and the cyanide combine : Ag+(aq)+CN−(aq)→AgCN (s)
As more CN- is added the silver and two cyanide combine to create a more stable compound: Ag+(aq)+2CN−(aq)→[Ag(CN)2]- (aq)
AgCN(s) + CN- (aq) → [Ag(CN)2]- (aq)
A19.1.23
As CN− is added,
\[\ce{Ag+}(aq)+\ce{CN-}(aq)⟶\ce{AgCN}(s)\]
As more CN− is added,
\[\ce{Ag+}(aq)+\ce{2CN-}(aq)⟶\ce{[Ag(CN)2]-}(aq)\]
\[\ce{AgCN}(s)+\ce{CN-}(aq)⟶\ce{[Ag(CN)2]-}(aq)\]
Q19.1.24
Predict which will be more stable, [CrO4]2− or [WO4]2−, and explain.
S19.1.24
According to the rules associated with Crystal Field Stabilizing Energies, stable molecules contain more electrons in the lower-energy molecular orbitals than in the high-energy molecular orbitals. In this case, both complexes have O4 as ligands, and both have a -2 charge. Therefore, you determine stability by comparing the metals. Chromium is in the 3d orbital, according to the periodic table. Tungsten (W) is in the 5d orbital. 3d is a lower energy level than 5d.Higher-level orbitals are more easily ionized, and make their base elemental form more stable. If the elemental form is more stable the oxidized form is less stable. Therefore, [CrO4]2− is more stable than [WO4]2−.
A19.1.24
[CrO4]2- is more stable because Chromium is in the 3d orbital while Tungsten is in the 4d orbital, which has a higher energy level and makes it less stable.
Q19.1.25
Give the oxidation state of the metal for each of the following oxides of the first transition series. (Hint: Oxides of formula M3O4 are examples of mixed valence compounds in which the metal ion is present in more than one oxidation state. It is possible to write these compound formulas in the equivalent format MO·M2O3, to permit estimation of the metal’s two oxidation states.)
- Sc2O3
- TiO2
- V2O5
- CrO3
- MnO2
- Fe3O4
- Co3O4
- NiO
- Cu2O
S19.1.25
The first step to solving this problem is looking at the rules of Oxidizing states for various elements:
chem.libretexts.org/Core/Analytical_Chemistry/Electrochemistry/Redox_Chemistry/Oxidation_State
The main rules that will be used in these problems will be the oxidation state rule 6 which states that oxidation state for Oxygen is (-2) and rule 2 which is that the total sum of the oxidation state of all atoms in any given species is equal to the net charge on that species. Solving these problems requires simple algebra. The oxidation states of both elements in the compound is equal to zero, so set the unknown oxidation of the element that is not oxygen to a variable \({x}\), and the oxidation state of Oxygen equal to \({-2}\). Then multiply both oxygen states by the number of atoms of the element present. Add the values together, set the equation equal to zero and solve for \({x}\).
- \(\ce{Sc2O3}={3{(-2)}}+{2{x}}={0}⟶{-6}+{2{x}}={0}⟶{x}={Sc}={+3}\) \(Sc^{3+}\)
- \(\ce{TiO2}={2{(-2)}}+{x}={0}⟶{-4}+{x}={0}⟶{x}={Ti}={+4}\) \(Ti^{4+}\)
- \(\ce{V2O5}={5{(-2)}}+{2{x}}={0}⟶{-10}+{2{x}}={0}⟶{x}={V}={+5}\) \(V^{5+}\)
- \(\ce{CrO3}={3{(-2)}}+{x}={0}⟶{-6}+{x}={0}⟶{x}={Cr}={+6}\) \(Cr^{6+}\)
- \(\ce{MnO2}={2{(-2)}}+{x}={0}⟶{-4}+{x}={0}⟶{x}={Mn}={+4}\) \(Mn^{4+}\)
- \(\ce{Fe3O4}=\ce{FeO}·\ce{Fe2O3}=\)
\(\ce{FeO}={-2}+{x}={0}⟶{x}={Fe}={+2}\) \(Fe^{2+}\)
\(\ce{Fe2O3}={3{(-2)}}+{2{x}}={0}⟶{-6}+{2x}={0}⟶{x}={Fe}={+3}\) \(Fe^{3=}\)
(One Fe Atom has an oxidation state of +2 and the other 2 Fe atoms have an oxidation state of +3)
7. \(\ce{Co3O4}=\ce{CoO}·\ce{Co2O3}=\)
\(\ce{CoO}={-2}+{x}={0}⟶{x}={Co}={+2}\) \(Co^{2+}\)
\(\ce{Co2O3}={3{(-2)}}+{2{x}}={0}⟶{-6}+{2x}={0}⟶{x}={Co}={+3}\) \(Co^{3+}\)
(One Co Atom has an oxidation state of +2 and the other 2 Co atoms have an oxidation state of +3)
8. \(\ce{NiO}={-2}+{x}={0}⟶{x}={Ni}={+2}\) \(Ni^{2+}\)
9. \(\ce{Cu2O}={-2}+{2{x}}={0}⟶{-2}+{2x}={0}⟶{x}={Cu}={+1}\) \(Cu^{1+}\)
A19.1.25
Sc3+; Ti4+; V5+; Cr6+; Mn4+; Fe2+ and Fe3+; Co2+ and Co3+; Ni2+; Cu+
19.2: Coordination Chemistry of Transition Metals
Q19.2.1
Indicate the coordination number for the central metal atom in each of the following coordination compounds:
- [Pt(H2O)2Br2]
- [Pt(NH3)(py)(Cl)(Br)] (py = pyridine, C5H5N)
- [Zn(NH3)2Cl2]
- [Zn(NH3)(py)(Cl)(Br)]
- [Ni(H2O)4Cl2]
- [Fe(en)2(CN)2]+ (en = ethylenediamine, C2H8N2)
S19.2.1
First we must identify whether or not the ligand has more than one bonded atom (bidentate/polydentate). Using the table below we are able to do this.
| Ligand | Number of bonded atoms |
|---|---|
| Ammine (NH3) | monodentate |
| Aqua (H2O) | monodentate |
| Bromo (Br) | monodentate |
| Chloro (Cl) | monodentate |
| Cyano (CN) | monodentate |
| Pyridine (C5H5N) | monodentate |
| Ethylenediamine (C2H8N2) | bidentate |
Now that we have identified the number of bonded atoms from each ligand, we can find the total number of atoms bonded to the central metal ion, giving us the coordination number.
- \([Pt(H_2O)_2Br_2]\): We can identify the metal ion in the complex as Pt, platinum, as the other two are listed as ligands above and are nonmetallic. We can now use the number of ligands and their bonding atoms to find its coordination number. From the table above we see that H2O has only one bonding atom and Br as well. So for each Br atom we have one bonding atom, and we have two of these, yielding 2 bonding atoms; this is the same for H2O, giving us a total number of 4 bonding atoms, and therefore a coordination number of 4.
- \([Pt(NH_3)(py)(Cl)(Br)]\) (py = pyridine, C5H5N): The metal ion in this complex, similarly to the first one, can be identified as Pt, platinum. The ligands can be identified as NH3, pyridine, Cl, and Br, which are all monodentate ligands and have one bonding atom each. Since we have four ligands, each with one bonding atom, the total number of bonding atoms on the metal ion is 4, therefore the complex has a coordination number of 4.
- \([Zn(NH_3)_2Cl_2]\): The metal ion in this complex can be identified as Zn, zinc, and the ligands can be identified as NH3 and Cl. Since these two are both monodentate ligands they have one bonding atom each. Since we have a total of two NH3 and two Cl ligands, we get a total of four monodentate ligands, giving us 4 bonding atoms and a coordination number of 4.
- \([Zn(NH_3)(py)(Cl)(Br)]\): The metal ion in this complex can be identified as Zn, zinc, and the ligands can be identified as NH3, pyridine, Cl, and Br, which are all monodentate ligands and have one bonding atom each. Since we have four ligands, each with one bonding atom, the total number of bonding atoms on the metal ion is 4, therefore the complex has a coordination number of 4.
- \([Ni(H_2O)_4Cl_2]\): The metal ion in this complex can be identified as Ni, nickel, and we can now use the number of ligands and their bonding atoms to find its coordination number. From the table above we see that H2O has only one bonding atom and Cl as well. So for each Cl atom we have one bonding atom, and we have two of these, yielding 2 bonding atoms. H2O is the same, having only one bonding atom, but there are four of these. So this gives us a total number of 6 bonding atoms, and therefore a coordination number of 6.
- \([Fe(en)_2(CN)_2]^+\) (en = ethylenediamine, C2H8N2): The metal ion in this complex can be identified as Fe, iron, and the ligands can be identified as (en) and CN. Since (en) is bidentate, meaning it has 2 bonding atoms, and there are two of these, the total number of bonding atoms from (en) is four. Since CN is monodentate, meaning it has one bonding atom, and there are two of these, the total number of bonding atoms from CN ligand is two. So, the total number of bonding atoms is 6, therefore the complex has a coordination number of 6.
A19.2.1
- The 2 aqua and the 2 bromo ligands form a total of 4 coordinate covalent bonds and as a result the coordination number is 4.
- The ammine, pyridine, chloro and bromo each form one coordinate covalent bond that gives a total of 4 and hence CN=4.
- Two ammine and two chloro ligands give a total of 4 coordinate covalent bonds and a CN = 4.
- One ammine, a pyrimidine, a chloro and a bromo ligand give a total of 4 covalent bonds, resulting in CN = 4.
- Four aqua ligands and two chloro ligands form a total of 6 coordinate covalent bonds and a CN =6.
- Ethylenediamine is a bidentate ligand that forms two coordinate covalent bonds; along with two cyano ligands, it forms a total of 6 bonds, and hence has a CN=6.
Q19.2.2
Give the coordination numbers and write the formulas for each of the following, including all isomers where appropriate:
- tetrahydroxozincate(II) ion (tetrahedral)
- hexacyanopalladate(IV) ion
- dichloroaurate ion (note that aurum is Latin for "gold")
- diamminedichloroplatinum(II)
- potassium diamminetetrachlorochromate(III)
- hexaamminecobalt(III) hexacyanochromate(III)
- dibromobis(ethylenediamine) cobalt(III) nitrate
S19.2.2
To determine coordination numbers we must count the total number of ligands bonded to the central metal and distinguish monodentate and polydentate ligands. To determine the formulas, we use the nomenclature rules and work backwards.
- "tetrahydroxo" = 4 hydroxide ligands; since hydroxide is a monodentate ligand, we have a total of 4 bonds to the central metal.
Coordination Number: 4
We review the basics of nomenclature and see that "tetra" = 4 and "hydroxo" = OH-. Since the charge on zinc is 2+, which is given in the nomenclature by the Roman numerals, we can calculate the total charge on the complex to be 2-.
Formula: [Zn(OH)4]2− - "hexacyano" = 6 cyanide ligands; since cyanide is a monodentate ligand, we have a total of 6 bonds to the central metal.
Coordination Number: 6
We review the basics of nomenclature and see that "hexa" = 6 and "cyano" = CN-. Since the charge on Pd is 4+, which is given in the nomenclature by the Roman numerals, we can calculate the total charge on the complex to be 2-.
Formula: [Pd(CN)6]2− - "dichloro" = 2 chloride ligands; since chloride is a monodentate ligand, we have a total of 2 bonds to the central metal.
Coordination Number: 2
We review the basics of nomenclature and see that "di" = 2 and "chloro" = Cl-. Since the charge on Au is always 1+, we can calculate the total charge on the complex to be 1-.
Formula: [AuCl2]− - "diammine" = 2 ammonia ligands and "dichloro" = 2 chloride ligands; since both ammonia and chloride ligands are monodentate, we have a total of 4 bonds to the central metal.
Coordination Number: 4
We review the basics of nomenclature and see that "di" = 2, "chloro" = Cl- and "ammine" = NH3. Since the charge on Pt is 2+, which is given in the nomenclature by the Roman numerals, we can calculate that the total charge is 0, so the complex is neutral.
Formula: [Pt(NH3)2Cl2] - "diammine" = 2 ammonia ligands and "tetrachloro" = 4 chloride ligands; since both ammonia and chloride ligands are monodentate, we have a total of 6 bonds to the central metal.
Coordination Number: 6
We review the basics of nomenclature and see that "di" = 2, "ammine" = NH3, "tetra" = 4 and "chloro" = Cl-. Since the charge on the central metal, Cr, is 3+, which is given in the nomenclature by the Roman numerals, we can calculate that the total charge of the complex is 1-. The "potassium" at the front of the nomenclature indicates that it is the corresponding cation to this anionic complex.
Formula: K[Cr(NH3)2Cl4] - Both of the metal complexes have "hexa" monodentate ligands, which means both have coordination numbers of 6.
Coordination Number: 6
We review the basics of nomenclature and see that "hexa" = 6, "ammine" = NH3, and "cyano" = CN-. Since the charge on Cr is 3+ and Co is 3+, which is given in the nomenclature by the Roman numerals, we find that these complexes' charges balance out.
Formula: [Co(NH3)6][Cr(CN)6] - "dibromo" = 2 bromide ligands "bis(ethylenediamine)" = 2 (en) ligands; bromide is a monodentate ligand while (en) is a bidentate ligand. Therefore, we have a coordination number of 6.
Coordination Number: 6
We review the basics of nomenclature and see that "di" = 2, "bromo" = Br-, "bis" = 2, and "ethylenediamine" = en. Since the charge on Co is 3+, which is given in the nomenclature by the Roman numerals, we find that the total charge of the complex is 1+. Nitrate is the corresponding anion to this cationic complex.
Formula: [Co(en)2Br2]NO3
A19.2.2
- 4, [Zn(OH)4]2−;
- 6, [Pd(CN)6]2−;
- 2, [AuCl2]−;
- 4, [Pt(NH3)2Cl2];
- 6, K[Cr(NH3)2Cl4];
- 6, [Co(NH3)6][Cr(CN)6];
- 6, [Co(en)2Br2]NO3
Q19.2.3
Give the coordination number for each metal ion in the following compounds:
- [Co(CO3)3]3− (note that CO32− is bidentate in this complex)
- [Cu(NH3)4]2+
- [Co(NH3)4Br2]2(SO4)3
- [Pt(NH3)4][PtCl4]
- [Cr(en)3](NO3)3
- [Pd(NH3)2Br2] (square planar)
- K3[Cu(Cl)5]
- [Zn(NH3)2Cl2]
S19.2.3
You can determine a compound's coordination number based on how many ligands are bound to the central atom.
1) In this compound, Cobalt is the central atom, and it has 3 CO32- molecules attached to it. However, CO32- is a bidentate ligand, which means it binds to the central atom in two places rather than one. This means that the coordination number of [Co(CO3)3]3- is 6. A coordination number of 6 means that the structure is most likely octahedral.
2) In this compound, Copper is the central atom. 4 ammonia molecules are attached to it. This means the coordination number is 4, and the structure is likely tetrahedral.
3) For this compound, we can ignore the (SO4)3 because it is not bound to the central atom. The central atom is cobalt, and it has 4 ammonia molecules and 2 bromine molecules bound to it. The coordination number is 6.
4) There are two compounds here, indicated by the brackets. The central atom for both is platinum. One of them has 4 ammonia molecules attached, and the other has 4 chlorine atoms attached. Both complexes have a coordination number of 4.
5) We can ignore (NO3)3 for this compound. The central atom is Chromium. There are 3 ethylenediamine molecules attached to the chromium. Ethylenediamine is a bidentate ligand, so the coordination number is 6.
6) Palladium is the central atom. 2 ammonia molecules and 2 bromine atoms are bound to the palladium atom. The coordination number is 4.
7) We can ignore the K3 structure. Copper is the central atom, and there are 5 chlorine molecules attached to it. The coordination number is 5, so the structure is either trigonal bipyramidal or square pyramidal.
8) In this compound, zinc is the central atom. There are 2 ammonia molecules and 2 chlorine atoms attached. This means that the coordination number is 4.
Q19.2.4
Sketch the structures of the following complexes. Indicate any cis, trans, and optical isomers.
- [Pt(H2O)2Br2] (square planar)
- [Pt(NH3)(py)(Cl)(Br)] (square planar, py = pyridine, C5H5N)
- [Zn(NH3)3Cl]+ (tetrahedral)
- [Pt(NH3)3Cl]+ (square planar)
- [Ni(H2O)4Cl2]
- [Co(C2O4)2Cl2]3− (note that \(\ce{C2O4^2-}\) is the bidentate oxalate ion, \(\ce{−O2CCO2-}\))
S19.2.4
Cis and trans are a type of geometric isomer, meaning there is a difference in the orientation in which the ligands are attached to the central metal. In cis, two of the same ligands are adjacent to one another and in trans, two of the same ligands are directly across from one another.
Optical isomers → have the ability to rotate light, optical isomers are also chiral. Only chiral complexes have optical isomers
Chiral → asymmetric, structure of its mirror image is not superimposable
Enantiomers: chiral optical isomers (compound can have multiple enantiomers)
Tetrahedral complex with 4 distinct ligands → always chiral
- For tetrahedral, if 2 ligands are the same, then it cannot be chiral, has a plane of symmetry
Solutions:
a. \([Pt(H_2O)_2Br_2]\) (square planar)
This complex has 2 kinds of ligands. The matching ligands can either be adjacent to each other and be cis, or they can be across from each other and be trans.
b. \([Pt(NH_3)(py)(Cl)(Br)]\) (square planar, py = pyridine, \(C_5H_5N\))
This complex has 4 different ligands. There is no plane of symmetry in any of the enantiomers, making the structures chiral and therefore has optical isomers.
c. \([Zn(NH_3)_3Cl]^+\) (tetrahedral)
There is a plane of symmetry from \(NH_3\) through \(Zn\) to the other \(NH_3\), therefore it is not chiral.
d. \([Pt(NH_3)_3Cl]^+\) (square planar)
There is a plane of symmetry from \(NH_3\) through \(Pt\) to the other \(NH_3\), therefore it is not chiral.
e. \([Ni(H_2O)_2Cl_2]\)
The \(Cl\) ligands can either be right next to each other, or directly across from one another allowing for both cis and trans geometries.
f. \([Co(C_2O_4)_2Cl_2]^-3\) (note that \(C_2O_4^-2\) is the bidentate oxalate ion, \(^−O_2CCO_2^-\)
There is a plane of symmetry from \(Cl\) through Co to the other \(Cl\) in a "trans" chlorine configuration, therefore it is not chiral in a chlorine "trans" configuration. However, there is no symmetry in the chlorine "cis" configuration, indicating multiple "cis" isomers.
A19.2.4
a. [Pt(H2O)2Br2]:
b. [Pt(NH3)(py)(Cl)(Br)]:
c. [Zn(NH3)3Cl]+ :
d. [Pt(NH3)3Cl]+ :
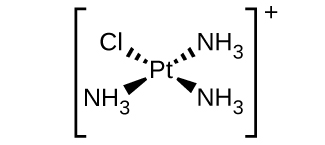
e. [Ni(H2O)4Cl2]:
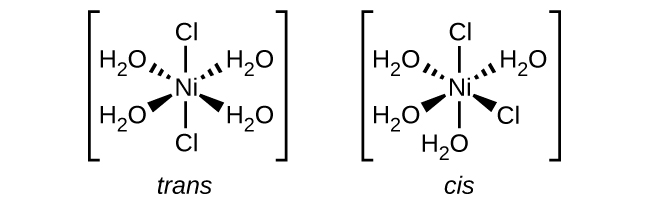
f. [Co(C2O4)2Cl2]3−:
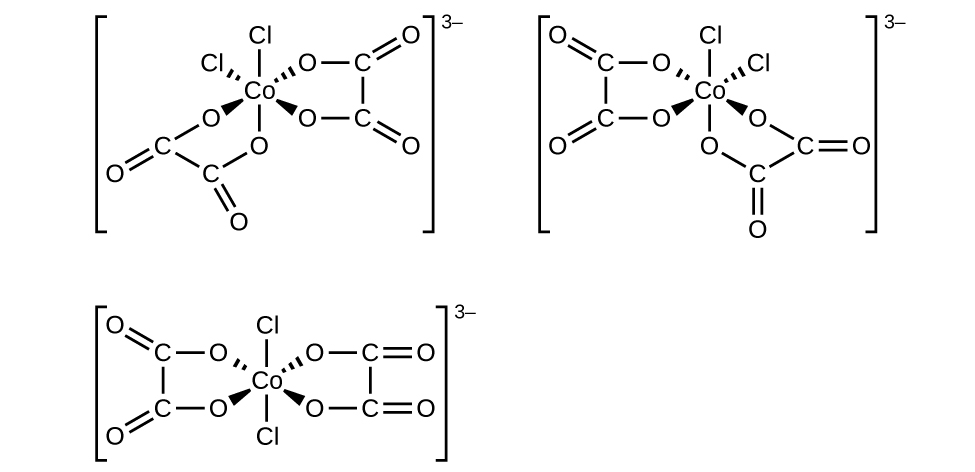
Q19.2.5
Draw diagrams for any cis, trans, and optical isomers that could exist for the following (en is ethylenediamine):
- [Co(en)2(NO2)Cl]+
- [Co(en)2Cl2]+
- [Pt(NH3)2Cl4]
- [Cr(en)3]3+
- [Pt(NH3)2Cl2]
S19.2.5
We are instructed to draw all geometric isomers and optical isomers for the specified compound. Optical isomers exist when an isomer configuration is not superimposable on its mirror image. This means there are two distinct molecular shapes. Often a left and right hand are cited as an example; if you were to take your right hand and place it upon your left, you cannot make the major parts of your hand align on top of one another. The basic idea when deciding whether something is optically active is to look for a plane of symmetry--if you are able to bisect a compound in a manner that establishes symmetry, then the compound does not have an optical isomer.
Cis isomers exist when there are 2 ligands of the same species placed at 90 degree angles from each other. Trans isomers exist when there are 2 ligands of the same species placed at 180 degree angles from each other.
Problem 1
This compound is an octahedral molecule, so the six ligands (atoms in the complex that are not the central transition metal) are placed around the central atom at 90 degree angles. Two optical isomers exist for [Co(en)2(NO2)Cl]+. The second isomer is drawn by taking the mirror image of the first.
Problem 2
This compound is also an octahedral molecule. Two cis (optical) isomers and one trans isomer exist for [Co(en)2Cl2]+. The trans isomer can be drawn by placing the chlorine ligands in positions where they form a 180 degree angle with the central atom. The first cis isomer can be drawn by placing the chlorine ligands in positions where they form a 90 degree angle with the central atom. The second cis isomer can be found by mirroring the first cis isomer, like we did in problem 1.
Problem 3
This compound is also an octahedral molecule. One trans isomer and one cis isomer of [Pt(NH3)2Cl4] exist. The trans isomer can be drawn by placing the ammonia ligands in positions where they form a 180 degree angle with the central atom. The cis isomer can be drawn by placing the ammonia ligands in positions where they form a 90 degree angle with the central atom.
Problem 4
This compound is also an octahedral molecule. Two optical isomers for [Cr(en)3]3+ exist. The second optical isomer can be drawn by taking the mirror image of the first optical isomer.
Problem 5
This compound is a square planar complex, so the ligands are placed around the central atom in a plane, at 90 angles. A trans isomer and a cis isomer exist for the complex [Pt(NH3)2Cl2]. The trans isomer can be drawn by placing the ammonia ligands in positions where they form a 180 degree angle in the plane with the central atom. The cis isomer can be drawn by placing the ammonia ligands in positions where they form a 90 degree angle in the plane with the central atom.
Q19.2.6
Name each of the compounds or ions given in Exercise Q19.2.3, including the oxidation state of the metal.
S19.2.6
Rules to follow for coordination complexes
1. Cations are always named before the anions.
2. Ligands are named before the metal atom or ion.
3. Ligand names are modified with an ‐o added to the root name of an anion. For neutral ligands the name of the molecule is used, with the exception of OH2, NH3, CO and NO.
4. The prefixes mono‐, di‐, tri‐, tetra‐, penta‐, and hexa‐ are used to denote the number of simple ligands.
5. The prefixes bis‐, tris‐, tetrakis‐, etc., are used for more complicated ligands or ones that already contain di‐, tri‐, etc.
6. The oxidation state of the central metal ion is designated by a Roman numeral in parentheses.
7. When more than one type of ligand is present, they are named alphabetically. Prefixes do not affect the order.
8. If the complex ion has a negative charge, the suffix –ate is added to the name of the metal.
9. In the case of complex‐ion isomerism the names cis, trans, fac, or mer may precede the formula of the complex‐ion name to indicate the spatial arrangement of the ligands. Cis means the ligands occupy adjacent coordination positions, and trans means opposite positions just as they do for organic compounds. The complexity of octahedral complexes allows for two additional geometric isomers that are peculiar to coordination complexes. Fac means facial, or that the three like ligands occupy the vertices of one face of the octahedron. Mer means meridional, or that the three like ligands occupy the vertices of a triangle one side of which includes the central metal atom or ion.
A19.2.6
- tricarbonatocobaltate(III) ion;
- tetraaminecopper(II) ion;
- tetraaminedibromocobalt(III) sulfate;
- tetraamineplatinum(II) tetrachloroplatinate(II);
- tris-(ethylenediamine)chromium(III) nitrate;
- diaminedibromopalladium(II);
- potassium pentachlorocuprate(II);
- diaminedichlorozinc(II)
Q19.2.7
Name each of the compounds or ions given in Exercise Q19.2.5.
S19.2.7
Given:
- [Co(en)2(NO2)Cl]+
- [Co(en)2Cl2]+
- [Pt(NH3)2Cl4]
- [Cr(en)3]3+
- [Pt(NH3)2Cl2]
Wanted:
Names of the above compounds.
1. [Co(en)2(NO2)Cl]+
Step 1: Attain the names of the ligands and metal cation. Names can be found here.
Co: Cobalt
en: Ethylenediamine
NO2: Nitro
Cl: Chloro
Step 2: Add the appropriate pre-fixes to each ligand depending on the number. Pre-fixes can be found here.
(en)2: bis(Ethylenediamine)
Step 3: Find the charges of the ligands. Charges can be found here.
en: 0
NO2: -1
Cl: -1
Step 4: Algebraically attain the charge of the metal cation using the overall charge of the complex ion and the individual ligand charges.
Co + 2(en) + (NO2) + Cl = 1
Co +2(0) + (-1) + (-1) = 1
Co = 3
Step 5: For the name alphabetically place the ligands, pre-fixes should not be accounted, and use roman numerals for the metal cation which should be placed last.
Chlorobis(ethylenediamine)nitrocobalt(III)
2. [Co(en)2Cl2]+
Step 1: Attain the names of the ligands and metal cation. Names can be found here.
Co: Cobalt
en: Ethylenediamine
Cl: Chloro
Step 2: Add the appropriate pre-fixes to each ligand depending on the number. Pre-fixes can be found here.
(en)2: bis(Ethylenediamine)
Cl2: dichloro
Step 3: Find the charges of the ligands. Charges can be found here.
en: 0
Cl: -1
Step 4: Algebraically attain the charge of the metal cation using the overall charge of the complex ion and the individual ligand charges.
Co + 2(en) +2(Cl) = 1
Co + 2(0) + 2(-1) = 1
Co = 3
Step 5: For the name alphabetically place the ligands, pre-fixes should not be accounted, and use roman numerals for the metal cation which should be placed last.
Dichlorobis(Ethylenediamine)cobalt(III)
3. [Pt(NH3)2Cl4]
Step 1: Attain the names of the ligands and metal cation. Names can be found here.
Pt: Platinum
NH3: Ammine
Cl: Chloro
Step 2: Add the appropriate pre-fixes to each ligand depending on the number. Pre-fixes can be found here.
(NH3)2: diammine
Cl4: tetrachloro
Step 3: Find the charges of the ligands. Charges can be found here.
NH3: 0
Cl: -1
Step 4: Algebraically attain the charge of the metal cation using the overall charge of the complex ion and the individual ligand charges.
Pt + 2(NH3) + 4(Cl) = 0
Pt + 2(0) + 4(-1) = 0
Pt = 4
Step 5: For the name alphabetically place the ligands, pre-fixes should not be accounted, and use roman numerals for the metal cation which should be placed last.
Diamminetetrachloroplatinum(IV)
4. [Cr(en)3]3+
Step 1: Attain the names of the ligands and metal cation. Names can be found here.
Cr: Cromium
en: ethylenediamine
Step 2: Add the appropriate pre-fixes to each ligand depending on the number. Pre-fixes can be found here.
(en)3: tris(ethylenediamine)
Step 3: Find the charges of the ligands. Charges can be found here.
en: 0
Step 4: Algebraically attain the charge of the metal cation using the overall charge of the complex ion and the individual ligand charges.
Cr + 3(en) = 3
Cr + 3(0) = 3
Cr = 3
Step 5: For the name alphabetically place the ligands, pre-fixes should not be accounted, and use roman numerals for the metal cation which should be placed last.
Tris(ethylenediamine)cromium(III)
5. [Pt(NH3)2Cl2]
Step 1: Attain the names of the ligands and metal cation. Names can be found here.
NH3: Ammine
Cl: Chloro
Pt: Platinum
Step 2: Add the appropriate pre-fixes to each ligand depending on the number.
(NH3)2: diammine
Cl2: dichloro
Step 3: Find the charges of the ligands. Charges can be found here.
NH3: 0
Cl: -1
Step 4: Algebraically attain the charge of the metal cation using the overall charge of the complex ion and the individual ligand charges.
Pt + 2(NH3) + 2(Cl) = 0
Pt + 2(0) + 2(-1) = 0
Pt = 2
Step 5: For the name alphabetically place the ligands, pre-fixes should not be accounted, and use roman numerals for the metal cation which should be placed last.
Diamminedichloroplatinum(II)
A19.2.7
- Chlorobis(ethylenediamine)nitrocobalt(III)
- Dichlorobis(Ethylenediamine)cobalt(III)
- Diamminetetrachloroplatinum(IV)
- Tris(ethylenediamine)cromium(III)
- Diamminedichloroplatinum(II)
Q19.2.8
Specify whether the following complexes have isomers.
- tetrahedral [Ni(CO)2(Cl)2]
- trigonal bipyramidal [Mn(CO)4NO]
- [Pt(en)2Cl2]Cl2
S19.2.8
Isomers are compounds that have the same number of atoms, but have different structures. Structural isomers (linkage, ionization, coordination) and stereoisomers (geometric and optical) can occur with several compounds.
1. tetrahedral \(\mathrm{[Ni(CO)_2(Cl)_2]}\)
(Fig 1.) In this model, nickel is the dark green central atom, carbonyl ligands are the pink atom, and chloro ligands are the light green atoms.
Immediately, we can cancel out the possibility of linkage, ionization, and coordination isomers. There are no other coordination complexes for coordination isomerism, there is no ligand that can bond to the atom in more than one way for it to exhibit linkage isomerism, and there are no ions outside the coordination sphere for ionization isomerism.
This is a tetrahedral structure which immediately rules out any geometric isomers since they require 90° and/or 180° bond angles. Tetrahedral structures have 109.5° angles.
To confirm that the structure has no optical isomer, we must determine if there is a plane of symmetry. Structures that have no plane of symmetry are considered chiral and would have optical isomers.
(Fig 2.) We can rotate the structure and find that there is indeed a plane of symmetry through the two chloro ligands and central atom and between the carbonyl ligands.
Since there is a plane of symmetry, we can conclude that there are no optical isomers.
Overall, there are no isomers that exist for this compound.
2. trigonal byprimidal \(\mathrm{[Mn(CO)_4(NO)]}\)
(Fig 3.) The central purple atom is manganese, the carbonyl ligands are the pink atoms, and the nitrosyl ligand is the fuschia atom.
There are no ions, other coordination complex, and ambidentate ligands. Therefore, no structural isomers exist for this structure.
Geometric isomers do not exist for this compound because there is only one nitrosyl ligand.
(Fig 4.) Dashed line bisects molecule and shows plane of symmetry. The molecule is rotated in this image.
In the image above, after the structure has been rotated, we can see that there is a plane of symmetry. Thus, there are no optical isomers.
No isomers (the ones mentioned above) exist for this compound.
3. \(\mathrm{[Pt(en)_{2}Cl_2]Cl_2}\)
(Fig 5.) The green atoms are the chloro ligands, the the central atom is platinum, and the grey/blue atoms are ethyldiamine ligands.
Coordination isomerism cannot exist for this complex because there are no other complexes. There are no linkage isomers because there are no ambidentate ligands. Ionization isomers cannot exist in this complex either, even though there is a neutral molecule outside the coordination sphere. If we exchange \(\mathrm{Cl_2}\) with one ethyldiamine molecule, There would be 5 ligands in the coordination sphere instead of 4. This difference in the ratio of metal atom to ligands means that an ionization isomer cannot exist.
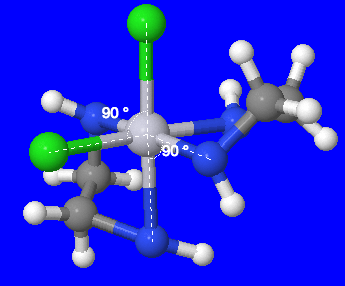
(Fig 6.) Here, one chloro ligand exchanged places with the ethyldiamine so that it can be at a 90° angle with the other chloro ligand.
The image above, shows the chloro and ethyldiamine ligands at a 90° angle with its other identical ligand. This is the cis isomer, while Fig. 5 shows the trans isomer.
Fig 5. shows that there is a plane of symmetry in the trans isomer. Therefore, that structure does not have an optical isomer. On the other hand, the cis isomer does not have a plane of symmetry and therefore has an optical isomer.
A19.2.8
none; none; The two Cl ligands can be cis or trans. When they are cis, there will also be an optical isomer.
Q19.2.9
Predict whether the carbonate ligand \(\ce{CO3^2-}\) will coordinate to a metal center as a monodentate, bidentate, or tridentate ligand.
S19.2.9
\(\ce{CO3^2−}\) can be either monodentate or bidentate, since two of its oxygen atoms have lone pairs as shown above and can form covalent bonds with a transition metal ion. In most cases carbonate is monodentate because of its trigonal planar geometry (there is 120 degrees between the oxygens so it's hard for both to bind to the same metal). However, in some cases it will bind to two different metals, making it bidentate.
A19.2.9
CO3-2 will coordinate to a metal center as a monodentate ligand.
Q19.2.10
Draw the geometric, linkage, and ionization isomers for [CoCl5CN][CN].
S19.2.10
Isomers are compounds with same formula but different atom arrangement. There are two subcategories: structural isomers, which are isomers that contain the same number of atoms of each kind but differ in which atoms are bonded to one another, and stereoisomers, isomers that have the same molecular formula and ligands, but differ in the arrangement of those ligands in 3D space.
There are three subcategories under structural isomers: ionization isomers, which are isomers that are identical except for a ligand has exchanging places with an anion or neutral molecule that was originally outside the coordination complex; coordination isomers, isomers that have an interchange of some ligands from the cationic part to the anionic part; and linkage isomers, in two or more coordination compounds in which the donor atom of at least one of the ligands is different.
There are also two main kinds of stereoisomers: geometric isomers, metal complexes that differ only in which ligands are adjacent to one another (cis) or directly across from one another (trans) in the coordination sphere of the metal, and optical isomers, which occurs when the mirror image of an object is non-superimposable on the original object.
Some of the isomers look almost identical, but that is because the CN ligand can be attached by both (but not at the same time) the C or N.
A19.2.10
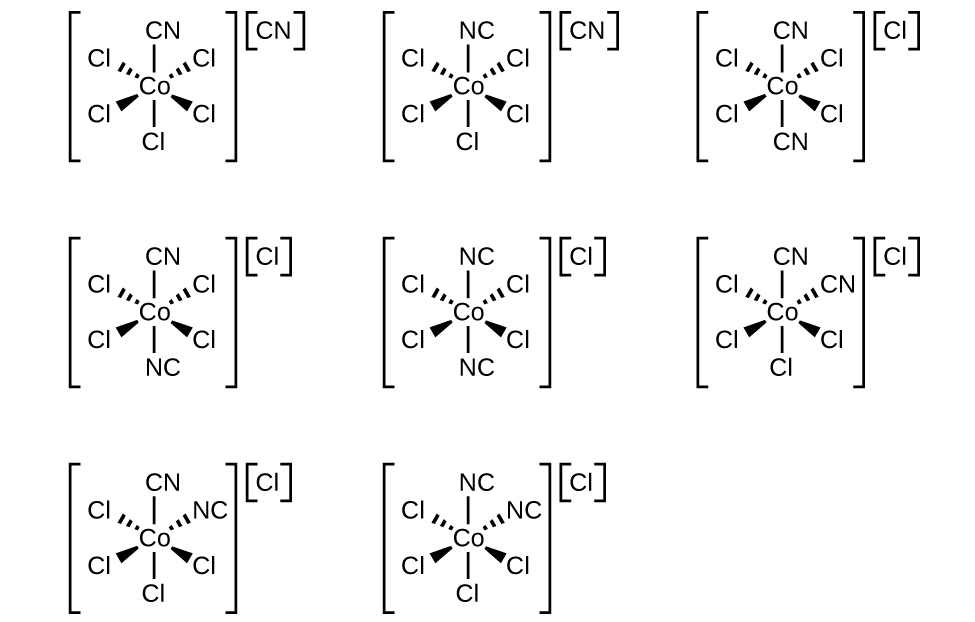
19.3: Spectroscopic and Magnetic Properties of Coordination Compounds
Q19.3.1
Determine the number of unpaired electrons expected for [Fe(NO2)6]3−and for [FeF6]3− in terms of crystal field theory.
S19.3.1
- The crystal field theory is is a model that describes the breaking of degeneracies of electron orbital states, usually d or f orbitals, due to a static electric field produced by a surrounding charge distribution.
- The degenerate d-orbitals split into two levels, e\(_{g}\) and t\(_{2g}\), in the presence of ligands.
- The energy difference between the two levels is called the crystal-field splitting energy, \(\Delta_{\circ}\).
- After 1 electron each has been filled in the three t\(_{2g}\) orbitals, the filling of the fourth electron takes place either in the e\(_{g}\) orbital or in the t\(_{2g}\), where the electrons pair up. depending on whether the complex is high spin or low spin.
- If the \(\Delta_{\circ}\) value of a ligand is less than the pairing energy (P), then the electrons enter the e\(_{g}\) orbital, but if the \(\Delta_{\circ}\) value of a ligand is more than the pairing energy (P), then the electrons enter the t\(_{2g}\) orbital.
- when the
is less than the pairing energy, the electrons prefer then eg orbitals because there is not enough energy to pair the electrons together. It will be high spin
- when the
is more then the pairing energy, the electrons prefer the t2g because there is enough energy to pair the electrons. It will be low spin.
Step 1: Determine the oxidation state of the Fe
For \([Fe(NO_{2}){_{6}}]^{3-}\) and \([FeF_{6}]^{3-}\), both \(NO_{2}\) and \(F_{6}\) have a charge of -1. Since there is 6 of them then that means the charge is -6 and in order for there to be an overall charge of -3, Fe has to have a +3 charge.
Step 2: Determine type of ligand
Based on the spectrochemical series we can see that \(NO_{2}^{-}\) is a stronger field ligand than F\(^{-}\), and therefore is a low spin complex because it has a high \(\Delta_{\circ}\) unlike F\(^{-}\) which is a high spin.
Step 3: Draw the crystal field
\([Fe(NO_{2}){_{6}}]^{3-}\)
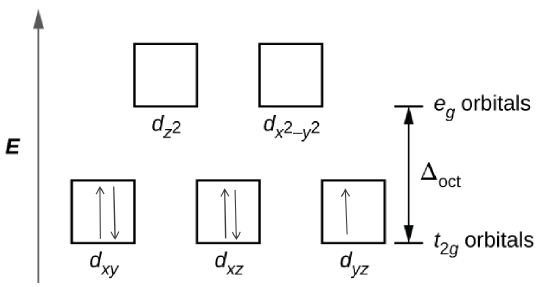
\([FeF_{6}]^{3-}\)
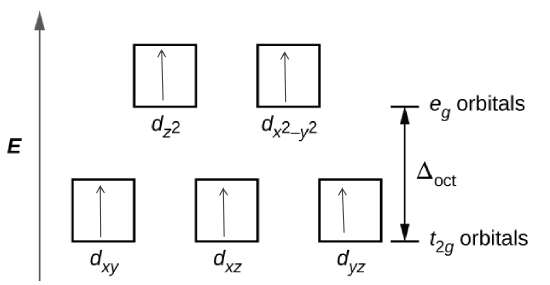
There is 1 unpaired electron for \([Fe(NO_{2}){_{6}}]^{3-}\), and 5 for \([FeF_{6}]^{3-}\) based on the crystal field theory.
A19.3.1
[Fe(NO2)6]3−:1 electron
[FeF6]3−:5 electrons
Q19.3.2
Draw the crystal field diagrams for [Fe(NO2)6]4− and [FeF6]2−. State whether each complex is high spin or low spin, paramagnetic or diamagnetic, and compare Δoct to P for each complex.
S19.3.2
a) \[[Fe(NO_2)_6]^{_{-4}}\]
- NO2- has a -1 charge. The overall ion has a -4 charge, therefore Fe must be +2 charge. (The math: \(x+(6)(-1)=-4, x+-6=-4, x=+2\) or 2+(6*-1)=-4)
- Fe2+ has 6 valence electrons.
- Next we look at the ligand bonded to Fe, which is NO2- . Based on the spectrochemical Series, NO2- is a strong field ligand meaning that it has a large DELTAo large splitting energy in comparison to the pairing energy, P. So the electrons would rather pair up, as it takes the least amount of energy.
- So [Fe(NO2)6]4− is low spin.
- All 6 electrons are paired up, so it is diamagnetic.
b) \[[FeF_6]^{_{-3}}\]
- F- has a -1 charge. The overall ion has a -3 charge, therefore Fe must be +3 charge. (The math: \(x+(-1)(6)=-3, x+-6=-3, x=+3\) or 3+(6*-1)=-3)
- F3+ has 5 valence electrons.
- Next we look at the ligand bonded to Fe, which is F- . Based on the Spectrochemical Series, F- is a weak field ligand, meaning that it has a small DELTAo or small splitting energy in comparison to the pairing energy, P. So the electrons would rather split up and move up to the higher energy level, rather than pairing up, as it takes the least amount of energy.
- So [Fe(NO2)6]4− is high spin.
- [FeF6]3− is paramagnetic because it has unpaired electrons.
A19.3.2
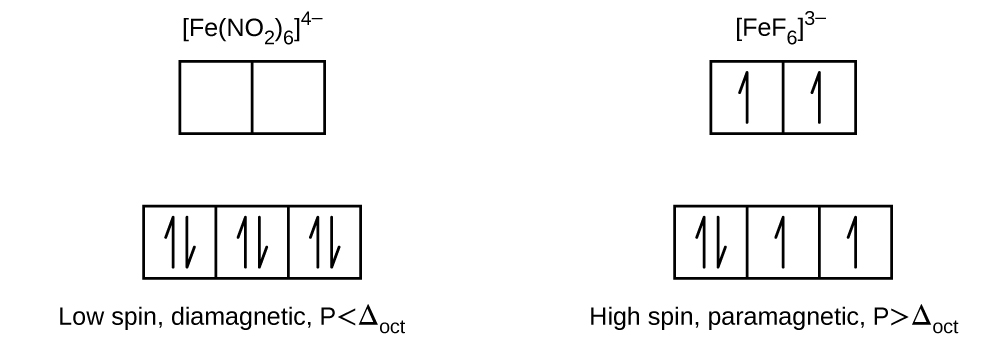
Q19.3.3
Give the oxidation state of the metal, number of d electrons, and the number of unpaired electrons predicted for [Co(NH3)6]Cl3.
S19.3.3
The oxidation state of the metal can be found by identifying the charge of one of each molecule in the coordinate compound, multiplying each molecule's charge by the respective number of molecules present, and adding the products. This final sum represents the charge of the overall coordination compound. You can then solve for the oxidation state of the metal algebraically. In this case, one chloride anion Cl- has a charge of -1. So three chloride anions have a total charge of -3. One ammine ligand NH3 has no charge so six ammine ligands have a total charge of zero. Finally, we are trying to solve for the oxidation state of a cobalt ion. Now we can write the equation that adds the total charges of each molecule or ion and is equal to the total charge of the overall coordinate compound.
\[ (\text{oxidation}\text{ state}\text{ of}\text{ Co})+(-3)+0=0 \]
\[ \text{oxidation}\text{ state}\text{ of}\text{ Co}=+3 \]
So the oxidation state of Co is +3. Now we need to identify the number of d-electrons in the Co3+ ion. The electron configuration for cobalt that has no charge is
\[ [\text{Ar}]4\text{s}^23\text{d}^7 \]
However, a Co3+ ion has 3 less electrons than its neutral counterpart and has an electron configuration of
\[ [\text{Ar}]3\text{d}^6 \]
For transition metals, the \( \text{s} \) electrons are lost first. So cobalt loses its two \( 4\text{s} \) electrons first and then loses a single \( 3\text{d} \) electron meaning Co3+ ion has 6 \( \text{d} \) electrons. To predict the number of unpaired electrons, we must first determine if the complex is high spin or low spin. Whether the complex is high spin or low spin is determined by the ligand in the coordinate complex. Specifically, the ligand must be identified as either a weak-field ligand or a strong-field ligand based on the spectrochemical series. Weak-field ligands induce high spin while strong-field ligands induce low spin. We can then construct the energy diagram or crystal field diagram of the designated spin that has the proper electron placings. The geometric shape of the compound must also be identified to construct the correct diagram. Finally, from this crystal field diagram we can determine the number of unpaired electrons. The number of electrons in the diagram is equal to the number of \( \text{d} \) electrons of the metal. The ligand in this case is NH3, which is a strong field ligand according to the spectrochemical series. This means that the complex is low spin. Additionally, six monodentate ligands means the ligand field is octahedral. The number of electrons that will be in the diagram is 6 since the metal ion Co3+ has 6 \( \text{d} \) electrons. Now the proper crystal field diagram can be constructed.
.jpg?revision=1&size=bestfit&width=180&height=230)
From the crystal field diagram, we can tell that the complex has no unpaired electrons.
A19.3.3
a) 3+
b) 6 d electrons
c) No unpaired electrons
Q19.3.4
The solid anhydrous solid CoCl2 is blue in color. Because it readily absorbs water from the air, it is used as a humidity indicator to monitor if equipment (such as a cell phone) has been exposed to excessive levels of moisture. Predict what product is formed by this reaction, and how many unpaired electrons this complex will have.
S19.3.4
From our knowledge of ligands and coordination compounds (or if you need a refresher Coordination Compounds), we can assume the product of CoCl2 in water. H2O is a common weak field ligand that forms six ligand bonds around the central Cobalt atom while the Chloride stays on the outer sphere. We can use this to determine the complex:
\([Co(H_2O)_6]Cl_2\)
From this formation, we can use the Crystal Field Theory (CFT)(Crystal Field Theory) to determine the number of unpaired electrons. This coordination compound has six ligand bonds attached to the central atom which means the CFT model will follow the octahedral splitting. Keep in mind that we know H2O is a weak field ligand and will produce a high spin. High spin is when the electrons pairing energy (P) is greater than the octahedral splitting energy. Thus, the electrons spread out and maximize spin.
In order to fill out our crystal field diagram, we need to determine the charge of cobalt. Because the H2O ligand is neutral, and there are two chlorine ions, we can deduce the charge of cobalt is plus two in order to make the coordination complex neutral. From here, we can use the electron configuration of Co2+ is [Ar]4s23d7. The electrons that are taken away from the cobalt atom in order to form the plus two charge will from the 4s orbital and leave the 3d orbital untouched. Thus, there will be 7 electrons in the crystal field diagram and appear as:
We can see here that there are 3 unpaired electrons.
A19.3.4
[Co(H2O)6]Cl2 with three unpaired electrons.
Q19.3.5
Is it possible for a complex of a metal in the transition series to have six unpaired electrons? Explain.
A19.3.5
Is it possible for a complex of a metal in the transition series to have six unpaired electrons? Explain.
It is not possible for a metal in the transition series to have six unpaired electrons. This is because transition metals have a general electron configuration of (n-1)d1-10 ns1-2 where n is the quantum number. The last electron will go into the d orbital which has 5 orbitals that can each contain 2 electrons, yielding 10 electrons total. According to Hund's Rule, electrons prefer to fill each orbital singly before they pair up. This is more energetically favorable. Since there are only 5 orbitals and due to Hund's Rule, the maximum number of unpaired electrons a transition metal can have is 5. Therefore, there cannot be a complex of a transition metal that has 6 unpaired electrons.
For example, lets look at iron's electron configuration. Iron has an electron configuration of 1s22s22p63s23p64s23d6. Now the most important orbital to look at is the d orbital which has 6 electrons in it, but there are only 4 unpaired electrons as you can see by this diagram:
3d: [↿⇂] [↿] [↿][↿][↿]
Each [ ] represents an orbital within the d orbital. This diagram follows Hund's rule and shows why no transition metal can have 6 unpaired electrons.
Q19.3.6
How many unpaired electrons are present in each of the following?
- [CoF6]3− (high spin)
- [Mn(CN)6]3− (low spin)
- [Mn(CN)6]4− (low spin)
- [MnCl6]4− (high spin)
- [RhCl6]3− (low spin)
S19.3.6
1. For [CoF6]3-, we first found the oxidation state of Co, which is 3+ since F has a 1- charge and since there is 6 F, Co's charge has to be 3+ for the overall charge to be 3-.
\[\textrm{charge of Co} + \textrm{-6} = \textrm{-6}\] \[\textrm{charge of Co} = \textrm{+3}\]
After finding the oxidation state, I then go to the periodic table to find its electron configuration: [Ar]3d6
We distribute the 6 d-orbital electrons along the complex and since it is high spin, the electrons is distributed once in each energy level before it is paired. There is only one pair and the other 4 electrons are unpaired, making the answer 4.

2. The same process is repeated. We find the charge of Mn, which is 3+, making the electron configuration: [Ar]3d4
\[\textrm{charge of Mn} + \textrm{-6} = \textrm{-3}\] \[\textrm{charge of Mn} = \textrm{+3}\]
There is a difference between this and number 1. This is low spin so instead of distributing one electron in each level before pairing it, I must distribute one electron on the bottom and then pair them all up before I'm able to move to the top portion. So since there is 4, there is only a pair at dyz and the other two electrons are unpaired. Making the number of unpaired electrons 2.
3.The same process as number 2 is applied. The only difference is that the charge of Mn is now 2+ so the electron configuration: [Ar]3d5.
\[\textrm{charge of Mn} + \textrm{-6} = \textrm{-4}\] \[\textrm{charge of Mn} = \textrm{+2}\]
Since is it low spin like number 2, I only need to add an extra electron to the next level, making that 2 pairs of electron and only 1 electron unpaired.

4. Since Cl has a -1 charge like CN, Mn's charge is also 2+ with the same electron configuration as number 3, which is 5.
\[\textrm{charge of Mn} + \textrm{-6} = \textrm{-4}\] \[\textrm{charge of Mn} = \textrm{+2}\]
With 5 electrons, this is high spin instead of low. So as stated in number 1, we pair distribute the electrons on all levels first. Since there are 5 electrons and 5 levels and they are al distributed, there are zero pairs, making that 5 unpaired electrons.

5. Using the same process as the problems above, Rh's charge is 3+, with the electron configuration: [Kr]4d6.
\[\textrm{charge of Rh} + \textrm{-6} = \textrm{-3}\] \[\textrm{charge of Mn} = \textrm{+3}\]
With a low spin and 6 electrons, all electrons are paired up, making it 0 electrons that are unpaired.

A19.3.6
4; 2; 1; 5; 0
Q19.3.7
Explain how the diphosphate ion, [O3P−O−PO3]4−, can function as a water softener that prevents the precipitation of Fe2+ as an insoluble iron salt.
S19.3.7
The diphosphate ion, [O3P−O−PO3]4− can function as a water softener keeping the iron in a water soluble form because of its more negative electrochemical potential than water's. This is similar to the way plating prevents metals from reacting with oxygen to corrode.
Mineral deposits are formed by ionic reactions. The Fe2+ will form an insoluble iron salt of iron(III) oxide-hydroxide when a salt of ferric iron hydrolyzes water. However, with the addition of [O3P−O−PO3]4−, the Fe2+ cations are more attracted to the PO3 group, forming a Fe(PO3) complex.
The excess minerals in this type of water is considered hard thus its name hard water.
Q19.3.8
For complexes of the same metal ion with no change in oxidation number, the stability increases as the number of electrons in the t2g orbitals increases. Which complex in each of the following pairs of complexes is more stable?
- [Fe(H2O)6]2+ or [Fe(CN)6]4−
- [Co(NH3)6]3+ or [CoF6]3−
- [Mn(CN)6]4− or [MnCl6]4−
S19.3.8
The Spectrochemical Series is as follows \[I^{-}<Br^{-}<SCN^{-}\approx Cl^{-}<F^{-}<OH^{-}<ONO^{-}<ox<H_{2}O<SCN^{-}<EDTA<NH_{3}<en<NO_{2}^{-}<CN\]
The strong field ligands (on the right) are low spin which fills in more electrons in the t2g orbitals. The weak field ligands (on the left) are high spin so it can fill electrons in the t2g orbitals and eg orbitals. In conclusion, more electrons are filled up from the strong field ligands because the electrons don't move up to the eg orbitals.
a. \([Fe(CN)_{6}]^{4-}\)
\(CN\) is a stronger ligand than \(H_{2}O\) so it is low spin, which fills up the t2g orbitals.
b. \([Co(NH_{3})_{6}]^{3+}\)
\(NH_{3}\) is a stronger ligand than \(F\).
c. \([Mn(CN)_{6}]^{4-}\)
\(CN\) is a stronger ligand than \(Cl^{-}\).
For more information regarding the shape of the complex and d-electron configuration, libretext provides more information on how to classify high and low spin complexes.
A19.3.8
[Fe(CN)6]4−; [Co(NH3)6]3+; [Mn(CN)6]4−
Q19.3.9
Trimethylphosphine, P(CH3)3, can act as a ligand by donating the lone pair of electrons on the phosphorus atom. If trimethylphosphine is added to a solution of nickel(II) chloride in acetone, a blue compound that has a molecular mass of approximately 270 g and contains 21.5% Ni, 26.0% Cl, and 52.5% P(CH3)3 can be isolated. This blue compound does not have any isomeric forms. What are the geometry and molecular formula of the blue compound?
S19.3.9
1)Find the empirical formula. There is a total of 270 grams. To find out how many grams of each element/compound there are, multiply the percentage by the mass (270).
\[(270g)(0.215)=58.05gNi\]
\[(270g)(0.26)=70.2gCl\]
\[(270g)(0.525)=141.75gP(CH_3)_3\]
Now that we have the grams of each element/compound, we can convert them to moles by using their molar mass.
\[(58.055gNi)(\frac{1mol.}{58.69gNi})=0.989mol.Ni\]
\[(70.2gCl)(\frac{1mol.}{35.45gCl})=1.98mol.Cl\]
\[(141.75gP(CH_3)_3)(\frac{1mol.}{76.07gP(CH_3)_3})=1.86mol.P(CH_3)_3\]
Now that we have the moles of all elements/compounds, we can find the ratio of all them to each other. To do this, we take the element/compound with the least amount of moles and divide all element/compound moles by this amount. In this case, Ni has the least number of moles.
\[\frac{0.989mol.Ni}{0.989mol.Ni}=1\]
\[\frac{1.98mol.Cl}{0.989mol.Ni}=approx.2\]
\[\frac{1.86mol.P(CH_3)_3}{0.989mol.Ni}=approx.2\]
We now know the ratio of all element/compounds in the blue compound.
The empirical formula is: NiCl(P(CH3)3)2
This formula shows us there are 4 ligands. There are 2 chlorine ligands and 2 trimethylphosphine ligands. This means that the blue compound has either a tetrahedral or square planar shape, where tetrahedral shapes are capable of different isomeric forms when all ligands are different (because if not, there is only 1 way for them to be arranged), and square planar shapes are capable of cis/trans forms. In the problem, it states this compound does not have any isomeric forms, therefore this has a tetrahedral shape.
A19.3.9
a) NiCl(P(CH3)3)2
b) Tetrahedral
Q19.3.10
Would you expect the complex [Co(en)3]Cl3 to have any unpaired electrons? Any isomers?
S19.3.10
Assign oxidation states to each element. Cl- has a -1 oxidation state. En is neutral, so 0. The entire complex is also neutral, so in order to balance the charges out, Co must be +3 because there are 3 chlorides, which gives a -3 charge.
STEP 2:
Write the electron configuration for \(Co^{3+}\). \([Ar]3d^6\). There are 6 electrons.
STEP 3:

Check where en lies on the spectrochemical series. Does it have a strong field strength? It does, so these electrons will exist at the d-level with high splitting energy because the magnitude of the pairing energy is less than the crystal field splitting energy in the octahedral field.
You will the notice that there aren't any unpaired electrons when you draw the Crystal Field Theory (CFT) diagram.
This complex does not have any geometric isomers because cis-trans structures cannot be formed. The mirror image is nonsuperimpoasable, which means the enantiomers are chiral molecules; if the mirror image is placed on top on the original molecule, then they will never be perfectly aligned to give the same molecule.
A19.3.10
The complex does not have any unpaired electrons. The complex does not have any geometric isomers, but the mirror image is nonsuperimposable, so it has an optical isomer.
Q19.3.11
Would you expect the Mg3[Cr(CN)6]2 to be diamagnetic or paramagnetic? Explain your reasoning.
S19.3.11
The first step to determine the magnetism of the complex is to calculate the oxidation state of the transition metal. In this case, the transition metal is Cr.
Before doing so, we need to find charge of the of the complex ion [Cr(CN)6]2 given that the oxidation state of Mg3 is 2+. Using the subscripts of the \(\displaystyle Mg^{2+}\) ion and the [Cr(CN)6]2 complex, we find that the oxidation state of [Cr(CN)6]2 , x, to be:
\(\displaystyle 3(+2)+2(x)=0\)
\(\displaystyle x=3\)
Now that we found the charge of the coordination complex, we are able to find the charge of the transition metal Cr given that the charge of CN is -1. Again, using the subscripts we find the oxidation state of Cr, y, to be:
\(\displaystyle y + 6(-1)=-3\)
\(\displaystyle y=3\)
Therefore, the oxidation state of the transition metal Cr is \(\displaystyle Cr^{3+}\)
Next, using the transition metal \(\displaystyle Cr^{3+}\) and the periodic table as reference, we can determine the electron configuration of \(\displaystyle Cr^{3+}\) to be \(\displaystyle [Ar]d^{3}\). This means that \(\displaystyle Cr^{3+}\) has 3 unpaired electrons in the 3d sublevel. Therefore, we find that since at least one electron is unpaired(in this case all 3 electrons are unpaired), Mg3[Cr(CN)6]2 is paramagnetic.
A19.3.11
a) Paramagnetic
Q19.3.12
Would you expect salts of the gold ion, Au+, to be colored? Explain.
S19.3.12
No. Colored ions have unpaired electrons in their outmost orbital. A partially filled d orbital, for example, can yield various colors. After completing the noble gas configuration, we see that Au+ has a configuration of [Xe] 4f145d10. Since Au+ has a completely filled d sublevel, we are certain that any salts of the gold ion, Au+ will be colorless.
*An example of a colored ion would be copper(II). Cu2+ has an electron configuration of [Ar]3d9. It has one unpaired electron. Copper(II) appears blue.
A19.3.12
No. Au+ has a complete 5d sublevel.
Q19.3.13
[CuCl4]2− is green. [Cu(H2O)6]2+is blue. Which absorbs higher-energy photons? Which is predicted to have a larger crystal field splitting?
S19.3.13
Although a color might appear a certain way, it actual absorbs a different color, opposite of it on the color wheel. 
In this case;
[CuCl4]2- appears green but is opposite of red on the color wheel which is absorbed and is characterized by wavelengths 620-800 nanometers.
[Cu(H2O)6]2+ appears blue but is opposite of orange on the color wheel which is absorbed and is characterized by wavelengths 580-620 nanometers.
When determining which absorbs the higher energy photons, one must look at the complex itself. A higher energy indicates a high energy photon absorbed and a lower energy indicates a lower energy photon absorbed. How can we determine this? By looking at the complex and more specifically the ligand attached and its location in the spectrochemical series.
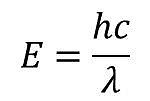

The ligands attached are Water and Chlorine and since Water is a stronger ligand than Chlorine according to the series, it also has larger energy, indicating a higher energy. This means that the complex [Cu(H2O)6]2+ absorbs a higher energy photon because of its a stronger ligand than chlorine.
Part 2 of this question also asks which complex is predicted to have a larger crystal field splitting. To determine this you also use the spectrochemical series and see which ligand is stronger. Since H2O is stronger than Cl- on the spectrochemical series, we can say [Cu(H2O)6]2+ has a higher crystal field splitting.
A19.3.13
a) [Cu(H2O)6]2+
b) [Cu(H2O)6]2+ has a higher crystal field splitting



