5.1: Hydrogen, Oxygen, and Water
- Page ID
- 46574
The words "acid" and "base" are
functional terms, and not labels.
They describe what a substance does,
rather than what it is.
R. von Handler (b. 1931)
IntroductionEdit
Almost all the reactions that a chemist is concerned with take place in solution rather than in gaseous or solid phases. Most of these reactions occur in aqueous solution, where water is the solvent. There are good reasons for this preference for liquid media. Molecules must come into contact to react, and the rates of migration of atoms or molecules within crystals usually are too slow to be useful. In contrast, molecules of gases are mobile, but gas volumes are inconveniently large, and many substances cannot be brought into the gas phase without decomposing. Solutions of reacting molecules in liquids offer an optimum combination of compactness, ease of handling, and rapidity of mixing of different substances.
As we saw in Chapter 1, water has special virtues as a solvent. It is polar, in the sense illustrated in Figure 5-1. The oxygen atom draws the electrons of the 0 - H bonds toward itself, acquiring a slight negative charge and leaving small positive charges on the two hydrogen atoms. Water therefore can interact with other polar molecules. Moreover, water molecules dissociate to a small extent into H+ and OH- ions, a property that is important in acid-base reactions. This chapter is concerned with reactions and equilibria in aqueous solution, especially those involving acids and bases.
Equilibria in Aqueous SolutionsEdit

If reactants and products in a chemical reaction are in solution, the form of the equilibrium-constant expression is the same as for gas reactions, but the logical units of concentration are moles per liter of solution (units of molarity).
aA + bBcC + dD (4-7) Keq =
(4-8)
Some reactions in aqueous solution involve water as a participant. A well-studied example is the hydrolysis ("splitting by water") of the ethyl acetate molecule to yield acetic acid and ethyl alcohol (ethanol):

Because all the other participant molecules themselves are polar, they dissolve well in water, which is therefore a good dispersing agent. In addition, water plays a direct role as a reactant molecule.
The equilibrium-constant expression for this reaction, in principle, is
K'eq =(5-2)
However, since water is present in such excess in its role as solvent, the water concentration is virtually unchanged during the reaction. In dilute solutions this is approximately the concentration of water in its pure state.
H2O =55.6 moles liter-1 (5-3)
This constant water concentration can be brought over to the left side of equation 5-2 and incorporated into the equilibrium constant, as we saw for condensed phases in Chapter 4, so the equilibrium-constant expression becomes
Keq = K'eq[H2O] =(5-4)
Other reactions in aqueous solution involve ions; an example is the precipitation of silver ions with chloride ions, in the form of insoluble silver chloride:
-
- Ag+ + Cl-
 AgCl(s)
AgCl(s)
- Ag+ + Cl-
In this process water is not a direct reactant or product, but it does interact with the ions to keep them in solution. Any ion in aqueous solution is hydrated, or surrounded by polar water molecules as in Figure 5-1d. If the central ion is positive (a cation), then the negatively charged oxygen atoms of the water molecules are pointed toward it; if the central ion is negative (an anion), the positively charged hydrogen atoms of the water molecules are closest.
Each hydrated ion thus is stabilized by an immediate environment of charges opposite in sign to its own charge. When a salt crystal dissolves in water, the attractions between ions of opposite charge in the crystal are broken. In compensation, similar attractions are set up between ions and the hydrating water molecules. Solubility of salt crystals is the result of a balance or competition between crystal forces and hydration forces. This is why salts do not dissolve in nonpolar solvents such as benzene, which cannot offer hydrating attractions.
Ionization of Water and the pH ScaleEdit
Water itself ionizes to a small extent:
H2O(l)H+ + OH- (5-5)
Each ion is surrounded with polar water molecules (as Na+ and OH- are in Figure 5-1d). The hydrated state of the proton, H+, is sometimes represented as H3O+, meaning H+ • H20. But this is an unnecessary and even misleading notation. A more accurate representation of a hydrated proton would be H9O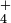 , or H+ • (H20)4, to represent the cluster:
, or H+ • (H20)4, to represent the cluster:
We will assume that H+ and OH-, like all other ions, are hydrated in aqueous solution, and we will therefore represent them simply as H+ and OH-.
| Kw = [H+][OH-] | ||||
| T (°C): | 0 | 25 | 40 | 60 |
| Kw | 0.115  10-14 10-14 |
1.008  10-14 10-14 |
2.95  10-14 10-14 |
9.5  10-14 10-14 |
The equilibrium-constant expression for the dissociation of water is
K'eq(5-6)
The constant [H2O] form can be combined with K'eq as before, producing
Kw = 55.6K'eq = [H+][OH-] (5-7)
This new equilibrium constant, Kw, is called the ion-product constant for water. Like most equilibrium constants, Kw varies with temperature. Some experimental values of the ion-product constant are given in Table 5-1.
| From the data in Table 5-1 and Le Chatelier's principle, predict whether the dissociation of water liberates or absorbs heat. |
|
Solution Since a higher temperature favors dissociation, dissociation is an endothermic or heat-absorbing process. From Appendix 3, ΔH(diss of H20) = +55.90 kJ mole-1. This is the energy required to break one O—H bond, thereby leaving both electrons with the oxygen atom. |
It is customary to take Kw,/sub> = 1.00  10,sup>-14 as being accurate enough for room-temperature equilibrium calculations. (It is also customary in acid base equilibrium calculations to write Kw as if it were an exact number, 10,sup>-14 rather than 1.00
10,sup>-14 as being accurate enough for room-temperature equilibrium calculations. (It is also customary in acid base equilibrium calculations to write Kw as if it were an exact number, 10,sup>-14 rather than 1.00  10-14.) This means that in pure water, where the concentrations of hydrogen and hydroxide ions are equal,
10-14.) This means that in pure water, where the concentrations of hydrogen and hydroxide ions are equal,
[H+] = [OH-] = 10-7 mole liter-1 (5-8)

Since large powers of 10 are clumsy to deal with, a logarithmic notation has been devised, called the pH scale (Figure 5-2). (The symbol pH stands for "negative power of hydrogen ion concentration.") The pH is the negative logarithm of [H+]:
pH = -log10[H+] (5-9)
If the hydrogen ion concentration is 10-7 mole liter-1, then
-
- pH = -log10(10-7) = +7
By an analogous definition,
pOH = -log10[OH-] (5-10)
and the pOH of pure water is also +7. The equilibrium constant Kw also can be expressed in logarithmic terms:
pKw = -log10Kw = +14 (5-11)
Finally, the equilibrium expression for dissociation of water,
[H+][OH-] = Kw = 10-14 (5-12)
can be written
pH + pOH = 14 (5-13)
In an acid solution, [H+] is greater than 10-7, so the pH is less than 7. The ion-product equilibrium still holds, and [OH-] can be found from the expression
[OH-] =(5-14)
or
pOH = pKw - pH = 14 - pH (5-15)
The approximate pH values of some common solutions are given in Table 5-2.
| Substance | pH |
|---|---|
| Commercial concentrated HCl (37% by weight) | ~ -1.1 |
| 1 M;; HCl solution | 0.0 |
| Gastric Juice | 1.4 |
| Lemon Juice | 2.1 |
| Orange Juice | 2.8 |
| Wine | 3.5 |
| Tomato Juice | 4.0 |
| Black Coffee | 5.0 |
| Urine | 6.0 |
| Rainwater | 6.5 |
| Milk | 6.9 |
| Pure Wtaer at 24°C | 7.0 |
| Blood | 7.4 |
| Baking soda solution | 8.5 |
| Borax solution | 9.2 |
| Limewater | 10.5 |
| Household ammonia | 11.9 |
| 1M NaOH solution | 14.0 |
| Saturated NaOH solution | ~15.0 |
| From Table 5-2, what is the hydrogen ion concentration of orange juice? What is the hydroxide ion concentration? |
|
Solution Since the pH of orange juice is 2.8, the hydrogen ion concentration is
The hydroxide ion concentration can be obtained by either of two equivalent methods:
or
|
| What is the ratio of hydrogen ions to hydroxide ions in pure water? In orange juice? |
|
Solution In pure water the ratio is 10-7 to 10-7 or 1 to 1. In orange juice, from Table 5-2, the ratio is 1.6 |
To maintain equilibrium, the added H+ ions from the juice have pushed the water dissociation reaction in the direction of undissociated H20, thereby removing OH- ions from the solution. Orange juice is not a particularly strong acid, and the enormous fluctuation of ionic ratios even in this example illustrates the usefulness of power-of-ten and logarithmic (pH, pOH, pK) notation.
Strong and Weak AcidsEdit


Arrhenius defined an acid (Chapter 2) as a substance that increases the hydrogen ion concentration of an aqueous solution, and a base as a substance that increases the hydroxide ion concentration. A more general definition was proposed in 1923 by Johannes Bronsted and T. M. Lowry. The Bronsted-Lowry definition can be applied to nonaqueous solutions as well: An acid is any substance that is capable of giving up a hydrogen ion, or proton, and a base is any substance that can combine with and therefore remove a hydrogen ion. Now that we understand that water molecules exist in equilibrium with their dissociated H+ and OH- ions, we can see that the two definitions are equivalent when water is the solvent. Arrhenius and Br~nsted acids are both hydrogen-ion-releasing substances. If a Br~nsted base combines with hydrogen ions, it shifts the equilibrium of equation 5-5 in favor of dissociation until balance is restored. More hy~ droxide ions are formed in the process, so in water a Br~nsted base is an Arrhenius base as well.
In aqueous solution, acids are classified as either strong or weak. Strong acids are completely dissociated or ionized, and they include hydrogen acids such as hydrochloric acid (HCl) and hydroiodic acid (HI), and oxyacids (oxygen-containing acids) such as nitric acid (HN03), sulfuric acid (H2S04), and perchloric acid (HCI04). Each of these acids loses one proton in solution, and the acid-dissociation constant, Ka, is so large (> 103) that too little undissociated acid remains to be measured. (HS0 loses a second proton and is a weak acid.)
loses a second proton and is a weak acid.)
Weak acids have measurable ionization constants in aqueous solution, because they do not dissociate completely. Examples (at 25°C) are
| Sulfuric: | HSO 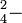 Ka Ka |
= ![\textstyle\frac{[H^+][SO_4^{2-}]}{[HSO_4^-]}](https://upload.wikimedia.org/math/f/8/d/f8dab122b4aa3e28bc19f47c12daa041.png) |
| (2nd Ionization) | = 1.2  10-2 (5-16) 10-2 (5-16) |
|
| Hydrofluoric: | HF  Ka Ka |
= ![\textstyle\frac{[H^+][F^-]}{[HF]}](https://upload.wikimedia.org/math/f/6/0/f6022a96e7d208db9b11438563e91968.png) |
= 3.5  10-4 (5-17) 10-4 (5-17) |
||
| Acetic: | CH3COOH 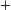 Ka Ka |
= ![\textstyle\frac{[H^+][CH_3COO^-]}{[CH_3COOH]}](https://upload.wikimedia.org/math/c/d/4/cd40665cb04cb67b1b25ff8318b7720f.png) |
= 1.76  10-5 (5-18) 10-5 (5-18) |
||
| Hydrocyanic: | HCN  Ka Ka |
= ![\textstyle\frac{[H^+][CN^-]}{[HCN]}](https://upload.wikimedia.org/math/5/2/5/5257701325d6c4b81a94f58ed63b35e4.png) |
= 4.9  10-10 (5-19) 10-10 (5-19) |
The distinction between strong and weak acids is somewhat artificial. The ionization of HCl is not simply a dissociation; it is, rather, the result of successful competition of H20 molecules with Cl- ions for the proton, H+:
HCl + xH2OH+ • (H2O)x + Cl- (5-20)
In the Bronsted-Lowry theory, any proton donor is an acid, and any proton acceptor is a base (Figure 5-3). Therefore, HCl is an acid, and Cl- is its conjugate base. Since HCl loses a proton readily it is a strong acid, and since Cl- has so little affinity for the proton it is a weak base. In contrast, HCN is a very weak acid, because relatively few HCN molecules release their proton. Its conjugate base, CN-, is a strong base by virtue of its high affinity for a proton.
Water is a somewhat stronger base than Cl-, and when it is present in excess, as in an aqueous solution of HCl, it takes virtually all the protons from HCl, leaving it completely ionized. CN,sup>- is a much stronger base than H2O, so only a small fraction of the protons from HCN become bound to the water molecules. In other words, HCN is only slightly ionized in aqueous solution, as its Ka of 4.9  10-10 indicates.
10-10 indicates.
Because water is present in great excess, any acid whose conjugate base is weaker than H2O (i.e., has a lesser affinity for protons than has H2O) will be ionized almost completely in aqueous solution. We cannot distinguish between the behavior of HCl and of HCl04 (perchloric acid) in water solution. Both are completely dissociated and are therefore strong acids. However, for a solvent with a lesser attraction for protons than water, we do find differences between HCl and HCl04. With diethyl ether as a solvent, perchloric acid is still a strong acid, but HCl is only partially ionized and hence is a weak acid. Diethyl ether does not solvate a proton as strongly as water does (Figure 5-4). (Solvation is a generalization of the concept of hydration, which applies to solvents other than water.) The equilibrium point in the reaction
HCl + xC2H5OC2H5H+ • (C2H5OC2H5)x + Cl- (5-21)
lies far to the left, so HCl is only partially dissociated in ether. Only in an extremely strong acid, such as perchloric acid, does the anion have so little attraction for the proton that it will release it to ether as an acceptor solvent. Clearly, by using solvents other than water, we can see differences in acidity (or proton affinity) that are masked in aqueous solution. This masking of relative acid strengths by solvents such as water is known as the leveling effect.

The dissociation constants for a number of acids in aqueous solution are listed in Table 5-3, with estimates of the Ka for strong acids that are "leveled" by the solvent in aqueous solution. The dissociation of protonated solvent, H3O+, into hydrated protons and H20, represents merely a shuffling of protons from one set of water molecules to another, and must have a Keq of 1.00. In liquid ammonia as a solvent, all acids whose conjugate bases are weaker than NH3 would be leveled by the solvent and would be totally ionized strong acids. Thus hydrofluoric acid and acetic acid are both strong acids in liquid ammonia.
The leveling effect of solvent and the origin of strong and weak acids are summarized in Figure 5-4. T he distinction between strong and weak acids depends on the solvent as much as it does on the inherent properties of the acids themselves. Nevertheless, in aqueous solution the distinction is real. As long as the discussion is confined to aqueous solutions (as ours will be from now on), we shall find it useful to think about and to treat the two classes of acids separately.
Strong and Weak BasesEdit
In Arrhenius' terminology a base is a substance that decreases the hydrogen ion concentration of a solution. Sodium hydroxide, potassium hydroxide, and similar compounds are bases because they dissolve and dissociate completely in aqueous solution to yield hydroxide ions:
NaOHNa + + OH- (5-22) KOH
K+ + OH-
These excess hydroxide ions then disturb the water dissociation equilibrium, and combine with some of the protons normally found in pure water:
H+ + OH-H2 [H+] =
10-7 (5-23)
In the more generalized Bronsted-Lowry defnition, the hydroxide ion itself is the base, because it is the substance that combines with the proton. The Na+ and K+ ions merely provide the positive ions that are necessary for overall electrical neutrality for the chemical compound.
The commonly encountered hydroxides of alkali metals (Li, Na, K) all dissolve and dissociate completely to produce the same Bronsted-Lowry base, OH-. These hydroxides all are strong bases, analogous to strong acids such as HCl and HNO3. Other substances such as ammonia and many organic nitrogen compounds also can combine with protons in solution and act as Bronsted-Lowry bases. These compounds are generally weaker bases than the hydroxide ion, because they have smaller attraction for protons. For example, when ammonia competes with OH- for protons in an aqueous solution, it is only partially successful. It can combine with only a portion of the H+ ions, thus will have a measurable equilibrium constant.
NH3 + H+NH
(5-24)
There is no logical reason why this reaction cannot be described by an acd-dissociation constant, as in Table 5-3. The ammonium ion, NH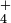 , is the Bronsted-Lowry conjugate acid of the base NH3. There is no reason why, in an acid-base pair, it is the acid that must be neutral and the base charged, as in HCl/Cl- and HCN/CN-. The NH
, is the Bronsted-Lowry conjugate acid of the base NH3. There is no reason why, in an acid-base pair, it is the acid that must be neutral and the base charged, as in HCl/Cl- and HCN/CN-. The NH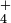 ion is just as respectable an acid as HCl or HCN, and although weaker than HCl, it is actually stronger than HCN. Thus, we can describe the ammonia reaction as an acid dissociation:
ion is just as respectable an acid as HCl or HCN, and although weaker than HCl, it is actually stronger than HCN. Thus, we can describe the ammonia reaction as an acid dissociation:
NH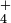
NH3 + H+ Ka= 5.6
10-10 (from Table 5-3) (5-25)
or, if we want to focus on the basic behavior of NH3,
NH3 + H+NH
Keq =
= 1.8
10+9 (5-26)
However, chemical language has become trapped by the older acid-base terminology introduced by Arrhenius, and you should be aware of this. Arrhenius thought of a base as a substance that releases OH- ions into aqueous solution. For alkali metal hydroxides such as NaOH the process was straightforward:
NaOHNa+ + OH- (5-27)
But what about NH3? Where do the hydroxide ions come from? Arrhenius assumed that when ammonia dissolved in water the reaction was
NH3 + H2NH4OH
NH
+ OH- (5-28)
This brought NH3 into line by postulating an intermediate-ammonium hydroxide base that dissociates completely; ammonium hydroxide would be a weak base that dissociates only partially. Arrhenius defined a base-dissociation constant, Kb, as
BOHB+ + OH<sup- Kb =
(5-29)
where B usually represents a metal. For ammonia, Ka and Kb would be related by
Ka =(5-30)
Kb =1.8
10-5 (5-31)
Unfortunately for Arrhenius' theory, there is no evidence that ammonium hydroxide, NH40H, exists as a real compound. It is more accurate to say that the polar ammonia molecule is hydrated like any other polar molecule: NH3 • (H20)x. Ammonia, NH3, combines directly with a proton and with water molecules:
NH3 + H+O + xH2NH
(in acid solutions) (5-32) NH3 + xH2O
NH
+ OH- (in acid solutions)
Nevertheless, Arrhenius' notation is too deeply embedded in the fabric of chemistry to dislodge, and we often will use Kb for weak bases rather than Ka for their conjugate acids. In general, the completely dissociated strong bases that we shall encounter will be hydroxide compounds, and the weak bases will be ammonia and organic nitrogen compounds such as those listed in Table 5-4. Kb always can be found from Ka and Kw and the expression
KaKb = Kw (5-33)

Solutions of Strong Acids and Bases: Neutralization and TitrationEdit
When an amount of strong acid is added to water, the effect is that of adding the same amount of hydrogen ions, since the acid is totally dissociated,
| What is the hydrogen ion concentration of a 0.0l00M nitric acid solution? What is the pH? |
|
Solution
The solution is quite acidic. |
| What are the hydrogen ion concentration and the pH of a 0.0050M sodium hydroxide solution? |
|
Solution The hydroxide ion contribution from completely dissociated NaOH is
This large quantity of hydroxide ions will repress the normal dissociation of water and enhance the reaction to the left:
The hydrogen ion concentration is found from the water equilibrium expression:
The solution is quite basic. |
| If we mix equal volumes of the solutions of the previous two examples, what will be the pH of the resulting solution? | ||||||
|
Solution If equal volumes are mixed, then the concentration of each solute will be halved, since the final volume is twice the volume of each starting solution. The final solution would be 0.0050M in nitric acid and 0.0025M in sodium hydroxide. But acid and base will react and neutralize one another until one or the other is used up:
or simply
since sodium and nitrate ions take no part in the neutralization reaction. In this case, sodium hydroxide is in shorter supply. When all the base has been neutralized, we still have
|
| How many milliliters of 0.10M HCl must we add to 200 ml of 0.0050M KOH to bring the pH down to 10.0? |
|
Solution Without HCl, the pH of the potassium hydroxide solution would be 11.7, as in Example 5. Let y equal the number of milliliters of HCl solution needed to yield a pH of 10.0. Since 0.0050 mole liter-1 is the same as 0.0050 millimoles ml-1, the total number of millimoles (m moles) of KOH is
The total number of millimoles of HCl that must be added is
Since the final solution is basic, nKOH - nHCl. The net amount of hydroxide ions left over after partial neutralization by HCl is
The final volume is
and therefore the final hydroxide in concentration is
A pH of 10.0 means a pOH of 4.0 and [OH-] = 10-4 mole liter-1, thus
and
|
Titration and Titration CurvesEdit
If we add equal numbers of equivalents of a strong acid and a strong base, they will neutralize one another completely, and the pH will be 7.0. As we saw in Chapter 2, this makes possible the titration method of measuring quantities of acid or base.
|
One hundred fifty milliliters of HCl solution of unknown concentration are titrated with 0.10M NaOH. Eighty milliliters of base solution are required to neutralize the acid. How many moles of HCl were present originally, and what was the acid-solution concentration? |
|
Solution The number of millimoles of base used is
This must be the same as the number of millimoles of acid originally present, if neutralization was complete.
|
A common way of determining the equivalence point of titration (the point at which neutralization occurs) is with an acid-base indicator. Indicators are weak organic acids or bases that have different colors in their ionized and neutral states (or in two ionized states). If their color change occurs in the neighborhood of pH 7, and if we add a few drops of indicator solution to the solution being titrated, we see this color change at the end point of the titration. We will discuss some common indicators in the section on weak acids. The matching of indicator color-change point and the end point of a titration does not have to be very exact, because the pH swings drastically through several units as neutralization becomes complete. This can make life easy for the analytical chemist, and it is worth looking more closely at the behavior of pH during titration. To illustrate what we have just said, let us calculate the titration curve for a typical strong acid and strong base.
| Fifty milliliters of 0.10M HN03 are titrated with 0.10M KOH, in an experimental arrangement such as that shown in Figure 2-3. Calculate the pH of the solution as a function of the volume of KOH solution added (v, in milliliters). |
|
Solution It is easiest to treat this calculation in three parts: before neutralization, at neutralization (equivalence point), and after neutralization. Before the equivalence point, calculate how much base has been added, assume that all of this base was used to neutralize some of the acid, and calculate how much acid would remain unneutralized, as a function of the volume of base solution added. |


| Original : | nHNO3 = | 50 ml  0.10 mmole ml-1 = 5.0 mmoles 0.10 mmole ml-1 = 5.0 mmoles |
| Added : | nKOH = | v ml  0.10 mmole ml-1 0.10 mmole ml-1 |
| Net Acid : | nacid = | 5.0 mmoles - 0.10v mmole |
| Total Volume : | V = | 50 + v ml |
| Hydrogen ion | ||
| concentration : | [H+]net = | 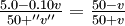 (0.10) mmole ml-1 (0.10) mmole ml-1 |
The calculation of [H+] for various values of v is shown in Table 5-5, and these calculations are plotted with open circles at the left of Figure 5-5. At the equivalence point, the amounts of acid and base are equal and the pH is 7.0. After the equivalence point, we only need to calculate how much base was added in excess of that required to neutralize the acid, and use this to find [OH-j, pOH, and pH:
| Original : | nHNO3 = | 50 mmoles (as before) |
| Added : | nKOH = | v ml  0.10 mmole ml-1 0.10 mmole ml-1 |
| Net Acid : | nbase = | 0.10v - 5.0 mmoles |
| Total Volume : | V = | 50 + v ml |
| Hydrogen ion concentration : | ||
| [OH-] |  (0.10) mmole ml-1 (0.10) mmole ml-1 |
This calculation for several values of v and the corresponding pH values are listed in Table 5-5 and are plotted with solid circles on the right of Figure 5-5. It now is obvious why the choice of an indicator is not too critical in such a titration. Any indicator that changes color between pH 4 and pH 10 will do.
Titrating a weak acid with a strong base, or a weak base with a strong acid, is more complicated because the weak component is only partially dissociated. Dissociation equilibria of the type discussed in the next section must be used. We will not be concerned in this chapter with such titrations, but they are treated in Appendix 5, with an example of a titration curve corresponding to Figure 5-5.
Equilibria with Weak Acids and BasesEdit
Because weak acids are only partially dissociated in water, the contribution of a weak acid such as acetic acid to the hydrogen ion concentration is less than the total concentration of added acid. The equilibrium-constant expression for dissociation of the acid must be used explicitly. These general principles can be illustrated with a concrete example, that of calculating the pH of a solution of 0.0l00M acetic acid. As we saw in Example 5-4 for nitric acid, a strong acid, a 0.0100M solution has a pH of 2.00. Because acetic acid is a weak acid and only partially dissociated, a 0.0 100M solution will have a hydrogen ion concentration of less than 0.0100M, and a pH greater than 2.0.
It is common to represent the acetate ion, CH3COO-, simply by Ac-, and the undissociated acetic acid molecule, CH3COOH, by HAc as if it were a simple inorganic acid. (The forms OAc- and HOAc also are used, to indicate that acetic acid is an oxyacid with the dissociating proton attached to an oxygen atom.) The dissociation of HAc is incomplete:
-
- HAc
 H+ + Ac-
H+ + Ac-
- HAc
and the equilibrium expression describing dissociation is
-
- Ka =
![\textstyle\frac{[H^+][Ac^-]}{[HAc]}](https://upload.wikimedia.org/math/3/3/9/339257471a7b20486eaf1510c314d874.png) = 1.76
= 1.76  10-5 (from Table 5-3)
10-5 (from Table 5-3)
- Ka =
We know the initial overall concentration, c0, of acetic acid:
-
- c0 = 0.0100 mole liter-1
and we know that at equilibrium some of this acetic acid remains undissociated and some of it has ionized to acetate ions, Ac-:
-
- c0 = [HAc] + [Ac-] (mass-balance equation)
This is called a mass-balance equation, because it states that total acetate is neither created nor destroyed during dissociation. We also know that the concentrations of hydrogen ions and acetate ions are equal, since dissociation of HAc is the only source of H+. (It is legitimate to neglect H+ from the dissociation of water, since acetic acid represses water dissociation even below its normal small extent.) Thus
-
- [H+] = [Ac-] (charge-balance equation)
This is known as a charge-balance equation, because it states that the total positive charge in the solution must equal the total negative charge. We now can use these data about conservation of acetate and neutrality of the solution to simplify the equilibrium-constant expression. Let the hydrogen ion concentration that we are seeking be [H+] = y, and eliminate [Ac-] at once using the charge-balance equation:
-
- Ka =
![\textstyle\frac{y^2}{[HAc]}](https://upload.wikimedia.org/math/b/c/2/bc263bb5a7e6fb1290ead8d14a7f6492.png) (eequilibrium equation)
(eequilibrium equation)
- Ka =
-
- c0 = [HAc] + y (mass-balance equation)
The second equation tells us that the concentration od undissociated HAc equals the original overall concentration, c0, minus the amount that has dissociated y:
-
- [HAc] = c0 - y
The equilibrium expression then is
-
- Ka =
 (5-34)
(5-34)
- Ka =
Substituting the value of Ka from Table 5-3, we get
-
- 1.76
 10-5 =
10-5 = 
- 1.76
or
-
- y2 + 1.76
 10-5y -
10-5y -  10-7 = 0
10-7 = 0
- y2 + 1.76
This is a quadratic equation, which can be solved with the quadratic formula. If ay2 + by + c = 0, then
y =(5-35)
For this problem, a = 1, b = 1.76  10-5, and c = -1.76
10-5, and c = -1.76  10-7.
10-7.
-
- y =

- y =
or
-
- y =

- y =
Only the positive answer is reasonable, because one cannot have a negative concentration. Thus the answer is
-
- y = 4.11
 10-4 mole liter-1
10-4 mole liter-1
- y = 4.11
Under certain physical conditions you can take a shortcut to avoid the quadratic formula. In this example, since you know that the acid is only slightly dissociated, you can try neglecting y in the denominator of the equilibrium expression for Ka, thereby assuming that it is small in comparison with 0.0100 mole liter-1, and that the concentration of undissociated acetic acid is virtually the same as the total acetic acid present. This assumption gives
-
- 1.76
 10-5 =
10-5 = 
- 1.76
and an approximate answer of
-
- y = 4.2
 10-4 = 0.00042 mole liter-1
10-4 = 0.00042 mole liter-1
- y = 4.2
This is close to the correct answer of 0.000411 mole liter-1. You can make a quick improvement by using this approximate value in the undissociated acetate concentration in the denominator:
-
- 1.76
 10-5 =
10-5 = 
-
-
-
- = 4.11
 10-4 mole liter-1
10-4 mole liter-1
- = 4.11
-
-
- 1.76
Repetition of the foregoing process until the answer remains constant from one cucle to the next is called the method of successive approximation. If your intuition for how much dissociation the acid undergoes is good enough, you can often solve equilibrium problem by an approximate solution and a quick correction in less time than it takes to solve the quadratic formula. If your original guess is not so good, two or three cycles of approximation may be required before you arrive at an unchanging value for y.
As our results show, acetic acid is indeed only slightly dissociated at 0.0100M concentration. Of the initial 0.0100 mole liter-1, 0.000411 mole has dissociated, and 0.0096 mole remains as dissolved but undissociated HAc molecules. The percent dissociation is
-
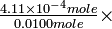 100 = 4.11%
100 = 4.11%
Since the hydrogen ion concentration is [H+] = 4.11  10-4 M, the pH of this solution is 3.39.
10-4 M, the pH of this solution is 3.39.
What happens if we dilute the acetic acid solution? Does a greater or lesser percent of the acetic acid then dissociate? Does the pH increase or decrease?
| What are the pH and percent dissociation in a solution of 0.00100M acetic acid? |
|
Solution The equilibrium expression is as before:
Neglecting y in comparison with co, the approximate solution is
and the solution obtained by using this value to correct the undissociated HAc concentration is
Using this second value to correctc0 in another cycle of approximation makes no change in y, so the process can be halted. Now the pH is 3.91 instead of 3.39, and the percent dissociation is
|
Although the actual hydrogen ion concentration is lower (witness the larger pH), a greater fraction of the HAc present is dissociated into ions. This is Le Chatelier's principle again. If a solution containing HAc, H+, and Ac- is diluted, thereby lowering its total concentration of all ions and molecules, the equilibrium will attempt to reestablish itself, as reactions change, in the direction that will increase the total concentration of solute particles of one kind or another. Compare this behavior with the effect of increasing the pressure on the ammonia gas equilibrium in Chapter 4.
IndicatorsEdit

An indicator is a weak acid (or a weak base) that has sharply different colors in its dissociated and undissociated states. Methyl orange (Figure 5-6) is a complex organic compound that is red n its neutral, un-ionizes form and yellow when ionized. It can be represented as the weak acid HIn:
-
- HIn
 H+ + In-
H+ + In- - red yellow
- HIn
Adding acid shifts the indicator equilibrium to the left, and adding base shifts it to the right. Hence methyl orange is red in acids and yellow in bases.
The intensity of color from indicators such as methyl orange is so great that the colors can be seen easily even when the amount added to a solution is too small to have an appreciable influence on the pH of the solution. Nevertheless, the ratio of dissociated to undissociated indicator depends on the hydrogen ion concentration
Ka =(5-36)
and
(5-37)
log10() = pH - pka (5-38)
For methyl orange, Ka = 1.6 X 10-4 and pKa = 3.8. The neutral (red) and dissociated (yellow) forms of the indicator are present at equal concentrations when the pH = 3.8. The eye is sensitive to color changes over a range of concentration ratios of approximately 100, or over two pH units. Below pH 2.8, a solution containing methyl orange is red, and above approximately 4.8 it is clearly yellow. As you can see from Figure 5-5, an indicator change over two pH units is quite satisfactory for strong acid-base titrations.
Methyl orange could be used for the titration in Figure 5-5, even though its pKa is far from the titration equivalence point of 7.0, only because the change in pH at the equivalence point is so large. For titrations of weak acids, this would not be true, and it would be better to pick an indicator with a pKa closer to the expected equivalence point. Other indicators are shown in Figure 5-7, along with the pH range in which their color changes occur. Phenolphthalein is a particularly convenient and common indicator, which changes from colorless to pink in the range of pH 8 to 10.
Contribution to [H+] from Dissociation of WaterEdit

Nothing has been said in the discussions of either strong acids or weak acids about a contribution to the hydrogen ion concentration from the dissociation of water itself. It has been tacitly assumed that all H+ comes from the acid. This is a valid assumption for all but the most dilute solutions of very weak acids such as HCN. The correction for water dissociation seldom is necessary, so it will not be covered in this chapter. A complete treatment is given in Appendix 5.
Weak Acids and Their SaltsEdit
What will happen to a weak acid such as acetic acid if we add some sodium acetate (NaAc), which is the salt of a strong base (NaOH) and acetic acid? The salt will dissolve and dissociate completely into sodium and acetate ions. From Le Chatelier's principle, we would expect these added acetate ions to force the weak acetic acid equilibrium system in the direction of less dissociation. This is exactly what happens. The acid-equilibrium expression is the same:
Ka =(5-39)
However, two sources of acetate ions now exist: NaAc and HAc. The acetate ion supplied by sodium acetate is measured by cs , the total molarity of the salt, since dissociation is complete. Acetate concentration from acetic acid is measured by the hydrogen ion concentration, since every dissociation of HAc to produce Ac- also produces a proton. Therefore, the total acetate ion concentration is
[Ac-]total = [Ac-]NaAc + [Ac-]HAc = cs + [H+] (5-40)
(Again, we have neglected any protons contributed by the dissociation of water.) The concentration of un-ionized acetic acid is the overall acid concentration, ca, less the acetate from dissociation:
[HAc] = ca - [Ac-]HAc = ca - [H+] (5-41)
If we represent the hydrogen ion concentration by y, we have
Ka =(5-42)
When the added salt concentration, cs, is zero, this is the simple weak acid-dissociation equilibrium expression that we have seen previously in equation 5-34.

|
What are the pH and percent dissociation of a solution of 0.0l0M acetic acid in the presence of (a) no NaAc, (b) 0.0050M NaAc, and (c) 0.010M NaAc? |
|
Solution From Le Chatelier's principle, we would expect the dissociation of HAc to be repressed as more NaAc is added. The pH should increase and the percent dissociation should decrease. (a) This problem was already solved in Section 5-6, yielding pH 3.39 and 4.11% dissociation. (b) For cs = 0.0050 mole liter-1,
This is most easily solved by successive approximations. As a first approximation we can assume that y will be smaller than 0.0050 or 0.010, and we can therefore neglect it when it is added to or subtracted from these quantities:
As a second approximation, we can use this trial value of y tpo "correct" 0.0050 to 0.005035, and 0.010 to 0.009965, and solve the equation again:
A third approximation is unnecessary, and the answer should be rounded to 3.5 ,math>\times</math> 10-5 mole liter-1:
(c) For cs = 0.010 mole liter-1
Notice that the acetic acid now dissociates so little that even the first approximation is adequate. |
Results for these and a few other sodium acetate concentrations are listed in Table 5-6 and are plotted in Figure 5-8. The first salt added has a large effect on the degree of dissociation and pH; later additions of salt cause less change. When acid and salt are present in equal concentrations, the pH is equal to the pKa of the acid.
|
||||||
| cs: | 0.0 | 0.001 | 0.002 | 0.005 | 0.010 | 0.020 |
| break | ||||||
| pH: | 3.4 | 3.8 | 4.1 | 4.5 | 4.8 | 5.1 |
| break | ||||||
| Percent dissociation | 4.1 | 1.5 | 0.84 | 0.35 | 0.18 | 0.09 |
| of acetic acid: | ||||||
BuffersEdit
If the concentrations of a solution of a weak acid and a salt of the acid anion are reasonably high, then the solution is resistant to changes in hydrogen ion concentration.
|
A solution is 0.050M in HAc and 0.050M in NaAc. Calculate the change in pH when 0.0010 mole of hydrochloric acid (HCl) is added to a liter of solution, assuming that the volume increase upon adding the HCl is negligible. Compare this to the pH if the same amount of HCI is added to a liter of pure water. |
|
Solution Before adding HCl the acetic acid equilibrium is
Thus
(Again, we were justified in ignoring y in the [Ac-] and [HAc] terms because the value is small compared to 0.050.) The added protons from HCl combine with the acetate ions to form more acetic acid:
Thus to a good approximation, all the added protons are used up, and the new acetic acid and acetate concentrations are
The pH changes from 4.75 to 4.74, a difference of only 0.01 unit. In the absence of HAc and NaAc, the same concentration of HCl would produce a pH of 3.0. |
This resistance to pH change is called buffering action, and the solution of HAc and NaAc is an acetate buffer. Buffers are used widely for pH control in laboratory chemistry, in the; chemical industry, and in living organisms. A carbonate buffer system in your bloodstream, involving the reaction
H+ + HCO
H2CO3
CO2 + H2O (5-43)
maintains the blood pH around 7.4. When a biochemist studies enzyme activity in the laboratory, he must use a buffer system to maintain a constant pH during the experiments, otherwise his results may have little meaning. One of the sillier disputes in commercial advertising is that between two pharmaceutical companies as to whether buffers added to aspirin to combat an acid reaction in the stomach are a benefit or an adulterant.
In general, if the concentration of strong acid added to a buffer solution is y moles liter-1, the equilibrium equation becomes
Ka =(5-44)
in which cs and ca are the salt and buffering acid concentrations, respectively. After addition of the foreign acid, the hydrogen ion concentration is
[H+] = Ka(5-45)
and the pH is
pH = pKa + log10(5-46)
If base is added, hydrogen ions are removed, and the same expressions can be used with a negative value of y.
|
A formic acid buffer is prepared with 0.010 mole liter-1 each of formic acid (HCOOH) and sodium formate (HCOONa). What is the pH of the solution? What is the pH if 0.0020 mole liter-1 of solid sodium hydroxide (NaOH) is added to a liter of buffer? What would be the pH of the sodium hydroxide solution without buffer? What would the pH have been after adding sodium hydroxide if the buffer concentrations had been 0.10 mole liter-1 instead of 0.0l0? |
||||||||||
|
Solution The answers are
|
In the preceding example, you can see the dramatic effect of the formate buffer in keeping the solution acidic in spite of the added base, and the importance of reasonably high buffer concentrations if the buffering capacity of the solution is not to be exceeded.
Salts of Weak Acids and Strong Bases : HydrolysisEdit
A sodium chloride solution is neutral, with a pH of 7.0. This is reasonable, because sodium hydroxide is a strong base and hydrochloric acid is a strong acid, and if equal amounts of each were added, neutralization would be complete. In contrast, sodium acetate is the salt of a strong base and a weak acid. Intuitively we would expect a sodium acetate solution to be somewhat basic, and it is. Some of the acetate ions from the salt combine with water to form undissociated acetic acid and hydroxide ions:
Ac- + H2OHAc + OH- (5-47)
This sometimes is called a hydrolysis reaction, the implication being that H2O breaks up crystals of sodium acetate. It does, when the salt crystal dissolves in water, but this is not the point. In solution the acetate ion acts as a base. It is as good a Bronsted base as ammonia, and the ammonium ion is a perfectly good acid, like HAc.
-
- NH3 + H2O
 NH
NH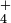 + OH-
+ OH-
- NH3 + H2O
We should not let the different charges on the acetate ion (-1) and ammonia (0) obscure the similarity of their acid-base behavior.
The equilibrium constant for acetate hydrolysis has the same form as any other base dissociation:
Kb =(5-48) Kb =
(5-30)
where, as usual, the virtually unchanging water concentration is incorporated into the equilibrium constant. This constant sometimes is written Kh for "hydrolysis constant," but the added nomenclature is unnecessary. It is a simple base-equilibrium constant of the kind we have seen before, except that acetate ion is the base.
As always, Kb is related to the cooresponding acid-dissociation constant, Ka, by
Kb =(5-49)
(Recall the ammonia-water equilibrium expressions at the end of Section 5-4.) This value is all we need to calculate the pH of a sodium acetate solution.
| What is the pH of a solution of 0.010M NaAc? |
|
Solution Acetate ions from NaAc combine with H20 to produce undissociated HAc molecules and OH- ions (equation 5-47). The equilibrium expression is
Let the hydroxide ion concentration be y. Since every reaction of an acetate ion with water produces one hydroxide ion and one undissociated HAc molecule, the concentration of each of the latter two species must bey moles liter-1. The remaining acetate ions are those originally present from NaAc minus those that have combined with water:
and we arrive at the familiar expression Kb = This is even easier to solve than the weak-acid problems. Since the equilibrium constant is so small, y will be correspondingly small and can be neglected in the denominator in comparison to 0.010. The result is
|
(As before, we have neglected any contribution to the hydrogen ion concentration from water molecules. Our procedure is accurate enough for most situations, including the purposes of this chapter. The full derivation is found in Appendix 5.)
Polyprotic Acids: Acids That Liberate More Than One Hydrogen IonEdit
If water is the solvent, sulfuric acid, H2SO4, loses one proton as a strong acid with an immeasurably large dissociation constant.
-
- H2SO4 → H+ + HSO

- H2SO4 → H+ + HSO
It also can lose a second proton as a weak acid with a measurable dissociation constant. Acids that can liberate more than one proton are called polyprotic acids.
-
- HSO

 H+ + SO
H+ + SO Ka2 = 1.20
Ka2 = 1.20  10-2 pKa2 = 1.92
10-2 pKa2 = 1.92
- HSO
For carbonic acid, H2C03, both dissociations are weak:
-
- H2CO3
 H+ + HCO
H+ + HCO Ka1 = 4.3
Ka1 = 4.3  10-7 pKa1 = 6.37
10-7 pKa1 = 6.37 - HCO

 H+ + CO
H+ + CO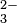 Ka2 = 5.61
Ka2 = 5.61  10-11 pKa2 = 10.25
10-11 pKa2 = 10.25
- H2CO3
The relative values of Ka1 and Ka2 for a given acid are intuitively reasonable. One would expect HCO , which already has a negative charge, to be less ready than neutral H2C03 to lose another proton.
, which already has a negative charge, to be less ready than neutral H2C03 to lose another proton.
-
- Phosphoric acid, H3 PO4, has three dissociations:
- H3PO4
 H+ + H2PO
H+ + H2PO pKa1 = 2.12
pKa1 = 2.12 - H2PO

 H+ + HPO
H+ + HPO pKa2 = 7.21
pKa2 = 7.21 - HPO

 H+ + PO
H+ + PO pKa3 = 12.67
pKa3 = 12.67
Thus, in an aqueous solution of phosphoric acid there will be seven ionic and molecular species present: H3PO4 , H2PO , HPO
, HPO , PO
, PO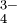 , H2O, H+, and OH-. Life might appear impossibly complicated, were we not able to make some approximations.
, H2O, H+, and OH-. Life might appear impossibly complicated, were we not able to make some approximations.
At a pH equal to the pKa for a particular dissociation, the two forms of the dissociating species are present in equal concentrations. For the second dissociation of phosphoric acid, for which pKa2 = 7.21,
-
-
-
- Ka2 =
![\textstyle\frac{[H^+][HPO_4^{2-}]}{[H_2PO_4^-]}](https://upload.wikimedia.org/math/5/3/2/5329c53cd739ccc318eeda1610575ce3.png)
- Ka2 =
-
- log
![\textstyle\frac{[HPO_4^{2-}]}{[H_2PO_4^-]}](https://upload.wikimedia.org/math/3/8/2/38280aa1e825e29c5dda11c2dd8df6bb.png) = pH - pKa2
= pH - pKa2
-
When pH = pKa2, we have the ratio
-
-
![\textstyle\frac{[HPO_4^{2-}]}{[H_2PO_4^-]}](https://upload.wikimedia.org/math/3/8/2/38280aa1e825e29c5dda11c2dd8df6bb.png) = 1.00
= 1.00
-
Hence, in a neutral solution, H2PO and HPO
and HPO are present in about the same concentrations. Very little undissociated H3PO4 will be found, since from the first dissociation constant,
are present in about the same concentrations. Very little undissociated H3PO4 will be found, since from the first dissociation constant,
-
-
-
- Ka1 =
![\textstyle\frac{[H^+][H_2PO_4^-]}{[H_3PO_4]}](https://upload.wikimedia.org/math/3/0/d/30d2e4c534e104521d650cae8862f78b.png)
- Ka1 =
-
- log
![\textstyle\frac{[H_2PO_4^-]}{[H_3PO_4]}](https://upload.wikimedia.org/math/d/a/0/da0fb5056212ccc8e2c228a287069e25.png) = pH - pKa1 = 7.00 - 2.12 = 4.88
= pH - pKa1 = 7.00 - 2.12 = 4.88 -
![\textstyle\frac{[H_2PO_4^-]}{[H_3PO_4]}](https://upload.wikimedia.org/math/d/a/0/da0fb5056212ccc8e2c228a287069e25.png) = 104.88 = 7.6
= 104.88 = 7.6  104 = 76,000
104 = 76,000
-
Similarly, little PO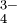 will exist:
will exist:
-
- log
![\textstyle\frac{[PO_4^{3-}]}{[HPO_4^{2-}]} =](https://upload.wikimedia.org/math/4/a/8/4a8ae9f95f42b5364d86e9b9ade008d1.png) pH - pKa3 = 7.00 - 12.67 = -5.67
pH - pKa3 = 7.00 - 12.67 = -5.67 -
![\textstyle\frac{[PO_4^{3-}]}{[HPO_4^{2-}]} =](https://upload.wikimedia.org/math/4/a/8/4a8ae9f95f42b5364d86e9b9ade008d1.png) 10-5.67 = 2.1
10-5.67 = 2.1  10-6 =
10-6 = 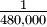
- log
The only phosphate species that we have to consider near pH = 7 are H2PO and HPO
and HPO . Similarly, in strong acid solutions near pH = 3, only H3P04 and H2P0
. Similarly, in strong acid solutions near pH = 3, only H3P04 and H2P0 are important. As long as the pKa's of successive dissociations are separated by three or four units (as they almost always are), matters are simplified.
are important. As long as the pKa's of successive dissociations are separated by three or four units (as they almost always are), matters are simplified.
There is still another simplification. When a polyprotic acid such as carbonic acid, H2CO3, dissociates, most of the protons present come from the first dissociation:
-
- H2CO,sub>3
 H+ + HCO
H+ + HCO pKa1 = 6.37
pKa1 = 6.37
- H2CO,sub>3
Since the second dissociation constant is smaller by four orders of magnitude (and the pKa2 larger by four units), the contribution of hydrogen ions from the second dissociation will be only one ten-thousandth as large. Correspondingly, the second dissociation has a negligible effect on the concentration of the product of the first dissociation, HCO .
.
|
At room temperature and 1 atm CO2 pressure, water saturated in CO2 has a carbonic acid concentration of approximately 0.040 mole liter-1. Calculate the pH and the concentrations of all carbonate species for a 0.040 M H2CO3 solution. |
||||||||||||
|
Solution Considering initially only the first dissociation:
From our experience with acetic acid, which has an even larger Ka, we should expect to be able to neglect y in the denominator. The extent of dissociation of an acid with such a small Ka will be very small:
This is the concentration of both hydrogen ion and bicarbonate ion, HCO
Consequently, carbonated beverages have an acidity somewhere between those of wine and tomato juice (see Table 5-2). For the second dissociation:
Since this second dissociation has only a minor effect on the first one, we can assume that the hydrogen ion and bicarbonate ion concentrations are effectively the same:
Note the rather surprising result that the concentration of the second dissociation product is equal to the second dissociation constant! |
|
Calculate the sulfide ion concentration in a solution saturated in H2S (0.10 mole liter-1, which may be gotten from the application of Henry's Law for the solubility of gases in water) (a) if the solution is made from distilled water and (b) if the solution is made pH = 3.0 with HCl. Use Ka values in Table 5-3. |
||||||||||||
|
Solution In distilled water, the first dissociation is
The dissociation constant is so small that y in the denominator can be neglected immediately. Dissociation will be extremely slight:
From the second dissociation:
As with the H2CO3 example, the anion produced by the second dissociation has a concentration equal to the second dissociation constant.
The acid has repressed the dissociation of H2S, making the sulfide ion concentration only one-hundredth of what it is in pure water. As we shall see in the next section, we can use acids to exert a fine control on sulfide concentration in analytical methods by controlling the pH. |
Equilibria With Slight Soluble SaltsEdit
When most solid salts dissolve in water, they dissociate almost completely into hydrated positive and negative ions. The solubility of a salt in water represents a balance between the attraction of the ions in the crystal lattice and the attraction between these ions and the polar water molecules. This balance may be a delicate one, easily changed in going from one compound to an apparently similar one, or from one temperature to another. It is not possible to give hard-and-fast rules as to whether a compound is soluble, or even to account for all observed behavior.
One important factor certainly is the electrostatic attraction between ions. Crystals made up of small ions that can be packed closely together are generally harder to pull apart than crystals made up of large ions. Therefore, for a given cation, fluorides (F-) and hydroxides (OH-) are less soluble than nitrates (NO ) and perchlorates (ClO
) and perchlorates (ClO ). Chlorides are intermediate in size, and their behavior is difficult to predict from general principles.
). Chlorides are intermediate in size, and their behavior is difficult to predict from general principles.
The charge on the ions also is important. More highly charged ions such as phosphates (PO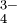 ) and carbonates (CO
) and carbonates (CO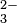 ) interact strongly with cations and are less soluble than the singly charged nitrates and perchlorates.
) interact strongly with cations and are less soluble than the singly charged nitrates and perchlorates.
The terms soluble and insoluble are relative, and the degree of solubility can be related to an equilibrium constant. For a "slightly soluble" salt such as silver chloride, an equilibrium exists between the dissociated ions and the solid compound:
AgCL(s)Ag+ + Cl- (5-51)
The equilibrium expression for this reaction is
Keq =(5-52)
As long as solid AgCl remains, its effect on the equilibrium does not change. As with the H2O concentration in the water dissociation equilibrium, the concentration of the solid salt can be incorporated into the equilibrium constant:
Ksp = Keq[AgCl(s)] = [Ag+][Cl-] (5-53)
This new equilibrium constant, Ksp, is called the solubility-product constant. For substances in which the ions are not in a 1: 1 ratio, the form of the solubility-product expression is analogous to our previous equilibrium expression:
-
-
PbCl2 
Pb2+ + 2Cl- Ksp = [Pb2+][Cl-]2 Al(OH)3 
Al3+ + 3OH- Ksp = [Al3+][OH-]3 Ag2CrO4 
2Ag+ + CrO 
Ksp = [Ag+]+[CrO  ]
]Ba3(PO4)2 
3Ba2+ + 2PO 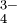
Ksp = [Ba2+]3[PO 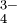 ]2
]2
-
Solubility equilibria are useful in predicting whether a precipitate will form under specified conditions, and in choosing conditions under which two chemical substances in solution can be separated by selective precipitation.
The solubility-product constant of a slightly soluble compound can be calculated from its solubility in moles liter-1.
| The solubility of AgCl in water is 0.000013 mole liter-1 at 25°C. What is its solubility-product constant, Ksp? | ||||||
|
Solution The equilibrium expression is
The concentrations of Ag+ and Cl- are equal because for each mole of solid AgCl that dissolves, 1 mole each of Ag+ and Cl- ions is produced. Hence the concentration of each ion is equal to the overall solubility, s, of the solid in moles liter-1:
|
At a certain temperature the solubility of Fe(OH)2 in water is 7.7  10-6 mole liter-1. Calculate its Ksp at that temperature. 10-6 mole liter-1. Calculate its Ksp at that temperature. |
|
Solution The equilibrium equation is
and the solubility-product expression is
Since one mole of dissolved Fe(OH)2 produces one mole of Fe2+ and two moles of OH-,
|
The solubility-product constants of a number of substances are listed in Table 5-7. Substances are grouped by anion and listed in the order of decreasing Ksp; anions are listed roughly in the order of decreasing solubility. Once the solubility-product constant is known, it can be used to calculate the solubility of a compound at a specified temperature.

| What is the solubility of lead sulfate, PbS04 , in water at 25°C? |
|
Solution The dissociation reaction is
Let the unknown solubility be s moles liter-1. Then since each mole of dissolved PbS04 produces 1 mole of each ion,
The solubility-product equation is
|
|
In Table 5-7 we see that cadmium carbonate, CdC03 , and silver carbonate, Ag2CO3, have approximately the same solubility-product constants. Compare their molar solubilities in water (at 25°C). |
|
Solution For cadmium carbonate,
For Ag2CO3 the expression is slightly different. If the solubility again is s moles liter-1, since each mole of salt produces 2 moles of Ag+ ions,
Although cadmium carbonate and silver carbonate have nearly the same solubility-product constants, their solubilities in moles liter-1 differ by a factor of 100 because the form of the solubility-product expression is different. The solubility of Ag2CO3 is sensitive to the square of the metal-ion concentration, because two silver ions per carbonate ion are necessary to build the solid crystal. |
Common-Ion EffectEdit
In the preceding example, the solubility of silver carbonate in pure water was calculated to be 1.3  10-4 mole liter-1. Will silver carbonate be more soluble or less soluble in silver nitrate solution? Le Chatelier's principle leads us to predict that a new, outside source of silver ions would shift the silver carbonate equilibrium reaction in the direction of less dissociation:
10-4 mole liter-1. Will silver carbonate be more soluble or less soluble in silver nitrate solution? Le Chatelier's principle leads us to predict that a new, outside source of silver ions would shift the silver carbonate equilibrium reaction in the direction of less dissociation:
Ag2 CO32Ag+ + CO
(5-54)
or that silver carbonate would be less soluble in a silver nitrate solution than in pure water. This decrease in the solubility of one salt in a solution of another salt that has a common cation or anion is called the common-ion effect.
| What is the solubility at 25°C of calcium fluoride, CaF2, (a) in pure water, (b) in 0.10M calcium chloride, CaCl2, and (c) in 0.10M sodium fluoride, NaF? | |||||||||
|
Solution (a) If the solubility in pure water is s, then
(b) In 0.01M CaCl2, the calcium ion concentration is the sum of the concentration of calcium ions from calcium chloride and from calcium fluoride, whose solubility we are seeking:
This is a cubic equation, but a moment's thought about the chemistry involved will eliminate the need to solve it as such. With such a small solubility-product constant, you can predict that the solubility of calcium fluoride will be very small in comparison with 0.10 mole liter-1. (You already should realize from (a) and Le Chatelier's principle that in this problem s will be less than 2.1
Therefore the approximation is justified. Only 4.7% as much CaF2 will dissolve in 0.10M CaCl2 as in pure water:
(c) In 0.10M NaF,
since fluoride ions come from NaF as well as from CaF2. The solubility-product equation is
Again, thinking about the chemical meaning will avoid the necessity of solving a cubic equation. The 2s term will be very small compared to 0.10 mole liter-1, therefore,
This approximation is even more valid than the previous one, since from the calculation
only 0.0019% as much CaF2 will dissolve in 0.10M NaF as in pure water. Fluoride is more effective than calcium as a common ion because it has a second-power effect on the solubility equilibrium. |
The common-ion method of controlling solubility often is used with solutions of sulfide ion, S2-, because many metals form insoluble sulfides, and the sulfide ion concentration can be controlled by adjusting the pH.
| What is the maximum possible concentration of Ni2+ ion in water at 25°C that is saturated with H2S and maintained at pH 3.0 with HCl? |
|
Solution From the solubility-product equilibrium equation we predict that too much nickel ion will cause the precipitation of nickel sulfide, NiS:
The only new twist to this problem is finding the sulfide ion concentration from the H2S equilibrium. Hydrogen sulfide dissociates in two steps, each with an equilibrium constant:
Because the overall dissociation is the sum of two dissociation steps, the overall equilibrium constant, Ka1.2, is the product of Ka1 and Ka2:
Saturated H2S is approximately 0.10M at 25°C (which can be gotten from Henry's Law for the solubility of gases in water), and the very small value of Ka1.2 means that dissociation of H2S is very slight. Hence we can write
in a saturated H2S solution. This "ion product" for saturated H2S is a useful relationship to remember. In this problem, the pH has been adjusted to 3.0 with hydrochloric acid, so
Therefore, the sulfide ion concentration can be calculated from
which gives
Since NiS will precipitate if the solubility product is exceeded, the highest value that the nickel ion concentration can have is
|
Separation of Compounds by PrecipitationEdit
Solubility-product constants can be used to devise methods for separating ions solution by selective precipitation. The entire traditional qualitative-analysis scheme is based on the use of these equilibrium constants to determine the correct precipitating ions and the correct strategy.
| A solution is 0.010M in barium chloride, BaCl2, and 0.020M in strontium chloride, SrCl2. Can either Ba2+ or Sr2+ be precipitated selectively with concentrated sodium sulfate, Na2SO4, solution? Which ion will precipitate first? When the second ion just begins to precipitate, what is the residual concentration of the first ion, and what fraction of the original amount of the first ion is left in solution? (For simplicity, assume that the Na2SO4 solution is so concentrated that the volume change in the Ba-Sr solution can be neglected.) |
|
Solution The upper limit on barium sulfate solubility is given by
With 0.010 mole liter-1 of Ba2+, precipitation of barium sulfate will not occur until the sulfate ion concentration increases to
Strontium sulfate will precipitate when the sulfate concentration is
Therefore, barium will precipitate first. When the sulfate concentration has risen to 3.8
The quantity is
or 0.39% of the original Ba2+ present. Thus 99.6% of the barium has been precipitated before any strontium begins to precipitate. |
SummaryEdit
In this chapter we have applied the concepts of chemical equilibrium to ions in aqueous solution, especially to acid-base and precipitation reactions. We have used the equilibrium-constant expression from Chapter 4, with concentrations expressed in units of moles per liter (moles liter-1). Since the concentration of water is effectively constant, especially in dilute solutions, we have incorporated all water concentration terms, [H20], into the equilibrium constants.
Water itself ionizes with an equilibrium or ion-product constant at room temperature of Kw = [H+][OH-] = 10-14. To avoid the inconvenience of large exponential numbers, a negative exponent notation is used, whereby pH = -log10[H+], pOH = -log10[OH-], and pKeq = -log10 Keq. In this notation, the dissociation of water can be represented by pH + pOH = pKw = 14. For pure water, [H+] and [OH-] must be the same, and equal to 10-7 mole liter-1, so the pH and pOH each are equal to 7. The pH is a convenient measure of acidity, since in acid solutions the pH is less than 7, and in basic solutions it is greater than 7.
According to the Bronsted-Lowry theory of acids and bases, any substance that gives up a proton is an acid, and any substance that can combine with a proton and remove it from solution is a base. When an acid loses its proton, it becomes the conjugate base. A strong acid such as HCl has a weak conjugate base, Cl-, and a weak acid such as HAc or NH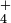 has a relatively strong conjugate base, Ac- or NH3. Any acid whose conjugate base is sufficiently weaker than H2O (has a lesser affinity for H+) will be dissociated completely in aqueous solution, hence it is classified as a strong acid. Acids that dissociate only partially in aqueous solution are weak acids.
has a relatively strong conjugate base, Ac- or NH3. Any acid whose conjugate base is sufficiently weaker than H2O (has a lesser affinity for H+) will be dissociated completely in aqueous solution, hence it is classified as a strong acid. Acids that dissociate only partially in aqueous solution are weak acids.
Strong acids and bases are simple to deal with, since their dissociation is complete in aqueous solution. When a strong acid is added to water, the increase in hydrogen ion concentration equals the concentration of added acid. Neutralization occurs when H+ from an acid combines with OH- from a base to form water molecules. The amount of acid present in a sample can be determined by finding out how much base of known strength is required to make the solution neutral as measured by an acid-base indicator. This is called titration, and it is a useful analytical procedure.
The equilibrium expression for a weak acid, equation 5-34, is obtained with the help of two conservation expressions: a mass-balance equation that says the total amount of acid anion is constant, and a charge-balance equation that says the solution must remain neutral as a whole. This simple expression can be solved as a quadratic equation or by the method of successive approximations, and it is valid as long as the solution is so acid that the contribution to [H+] from the dissociation of water can be neglected. If this is not the case, then a more complete expression (Appendix 5) must be used. Acid-base indicators themselves are weak acids or weak bases whose dissociated and undissociated forms have different colors.
A buffer is a mixture of a weak acid and its salt with a strong base, or alternatively, of a weak base and its salt with a strong acid. The equilibrium between the acid and salt form of the substances shifts to counteract the effect of added acid or base, making the buffered solution resistant to pH change. The pH in such solutions can be calculated from equations 5-42 and 5-46.
Hydrolysis is the interaction of the salt of a weak acid (or weak base) with water to form undissociated acid (or base) and OH- (or H+) ions. What is sometimes described as a hydrolysis constant is actually nothing more than the dissociation constant for the conjugate of the weak acid or base. The base constant, Kb, and the acid constant, Ka, are related by KaKb = Kw.
Some acids can release more than one proton in successive dissociations. These are called polyprotic acids. As long as the successive dissociation constants, K1, K2 , and so on, differ by factors of 10-4 or 10-5, the successive dissociations can be treated as separate events.
Most of the general comments just made about solving acid-base equilibrium problems are applicable to solubility equilibria, for situations in which ions combine to form an insoluble salt. Solubility-product calculations are more useful to indicate whether precipitation will occur under certain conditions, what the upper limit on concentration of an ion in solution may be, and whether two ions can be separated in solution by selective precipitation.



 6.3
6.3 ![\textstyle\frac{K_w}{[OH^-]} = \frac{10^{-14}}{0.0050} =](https://upload.wikimedia.org/math/3/4/0/340e010d24433ba942e9edb709162219.png) 2.0
2.0 
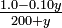 = 10-4
= 10-4 0.053 m moles ml-1 or mole liter-1
0.053 m moles ml-1 or mole liter-1

 12.4%
12.4% (from equation 5-42)
(from equation 5-42) 3.52
3.52  3.48
3.48 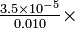 100 = 0.35%
100 = 0.35%![\textstyle\frac{[H^+][Ac^-]}{[HAc]} = \frac{y(0.050)}{(0.050)}](https://upload.wikimedia.org/math/e/d/e/ede609165664bce5f238319d62d5060b.png)
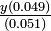
 1.83
1.83 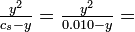 5.68
5.68 ![\textstyle\frac{K_w}{[OH^-]} = \frac{10^{-14}}{2.4 \times 10^{-6}}](https://upload.wikimedia.org/math/4/e/1/4e1a4a726bc3b30e45052a6a3b272184.png) = 4.2
= 4.2  in which y = [H^+]
in which y = [H^+]![\textstyle\frac{[H^+][CO_3^{2-}]}{[HCO_3^-]}](https://upload.wikimedia.org/math/f/4/2/f4216beaea2019904ed06cc660228366.png)
![\textstyle\frac{[HCO_3^-]}{[H^+]} \times](https://upload.wikimedia.org/math/c/f/a/cfa94ccdc702c926067a2a00491ce2af.png) Ka2 = Ka2 = 5.6
Ka2 = Ka2 = 5.6 
![\textstyle\frac{[HS^-]}{[H^+]} \times](https://upload.wikimedia.org/math/7/0/5/705734bb0bbb1ade0c494d0d8eaf1158.png) Ka2 = Ka2 = 1.1
Ka2 = Ka2 = 1.1 ![\textstyle\frac{[H^+][HS^-]}{[H_2S]} = \frac{1.0 \times 10^{-3} [HS^-]}{0.10} =](https://upload.wikimedia.org/math/a/e/1/ae152bb34d5afa5fa506a43e2ab4565c.png) 9.1
9.1 ![\textstyle\frac{[H^+][S^{2-}]}{[HS^-]} = \frac{1.0 \times 10^{-3}[S^{2-}]}{9.1 \times 10^{-6}}](https://upload.wikimedia.org/math/5/d/c/5dc2c25e52284f3f2917e11f40325947.png) = 1.1
= 1.1  1.0
1.0  = 9.75
= 9.75 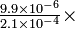 100 = 4.7%
100 = 4.7% 100 = 0.0019%
100 = 0.0019%![\textstyle\frac{[H^+][HS^-]}{[H_2S]} \times \frac{[H^+][S^{2-}]}{[HS^-]} = \frac{[H^+]^2[S^{2-}]}{[H_2S]}](https://upload.wikimedia.org/math/8/4/7/8472f7f2fa28040526d72d9870d745ef.png)
![\times \textstyle\frac{[H_2S]}{[H^+]^2} =](https://upload.wikimedia.org/math/5/c/b/5cb722e7b4943dc716a3104f94f10625.png) 1.0
1.0 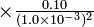
 = 3
= 3  = 1.5
= 1.5 ![\textstyle\frac{K_{sp(SrSO_4)}}{[Sr^{2+}]} = \frac{7.6 \times 10^{-7}}{0.020} =](https://upload.wikimedia.org/math/c/8/d/c8dc0fe7daa3d0005a9899644663b342.png) 3.8
3.8  = 3.9
= 3.9  100 = 0.39%
100 = 0.39%