3.10.1: Biology- Muscle Energy from ATP
- Page ID
- 50746
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
We have looked at sugar as a source of [Weight of Food and Energy Production | energy production] in our bodies in the last few sections, but we also know that the mechanism by which chemical energy is delivered to muscles isn't the same as the mechanism for combustion of a sugar:
C6H12O6(s) + 6 O2(g) → 6 CO2(g) + 6 H2O(l)
(25o, 1 Atm) ΔHm = –2808 kJ mol–1 (1)
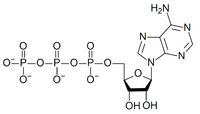
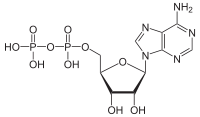
In our bodies, adenosine Triphosphate, ATP, and adenosine diphosphate, ADP, are central intermediaries in energy delivery.
|
ATP |
ADP |
The energy of sugar metabolism is stored in the form of accumulated ATP, and when energy is needed by muscle, it is delivered by the reaction [1]
ATP4- + H2O → ADP3- + HPO42- + H+ ΔHm = ~ –22 kJ mol–1[2]
which is shown in an animation here.
But it just doesn't make sense that this reaction should release energy, because it involves breaking a bond to remove HPO42-, and breaking a bond should require energy. Since so many biologists misinterpret this reaction, we'll try to explain where the heat energy that drives our muscles (and growth) comes from more clearly, in terms of standard enthalpies of formation, below.
It's clear that there is almost an infinite number of chemical reactions whose heat energy is important to know, and chemists have measured the enthalpy changes for so many reactions that it would take several large volumes to list all the thermochemical equations. Fortunately Hess' law makes it possible to list a single value, the standard enthalpy of formation ΔHf, for each compound. The standard enthalpy of formation is the enthalpy change when 1 mol of a pure substance is formed from its elements. Each element must be in the physical and chemical form which is most stable at normal atmospheric pressure and a specified temperature (usually 25°C).
For example, if we know that ΔHf[H2O(l)] = –285.8 kJ mol–1, we can immediately write the thermochemical equation
H2(g) + ½O2(g) → H2O(l) ΔHm = –285.8 kJ mol–1 (2) The elements H and O appear as diatomic molecules and in gaseous form because these are their most stable chemical and physical states. Note also that 285.8 kJ are given off per mole of H2O(l) formed, because stronger bonds are formed (2 H-O bonds), releasing a lot of energy, while weaker bonds (O-O and H-H) are broken, requiring less energy. Equation (1) must specify formation of 1 mol H2O(l), and so the coefficient of O2 must be ½.
The heat of formation of Cl atoms makes it clear that bond breaking is endothermic:
Cl2 → 2 Cl ΔHm = +121.68 kJ mol–1 (3)
In some cases, such as that of water, the elements will react directly to form a compound, and measurement of the heat absorbed serves to determine ΔHf. Quite often, however, elements do not react directly with each other to form the desired compound, and ΔHf must be calculated by combining the enthalpy changes for other reactions. This is the case for ATP
Some Standard Enthalpies of Formation at 25°C.
| Compound |
ΔHf/kJ mol–1 |
ΔHf/kcal mol–1 |
Compound |
ΔHf/kJ mol–1 |
ΔHf/kcal mol–1 |
|---|---|---|---|---|---|
| AgCl(s) | –127.068 | –30.35 | H2O(g) | –241.818 | –57.79 |
| AgN3(s) | +620.6 | +148.3 | H2O(l) | –285.8 | –68.3 |
| Ag2O(s) | –31.0 | –7.41 | H2O2(l) | –187.78 | –44.86 |
| Al2O3(s) | –1675.7 | –400.40 | H2S(g) | –20.63 | –4.93 |
| Br2(l) | 0.0 | 0.00 | HgO(s) | –90.83 | –21.70 |
| Br2(g) | +30.907 | +7.385 | I2(s) | 0.0 | 0.0 |
| C(s), graphite | 0.0 | 0.00 | I2(g) | +62.438 | +14.92 |
| C(s), diamond | +1.895 | +0.453 | KCl(s) | –436.747 | –104.36 |
| CH4(g) | –74.81 | –17.88 | KBr(s) | –393.798 | –94.097 |
| CO(g) | –110.525 | –26.41 | MgO(s) | –601.7 | –143.77 |
| CO2(g) | –393.509 | –94.05 | NH3(g) | –46.11 | –11.02 |
| C2H2(g) | +226.73 | +54.18 | NO(g) | +90.25 | +21.57 |
| C2H4(g) | +52.26 | +12.49 | NO2(g) | +33.18 | +7.93 |
| C2H6(g) | –84.68 | –20.23 | N2O4(g) | +9.16 | +2.19 |
| C6H6(l) | +49.03 | +11.72 | NF3(g) | –124.7 | –29.80 |
| CaO(s) | –635.09 | –151.75 | NaBr(s) | –361.062 | –86.28 |
| CaCO3(s) | –1206.92 | –288.39 | NaCl(s) | –411.153 | –98.24 |
| CuO(s) | –157.3 | –37.59 | O3(g) | +142.7 | +34.11 |
| Fe2O3(s) | –824.2 | –196.9 | SO2(g) | –296.83 | –70.93 |
| HBr(g) | –36.4 | –8.70 | SO3(g) | –395.72 | –94.56 |
| HCl(g) | –92.307 | –22.06 | ZnO(s) | –348.28 | –83.22 |
| HI(g) | +26.48 | +6.33 |
One further point arises from the definition of ΔHf. The standard enthalpy of formation for an element in its most stable state must be zero. If we form oxygen from its elements, for example, we are talking about the reaction
O2(g) → O2(g)
Since the oxygen is unchanged, there can be no enthalpy change, and ΔHf = 0 kJ mol–1.
Standard enthalpies of formation for some common compounds are given in the table above. These values may be used to calculate ΔHm for any chemical reaction so long as all the compounds involved appear in the tables. To see how and why this may be done, consider the following example.
EXAMPLE 1 Use standard enthalpies of formation below to estimate [3] ΔHm for the reaction
P2O74- + H2O → 2 HPO42- (1)
Note that this is like the decomposition (or "hydrolysis") of ATP to give ADP + HPO42-, but simpler. It still releases energy, even though a bond is ostensibly broken between ATP and HPO42-, requiring energy. What isn't seen is very important: The heats of formation of ions include "solvation energies", or in this case, energies released when water molecules bond to the HPO42-, releasing significant amounts of energy. The energy released by "hydration" is greater than the energy required to break the P-O-P bond in ATP.
| chemical species | ΔHf, kJ mol-1 |
|---|---|
| ATP4- | -2982 |
| H2O (l) | -287 |
| ADP3- | -2000 |
| HPO42- | -1299 |
| P2O74- | -2286 |
| H+ | O |
Solution
We can imagine that the reaction takes place in two steps, each of which involves only a standard enthalpy of formation. In the first step the reactants are broken into their elements, and in the second step the elements are recombined to give the products.
First, P2O74- ("pyrophosphate ion") is decomposed to its elements:
P2O74-(aq) → 2P(s) + 7/2 O2(g) ΔHm = ΔH2 (2)
Since this is the reverse of formation of 1 mol P2O74- from its elements, the enthalpy change is ΔH2 = {–ΔHf [P2O74-(aq)]} = [– (–2286 kJ mol–1)] = +2286 kJ mol–1. It's positive (endothermic) because bond breaking takes energy.
Next, we break water into its elements:
H2O (l) → H2 + 1/2O2 (3)
Again ΔH3 = -(-287) = +287 kJ mol–1.
In the second step the elements are combined to give 2 mol HPO42-("inorganic monophosphate ion" or "hydrogen phosphate ion"): 2P(s) + H2 + 2O2(g) → 2 HPO42-(aq) ΔHm = ΔH4 (4) In this case ΔH4 = 2 × ΔHf [HPO42-(aq)] = 2 × (– 1299 kJ mol–1) = – -2598 kJ mol–1 You can easily verify that the sum of Eqs. (2) and (3) is P2O74- + H2O → 2 HPO42- ΔHm = ΔHnet Therefore ΔHnet = ΔH2 + ΔH3 + ΔH4= 287 kJ mol–1 +2286 kJ mol–1 – 2598 kJ mol–1 = – 25 kJ mol–1 Note carefully how Example 2 was solved. In step 1 the reactant compound P2O74- (aq) was hypothetically decomposed to its elements. This equation was the reverse of formation of the compound, and so ΔH2 was opposite in sign from ΔHf. In step 2 we had the hypothetical decomposition of the other reactant, water, again ΔH2 was opposite in sign from ΔHf. Finally, 2 moles of the product HPO42-(aq) were formed from its elements. Since 2 mol were obtained, the enthalpy change was doubled but its sign remained the same.
Any chemical reaction can be approached similarly. To calculate ΔHm we add all the ΔHf values for the products, multiplying each by the appropriate coefficient, as in step 2 above. Since the signs of ΔHf for the reactants had to be reversed in step 1, we subtract them, again multiplying by appropriate coefficients. This can he summarized by the equation
ΔHm = ∑ ΔHf (products) – ∑ ΔHf (reactants) (4) The symbol Σ means “the sum of.” Since ΔHf values are given per mole of compound, you must be sure to multiply each ΔHf by an appropriate coefficient derived from the equation for which ΔHm is being calculated.
EXAMPLE 2 Use the table of standard enthalpies of formation at 25°C to calculate ΔHm for the reaction
ATP4- + H2O → ADP3- + HPO42- + H+
Once more, remember that the energy released comes from the fact that bonding between water molecules and the HPO42- that is released releases more energy than it takes to break the P-O-P bond in ATP to give ADP + HPO42-.
Solution Using Eq. (4), we have
ΔHm = ∑ ΔHf (products) – ∑ ΔHf (reactants) = [ΔHf(ADP3-) + ΔHf (HPO42-)] – [ΔHf ((H2O)+ ΔHf (ATP4-)]
= (–2000) kJ mol–1 + (-1299) kJ mol–1 – (–2982 kJ mol–1) – (–287 kJ mol–1) = -30 kJ mol–1
Note that we were careful to use ΔHf [H2O(l)] not ΔHf [H2O(g)].
Even though water vapor is not the most stable form of water at 25°C, we can still use its ΔHf value to do an interesting calculation: Find the heat energy required to vaporize 1 mole of water (we know that should be positive. It takes energy to boil water because we're breaking bonds of attraction between water molecules. H2O(l) → H2O(g)] ΔHf = ?
ΔHm = ∑ ΔHf (products) – ∑ ΔHf (reactants)
= - 241.8 -(- 285.8) = - 241.8 + 285.8 = + 44 kJ mol–1
References
- www.users.csbsju.edu/~hjakubo...TP_ADPtemp.htm
- Values for ΔHm range from below 20kJ mol–1 released to over 30 because biological systems are complex, and the heat energy changes depend on the presence or absence of many other molecules and ions. The reaction occurs under a range of conditions.
- We use the word "estimate" here because biological systems are complex, and the heat energy changes depend on the presence of many other molecules. The values given are imprecise.