2.7: Magnetic Measurements
- Page ID
- 202257
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)There are a number of physical methods used to study the properties of metal ions in biology. These studies may be performed on simple model compounds. Model compounds are coordination compounds that are designed to mimic the coordination environment of a metal ion in biology. For example, if a metal ion appears to be coordinated by three histidines, then an appropriate model complex might have three ligands with nitrogen donors. The hope is that the simpler coordination complex might still provide some insight into the properties of the metal centre in the much more complicated metalloprotein.
Frequently, measurements can also be perfomed on the metalloprotein itself. Obviously this provides much more useful information, at least potentially, because the results describe the system of interest, rather than some simplified model. However, sometimes obtaining the data reliably can be more troublesome with the metalloprotein.
Physical methods used in the study of metalloproteins include cyclic voltammetry and other electrochemical studies. These methods tell us directly about the reduction potential of the material. In addition, electron paramagnetic resonance spectroscopy (EPR) can provide information about the environment of unpaired electrons in the sample. That information can give us some insight into the structure of a metal complex.
Like EPR, magnetic measurements can provide some information about the presence of unpaired electrons in a sample. That's because unpaired electrons have an attraction for magnetic fields. We can actually measure that attraction to a magnetic field to get some idea about how many unpaired electrons are present. In principle, the more unpaired electrons, the stronger the attraction to the magnetic field. That means magnetic measurements can tell us about the oxidation state of a material.
In principle, a magnetic measurement can be done very simply. All it takes is a balance and a magnetic field. We all know how a balance works. In a simple model from an earlier time, we place the sample in one pan. The balance tips over. We find the appropriate weight - a little piece of carefully prepared metal, certified by some bureau of standards. The balance balances. The masses in the two pans must be equal.

With a Gouy balance, the same idea applies, but we throw in a magnetic field, too. Even when it should be balanced, the balance tips, because of an attraction to the magnetic field. We need to find another weight that will get the balance even again. The weight needed to balance the scale is proportional to the attraction of the material to the magnetic field.
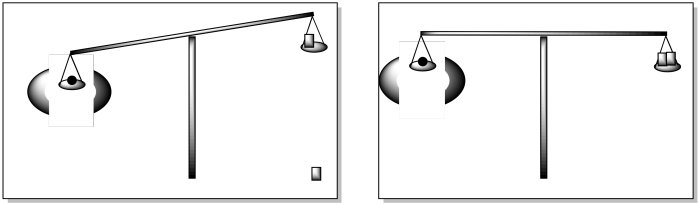
All sorts of calculations ensue. We know the mass of the original material, and we presumably know its molecular weight, so we know the number of moles of material in the balance. That leads us to a value called the molar magnetic susceptibility, ΧM. The magnetic moment actually changes with temperature, so we need to take that into account to report "the effective magnetic moment."
\[\mu_{eff} = 2.83 \sqrt{XMT} \beta \nonumber\]
n which T is the temperature and β is a constant, the Bohr magneton. Usually the value is just left in units of β so we don't have to plug that value in.
In a very simple world, that effective magnetic moment is simply caused by the unpaired electrons in the sample. The predicted value is called μso, the "spin only" magnetic moment. That value is related to the unpaired spins by:
\[\mu_{so} = \sqrt{n(n+2)} \beta \nonumber\]
in which n is the number of unpaired electrons.
Ideally, the effective magnetic moment is just caused by those unpaired electrons, so it is the same as the spin only effective moment.
\[\mu_{so} = \mu_{eff} \nonumber\]
That makes it fairly easy to estimate the number of unpaired electrons in the sample, given the effective magnetic moment.
Estimate μeff for each of the following numbers of unpaired electrons.
a) n = 0 b) n = 1 c) n = 2 d) n = 3 e) n = 4 f) n = 5
- Answer a
-
a) 0 β
- Answer b
-
b) 1.73 β
- Answer c
-
c) 2.83 β
- Answer d
-
d) 3.87 β
- Answer e
-
e) 4.89 β
- Answer f
-
f) 5.92 β
Overall, the relationship is simpler than it initially appears. The effective magnetic moment, in Bohr magneton units, is always approximately one more than the number of unpaired electrons.
\[n= \mu_{eff} -1 \nonumber\]
Estimate μeff for each of the following metals.
a) octahedral Co2+ (high spin) b) octahedral V2+ c) tetrahedral Mn4+ (low spin) d) tetrahedral Mo4+
e) octahedral Cr2+ (high spin) f) octahedral Co3+ (low spin) g) octahedral Mn2+ (high spin)
- Answer a
-
a) approx. 4 β
- Answer b
-
b) approx. 4 β
- Answer c
-
c) approx. 2 β
- Answer d
-
d) approx. 3 β
- Answer e
-
e) approx. 5 β
- Answer f
-
f) approx. 0 β
- Answer g
-
g) approx. 6 β


