4.8: Chemical Formulas, the Mole, and Percentage Composition
- Page ID
- 285259
Chemical formulas represent the composition of chemical compounds. A number of chemical formulas have been shown so far including H2O for water and NH3 for ammonia. A chemical formula of a compound contains a lot of significant information as shown in Figure 4.11. Included is the following:
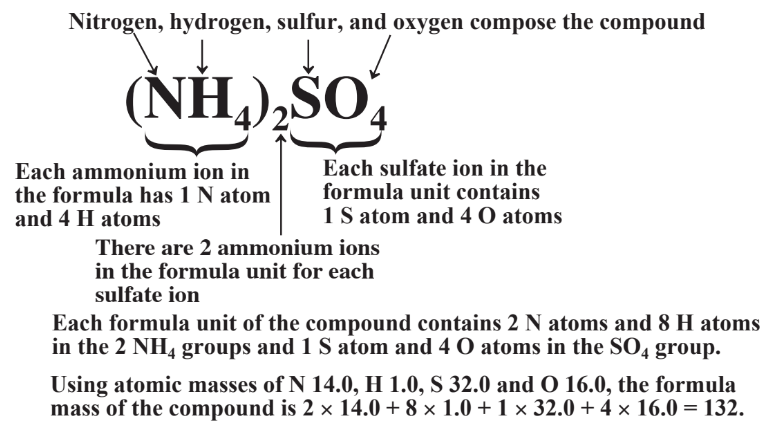
• The elements that compose the compound
• The relative numbers of each kind of atom in the compound
• How the atoms are grouped, such as in ions (for example, SO42-) present in the compound
• With a knowledge of atomic masses, the molar mass of the compound
• With a knowledge of atomic masses, the percentage composition of the compound
Where the symbols of the elements represent letters in the alphabet of chemical language, the formulas of compounds represent words composed of those letters. As discussed in Chapter 5, formulas are put together in chemical equations that act as sentences in the chemical language to describe what chemical substances do.
The Mole
With a knowledge of atomic masses, the percentage composition of a compound is readily calculated from its formula. Before doing such a calculation for ammonium sulfate, however, it is useful to introduce the concept of the mole. Chemists use the mole to express quantities of materials containing a specific number of specified entities, which may be atoms of elements, molecules of elements that exist as diatomic molecules, formula units of ionic compounds, or molecules of covalent compounds. A mole of a substance is simply the atomic mass, molecular mass, or formula mass followed by grams. This quantity is called the molar mass. The masses of a mole of several typical substances are given below:
Atoms of neon, atomic mass 20.1: 20.1 g/mol
Molecules of H2, atomic mass 1.0, molecular mass 2.0: 2.0 g/mole
Molecules of CH4, molecular mass 16.0: 16.0 g/mole
Formula units of ionic CaO, formula mass 56.1: 56.1 g/mol
The number of specified entities in a mole of a substance is always the same regardless of the substance. This number is very large, 6.02×1023, and is called Avogadro’s number. As examples, a mole of neon contains 6.02×1023 neon atoms, a mole of elemental hydrogen contains 6.02×1023 H2 molecules (but 2×6.02×1023 H atoms), and a mole of CaO contains 6.02×1023 formula units (pairs of Ca2+ and O2- ions) of CaO.
The calculation of the percentage composition of (NH4)2SO4 is given below. Note that the molar mass of the compound is 132 g/mol, each mol of the substance contains 2×1 = 2 mol of N, 2×4 = 8mol of H, 1 mol of S, and 4 mol of O.
\(\textrm{2 mol N} \times \textrm{14.0g N/mol N} = 28.0 \textrm{g N, % N} = \frac{28.0g}{132 g} \times 100 = 21.2 \textrm{% N}\)
\(\textrm{8 mol H} \times \textrm{1.0 g H/mol H} = 8.0 \textrm{ g H, % H} = \frac{8.0g}{132g} \times 100 = 6.1 \textrm{ % H}\)
\(\textrm{1 mol S} \times \textrm{32.0 g S/mol S} = 32.0 \textrm{ g S, % S} = \frac{32.0 g}{132g} \times 100 = 24.2 \textrm{% S}\)
\(\textrm{4 mol O} \times \textrm{16.0 g O/mol O} = 64.0 \textrm{ g O, % O} = \frac{64.0 g}{132g} \times 100 = 48.5 \textrm{% O}\)
Example: Given the atomic masses Ca 40.0, C 12.0, and O 16.0, what is the percentage composition of calcium oxalate, CaC2O4?
Answer: 31.3% Ca, 18.8% C, 50.0% O


