Metric/Imperial Conversion Errors
- Page ID
- 349
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)To learn more about systems of measurement, visit the SI Unit page and the Non-SI Unit page. As the four examples below can attest, small errors in these unit systems can harbor massive ramifications.
The Mars Climate Orbiter: A Multimillion Dollar Mistake
Although NASA declared the metric system as its official unit system in the 1980s, conversion factors remain an issue. The Mars Climate Orbiter, meant to help relay information back to Earth, is one notable example of the unit system struggle. The orbiter was part of the Mars Surveyor ’98 program, which aimed to better understand the climate of Mars. As the spacecraft journeyed into space on September 1998, it should have entered orbit at an altitude of 140-150 km above Mars, but instead went as close as 57km. This navigation error occurred because the software that controlled the rotation of the craft’s thrusters was not calibrated in SI units. The spacecraft expected newtons, while the computer, which was inadequately tested, worked in pound forces; one pound force is equal to about 4.45 newtons. Unfortunately, friction and other atmospheric forces destroyed the Mars Climate Orbiter. The project cost $327.6 million in total. Tom Gavin, an administrator for NASA's Jet Propulsion Laboratory in Pasadena, stated, "This is an end-to-end process problem. A single error like this should not have caused the loss of Climate Orbiter. Something went wrong in our system processes in checks and balances that we have that should have caught this and fixed it."
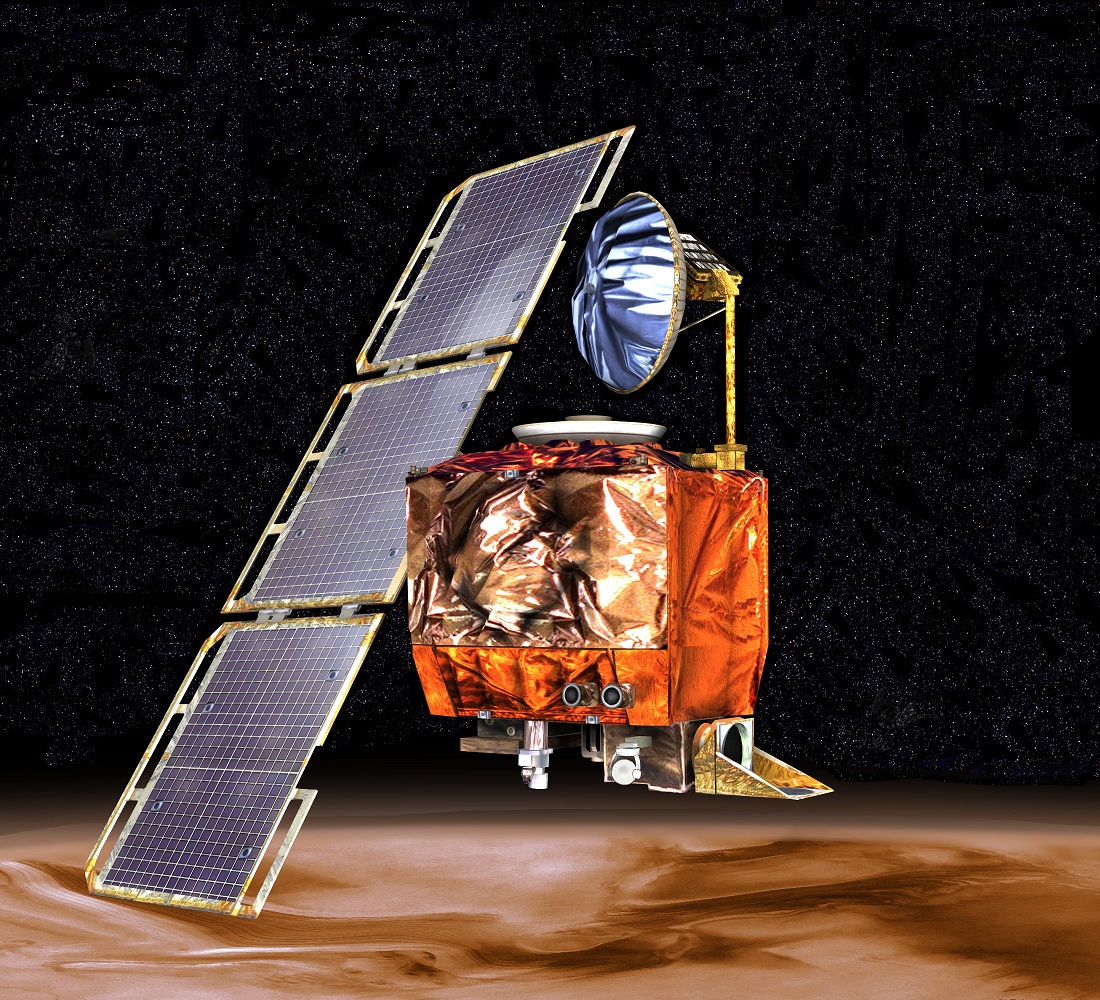
The Mars Climate Orbiter, image courtesy NASA/JPL-Caltech
NASA's Constellation Program: A Possible Casualty of Metric/Imperial Conversions
Another NASA related conversion concern involves the Constellation project, which is focused mainly on manned spaceflight. Established in 2005, it includes plans for another moon landing. The Constellation project is partially based upon decades-old projects such as the Ares rocket and the Orion crew capsule. These figures and plans are entirely in British Imperial units; converting this work into metric units would cost approximately $370 million.

Work on the Constellation Project, image courtesy NASA/Kim Shiflett
Disneyland Tokyo: A Bumpy Blunder
Tokyo Disneyland’s Space Mountain roller coaster came to a sudden halt just before the end of a ride on December 5, 2003. This startling incident was due a broken axle. The axle in question fractured because it was smaller than the design’s requirement; because of the incorrect size, the gap between the bearing and the axle was over 1 mm – when it should have been a mere 0.2 mm (the thickness of a dime vs. the thickness of two sheets of common printer paper.) The accumulation of excess vibration and stress eventually caused it to break. Though the coaster derailed, there were no injuries. Once again, unit systems caused the accident. In September 1995, the specifications for the coaster’s axles and bearings were changed to metric units. In August 2002, however, the British-Imperial-unit plans prior to 1995 were used to order 44.14 mm axels instead of the needed 45 mm axels.
Air Canada Flight 143: Unit-Caused Fuel Shortage
A Boeing 767 airplane flying for Air Canada on July 23, 1983 diminished its fuel supply only an hour into its flight. It was headed to Edmonton from Montreal, but it received low fuel pressure warnings in both fuel pumps at an altitude of 41,000 feet; engine failures followed soon after. Fortunately, the captain was an experienced glider pilot and the first officer knew of an unused air force base about 20 kilometers away. Together, they landed the plan on the runway, and only a few passengers sustained minor injuries. This incident was due partially to the airplane’s fuel indication system, which had been malfunctioning. Maintenance workers resorted to manual calculations in order to fuel the craft. They knew that 22,300 kg of fuel was needed, and they wanted to know how much in liters should be pumped. They used 1.77 as their density ratio in performing their calculations. However, 1.77 was given in pounds per liter, not kilograms per liter. The correct number should have been 0.80 kilograms/liter; thus, their final figure accounted for less than half of the necessary fuel.

The Air Canada craft, image courtesy Akradecki
If Jimmy walks 5 miles, how many kilometers did he travel?
- Solution
-
\[5 \;\cancel{miles} \times \left (\dfrac{1.6\; kilometers }{1\; \cancel{mile}}\right) = 8\; kilometers \nonumber \]
A solid rocket booster is ordered with the specification that it is to produce a total of 10 million pounds of thrust. If this number is mistaken for the thrust in Newtons, by how much, in pounds, will the thrust be in error? (1 pound = 4.5 Newtons)
- Solution
-
10,000,000 Newtons x (1 pound / 4.448 Newtons) = 2,200,000 pounds.
10,000,000 pounds - 2,200,000 pounds = 7.800,000 pounds.
The error is a missing 7,800,000 pounds of thrust.
The outer bay tank at the Monterey Bay Aquarium holds 1.3 million gallons. If NASA takes out all the fish in this tank and sends them to swim around in space, what is the theoretical volume of all the fish in liters? Assume there are 3,027,400 liters of water left in the tank after the fish are removed.
- Solution
-
\[3,027,400\; \cancel{liters} \times \left(\dfrac{0.264 \;gallons}{1\; \cancel{liter}}\right) = 800,000\;\text{gallons remaining in tank} \nonumber \]
The volume of the space fish is 1,300,000 - 800,000 = 500,000 gallons, which converts to 1,892,100 liters worth of fish swimming around the solar system.
A bolt is ordered with a thread diameter of 1.25 inches. What is this diameter in millimeters? If the order was mistaken for 1.25 centimeters, by how many millimeters would the bolt be in error?
- Solution
-
\[1.25\; \cancel{\rm{ inches}} \times \dfrac{25.4\; \rm{millimeters}}{1\; \cancel{ \rm{inch}}} = 31.75 \; \rm{millimeters} \nonumber \]
Since 1.25 centimeters x (10 millimeters / 1 centimeter) = 12.5 millimeters, the bolt would delivered 31.75 - 12.5 = 19.25 millimeters too small.
The Mars Climate Orbiter was meant to stop about 160 km away from the surface of Mars, but it ended up within 36 miles of the surface. How far off was it from its target distance (in km)? If the Orbiter is able to function as long as it stays at least 85 km away from the surface, will it still be functional despite the mistake?
- Solution
-
\[36 \; \cancel{\text{miles}} \times \dfrac {1.6 \; \text{kilometers} }{1\; \cancel{\text{mile}}} = 57.6 \;\text{km kilometers from surface} \nonumber \]
The difference then is (in kilometers): 160 - 57.6 kilometers = 102.4 kilometers away from targeted distance. Hence, the Orbiter is unable to function due to this mistake since it is beyond the 85 km error designed into its function.
References
- Nelson, Wade. “Gimli Glider.” Mobility Forum: The Journal of the Air Mobility Command’s Magazine. 10.1. (2001): 24-29.
- Lawler, Andrew. “Obama Backs Big Launcher and Bigger NASA Budget.” Science. 327.5961. (2010): 18.
- Cowen, Ron. "NASA Loses Climate Obiter." Science News. 156.14. (1999): 214.

