Cell EMF
- Page ID
- 36530
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\dsum}{\displaystyle\sum\limits} \)
\( \newcommand{\dint}{\displaystyle\int\limits} \)
\( \newcommand{\dlim}{\displaystyle\lim\limits} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)- Explain electromotive force.
- Construct the reference hydrogen electrode and explain why it is a reference.
- Distinguish reduction potentials from oxidation potentials.
- Calculate the standard potential from the reduction potentials.
Electromotive Force (EMF)
The electromotive force (EMF) is the maximum potential difference between two electrodes of a galvanic or voltaic cell. This quantity is related to the tendency for an element, a compound or an ion to acquire (i.e. gain) or release (lose) electrons. For example, the maximum potential between \(\ce{Zn}\) and \(\ce{Cu}\) of a well known cell
\(\ce{Zn_{\large{(s)}}\, |\, Zn^2+\: (1\: M)\, ||\, Cu^2+\: (1\: M)\, |\, Cu_{\large{(s)}}}\)
has been measured to be 1.100 V. A concentration of 1 M in an ideal solution is defined as the standard condition, and 1.100 V is thus the standard electromotive force, DEo, or standard cell potential for the \(\ce{Zn-Cu}\) galvanic cell.
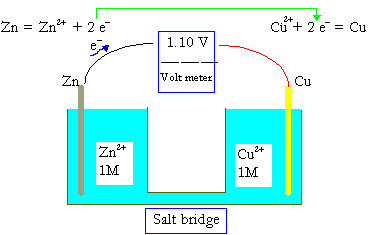
The standard cell potential, DEo, of a galvanic cell can be evaluated from the standard reduction potentials of the two half cells Eo. The reduction potentials are measured against the standard hydrogen electrode (SHE):
\(\mathrm{Pt_{\large{(s)}} \,|\, H_{2\: \large{(g,\: 1.0\: atm)}} \,|\, H^+\: (1.0\: M)}\).
Its reduction potential or oxidation potential is defined to be exactly zero.
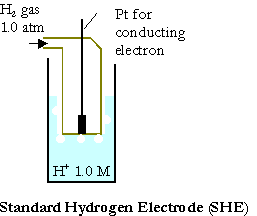
The reduction potentials of all other half-cells measured in volts against the SHE are the difference in electrical potential energy per coulomb of charge.
Note that the unit for energy J = Coulomb volt, and the Gibbs free energy G is the product of charge q and potential difference E:
G in J = q E in C V
for electric energy calculations.
Evaluating Standard Cell Potential DE° of Galvanic Cells
A galvanic cell consists of two half-cells. The convention in writing such a cell is to put the (reduction) cathode on the right-hand side, and the (oxidation) anode on the left-hand side. For example, the cell
\(\ce{Pt\, |\, H2\, |\, H+\, ||\, Zn^2+\, |\, Zn}\)
consists of the oxidation and reduction reactions:
- \(\ce{H2 \rightarrow 2 e^- + 2 H+} \hspace{15px} \textrm{anode (oxidation) reaction}\)
- \(\ce{Zn^2+ + 2 e^- \rightarrow Zn} \hspace{15px} \textrm{cathode (reduction) reaction}\)
If the concentrations of \(\ce{H+}\) and \(\ce{Zn^2+}\) ions are 1.0 M and the pressure of \(\ce{H2}\) is 1.0 atm, the voltage difference between the two electrodes would be -0.763 V(the \(\ce{Zn}\) electrode being the negative electrode). The conditions specified above are called the standard conditions and the EMF so obtained is the standard reduction potential.
Note that the above cell is in reverse order compared to that given in many textbooks, but this arrangement gives the standard reduction potentialsdirectly, because the \(\ce{Zn}\) half cell is a reduction half-cell. The negative voltage indicates that the reverse chemical reaction is spontaneous. This corresponds to the fact that \(\ce{Zn}\) metal reacts with an acid to produce \(\ce{H2}\) gas.
As another example, the cell
\(\ce{Pt\, |\, H2\, |\, H+\, ||\, Cu+\, |\, Cu}\)
consists of an oxidation and a reduction reaction:
- \(\ce{H2 \rightarrow 2 e^- + 2 H+} \hspace{15px} \textrm{anode reaction}\)
- \(\ce{Cu^2+ + 2 e^- \rightarrow Cu} \hspace{15px} \textrm{cathode reaction}\)
and the standard cell potential is 0.337 V. The positive potential indicates a spontaneous reaction,
\(\ce{Cu^2+ + H2 \rightarrow Cu + 2 H+}\)
but the potential is so small that the reaction is too slow to be observed.
What is the potential for the following cell?
\(\mathrm{Zn\, |\, Zn^{2+}\:(1.0\: M)\, ||\, Cu^{2+}\:(1.0\: M)\, |\, Cu}\)
Solution
From a table of standard reduction potentials we have the following values
\(\ce{Cu^2+ + 2 e^- \rightarrow Cu} \hspace{15px} E^\circ = 0.337 \tag{1}\)
\(\ce{Zn \rightarrow Zn^2+ + 2 e^-} \hspace{15px} E^* = 0.763 \tag{2}\)
Add (1) and (2) to yield
\(\ce{Zn + Cu^2+ \rightarrow Zn^2+ + Cu} \hspace{15px} \ce D E^\circ = E^\circ + E^* = \textrm{1.100 V}\)
Note that E* is the oxidation standard potential, and E° is the reduction standard potential, E* = - E°. The standard cell potential is represented by dE°.
DISCUSSION
The positive potential confirms your observation that zinc metal reacts with cupric ions in solution to produce copper metal.
What is the potential for the following cell?
\(\mathrm{Ag\, |\, Ag^+\:(1.0\: M)\, ||\, Li^+\:(1.0\: M)\, |\, Li}\)
Solution
From the table of standard reduction potentials, you find
\(\ce{Li+ + e^- \rightarrow Li} \hspace{15px} E^\circ = -3.045 \tag{3}\)
\(\ce{Ag \rightarrow Ag+ + e^-}\hspace{15px} E^* = -0.799 \tag{4}\)
According to the convention of the cell, the reduction reaction is on the right. The cell on your left-hand side is an oxidation process. Thus, you add (4) and (3) to obtain
\(\ce{Li+ + Ag \rightarrow Ag+ + Li} \hspace{15px} \ce d E^\circ = \textrm{-3.844 V}\)
DISCUSSION
The negative potential indicates that the reverse reaction should be spontaneous.
Some calculators use a lithium battery. The atomic weight of \(\ce{Li}\) is 6.94, much lighter than \(\ce{Zn}\) (65.4).
Summary
- The electromotive force (EMF) is the maximum potential difference between two electrodes of a galvanic or voltaic cell.
- The standard reduction potential of \(M^{\ce n+},\: 1\: \ce M \,|\, M\) couple is the standard cell potential of the galvanic cell:
\(\mathrm{Pt\, |\, H_2,\: 1\: atm\, |\, H^+,\: 1\: M\, ||\, \mathit M^{n+},\: 1\: M\, |\, \mathit M}\)
- The standard oxidation potential of \(M \,|\, M^{\ce n+},\: 1\: \ce M\) couple is the standard cell potential of the galvanic cell:
\(\mathrm{\mathit M\, |\, \mathit M^{n+},\: 1\: M\, ||\, H^+,\: 1\: M\, |\, H_2,\: 1\: atm\, |\, Pt}\)
- If the cell potential is negative, the reaction is reversed. In this case, the electrode of the galvanic cell should be written in a reversed order.
Questions
- \(\ce{|\, left\, |\, left^+\, ||\, right^+\, |\, right\, |}\)?
- Reduction potentials of half cells are measured against what?
- The zinc half cell \(\ce{Zn \,|\, Zn^2+\: 1\: M}\).
- The hydrogen half cell \(\ce{Pt \,|\, H2 \,|\, H+\: 1\: M}\).
- The hydrogen half cell \(\ce{H+\: 1\: M \,|\, H2 \,|\, Pt}\).
- The copper half cell \(\ce{Cu^2+\: 1\: M \,|\, Cu}\).
- The hydrogen half cell \(\ce{Pt \,|\, H2 \,|\, H+\: 10^{-7}\: M}\).
- \(\ce{Pt \,|\, H2 \,|\, H+ \,||\, Cl2 \,|\, Cl- \,|\, Pt}\) positive or negative?
Solutions
- Answer... Right
Consider...
Oxidation takes place in the left hand cell.
Reduction takes place in the right hand cell or cathode. - Answer... B.
Consider...\(\ce{Pt \,|\, H2 \,|\, H+\: 1\: M \,||\, right^+ \,|\, right}\)
gives the reduction potential. - Answer... Positive
Consider...\(\begin{align}
The reaction is spontaneous.
\ce{Cl2 + 2 e^- \rightarrow 2 Cl-} &\hspace{15px}E^\circ = 1.36\\
\mathrm{H_2 \rightarrow 2 H^+ + 2 e^-} &\hspace{15px }E^\circ = 0.00\\
\overline{\hspace{140px}}&\overline{\hspace{100px}}\\
\ce{Cl2 + H2 \rightarrow 2 HCl} \hspace{15px} &\hspace{15px}\ce DE^\circ = 1.36\: \ce V
\end{align}\)
Contributors and Attributions
Chung (Peter) Chieh (Professor Emeritus, Chemistry @ University of Waterloo)

