Error and Propagation of Error
- Page ID
- 279968
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Activity 1
Learning Objectives:
Following this activity, students will be able to…
- Differentiate between accuracy and precision.
- Describe two main types of error in chemical analysis.
- Express experimental error as either absolute or relative uncertainty.
- Use the real rule of significant figures when expressing values that have an associated uncertainty.
- If the bull’s eyes below represent obtaining an accurate result in a chemical analysis, mark each of the diagrams below with markings that represent the accuracy and precision of repeated trials described.
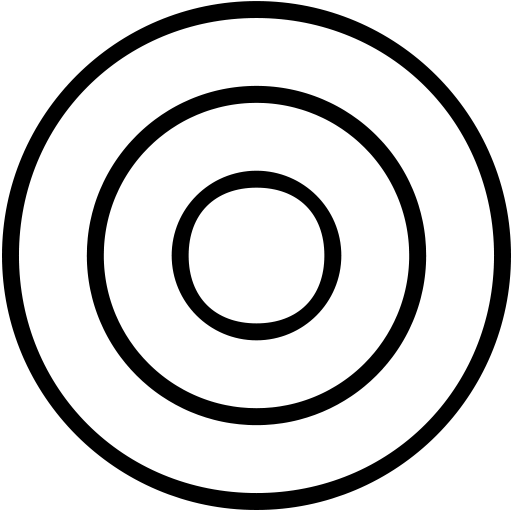
Not precise
Not accurate

Precise
Not accurate

Not precise
Accurate

Precise
Accurate
- Two main types of experimental error exist in chemical analysis: systematic and random. Provided a definition of each.
- Systematic error –
- Random error –
- Systematic error –
- Which of the two types of error can be corrected? Think of a real-world example of this type of error.
- Although it cannot be completely eliminated, the effect of the other type of error on the quality of data can be minimized by doing one simple (though sometimes time-consuming) thing. What is it? Why would this not work to eliminate the other type of error?
- The graph below shows an analytical chemistry lab’s results for their analysis of calcium. What type(s) of error is/are present? Explain your response.

- Error can be expressed as absolute uncertainty or relative uncertainty. Absolute uncertainty has the same units as the measured quantity (e.g., 5.00±0.03 mL). Relative uncertainty is usually represented as a percent relative to the measured value. Complete the table below.
|
Measurement magnitude |
Absolute uncertainty |
% Relative uncertainty |
|---|---|---|
|
5.00 mL |
0.03 mL |
|
|
15.2 ppm |
|
3% |
|
|
3 mM |
6% |
- Let’s talk about significant figures. Students always hate “sig figs,” but they are important, especially in analytical chemistry. Consider the results reported by two students who worked together to determine the caffeine content in soda.
- Student A: “One can of soda contains 46 mg caffeine.”
- Student B: “One can of soda contains 46.02508 mg caffeine.”
Which student are you more likely to believe?
- When we have an uncertainty associated with a measured value, we have a little bit more information to go on and can use the “real rule” of sig figs. In your own words, state the real rule of sig figs below and use it to appropriately round the values below.
Real Rule of Sig Figs:
|
Value |
Uncertainty |
Rounded (include uncertainty) |
|---|---|---|
|
235.064789 µM |
3.4902390 µM |
|
|
93.894290 mg |
0.037892 mg |
|
|
2.57892 ×10-5 |
1.23648 ×10-6 M |
|
Activity 2
Learning Objectives:
Following this activity, students will be able to…
- Propagate error through mathematical operations.
- Addition/subtraction
- Multiplication/division
- Logarithms (i.e., pH calculations)
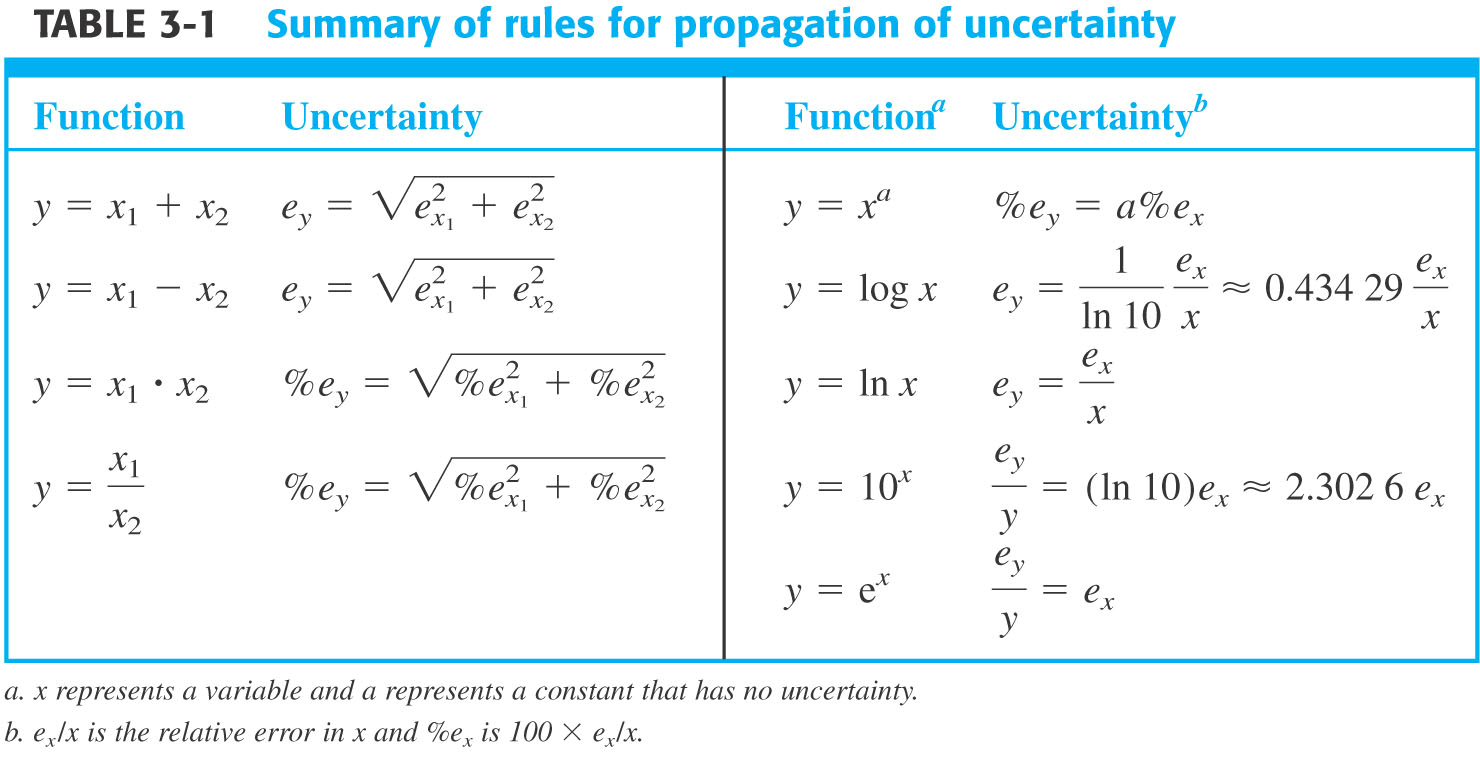
Table from Harris textbook
- Apply the propagation of uncertainty in the context of laboratory work.
- Complete the following mathematical operations and report the following answers with the proper number of significant digits.
- 4.32(±0.03) + 8.24(±0.06) =
- 154.2(±0.3) × 3.05(±0.01) =
- log [1.56(±0.03)×105] =
- 4.32(±0.03) + 8.24(±0.06) =
- You need to pipette 30 mL in lab as accurately as possible, but you only have a Class B 10-mL pipette and a Class A 5-mL volumetric pipette. Is it more accurate to use the Class B pipette three times or the Class A pipette six times? The Class B pipette has a tolerance of 0.04 mL and the Class A pipette has a tolerance of 0.01 mL.
- A diluted solution of nitric acid is prepared by pipetting 1.50(±0.1) mL of 14.8±0.1 M HNO3 into a 250.0(±0.1)–mL volumetric flask. Calculate the concentration of the diluted solution with the proper significant figures and absolute uncertainty.
- An acid is found to have [H+] = 1.56(±0.03)×10-2 M. What is the pH of the solution with absolute uncertainty? Note: when values are represented as given above, the scientific notation is understood to apply to both the number and its uncertainty so it is the same as 0.0156(±0.0003).
- A solution has pH = 9.37(±0.05). What is [H+] with absolute uncertainty?
Contributors and Attributions
- Dr. Kate Mullaugh, College of Charleston (mullaughkm@cofc.edu)
- Sourced from the Analytical Sciences Digital Library


