28.7: Size-Exclusion Chromatography
- Page ID
- 81129
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Steric exclusion chromatography is a technique that separates compounds solely on the basis of size. In order for the results of steric exclusion separations to be meaningful, there can be no directed forces between the compounds being separated and the surface of the particles used as the stationary phase. Instead, the particles are prepared with well-characterized pore sizes. Figure 50 shows a particle with one pore in it. Figure 50 also shows representations for several molecules of different size (note, the pore size and molecule size are not representative of the scale that would exist in real steric exclusion phases – the pore is bigger than would actually occur and the molecules would never have sizes on the order of that of the particle).
Figure 50. Representation of a particle with a pore.
The smallest molecule is small enough to fit entirely into all regions of the pore. The largest molecule is too big to fit into the pore. The intermediate sized one can only sample some of the pore volume. Provided these molecules have no interaction with the surface of the particle and are only separated on the basis of how much of the pore volume they can sample, the largest molecule would elute first from the column and the smallest one last.
Suppose we took a molecule that was even larger than the biggest one in the picture above. Note that it would not fit into any of the pores either, and would elute with the biggest in the picture. If we wanted to separate these large species, we would need a particle with even larger pores. Similarly, if we took a smaller molecule than the smallest pictured above, it would sample all of the pore volume and elute with the smallest one pictured above. To separate these two compounds, we would need particles with smaller pores.
Steric exclusion chromatography requires large compounds, and is not generally effective on things with molecular weights of less than 1000. It is commonly used in biochemistry for the separation of proteins and nucleic acids. It is also used for separation or characterization purposes in polymer chemistry (a polymer is a large compound prepared from repeating monomeric units – polyethylene is a long, linear polymer made of repeating ethylene units).
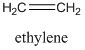
One critical question is how to remove the possibility of an attractive force between the compounds being separated and the surface of the porous particles. The way this is done is to use a particle with very different properties than the compound being separated, and to use a solvent that the solutes are very soluble in. For example, if we want to separate proteins or nucleic acids, which are polar and very soluble in water, we would need to use porous particles made from an organic material that had a relatively non-polar surface. If we wanted to separate polyethylenes of different chain lengths, which are non-polar, we would dissolve the polyethylene in a non-polar organic solvent and use porous particles made from a material that had a highly polar surface. This might involve the use of a polydextran (carbohydrate), which has hydroxyl groups on the surface of the pores.
The classic organic polymer that has been used to prepare porous particles for the steric exclusion separation of water-soluble polymers is a co-polymer of styrene and divinylbenzene.
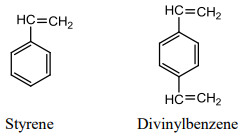
If we just had styrene, and conducted a polymerization, we would get a long, single-bonded chain of carbon atoms with phenyl rings attached to it. Linear polystyrenes are soluble in certain non-polar organic solvents. The divinylbenzene acts as a cross-linking agent that bridges individual styrene chains (Figure 51). A typical ratio of styrene to divinylbenzene is 12:1. The cross-linking serves to make the polymer into particles rather than linear chains. The cross-linked polymer is an insoluble material with a very high molecular weight. By controlling the reaction conditions including the amount of cross-linker and rate of reaction, it is possible to make polystyrenes with a range of pore sizes.
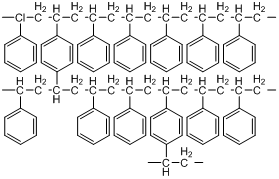
Figure 51. Cross-linked styrene-divinylbenzene copolymer.
If we think about what is inside the column, there are four important volume terms to consider. One is the total volume of the column (VT). The second is the interstitial volume between the particles (VI). The third is the volume of all of the pores (VP). The last one is the actual volume occupied by the mass of the material that makes up the particles.
It turns out that VI is usually about 0.3 of VT for a column. There is simply no way to crunch the particles closer together to reduce this term appreciably.
\[V_I = 0.3 V_T\]
It turns out that the maximum pore volume that can be achieved is about 0.4 of VT. If you make this larger, the particles have more pores than structure, become excessively fragile, and get crushed as you try to push a liquid mobile phase through the column.
\[V_P = 0.4 V_T\]
Totaling this up, we can write that:
\[V_I + VP = 0.7 V_T\]
Since there are no directed forces in a steric exclusion column, this means that all of the separation must happen in one column volume, or in 0.7 VT. If we then showed a chromatogram for a series of compounds being separated on a steric exclusion, it might look like that shown in Figure 52.
Figure 52. Representation of a chromatogram on a steric exclusion column.
The peak labeled 1, which has the shortest retention time (0.3 VT), corresponds to all molecules in the sample that had a size that was too large to fit into the pores. It cannot be known whether this is one compound or a mixture of large compounds. The peak labeled 5, which has the longest retention time (0.7 VT), corresponds to all molecules in the sample that had a size small enough to sample all of the pore volume. It cannot be known whether it is one compound or a mixture of two or more small compounds. The peaks labeled 2 through 4 sample some of the pore volume depending on their size. The peak labeled 6 elutes with a retention volume that is greater than 0.7 VT. How would we explain the elution volume of this peak? The only way we can do so is if there are attractive forces between this molecule and the surface of the particles. This is a serious problem and we would need to examine this system and eliminate the cause of this attractive force.
Note that this method separates things on the basis of their size. What people try to do when using steric exclusion chromatography is equate size with molecular weight. The two representations for the shape of a molecule shown in Figure 53 point out a potential problem with equating size with molecular weight. One molecule is spherical. The other is rod shaped. The spherical molecule probably has a larger molecular weight, because it does occupy more volume. However, the size of a molecule is determined by what is known as its hydrodynamic radius. This is the volume that the molecule sweeps out in space as it tumbles. If you took the rod shaped molecule below and allowed it to tumble, you would see that its size is essentially comparable to that of the spherical molecule.
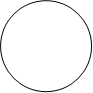
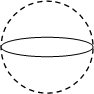
Figure 53. Representation of the molecular size of spherical (left) and rod-shaped (right) molecules.
When performing steric exclusion chromatography, you need to use a series of molecular weight standards. It is essential that these standards approximate the molecular features and shapes of the molecules you are analyzing. If you are analyzing proteins, you need to use proteins as standards. The same would apply to nucleic acids, or to particular organic polymers. Figure 54 shows the typical outcome of the calibration of elution time with molecular weight (always plotted on a log scale) for a steric exclusion column.
Figure 54. Generalized calibration curve for a steric exclusion column.
Note that excessively large molecules never enter the pores and elute together at 0.3 VT. Excessively small molecules sample all of the pore volume and elute at 0.7 VT. There is then some range of size (or molecular weight) where the compounds sample some of the pore volume and elute at volumes between 0.3 and 0.7 VT.
The plots that would occur for particles with the next larger (dashed line) and smaller pore sizes (dotted line) are shown in Figure 55.
Figure 55. Calibration curves for a steric exclusion column. The dashed line is the column with the largest pore sizes. The dotted line is the column with the smallest pore sizes.

