2.1: Measurements in Analytical Chemistry
- Page ID
- 5769
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Analytical chemistry is a quantitative science. Whether determining the concentration of a species, evaluating an equilibrium constant, measuring a reaction rate, or drawing a correlation between a compound’s structure and its reactivity, analytical chemists engage in “measuring important chemical things.”1 In this section we briefly review the use of units and significant figures in analytical chemistry.
Units of Measurement
A measurement usually consists of a unit and a number expressing the quantity of that unit. We may express the same physical measurement with different units, which can create confusion. For example, the mass of a sample weighing 1.5 g also may be written as 0.0033 lb or 0.053 oz. To ensure consistency, and to avoid problems, scientists use a common set of fundamental units, several of which are listed in Table \(\PageIndex{1}\). These units are called SI units after the Système International d’Unités.
It is important for scientists to agree upon a common set of units. In 1999 NASA lost a Mar’s Orbiter spacecraft because one engineering team used English units and another engineering team used metric units. As a result, the spacecraft came too close to the planet’s surface, causing its propulsion system to overheat and fail.
Some measurements, such as absorbance, do not have units. Because the meaning of a unitless number may be unclear, some authors include an artificial unit. It is not unusual to see the abbreviation AU, which is short for absorbance unit, following an absorbance value. Including the AU clarifies that the measurement is an absorbance value.
We define other measurements using these fundamental SI units. For example, we measure the quantity of heat produced during a chemical reaction in joules, (J), where
\[\mathrm{1\: J = 1\, \dfrac{m^2kg}{s^2}}\]
Table \(\PageIndex{2}\) provides a list of some important derived SI units, as well as a few common non-SI units.
| Measurement | Unit | Symbol | Definition (1 unit is...) |
|---|---|---|---|
| mass | kilogram | kg | ...the mass of the international prototype, a Pt-Ir object housed at the Bureau International de Poids and Measures at Sèvres, France.† |
| distance | meter | m | ...the distance light travels in (299 792 458)-1 seconds. |
| temperature | Kelvin | K | ...equal to (273.16)–1, where 273.16 K is the triple point of water (where its solid, liquid, and gaseous forms are in equilibrium). |
| time | second | s | ...the time it takes for 9 192 631 770 periods of radiation corresponding to a specific transition of the 133Cs atom. |
| current | ampere | A | ...the current producing a force of 2 × 10-7 N/m when maintained in two straight parallel conductors of infinite length separated by one meter (in a vacuum). |
| amount of substance | mole | mol | ...the amount of a substance containing as many particles as there are atoms in exactly 0.012 kilogram of 12C. |
† The mass of the international prototype changes at a rate of approximately 1 mg per year due to reversible surface contamination. The reference mass, therefore, is determined immediately after its cleaning by a specified procedure.
| Measurement | Unit | Symbol | Equivalent SI Units |
|---|---|---|---|
| length | angstrom (non-SI) | Å | 1 Å = 1 × 10–10 m |
| volume | liter (non-SI) | L | 1 L = 10–3 m3 |
| force | newton (SI) | N | 1 N = 1 m·kg/s2 |
| pressure | pascal (SI) | Pa | 1 Pa = 1 N/m2 = 1 kg/(m·s2) |
| atmosphere (non-SI) | atm | 1 atm = 101,325 Pa | |
| energy, work, heat | joule (SI) | J | 1 J = N·m = 1 m2·kg/s2 |
| calorie (non-SI) | cal | 1 cal = 4.184 J | |
| electron volt (non-SI) | eV | 1 eV = 1.602 177 33 × 10–19 J | |
| power | watt (SI) | W | 1 W = 1 J/s = 1 m2·kg/s3 |
| charge | coulomb (SI) | C | 1 C = 1 A·s |
| potential | volt (SI) | V | 1 V = 1 W/A = 1 m2·kg/(s3·A) |
| frequency | hertz (SI) | Hz | 1 Hz = s–1 |
| temperature | Celsius (non-SI) | oC | oC = K – 273.15 |
Chemists frequently work with measurements that are very large or very small. A mole contains 602 213 670 000 000 000 000 000 particles and some analytical techniques can detect as little as 0.000 000 000 000 001 g of a compound. For simplicity, we express these measurements using scientific notation; thus, a mole contains 6.022 136 7 × 1023 particles, and the detected mass is 1 × 10–15 g. Sometimes it is preferable to express measurements without the exponential term, replacing it with a prefix (Table 2.3). A mass of 1×10–15 g, for example, is the same as 1 fg, or femtogram.
Writing a lengthy number with spaces instead of commas may strike you as unusual. For numbers containing more than four digits on either side of the decimal point, however, the currently accepted practice is to use a thin space instead of a comma.
| Prefix | Symbol | Factor | Prefix | Symbol | Factor | Prefix | Symbol | Factor |
|---|---|---|---|---|---|---|---|---|
| yotta | Y | 1024 | kilo | k | 103 | micro | μ | 10–6 |
| zetta | Z | 1021 | hecto | h | 102 | nano | n | 10–9 |
| eta | E | 1018 | deka | da | 101 | pico | p | 10-12 |
| peta | P | 1015 | - | - | 100 | femto | f | 10–15 |
| tera | T | 1012 | deci | d | 10–1 | atto | a | 10–18 |
| giga | G | 109 | centi | c | 10–2 | zepto | z | 10–21 |
| mega | M | 106 | milli | m | 10–3 | yocto | y | 10–24 |
Uncertainty in Measurements
A measurement provides information about its magnitude and its uncertainty. Consider, for example, the balance in Figure \(\PageIndex{1}\), which is recording the mass of a cylinder. Assuming that the balance is properly calibrated, we can be certain that the cylinder’s mass is more than 1.263 g and less than 1.264 g. We are uncertain, however, about the cylinder’s mass in the last decimal place since its value fluctuates between 6, 7, and 8. The best we can do is to report the cylinder’s mass as 1.2637 g ± 0.0001 g, indicating both its magnitude and its absolute uncertainty.
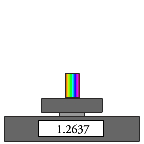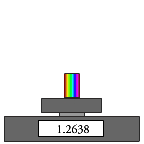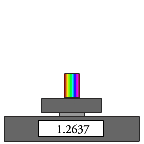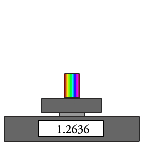
Figure \(\PageIndex{1}\): When weighing an object on balance, the measurement fluctuates in the final decimal place. We record this cylinder’s mass as 1.2637 g ± 0.0001 g.
Significant Figures
Significant figures are a reflection of a measurement’s magnitude and uncertainty. The number of significant figures in a measurement is the number of digits known exactly plus one digit whose value is uncertain. The mass shown in Figure \(\PageIndex{1}\), for example, has five significant figures, four which we know exactly and one, the last, which is uncertain.
Suppose we weigh a second cylinder, using the same balance, obtaining a mass of 0.0990 g. Does this measurement have 3, 4, or 5 significant figures? The zero in the last decimal place is the one uncertain digit and is significant. The other two zero, however, serve to show us the decimal point’s location. Writing the measurement in scientific notation (9.90 × 10–2) clarifies that there are but three significant figures in 0.0990.
In the measurement 0.0990 g, the zero in green is a significant digit and the zeros in red are not significant digits.
Example \(\PageIndex{1}\)
How many significant figures are in each of the following measurements? Convert each measurement to its equivalent scientific notation or decimal form.
- 0.0120 mol HCl
- 605.3 mg CaCO3
- 1.043 × 10–4 mol Ag+
- 9.3 × 104 mg NaOH
Solution
- Three significant figures; 1.20 × 10–2 mol HCl
- Four significant figures; 6.053 × 102 mg CaCO3
- Four significant figures; 0.000 104 3 mol Ag+
- Two significant figures; 93 000 mg NaOH
There are two special cases when determining the number of significant figures. For a measurement given as a logarithm, such as pH, the number of significant figures is equal to the number of digits to the right of the decimal point. Digits to the left of the decimal point are not significant figures since they only indicate the power of 10. A pH of 2.45, therefore, contains two significant figures.
The log of 2.8 × 102 is 2.45. The log of 2.8 is 0.45 and the log of 102 is 2. The 2 in 2.45, therefore, only indicates the power of 10 and is not a significant digit.
An exact number has an infinite number of significant figures. Stoichiometric coefficients are one example of an exact number. A mole of CaCl2, for example, contains exactly two moles of chloride and one mole of calcium. Another example of an exact number is the relationship between some units. There are, for example, exactly 1000 mL in 1 L. Both the 1 and the 1000 have an infinite number of significant figures.
Using the correct number of significant figures is important because it tells other scientists about the uncertainty of your measurements. Suppose you weigh a sample on a balance that measures mass to the nearest ±0.1 mg. Reporting the sample’s mass as 1.762 g instead of 1.7623 g is incorrect because it does not properly convey the measurement’s uncertainty. Reporting the sample’s mass as 1.76231 g also is incorrect because it falsely suggest an uncertainty of ±0.01 mg.
Significant Figures in Calculations
Significant figures are also important because they guide us when reporting the result of an analysis. In calculating a result, the answer can never be more certain than the least certain measurement in the analysis. Rounding answers to the correct number of significant figures is important.
For addition and subtraction round the answer to the last decimal place that is significant for each measurement in the calculation. The exact sum of 135.621, 97.33, and 21.2163 is 254.1673. The last common decimal place shared by each is shown in red. Since the last digit that is significant for all three numbers is in the hundredth’s place
\[\begin{align}
&135.6{\color{Red} 2}1\\
&\phantom{1}97.3{\color{Red} 3}\\
&\underline{\phantom{1}21.2{\color{Red} 1}63}\\
&254.1673
\end{align}\]
we round the result to 254.17. When working with scientific notation, convert each measurement to a common exponent before determining the number of significant figures. For example, the sum of 4.3 × 105, 6.17 × 107, and 3.23 × 104 is 622 × 105, or 6.22 × 107. The last common decimal place shared by each is shown in red.
\[\begin{align}
61{\color{Red} 7}\phantom{.323}\times 10^5\\
{\color{Red} 4}.3\phantom{23}\times 10^5\\
\underline{\phantom{62}{\color{Red} 0}.323\times 10^5}\\
621.623 \times 10^5
\end{align}\]
For multiplication and division round the answer to the same number of significant figures as the measurement with the fewest significant figures. For example, dividing the product of 22.91 and 0.152 by 16.302 gives an answer of 0.214 because 0.152 has the fewest significant figures.
\[\dfrac{22.91 \times 0.{\color{Red} 152}}{16.302} = 0.2131 = 0.214\]
There is no need to convert measurements in scientific notation to a common exponent when multiplying or dividing.
Finally, to avoid “round-off” errors it is a good idea to retain at least one extra significant figure throughout any calculation. Better yet, invest in a good scientific calculator that allows you to perform lengthy calculations without recording intermediate values. When your calculation is complete, round the answer to the correct number of significant figures using the following simple rules.
- Retain the least significant figure if it and the digits that follow are less than half way to the next higher digit. For example, rounding 12.442 to the nearest tenth gives 12.4 since 0.442 is less than half way between 0.400 and 0.500.
- Increase the least significant figure by 1 if it and the digits that follow are more than half way to the next higher digit. For example, rounding 12.476 to the nearest tenth gives 12.5 since 0.476 is more than half way between 0.400 and 0.500.
- If the least significant figure and the digits that follow are exactly halfway to the next higher digit, then round the least significant figure to the nearest even number. For example, rounding 12.450 to the nearest tenth gives 12.4, while rounding 12.550 to the nearest tenth gives 12.6. Rounding in this manner ensures that we round up as often as we round down.
It is important to recognize that the rules for working with significant figures are generalizations. What is conserved in a calculation is uncertainty, not the number of significant figures. For example, the following calculation is correct even though it violates the general rules outlined earlier.
\[\dfrac{101}{99} = 1.02\]
Since the relative uncertainty in each measurement is approximately 1% (101 ± 1, 99 ± 1), the relative uncertainty in the final answer also must be approximately 1%. Reporting the answer as 1.0 (two significant figures), as required by the general rules, implies a relative uncertainty of 10%, which is too large. The correct answer, with three significant figures, yields the expected relative uncertainty. Chapter 4 presents a more thorough treatment of uncertainty and its importance in reporting the results of an analysis.
Exercise \(\PageIndex{1}\)
For a problem involving both addition and/or subtraction, and multiplication and/or division, be sure to account for significant figures at each step of the calculation. With this in mind, to the correct number of significant figures, what is the result of this calculation?
\[\dfrac{0.250 \times (9.93\times10^{-3}) - 0.100 \times (1.927\times10^{-2})}{ 9.93\times10^{-3} + 1.927\times10^{-2}} =\]
Click here to review your answer to this exercise.


