2.6: Intermolecular Interactions

 Learning Objectives

• Define phase.
• Identify the types of interactions between molecules.

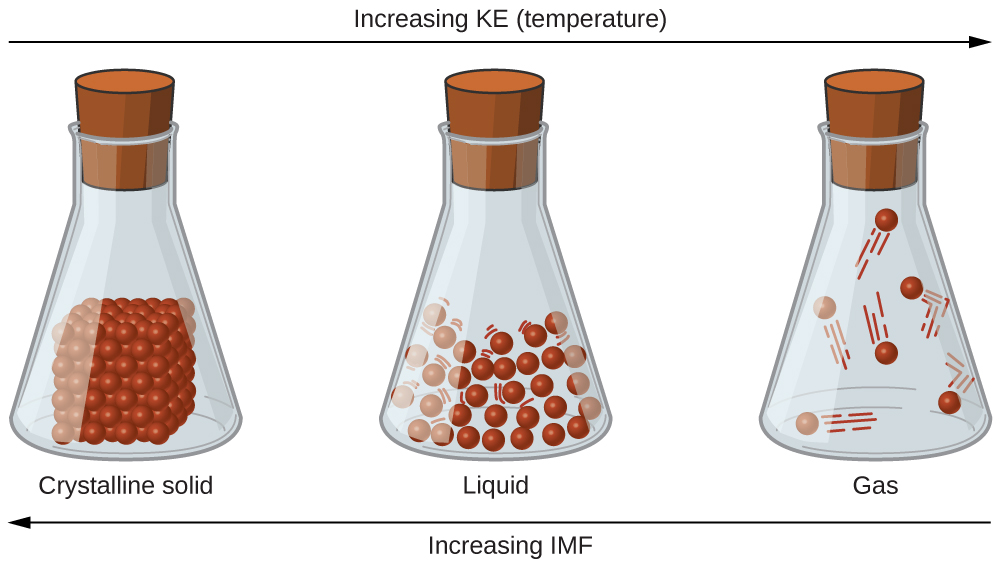
A phase is a certain form of matter that includes a specific set of physical properties. That is, the atoms, the molecules, or the ions that make up the phase do so in a consistent manner throughout the phase. Science recognizes three stable phases: the solid phase, in which individual particles can be thought of as in contact and held in place; the liquid phase, in which individual particles are in contact but moving with respect to each other; and the gas phase, in which individual particles are separated from each other by relatively large distances (see Figure 8.1.1). Not all substances will readily exhibit all phases. For example, carbon dioxide does not exhibit a liquid phase unless the pressure is greater than about six times normal atmospheric pressure. Other substances, especially complex organic molecules, may decompose at higher temperatures, rather than becoming a liquid or a gas.

For many substances, there are different arrangements the particles can take in the solid phase, depending on temperature and pressure.

Which phase a substance adopts depends on the pressure and the temperature it experiences. Of these two conditions, temperature variations are more obviously related to the phase of a substance. When it is very cold, H₂O exists in the solid form as ice. When it is warmer, the liquid phase of H₂O is present. At even higher temperatures, H₂O boils and becomes steam.

Pressure changes can also affect the presence of a particular phase (as we indicated for carbon dioxide), but its effects are less obvious most of the time. We will mostly focus on the temperature effects on phases, mentioning pressure effects only when they are important. Most chemical substances follow the same pattern of phases when going from a low temperature to a high temperature: the solid phase, then the liquid phase, and then the gas phase. However, the temperatures at which these phases are present differ for all substances and can be rather extreme. Table \(\PageIndex{1}\) shows the temperature ranges for solid, liquid, and gas phases for three substances. As you can see, there is extreme variability in the temperature ranges.

Table \(\PageIndex{1}\): Temperature Ranges for the Three Phases of Various Substances

Substance	Solid Phase Below	Liquid Phase Above	Gas Phase Above
hydrogen (H2)	−259°C	−259°C	−253°C
water (H2O)	0°C	0°C	100°C
sodium chloride (NaCl)	801°C	801°C	1413°C
The melting point of a substance is the temperature that separates a solid and a liquid. The boiling point of a substance is the temperature that separates a liquid and a gas.

What accounts for this variability? Why do some substances become liquids at very low temperatures, while others require very high temperatures before they become liquids? It all depends on the strength of the intermolecular forces (IMF) between the particles of substances and the kinetic energies (KE) of its molecules. (Although ionic compounds are not composed of discrete molecules, we will still use the term intermolecular to include interactions between the ions in such compounds.) Substances that experience strong intermolecular interactions require higher temperatures to become liquids and, finally, gases. Substances that experience weak intermolecular interactions do not need much energy (as measured by temperature) to become liquids and gases and will exhibit these phases at lower temperatures.

Figure \(\PageIndex{1}\): Transitions between solid, liquid, and gaseous states of a substance occur when conditions of temperature or pressure favor the associated changes in intermolecular forces. (Note: The space between particles in the gas phase is much greater than shown.)

Three sealed flasks are labeled, “Crystalline solid,” “Liquid,” and “Gas,” from left to right. The first flask holds a cube composed of small spheres sitting on the bottom while the second flask shows a lot of small spheres in the bottom that are spaced a small distance apart from one another and have lines around them to indicate motion. The third flask shows a few spheres spread far from one another with larger lines to indicate motion. There is a right-facing arrow that spans the top of all three flasks. The arrow is labeled, “Increasing K E ( temperature ).” There is a left-facing arrow that spans the bottom of all three flasks. The arrow is labeled, “Increasing I M F.”

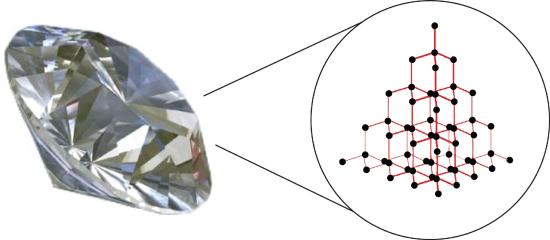
Covalent Network Materials

Substances with the highest melting and boiling points have covalent network bonding. This type of intermolecular interaction is actually a covalent bond. In these substances, all the atoms in a sample are covalently bonded to one another; in effect, the entire sample is essentially one giant molecule. Many of these substances are solid over a large temperature range because it takes a lot of energy to disrupt all the covalent bonds at once. One example of a substance that shows covalent network bonding is diamond (Figure \(\PageIndex{2}\)). Diamond is composed entirely of carbon atoms, each bonded to four other carbon atoms in a tetrahedral geometry. Melting a covalent network solid is not accomplished by overcoming the relatively weak intermolecular forces. Rather, all of the covalent bonds must be broken, a process that requires extremely high temperatures. Diamond, in fact, does not melt at all. Instead, it vaporizes to a gas at temperatures above 3,500°C. Diamond is extremely hard and is one of the few materials that can cut glass.

Figure \(\PageIndex{2}\): Diamond. Diamond, a form of pure carbon, has covalent network bonding. It takes a very high temperature—over 3,500°C—for diamond to leave the solid state. Source: Photo © Thinkstock

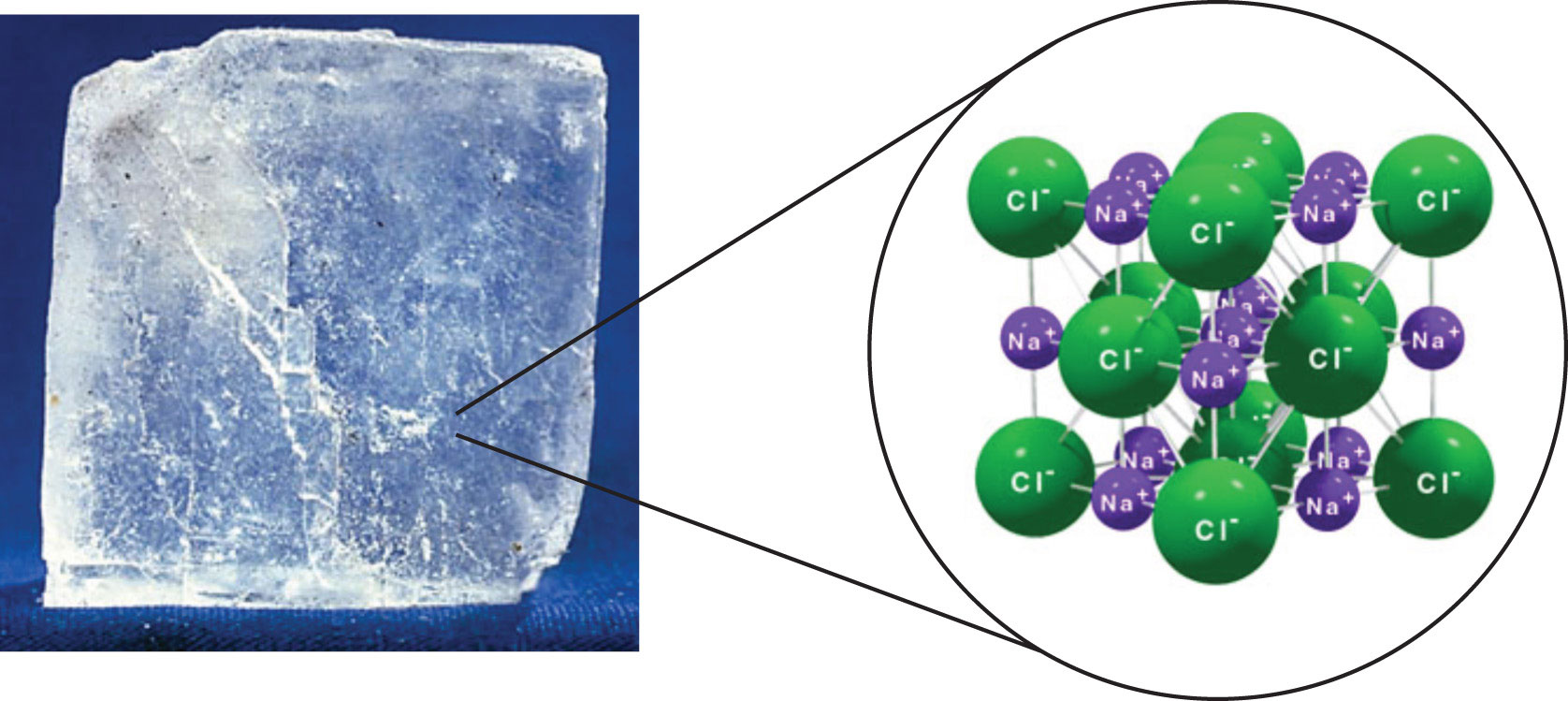
Ionic Compounds

The strongest force between any two particles is the ionic bond, in which two ions of opposing charge are attracted to each other. Thus, ionic interactions between particles are another type of intermolecular interaction. Substances that contain ionic interactions are relatively strongly held together, so these substances typically have high melting and boiling points. Sodium chloride (Figure \(\PageIndex{3}\)) is an example of a substance whose particles experience ionic interactions (Table \(\PageIndex{1}\)). These attractive forces are sometimes referred to as ion-ion interactions.

Figure \(\PageIndex{3}\): Sodium Chloride. Solid NaCl is held together by ionic interactions. Source: Photo © Thinkstock

Covalent Molecular Compounds

There are two different covalent structures: molecular and network. Covalent network compounds like SiO₂ (quartz) have structures of atoms in a network like diamond described earlier. In this section, we are dealing with the molecular type that contains individual molecules. The bonding between atoms in the individual molecule is covalent but the attractive forces between the molecules are called intermolecular forces (IMF).

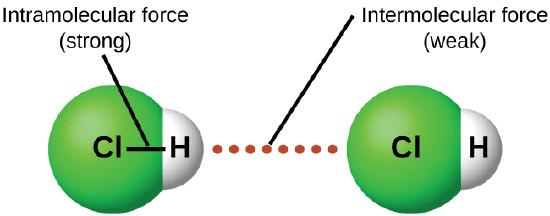
In contrast to intramolecular forces (see Figure 8.1.4), such as the covalent bonds that hold atoms together in molecules and polyatomic ions, intermolecular forces hold molecules together in a liquid or solid. Intermolecular forces are generally much weaker than covalent bonds. For example, it requires 927 kJ to overcome the intramolecular forces and break both O–H bonds in 1 mol of water, but it takes only about 41 kJ to overcome the intermolecular attractions and convert 1 mol of liquid water to water vapor at 100°C. (Despite this seemingly low value, the intermolecular forces in liquid water are among the strongest such forces known!) Given the large difference in the strengths of intra- and intermolecular forces, changes between the solid, liquid, and gaseous states almost invariably occur for molecular substances without breaking covalent bonds.

Figure \(\PageIndex{4}\): Intramolecular forces keep a molecule intact. Intermolecular forces hold multiple molecules together and determine many of a substance’s properties.

In this section, we will discuss the three types of IMF in molecular compounds: dipole-dipole, hydrogen bonding and London dispersion forces.

Dipole-dipole Intermolecular Forces

As discussed in Section 4.4, covalent bond that has an unequal sharing of electrons is called a polar covalent bond. (A covalent bond that has an equal sharing of electrons, as in a covalent bond with the same atom on each side, is called a nonpolar covalent bond.) A molecule with a net unequal distribution of electrons in its covalent bonds is a polar molecule. HF is an example of a polar molecule (see Figure 8.1.5).

Figure \(\PageIndex{5}\): Polar Covalent Bonds. The electrons in the HF molecule are not equally shared by the two atoms in the bond. Because the fluorine atom has nine protons in its nucleus, it attracts the negatively charged electrons in the bond more than the hydrogen atom does with its one proton in its nucleus. Thus, electrons are more strongly attracted to the fluorine atom, leading to an imbalance in the electron distribution between the atoms. The fluorine side of the bond picks up a partial overall negative charge (represented by the δ− in the diagram), while the hydrogen side of the bond has an overall partial positive charge (represented by the δ+ in the diagram). Such a bond is called a polar covalent bond.

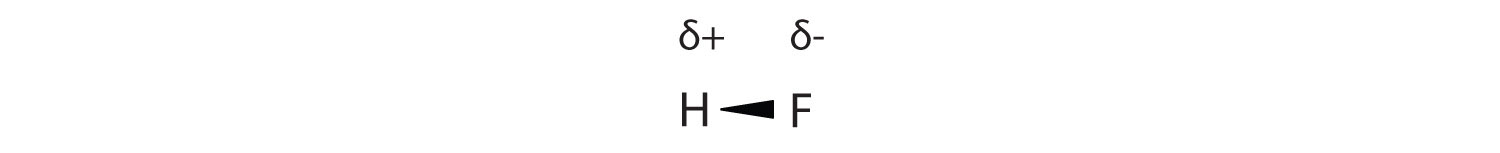
The charge separation in a polar covalent bond is not as extreme as is found in ionic compounds, but there is a related result: oppositely charged ends of different molecules will attract each other. This type of intermolecular interaction is called a dipole-dipole interaction. Many molecules with polar covalent bonds experience dipole-dipole interactions.

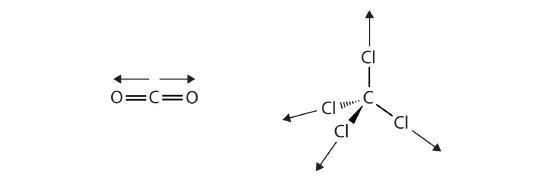
The covalent bonds in some molecules are oriented in space in such a way that the bonds in the molecules cancel each other out. The individual bonds are polar, but due to molecular symmetry, the overall molecule is not polar; rather, the molecule is nonpolar. Such molecules experience little or no dipole-dipole interactions. Carbon dioxide (CO₂) and carbon tetrachloride (CCl₄) are examples of such molecules (Figure \(\PageIndex{6}\)).

Figure \(\PageIndex{6}\): Nonpolar Molecules. Although the individual bonds in both CO₂ and CCl₄ are polar, their effects cancel out because of the spatial orientation of the bonds in each molecule. As a result, such molecules experience little or no dipole-dipole interaction.

Recall from the Sections 4.4 and 4.5, on chemical bonding and molecular geometry that polar molecules have a partial positive charge on one side and a partial negative charge on the other side of the molecule—a separation of charge called a dipole. Consider a polar molecule such as hydrogen chloride, HCl. In the HCl molecule, the more electronegative Cl atom bears the partial negative charge, whereas the less electronegative H atom bears the partial positive charge. An attractive force between HCl molecules results from the attraction between the positive end of one HCl molecule and the negative end of another. This attractive force is called a dipole-dipole attraction—the electrostatic force between the partially positive end of one polar molecule and the partially negative end of another, as illustrated in Figure \(\PageIndex{7}\).

Figure \(\PageIndex{7}\): This image shows two arrangements of polar molecules, such as HCl, that allow an attraction between the partial negative end of one molecule and the partial positive end of another.

Two pairs of molecules are shown where each molecule has one larger blue side labeled “delta sign, negative sign” and a smaller red side labeled “delta sign, positive sign. In the first pair, the red sides of the two molecules both face to the left and the blue side to the right. A horizontal dotted line lies in between the two. In the second pair, the molecules face up and down, with the red and blue ends aligning. A horizontal dotted line lies between the red and blue ends facing upward and another lies between the red and blue ends facing downward.

The effect of a dipole-dipole attraction is apparent when we compare the properties of HCl molecules to nonpolar F₂ molecules. Both HCl and F₂ consist of the same number of atoms and have approximately the same molecular mass. At a temperature of 150 K, molecules of both substances would have the same average kinetic energy. However, the dipole-dipole attractions between HCl molecules are sufficient to cause them to “stick together” to form a liquid, whereas the relatively weaker dispersion forces between nonpolar F₂ molecules are not, and so this substance is gaseous at this temperature. The higher normal boiling point of HCl (188 K) compared to F₂ (85 K) is a reflection of the greater strength of dipole-dipole attractions between HCl molecules, compared to the attractions between nonpolar F₂ molecules. We will often use values such as boiling or freezing points as indicators of the relative strengths of IMFs of attraction present within different substances.

Example \(\PageIndex{1}\)

Predict which will have the higher boiling point: N₂ or CO. Explain your reasoning.

Solution

CO and N₂ are both diatomic molecules with masses of about 28 amu, so they experience similar London dispersion forces. Because CO is a polar molecule, it experiences dipole-dipole attractions. Because N₂ is nonpolar, its molecules cannot exhibit dipole-dipole attractions. The dipole-dipole attractions between CO molecules are comparably stronger than the dispersion forces between nonpolar N₂ molecules, so CO is expected to have the higher boiling point.

Exercise \(\PageIndex{1}\)

Predict which will have the higher boiling point: \(\ce{ICl}\) or \(\ce{Br2}\). Explain your reasoning.

Answer

ICl. ICl and Br₂ have similar masses (~160 amu) and therefore experience similar London dispersion forces. ICl is polar and thus also exhibits dipole-dipole attractions; Br₂ is nonpolar and does not. The relatively stronger dipole-dipole attractions require more energy to overcome, so ICl will have the higher boiling point.

Hydrogen Bonding Intermolecular Forces

Molecules with hydrogen atoms bonded to electronegative atoms such as O, N, and F tend to exhibit unusually strong intermolecular interactions due to a particularly strong type of dipole-dipole attraction called hydrogen bonding. The very large difference in electronegativity between the H atom (2.1) and the atom to which it is bonded (4.0 for an F atom, 3.5 for an O atom, or 3.0 for a N atom), combined with the very small size of a H atom and the relatively small sizes of F, O, or N atoms, leads to highly concentrated partial charges with these atoms. Because the hydrogen atom does not have any electrons other than the ones in the covalent bond, its positively charged nucleus is almost completely exposed, allowing strong attractions to other nearby lone pairs of electrons.

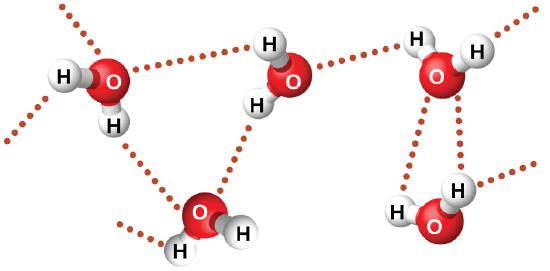
Examples of hydrogen bonds include HF⋯HF, H₂O⋯HOH, and H₃N⋯HNH₂, in which the hydrogen bonds are denoted by dots. Figure \(\PageIndex{8}\) illustrates hydrogen bonding between water molecules. The physical properties of water, which has two O–H bonds, are strongly affected by the presence of hydrogen bonding between water molecules. Most molecular compounds that have a mass similar to water are gases at room temperature. However, because of the strong hydrogen bonds, water molecules are able to stay condensed in the liquid state.

Figure \(\PageIndex{8}\): Water molecules participate in multiple hydrogen-bonding interactions with nearby water molecules.

Five water molecules are shown near one another, but not touching. A dotted line lies between many of the hydrogen atoms on one molecule and the oxygen atom on another molecule.

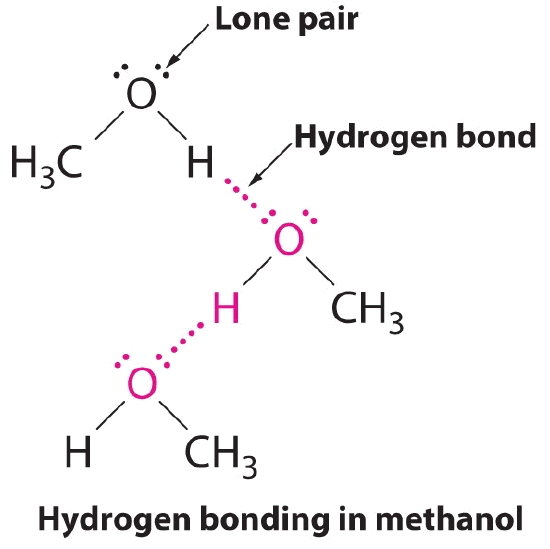
A hydrogen bond is an intermolecular attractive force in which a hydrogen atom, that is covalently bonded to a small, highly electronegative atom, is attracted to a lone pair of electrons on an atom in a neighboring molecule. Figure \(\PageIndex{9}\) shows how methanol (CH₃OH) molecules experience hydrogen bonding. Methanol contains both a hydrogen atom attached to O; methanol can thus form hydrogen bonds by acting as either a hydrogen bond donor or a hydrogen bond acceptor (lone pair). The hydrogen-bonded structure of methanol is as follows:

Figure \(\PageIndex{9}\): Methanol molecules participate in multiple hydrogen-bonding interactions with nearby water molecules.

Despite use of the word “bond,” keep in mind that hydrogen bonds are intermolecular attractive forces, not intramolecular attractive forces (covalent bonds). Hydrogen bonds are much weaker than covalent bonds, but are generally much stronger than other dipole-dipole attractions and dispersion forces.

Effect of Hydrogen Bonding on Boiling Points

Consider the compounds dimethylether (CH₃OCH₃), ethanol (CH₃CH₂OH), and propane (CH₃CH₂CH₃). Their boiling points, not necessarily in order, are −42.1 °C, −24.8 °C, and 78.4 °C. Match each compound with its boiling point. Explain your reasoning.

Solution

The shapes of CH₃OCH₃, CH₃CH₂OH, and CH₃CH₂CH₃ are similar, as are their molar masses (46 g/mol, 46 g/mol, and 44 g/mol, respectively), so they will exhibit similar dispersion forces. Since CH₃CH₂CH₃ is nonpolar, it may exhibit only dispersion forces. Because CH₃OCH₃ is polar, it will also experience dipole-dipole attractions. Finally, CH₃CH₂OH has an −OH group, and so it will experience the uniquely strong dipole-dipole attraction known as hydrogen bonding. So the ordering in terms of strength of IMFs, and thus boiling points, is CH₃CH₂CH₃ < CH₃OCH₃ < CH₃CH₂OH. The boiling point of propane is −42.1 °C, the boiling point of dimethylether is −24.8 °C, and the boiling point of ethanol is 78.5 °C.

Exercise \(\PageIndex{2}\)

Ethane (CH₃CH₃) has a melting point of −183 °C and a boiling point of −89 °C. Predict the melting and boiling points for methylamine (CH₃NH₂). Explain your reasoning.

Answer

The melting point and boiling point for methylamine are predicted to be significantly greater than those of ethane. CH₃CH₃ and CH₃NH₂ are similar in size and mass, but methylamine possesses an −NH group and therefore may exhibit hydrogen bonding. This greatly increases its IMFs, and therefore its melting and boiling points. It is difficult to predict values, but the known values are a melting point of −93 °C and a boiling point of −6 °C.

Hydrogen Bonding and DNA

Deoxyribonucleic acid (DNA) is found in every living organism and contains the genetic information that determines the organism’s characteristics, provides the blueprint for making the proteins necessary for life, and serves as a template to pass this information on to the organism’s offspring. A DNA molecule consists of two (anti-)parallel chains of repeating nucleotides, which form its well-known double helical structure, as shown in Figure \(\PageIndex{10}\).

Figure \(\PageIndex{10}\): Two separate DNA molecules form a double-stranded helix in which the molecules are held together via hydrogen bonding. (credit: modification of work by Jerome Walker, Dennis Myts)

Two images are shown. The first lies on the left side of the page and shows a helical structure like a twisted ladder where the rungs of the ladder, labeled “Base pair” are red, yellow, green and blue paired bars. The red and yellow bars, which are always paired together, are labeled in the legend, which is titled “Nitrogenous bases” as “adenine” and “thymine,” respectively. The blue and green bars, which are always paired together, are labeled in the legend as “guanine” and “cytosine,” respectively. At the top of the helical structure, the left-hand side rail, or “Sugar, dash, phosphate backbone,” is labeled as “3, prime” while the right is labeled as “5, prime.” These labels are reversed at the bottom of the helix. To the right of the page is a large Lewis structure. The top left corner of this structure, labeled “5, prime,” shows a phosphorus atom single bonded to three oxygen atoms, one of which has a superscripted negative charge, and double bonded to a fourth oxygen atom. One of the single bonded oxygen atoms is single bonded to the left corner of a five-membered ring with an oxygen atom at its top point and which is single bonded to an oxygen atom on the bottom left. This oxygen atom is single bonded to a phosphorus atom that is single bonded to two other hydrogen atoms and double bonded to a fourth oxygen atom. The lower left of these oxygen atoms is single bonded to another oxygen atom that is single bonded to a five-membered ring with an oxygen in the upper bonding site. The bottom left of this ring has a hydroxyl group attached to it while the upper right carbon is single bonded to a nitrogen atom that is part of a five-membered ring bonded to a six-membered ring. Both of these rings have points of unsaturation and nitrogen atoms bonded into their structures. On the right side of the six-membered ring are two single bonded amine groups and a double bonded oxygen. Three separate dotted lines extend from these sites to corresponding sites on a second six-membered ring. This ring has points of unsaturation and a nitrogen atom in the bottom right bonding position that is single bonded to a five-membered ring on the right side of the image. This ring is single bonded to a carbon that is single bonded to an oxygen that is single bonded to a phosphorus. The phosphorus is single bonded to two other oxygen atoms and double bonded to a fourth oxygen atom. This group is labeled “5, prime.” The five-membered ring is also bonded on the top side to an oxygen that is bonded to a phosphorus single bonded to two other oxygen atoms and double bonded to a fourth oxygen atom. The upper left oxygen of this group is single bonded to a carbon that is single bonded to a five-membered ring with an oxygen in the bottom bonding position. This ring has a hydroxyl group on its upper right side that is labeled “3, prime” and is bonded on the left side to a nitrogen that is a member of a five-membered ring. This ring is bonded to a six-membered ring and both have points of unsaturation. This ring has a nitrogen on the left side, as well as an amine group, that have two dotted lines leading from them to an oxygen and amine group on a six membered ring. These dotted lines are labeled “Hydrogen bonds.” The six membered ring also has a double bonded oxygen on its lower side and a nitrogen atom on its left side that is single bonded to a five-membered ring. This ring connects to the two phosphate groups mentioned at the start of this to form a large circle. The name “guanine” is written below the lower left side of this image while the name “cytosine” is written on the lower right. The name “thymine” is written above the right side of the image and “adenine” is written on the top right. Three sections are indicated below the images where the left is labeled “Sugar, dash, phosphate backbone,” the middle is labeled “Bases” and the right is labeled “Sugar, dash, phosphate backbone.”

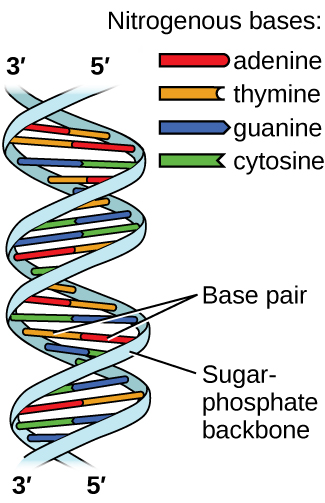
Each nucleotide contains a (deoxyribose) sugar bound to a phosphate group on one side, and one of four nitrogenous bases on the other. Two of the bases, cytosine (C) and thymine (T), are single-ringed structures known as pyrimidines. The other two, adenine (A) and guanine (G), are double-ringed structures called purines. These bases form complementary base pairs consisting of one purine and one pyrimidine, with adenine pairing with thymine, and cytosine with guanine. Each base pair is held together by hydrogen bonding. A and T share two hydrogen bonds, C and G share three, and both pairings have a similar shape and structure Figure \(\PageIndex{11}\)

Figure \(\PageIndex{11}\): The geometries of the base molecules result in maximum hydrogen bonding between adenine and thymine (AT) and between guanine and cytosine (GC), so-called “complementary base pairs.”

A large Lewis structure is shown. The top left corner of this structure, labeled “5, prime,” shows a phosphorus atom single bonded to three oxygen atoms, one of which has a superscripted negative charge, and double bonded to a fourth oxygen atom. One of the single bonded oxygen atoms is single bonded to the left corner of a five-membered ring with an oxygen atom at its top point and which is single bonded to an oxygen atom on the bottom left. This oxygen atom is single bonded to a phosphorus atom that is single bonded to two other hydrogen atoms and double bonded to a fourth oxygen atom. The lower left of these oxygen atoms is single bonded to another oxygen atom that is single bonded to a five-membered ring with an oxygen in the upper bonding site. The bottom left of this ring has a hydroxyl group attached to it while the upper right carbon is single bonded to a nitrogen atom that is part of a five-membered ring bonded to a six-membered ring. Both of these rings have points of unsaturation and nitrogen atoms bonded into their structures. On the right side of the six-membered ring are two single bonded amine groups and a double bonded oxygen. Three separate dotted lines extend from these sites to corresponding sites on a second six-membered ring. This ring has points of unsaturation and a nitrogen atom in the bottom right bonding position that is single bonded to a five-membered ring on the right side of the image. This ring is single bonded to a carbon that is single bonded to an oxygen that is single bonded to a phosphorus. The phosphorus is single bonded to two other oxygen atoms and double bonded to a fourth oxygen atom. This group is labeled “5, prime.” The five-membered ring is also bonded on the top side to an oxygen that is bonded to a phosphorus single bonded to two other oxygen atoms and double bonded to a fourth oxygen atom. The upper left oxygen of this group is single bonded to a carbon that is single bonded to a five-membered ring with an oxygen in the bottom bonding position. This ring has a hydroxyl group on its upper right side that is labeled “3, prime” and is bonded on the left side to a nitrogen that is a member of a five-membered ring. This ring is bonded to a six-membered ring and both have points of unsaturation. This ring has a nitrogen on the left side, as well as an amine group, that have two dotted lines leading from them to an oxygen and amine group on a six membered ring. These dotted lines are labeled “Hydrogen bonds.” The six membered ring also has a double bonded oxygen on its lower side and a nitrogen atom on its left side that is single bonded to a five-membered ring. This ring connects to the two phosphate groups mentioned at the start of this to form a large circle. The name “guanine” is written below the lower left side of this image while the name “cytosine” is written on the lower right. The name “thymine” is written above the right side of the image and “adenine” is written on the top right. Three sections are indicated below the images where the left is labeled “Sugar, dash, phosphate backbone,” the middle is labeled “Bases” and the right is labeled “Sugar, dash, phosphate backbone.”

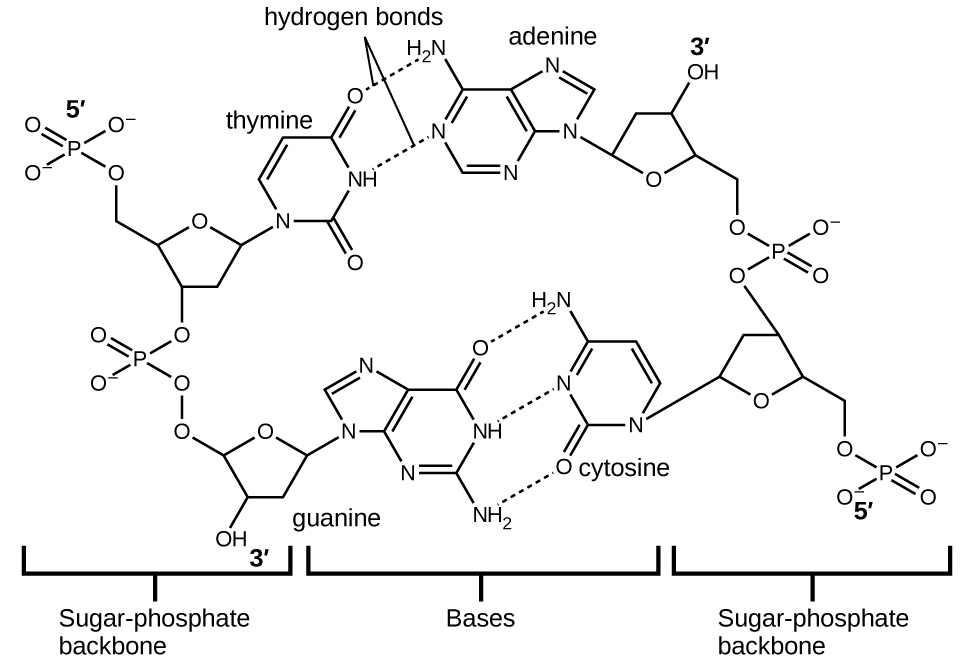
The cumulative effect of millions of hydrogen bonds effectively holds the two strands of DNA together. Importantly, the two strands of DNA can relatively easily “unzip” down the middle since hydrogen bonds are relatively weak compared to the covalent bonds that hold the atoms of the individual DNA molecules together. This allows both strands to function as a template for replication.

London Dispersion Forces

Finally, there are forces between all molecules that are caused by electrons being in different places in a molecule at any one time, which sets up a temporary separation of charge that disappears almost as soon as it appears. These are very weak intermolecular interactions and are called dispersion forces (or London forces). (An alternate name is London dispersion forces.) Molecules that experience no other type of intermolecular interaction will at least experience dispersion forces. Substances that experience only dispersion forces are typically soft in the solid phase and have relatively low melting points. Examples include waxes, which are long hydrocarbon chains that are solids at room temperature because the molecules have so many electrons. The resulting dispersion forces between these molecules make them assume the solid phase at normal temperatures.

Dispersion forces that develop between atoms in different molecules can attract the two molecules to each other. The forces are relatively weak, however, and become significant only when the molecules are very close. Larger and heavier atoms and molecules exhibit stronger dispersion forces than do smaller and lighter atoms and molecules. F₂ and Cl₂ are gases at room temperature (reflecting weaker attractive forces); Br₂ is a liquid, and I₂ is a solid (reflecting stronger attractive forces). Trends in observed melting and boiling points for the halogens clearly demonstrate this effect, as seen in Table 8.1.2.

Table \(\PageIndex{2}\): Melting and Boiling Points of the Halogens

Halogen	Molar Mass	Atomic Radius	Melting Point	Boiling Point
fluorine, F2	38 g/mol	72 pm	53 K	85 K
chlorine, Cl2	71 g/mol	99 pm	172 K	238 K
bromine, Br2	160 g/mol	114 pm	266 K	332 K
iodine, I2	254 g/mol	133 pm	387 K	457 K
astatine, At2	420 g/mol	150 pm	575 K	610 K

The increase in melting and boiling points with increasing atomic/molecular size may be rationalized by considering how the strength of dispersion forces is affected by the electronic structure of the atoms or molecules in the substance. In a larger atom, the valence electrons are, on average, farther from the nuclei than in a smaller atom. Thus, they are less tightly held and can more easily form the temporary dipoles that produce the attraction. The measure of how easy or difficult it is for another electrostatic charge (for example, a nearby ion or polar molecule) to distort a molecule’s charge distribution (its electron cloud) is known as polarizability. A molecule that has a charge cloud that is easily distorted is said to be very polarizable and will have large dispersion forces; one with a charge cloud that is difficult to distort is not very polarizable and will have small dispersion forces.

Example \(\PageIndex{3}\)

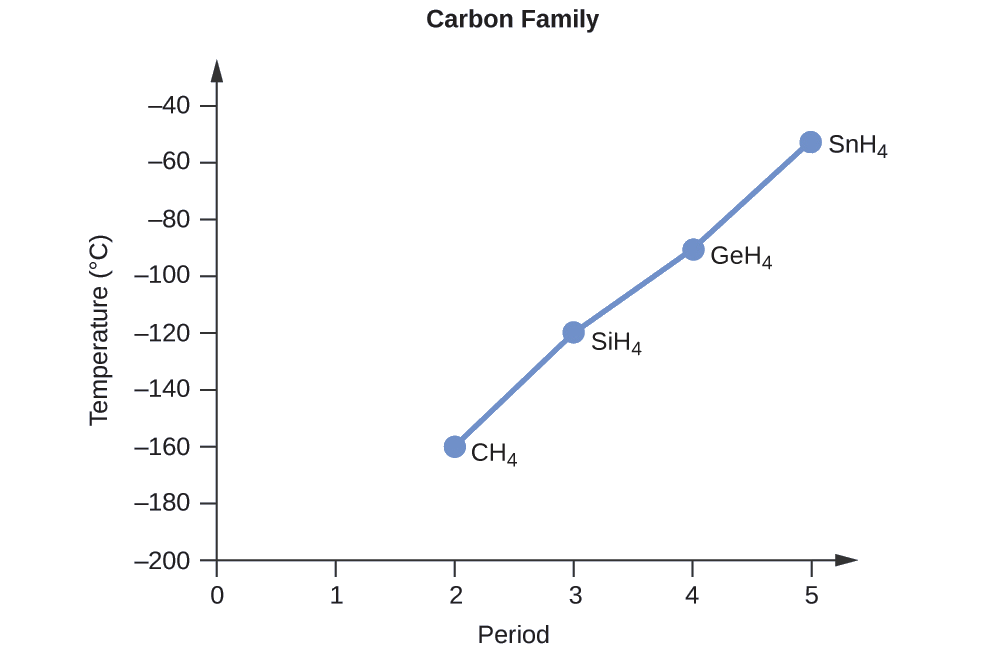
Order the following compounds of a group 14 element and hydrogen from lowest to highest boiling point: CH₄, SiH₄, GeH₄, and SnH₄. Explain your reasoning.

Solution

Applying the skills acquired in the chapter on chemical bonding and molecular geometry, all of these compounds are predicted to be nonpolar, so they may experience only dispersion forces: the smaller the molecule, the less polarizable and the weaker the dispersion forces; the larger the molecule, the larger the dispersion forces. The molar masses of CH₄, SiH₄, GeH₄, and SnH₄ are approximately 16 g/mol, 32 g/mol, 77 g/mol, and 123 g/mol, respectively. Therefore, CH₄ is expected to have the lowest boiling point and SnH₄ the highest boiling point. The ordering from lowest to highest boiling point is expected to be

CH₄ < SiH₄ < GeH₄ < SnH₄

A graph of the actual boiling points of these compounds versus the period of the group 14 elementsshows this prediction to be correct:

 

A line graph, titled “Carbon Family,” is shown where the y-axis is labeled “Temperature, ( degree sign C )” and has values of “negative 200” to “negative 40” from bottom to top in increments of 20. The x-axis is labeled “Period” and has values of “0” to “5” in increments of 1. The first point on the graph is labeled “C H subscript 4” and is at point “2, negative 160.” The second point on the graph is labeled “S i H subscript 4” and is at point “3, negative 120” while the third point on the graph is labeled “G e H subscript 4” and is at point “4, negative 100.” The fourth point on the graph is labeled “S n H subscript 4” and is at point “5, negative 60.”

Exercise \(\PageIndex{3}\)

Order the following hydrocarbons from lowest to highest boiling point: C₂H₆, C₃H₈, and C₄H₁₀.

Answer

All of these compounds are nonpolar and only have London dispersion forces: the larger the molecule, the larger the dispersion forces and the higher the boiling point. The ordering from lowest to highest boiling point is therefore

C₂H₆ < C₃H₈ < C₄H₁₀.

Applications: Geckos and Intermolecular Forces

Geckos have an amazing ability to adhere to most surfaces. They can quickly run up smooth walls and across ceilings that have no toe-holds, and they do this without having suction cups or a sticky substance on their toes. And while a gecko can lift its feet easily as it walks along a surface, if you attempt to pick it up, it sticks to the surface. How are geckos (as well as spiders and some other insects) able to do this? Although this phenomenon has been investigated for hundreds of years, scientists only recently uncovered the details of the process that allows geckos’ feet to behave this way.

Figure \(\PageIndex{12}\): Geckos’ toes contain large numbers of tiny hairs (setae), which branch into many triangular tips (spatulae). Geckos adhere to surfaces because of van der Waals attractions between the surface and a gecko’s millions of spatulae. By changing how the spatulae contact the surface, geckos can turn their stickiness “on” and “off.” (credit photo: modification of work by “JC*+A!”/Flickr)

Three figures are shown. The first is a photo of the bottom of a gecko’s foot. The second is bigger version which shows the setae. The third is a bigger version of the setae and shows the spatulae.

Geckos’ toes are covered with hundreds of thousands of tiny hairs known as setae, with each seta, in turn, branching into hundreds of tiny, flat, triangular tips called spatulae. The huge numbers of spatulae on its setae provide a gecko, shown in Figure 8.1.12, with a large total surface area for sticking to a surface. In 2000, Kellar Autumn, who leads a multi-institutional gecko research team, found that geckos adhered equally well to both polar silicon dioxide and nonpolar gallium arsenide. This proved that geckos stick to surfaces because of dispersion forces—weak intermolecular attractions arising from temporary, synchronized charge distributions between adjacent molecules. Although dispersion forces are very weak, the total attraction over millions of spatulae is large enough to support many times the gecko’s weight.

In 2014, two scientists developed a model to explain how geckos can rapidly transition from “sticky” to “non-sticky.” Alex Greaney and Congcong Hu at Oregon State University described how geckos can achieve this by changing the angle between their spatulae and the surface. Geckos’ feet, which are normally nonsticky, become sticky when a small shear force is applied. By curling and uncurling their toes, geckos can alternate between sticking and unsticking from a surface, and thus easily move across it. Further investigations may eventually lead to the development of better adhesives and other applications.

Boiling Points and Bonding Types

In order for a substance to enter the gas phase, its particles must completely overcome the intermolecular forces holding them together. Therefore, a comparison of boiling points is essentially equivalent to comparing the strengths of the attractive intermolecular forces exhibited by the individual molecules. For small molecular compounds, London dispersion forces are the weakest intermolecular forces. Dipole-dipole forces are somewhat stronger, and hydrogen bonding is a particularly strong form of dipole-dipole interaction. However, when the mass of a nonpolar molecule is sufficiently large, its dispersion forces can be stronger than the dipole-dipole forces in a lighter polar molecule. Thus, nonpolar Cl₂ has a higher boiling point than polar HCl.

Table \(\PageIndex{3}\): Intermolecular Forces and Boiling Points

Substance	Strongest Intermolecular Force	Boiling Point \(\left( ^\text{o} \text{C} \right)\)
\(\ce{H_2}\)	dispersion	-253
\(\ce{Ne}\)	dispersion	-246
\(\ce{O_2}\)	dispersion	-183
\(\ce{Cl_2}\)	dispersion	-34
\(\ce{HCl}\)	dipole-dipole	-85
\(\ce{HBr}\)	dipole-dipole	-66
\(\ce{H_2S}\)	dipole-dipole	-61
\(\ce{NH_3}\)	hydrogen bonding	-33
\(\ce{HF}\)	hydrogen bonding	20
\(\ce{H_2O}\)	hydrogen bonding	100

Example \(\PageIndex{4}\): Intermolecular Forces

What intermolecular forces besides dispersion forces, if any, exist in each substance? Are any of these substances solids at room temperature?

1. potassium chloride (KCl)
2. ethanol (C₂H₅OH)
3. bromine (Br₂)

Solution

1. Potassium chloride is composed of ions, so the intermolecular interaction in potassium chloride is ionic forces. Because ionic interactions are strong, it might be expected that potassium chloride is a solid at room temperature.
2. Ethanol has a hydrogen atom attached to an oxygen atom, so it would experience hydrogen bonding. If the hydrogen bonding is strong enough, ethanol might be a solid at room temperature, but it is difficult to know for certain. (Ethanol is actually a liquid at room temperature.)
3. Elemental bromine has two bromine atoms covalently bonded to each other. Because the atoms on either side of the covalent bond are the same, the electrons in the covalent bond are shared equally, and the bond is a nonpolar covalent bond. Thus, diatomic bromine does not have any intermolecular forces other than dispersion forces. It is unlikely to be a solid at room temperature unless the dispersion forces are strong enough. Bromine is a liquid at room temperature.

Exercise \(\PageIndex{4}\)

What intermolecular forces besides dispersion forces, if any, exist in each substance? Are any of these substances solids at room temperature?

1. methylamine (CH₃NH₂)
2. calcium sulfate (CaSO₄)
3. carbon monoxide (CO)

Answer

a. dipole-dipole, hydrogen bonding

b. ionic forces (solid at room temperature)

c. dipole-dipole

Key Takeaways

• A phase is a form of matter that has the same physical properties throughout.
• Molecules interact with each other through various forces: dipole-dipole interactions, hydrogen bonding, and dispersion forces.
• Dipole-dipole attractions result from the electrostatic attraction of the partial negative end of one dipolar molecule for the partial positive end of another.
• Hydrogen bonds are a special type of dipole-dipole attraction that results when hydrogen is bonded to one of the three most electronegative elements: F, O, or N.
• The temporary dipole that results from the motion of the electrons in an atom can induce a dipole in an adjacent atom and give rise to the London dispersion force. London forces increase with increasing molecular size.