7.1: Kinetic Molecular Theory: A Model for Gases
- Page ID
- 66801
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
Skills to Develop
- Compare the properties of gases, liquids, and solids.
- Convert between units of volume, pressure, and temperature.
- State the relationship between temperature and kinetic energy.
The Kinetic Molecular Theory allows us to explain the existence of the three phases of matter: solid, liquid, and gas. In addition, it helps explain the physical characteristics of each phase and how phases change from one to another. The Kinetic Molecular Theory is essential for the explanations of gas pressure, compressibility, diffusion, and mixing. Our explanations for reaction rates and equilibrium also rest on the concepts of the Kinetic Molecular Theory.
Approximately \(20\%\) of the atmosphere is oxygen. This gas is essential for life. In environments where oxygen is in low supply, it can be provided from a tank. Since gases are very compressible, a large amount of oxygen can be stored in a relatively small container. When it is released, the volume expands and the pressure decreases. The gas is then available for breathing under normal pressure.
Kinetic-Molecular Theory
The kinetic-molecular theory is a theory that explains the states of matter and is based on the idea that matter is composed of tiny particles that are always in motion. The theory helps explain observable properties and behaviors of solids, liquids, and gases. However, the theory is most easily understood as it applies to gases and it is with gases that we will begin our detailed study. The theory applies specifically to a model of gas called an ideal gas. An ideal gas is an imaginary gas whose behavior perfectly fits all the assumptions of the kinetic-molecular theory. In reality, gases are not ideal, but are very close to being so under most everyday conditions.
The kinetic-molecular theory as it applies to gases has five basic assumptions.
- Gases consist of very large numbers of tiny spherical particles that are far apart from one another compared to their size. The particles of a gas may be either atoms or molecules. The distance between the particles of a gas is much, much greater than the distances between the particles of a liquid or a solid. Most of the volume of a gas, therefore, is composed of the empty space between the particles. In fact, the volume of the particles themselves is considered to be insignificant compared to the volume of the empty space.
- Gas particles are in constant rapid motion in random directions. The fast motion of gas particles gives them a relatively large amount of kinetic energy. Recall that kinetic energy is the energy that an object possesses because of its motion. The particles of a gas move in straight-line motion until they collide with another particle or with one of the walls of its container.
- Collisions between gas particles and between particles and the container walls are elastic collisions. An elastic collision is one in which there is no overall loss of kinetic energy. Kinetic energy may be transferred from one particle to another during an elastic collision, but there is no change in the total energy of the colliding particles.
- There are no forces of attraction or repulsion between gas particles. Attractive forces are responsible for particles of a real gas condensing together to form a liquid. It is assumed that the particles of an ideal gas have no such attractive forces. The motion of each particle is completely independent of the motion of all other particles.
- The average kinetic energy of gas particles is dependent upon the temperature of the gas. As the temperature of a sample of gas is increased, the speeds of the particles are increased. This results in an increase in the kinetic energy of the particles. Not all particles of gas in a sample have the same speed and so they do not have the same kinetic energy. The temperature of a gas is proportional to the average kinetic energy of the gas particles.
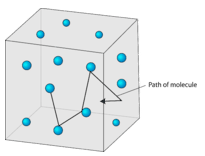
Figure 13.1.1: Gas particles are in random straight-line motion according to the kinetic-molecular theory. The space between particles is very large compared to the particle size.
Summary
- Assumptions of the kinetic-molecular theory:
- Gases consist of very large numbers of tiny spherical particles that are far apart from one another compared to their size.
- Gas particles are in constant rapid motion in random directions.
- Collisions between gas particles and between particles and the container walls are elastic collisions.
- There are no forces of attraction or repulsion between gas particles.
- The average kinetic energy of gas particles is dependent upon the temperature of the gas.
Contributors
Gases are tremendously compressible, can exert massive pressures, expand nearly instantaneously into a vacuum, and fill every container they are placed in regardless of size. All of these properties of gases are due to their molecular arrangement.
Volume of Gases
In dealing with gases, we lose the meaning of the word "full". A glass of water may be 1/4 full or 1/2 full, but a container containing a gaseous substance is always full. The same amount of gas will fill a quart jar, or a gallon jug, a barrel, or a house. The gas molecules separate farther from each other and spread out uniformly until they fill whatever container they are in. Gases can be compressed to small fractions of their original volume and expand to fill virtually any volume. If gas molecules are pushed together to the point that they touch, the substance would then be in the liquid form. One method of converting a gas to a liquid is to cool it and another method is to compress it.
The two most common ways of expressing volume are using \(\text{mL}\) and \(\text{L}\). You will need to be able to convert between these two units. The relationship is as follows:
\[1000 \: \text{mL} = 1 \: \text{L}\]
Pressure of Gases
The constant random motion of the gas molecules causes them to collide with each other and with the walls of their container. These collisions of gas molecules with their surroundings exert a pressure on the surroundings. When you blow up a balloon, the air particles inside the balloon push against the elastic sides, the walls of the balloon are pushed outward and kept firm. This pressure is produced by air molecules pounding on the inside walls of the balloon.
There are three units of pressure commonly used in chemistry. Pressure is commonly measured on a device called a monometer, similar to the barometer which a meteorologist uses. Pressures in monometers are typically recorded in units of millimeters of mercury, abbreviated \(\text{mm} \: \ce{Hg}\). Pressure is defined as the force exerted divided by the area over which the force is exerted.
\[\text{pressure} = \frac{\text{force}}{\text{area}}\]
The air molecules in our atmosphere exert pressure on every surface that is in contact with air. The air pressure of our atmosphere at sea level is approximately \(15 \: \text{lbs/in}^2\). This pressure is unnoticed, because the air is not only outside the surfaces but also inside allowing the atmospheric air pressure to be balanced. The pressure exerted by our atmosphere will become quickly noticed, however, if the air is removed or reduced inside an object. A common demonstration of air pressure makes use of a one-gallon metal can. The can has a few drops of water placed inside and is then heated to boiling. The water inside the can vaporizes and expands to fill the can, pushing the air out. The lid is then tightly sealed on the can. As the can cools, the water vapor inside condenses back to liquid water leaving the inside of the an with a lack of air molecules. As the water vapor condenses to liquid water, the air pressure outside the can slowly crushes the can flat.
People, of course also have atmospheric pressure pressing on them. An average sized person probably has a total force exerted on them from the atmosphere in excess of 25,000 pounds. Fortunately, people also have air inside them to balance the force. A device to measure atmospheric pressure, the barometer, was invented in 1643 by an Italian scientist named Evangelista Torricelli (1608 - 1647) who had been a student of Galileo. Torricelli's barometer was constructed by filling a glass tube, open at one end and closed at the other, with liquid mercury and then inverting the tube in a dish of mercury.
The mercury in the tube fell to a height such that the difference between the surface of the mercury in the dish and the top of the mercury column in the tube was 760 millimeters. The volume of empty space above the mercury in the tube was a vacuum. The explanation for why the mercury stays in the tube is that there are no air molecules pounding on the top of the mercury in the tube. The weight of the mercury in the tube divided by the area of the opening in the tube is exactly equal to the atmospheric pressure.
The height to which the mercury is held would only be 760 millimeters when air pressure is normal and at sea level. The atmospheric pressure changes due to weather conditions and the height of the mercury in the barometer will change with it. Atmospheric pressure also varies with altitude. Higher altitudes have lower air pressure because the air is "thinner" - fewer air molecules per unit volume. In the mountains, at an altitude of 9600 feet, the normal atmospheric pressure will only support a mercury column of \(520 \: \text{mm} \: \ce{Hg}\).
For various reasons, chemistry has many different units for measuring and expressing gas pressure. You will need to be familiar with most of them so you can convert them into preferred units. Because instruments for measuring pressure often contain a column of mercury, the most commonly used units for pressure are based on the height of the mercury column that the gas can support. The original unit in chemistry for gas pressure was \(\text{mm} \: \ce{Hg}\) (millimeters of mercury). Standard atmospheric pressure at sea level is \(760 \: \text{mm} \: \ce{Hg}\). This unit is something of a problem because while it is a pressure unit, it looks a lot like a length unit. Students, in particular, occasionally leave off the \(\ce{Hg}\) and then it definitely appears to be a length unit. To eliminate this problem, the unit was given another name. It was called the \(\text{torr}\) in honor of Torricelli. \(760 \: \text{torr}\) is exactly the same as \(760 \: \text{mm} \: \ce{Hg}\). For certain work, it became convenient to express gas pressure in terms of multiples of normal atmospheric pressure at sea level and so the unit atmosphere \(\left( \text{atm} \right)\) was introduced. The conversion you need to know between various pressure units are:
\[1.00 \: \text{atm} = 760 \: \text{mm} \: \ce{Hg} = 760 \: \text{torr}\]
Example 11.1.1
Convert \(425 \: \text{mm} \: \ce{Hg}\) to \(\text{atm}\).
Solution:
The conversion factor is \(760 \: \text{mm} \: \ce{Hg} = 1.00 \: \text{atm}\)
\[425 \: \text{mm} \: \ce{Hg} \times \frac{1 \: \text{atm}}{760 \: \text{mm} \: \ce{Hg}} = 0.559 \: \text{atm}\]
This example shows how to perform this conversion using dimensional analysis. If you are memorizing type, you can just memorize that to convert from \(\text{mm} \: \ce{Hg}\) to \(\text{atm}\) you must divide by 760.
Gas Temperature and Kinetic Energy
Kinetic energy is the energy of motion and therefore, all moving objects have kinetic energy. The mathematical formula for calculating the kinetic energy of an object is \(KE = \frac{1}{2} mv^2\), where \(m\) is the mass and \(v\) is the velocity of the object or particle. This physics formula applies to all objects in exactly the same way whether we are talking about the moon moving in its orbit, a baseball flying toward home plate, or a gas molecule banging around in a bottle. All of these objects have kinetic energy and their kinetic energies can all be calculated with the same formula. The kinetic energy of a molecule would be calculated in exactly this same way. You should note that if the mass of an object is doubled while its velocity remains the same, the kinetic energy of the object would also be doubled. If, on the other hand, the velocity is doubled while the mass remains the same, the kinetic energy would be quadrupled because of the square in the formula.
When you measure the temperature of a group of molecules, what you are actually measuring is their average kinetic energy. They are the same thing but expressed in different units. The formula for this relationship is \(KE_\text{avg} = \frac{3}{2}RT\) where \(R\) is the gas constant and \(T\) is the absolute temperature, measured in Kelvin. When a substance is heated, the average kinetic energy of the molecules is increased. Since the mass of the molecules cannot be increased by heating, it is clear that the velocity of the molecules in increasing.
Remember, the motion of molecules is related to their temperature. If you think of the average kinetic energy of a group of molecules and temperature measured in degrees Kelvin, the relationship is a direct proportion. That means that if the temperature, in Kelvin, is doubled the kinetic energy of the particles is also doubled. It is absolutely vital that you keep in mind that the mathematical relationship between the temperature and the average kinetic energy of molecules only exists when the temperature is expressed in the Kelvin scale. In order for the direct proportion to exist, the molecules must have zero kinetic energy when the temperature is zero. The temperature at which molecular motion stops is \(0 \: \text{K}\) \(\left( -273^\text{o} \text{C} \right)\). It is surely apparent to you that molecules do NOT have zero kinetic energy at \(0^\text{o} \text{C}\). Balloons and automobile tires to not go flat when the outside temperature reaches \(0^\text{o} \text{C}\). If temperature is measured in Kelvin degrees, then the average kinetic energy of a substance at \(100 \: \text{K}\) is exactly double the average kinetic energy of a substance at \(50 \: \text{K}\). Make sure all the calculations you do dealing with the kinetic energy of molecules is done with Kelvin temperatures.
Some important principles can be derived from this relationship:
1. All gases at the same temperature have the same kinetic energy.
2. Heavier gases must move more slowly in order to have the same kinetic energy as lighter gases.
Example 11.1.2
If molecules of \(\ce{H_2}\), \(\ce{O_2}\), and \(\ce{N_2}\) are all placed in the same container at the same temperature, which molecules will have the greatest velocity?
Solution:
Because they are at the same temperature, they will have the same energy. However, lighter particles must move faster in order to have the same kinetic energy. We must, therefore, look at their masses. Use your periodic table:
Mass of \(\ce{H_2} = 2 \left( 1.008 \: \text{g/mol} \right) = 2.016 \: \text{g/mol}\)
Mass of \(\ce{O_2} = 2 \left( 16.00 \: \text{g/mol} \right) = 32.00 \: \text{g/mol}\)
Mass of \(\ce{N_2} = 2 \left( 14.01 \: \text{g/mol} \right) = 28.02 \: \text{g/mol}\)
Because \(\ce{H_2}\) is the lightest, it must have the greatest velocity in order to have the same energy (the same temperature) as the other gases.
Summary
The collisions between molecules are perfectly elastic. The phrase "perfectly elastic collision" comes from physics and means that kinetic energy is conserved in collisions. The molecules of an ideal gas have no attraction or repulsion for each other. At any given moment, the molecules of a gas have different kinetic energies. We deal with this variation by considering the average kinetic energy of the molecules. The average kinetic energy of a group of molecules is measured by temperature. Molecules of a gas are so far apart, on average, that the volume of the molecules themselves is negligible compared to the volume of the gas.
Molecular collisions with container walls cause the gas to exert pressure. Because of the molecular motion of molecules, they possess kinetic energy at all temperatures above absolute zero. Temperature is directly proportional to the average kinetic energy of gas molecules. Lighter gases will have higher velocities than heavier gases, at the same temperature and pressure. In the Kelvin scale, \(0 \: \text{K}\) means the particles have no kinetic energy. Doubling the temperature in Kelvin doubles the kinetic energy of particles. Real gases tend to deviate from ideal gases at high pressures and low temperatures, as the attractive forces between molecules and the volume of gas molecules becomes significant.
Vocabulary
- Kelvin temperature: The absolute temperature scale where \(0 \: \text{K}\) is the theoretical absence of all thermal energy (no molecular motion).
- Kinetic energy: Kinetic energy is the energy a body possesses due to its motion, \(KE = \frac{1}{2} mv^2\).
- Kinetic theory: Used to explain the properties of gases.
- Pressure: A measure of the force with which gas particles collide with the walls of their containers.
- Temperature: A measurement of the kinetic energy of particles.

